Vector notation

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Types of quadrilaterals Algebraic notation Parallel lines

This topic is relevant for:

GCSE Maths Geometry and Measure Vectors

Vector Notation

Here we will learn about vector notation, including what vectors are and how we use notation in mathematics to write about them.

There are also vector worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is vector notation?

Vector notation is how we write vectors in mathematics.

A vector is a quantity which has both magnitude and direction. It can be used to show a movement.

We can write vectors in several ways:

Using an arrow,
Using boldface
Underlined.

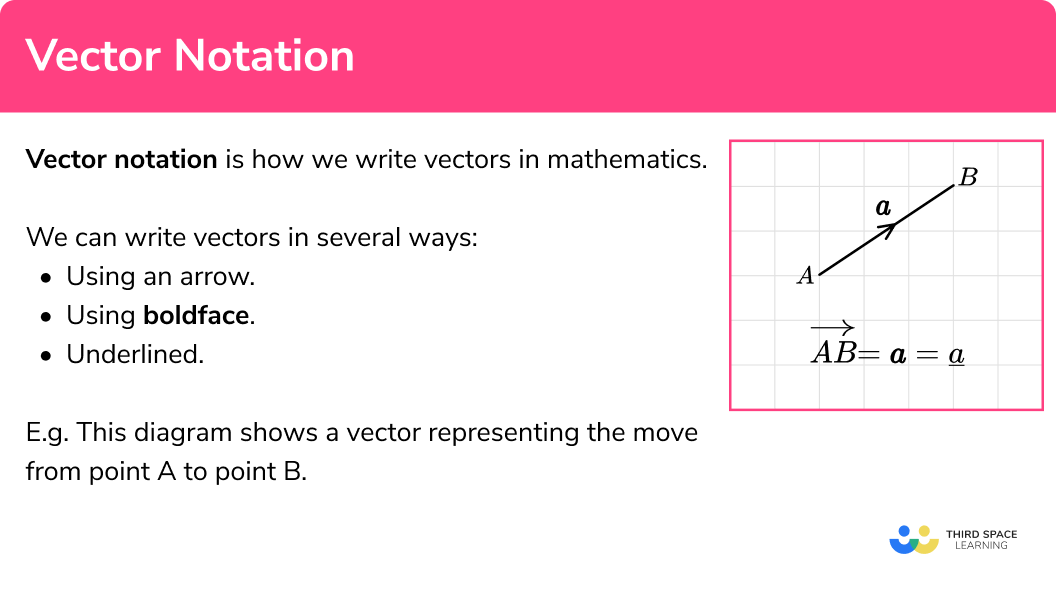
E.g.

\overrightarrow{AB} =\textbf{a}=\underline{a}

This diagram shows a vector representing the move from point A to point B.

Parallel vectors with the same direction and the same length are the same (equivalent):

E.g.

Each of these all represent the same vector $\textbf{a}$ .

Parallel vectors of the same length with opposite directions are called negative vectors:

E.g.

A vector representing the move from point B to point A would be in the opposite direction, but have the same length.

\overrightarrow{BA}=-\textbf{a}=-\underline{a}

A vector can be multiplied by a scalar to change the length of it:

The length is also referred to as the magnitude of the vector

E.g.

Vectors can also be added together:

E.g.

This diagram shows how to get from point A to point C, going via point B.

Vector $\textbf{a}$ is added to vector $\textbf{b}$ .

\begin{aligned} \overrightarrow{AC}&=\overrightarrow{AB}+\overrightarrow{BC}\\\\ &= \ \textbf{a} \ +\ \textbf{b}\\\\ \text{or handwritten as}\\\\ &=\ \underline{a} \ +\ \underline{b} \end{aligned}

Adding a negative vector becomes a subtraction.

\begin{aligned} &\textbf{a} \ + \ - \ \textbf{b}=\textbf{a} \ - \ \textbf{b}\\\\ &\text{or handwritten as}\\\\ &\underline{a} \ + \ - \ \underline{b} = \underline{a} \ - \ \underline{b} \end{aligned}

What is vector notation?

How to use vector notation

In order to use vector notation:

Check the starting point and the end point.
Decide the route.
Write the vector.
Simplify your answer.

How to use vector notation

Vectors worksheet (includes vector notation)

Get your free vector notation worksheet of 20+ vectors questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Vectors worksheet (includes vector notation)

Get your free vector notation worksheet of 20+ vectors questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on vectors

Vector notation is part of our series of lessons to support revision on vectors. You may find it helpful to start with the main vectors lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Vector notation examples

Example 1: using vector notation

Write the vector $\overrightarrow{AO}$ in terms of $\textbf{a}$ and $\textbf{b}$

Check the starting point and the end point.

\overrightarrow{AO}

The vector starts at point A and ends at point O.

2Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors.

Here we travel in the opposite direction to vector $\textbf{a}$ .

3Write the vector.

Write the route from point A to point O.

\overrightarrow{AO}=-\textbf{a}=-\underline{a}

Example 2: using vector notation

Write the vector $\overrightarrow{AC}$ in terms of $\textbf{a}$ and $\textbf{b}$

Check the starting point and the end point.

\overrightarrow{AC}

The vector starts at point A and ends at point C.

Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors.

Write the vector.

Write the route from point A to point C.

$\begin{aligned} \overrightarrow{AC}&=\overrightarrow{AB}+\overrightarrow{BC}\\\\ &=\ \textbf{a} \ +\ \textbf{b}\\\\ \text{or handwritten as}\\\\ &=\ \underline{a} \ +\ \underline{b} \end{aligned}$

Example 3: using vector notation

Write the vector $\overrightarrow{BA}$ in terms of $\textbf{a}$ and $\textbf{b}$

Check the starting point and the end point.

\overrightarrow{BA}

The vector starts at point B and ends at point A.

Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors. We can not go directly from point B to point A because there is no vector. We have to go via point C. As we need to go backwards along vector $\textbf{b}$ , we need a negative vector.

Write the vector.

Write the route from point B to point A.

$\begin{aligned} \overrightarrow{BA}&=\overrightarrow{BO}+\overrightarrow{OA}\\\\ &=\ -\textbf{b} \ +\ \textbf{a}\\ \text{or handwritten as}\\\\ &=\ -\underline{b} \ +\ \underline{a} \end{aligned}$

Alternatively, the final answer could be written as:

$\overrightarrow{BA}=\textbf{a}-\textbf{b}=\underline{a}-\underline{b}$

Example 4: leaving the answer in its simplest form

Write the vector $\overrightarrow{BC}$ in terms of $\textbf{a}$ and $\textbf{b}$

Check the starting point and the end point.

\overrightarrow{BC}

The vector starts at point B and ends at point C.

Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors. We can not go directly from point B to point C. We have to go via point O and point A. As we need to go backwards along vector $\textbf{b}$ , we need a negative vector.

Write the vector.

Write the route from point B to point C.

$\begin{aligned} \overrightarrow{BC}&=\overrightarrow{BO}+\overrightarrow{OA}+\overrightarrow{AC}\\\\ &=\ -\textbf{b} \ +\ \textbf{a} \ + 2\textbf{b}\\\\ \text{or handwritten as}\\\\ &=\ -\underline{b} \ +\ \underline{a} \ + \ 2\underline{b} \end{aligned}$

Simplify your answer.

To simplify we collect like terms. So the final answer is:

$\overrightarrow{BC}=\textbf{a}+\textbf{b}=\underline{a}+\underline{b}$

Example 5: leaving the answer in its simplest form

Write the vector $\overrightarrow{CA}$ in terms of $\textbf{a}$ and $\textbf{b}$

Check the starting point and the end point.

\overrightarrow{CA}

The vector starts at point C and ends at point A.

Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors. We can not go directly from point C to point A. We have to go via point B and point O. As we are going in the opposite direction at times, we will need negative vectors.

Write the vector.

Write the route from point C to point A.

$\begin{aligned} \overrightarrow{CA}&=\overrightarrow{CB}+\overrightarrow{BO}+\overrightarrow{OA}\\\\ &=\ -5\textbf{a} \ -\ 2\textbf{b} \ +\ 4\textbf{a}\\\\ \text{or handwritten as}\\\\ &=\ -5\underline{a} \ -\ 2\underline{b} \ + \ 4\underline{a} \end{aligned}$

Simplify your answer.

To simplify we collect like terms. So the final answer is:

$\overrightarrow{CA}=-\textbf{a}-2\textbf{b}=-\underline{a}-2\underline{b}$

Example 6: leaving the answer in its simplest form

Here is a hexagon.

Side OA is parallel to side BE

Side OB is parallel to side CD

Side AC is parallel to side ED

Write the vector $\overrightarrow{OE}$ in terms of $\textbf{a}, \; \textbf{b}$ and $\textbf{c}$

Check the starting point and the end point.

\overrightarrow{OE}

The vector starts at point O and ends at point E.

Decide the route.

Start at the starting point and go along the sides of the shape to the end point.

However, you can only go along the lines which have vectors. But we can use the information to add more vectors to the diagram.

Write the vector.

Write the route from point O to point E.

$\begin{aligned} \overrightarrow{OE}&=\overrightarrow{OA}+\overrightarrow{AC}+\overrightarrow{CD}+\overrightarrow{DE}\\\\ &=\ \ \textbf{a} \ +\ \textbf{c} \ +\ \textbf{b} \ -\ \textbf{c}\\\\ \text{or handwritten as}\\\\ &=\ \ \underline{a} \ +\ \underline{c} \ + \ \underline{b} \ - \ \underline{c} \end{aligned}$

Simplify your answer.

To simplify we collect like terms. So the final answer is:

$\overrightarrow{OE}=\textbf{b}+\textbf{a}=\underline{b}+\underline{a}$

Alternatively, we could have gone from point O to point B and then to point E.

This would be

$\begin{aligned} \overrightarrow{OE}&=\overrightarrow{OB}+\overrightarrow{BE}\\\\ &=\ \textbf{b} \ +\ \textbf{a}\\\\ \text{or handwritten as}\\\\ &=\ \underline{b} \ +\ \underline{a} \end{aligned}$

Which is identical to our previous answer.

Common misconceptions

Final answers may just involve one vector

A question may ask you to write a vector in terms of vector $\textbf{a}$ and vector $\textbf{b}$ , but the final answer may just involve one of the vectors.

Vectors can involve fractions or decimals

Here is a diagram of vector $\textbf{c}$ . A vector in the same direction, but half of its length will be $\frac{1}{2}\textbf{c}$ or $0.5\textbf{a}$ .

Vector Notation Common Misconceptions Image

Practice vector notation questions

\textbf{b}

\textbf{a}+\textbf{b}

\textbf{a}-\textbf{b}

-\textbf{b}

We need to go in the opposite direction to vector $\textbf{b}$ , so we need a negative vector $\textbf{b}$ .

\overrightarrow{BO}=-\textbf{b}=-\underline{b}

Vector Notation Practice Question 1 Explanation Image

\textbf{a}-3\textbf{b}

\textbf{a}+\textbf{b}

\textbf{a}+3\textbf{b}

\textbf{a}-\textbf{b}

We need to go from point O to point B via point A.

\begin{aligned} \overrightarrow{OB}&=\overrightarrow{OA}+\overrightarrow{AB}\\\\ &=\ \ \textbf{a} \ +\ 3\textbf{b}\\\\ \text{or handwritten as}\\\\ &=\ \ \underline{a} \ +\ 3\underline{b} \end{aligned}

Vector Notation Practice Question 2 Explanation Image

\textbf{b}-\textbf{a}

2\textbf{b}+\textbf{a}

\textbf{a}+\textbf{b}

\textbf{a}-\textbf{b}

We need to go from point A to point B via point O. We need to go in the opposite direction to vector $\textbf{a}$ , so we need a negative vector $\textbf{a}$ .

\begin{aligned} \overrightarrow{AB}&=\overrightarrow{AO}+\overrightarrow{OB}\\\\ &= -\textbf{a} \ +\ \textbf{b}\\\\ &= \ \ \textbf{b} \ – \ \textbf{a}\\\\ \text{or handwritten as}\\\\ &=\ \ \underline{b} \ -\ \underline{a} \end{aligned}

Vector Notation Practice Question 3 Explanation Image

7\textbf{b}-\textbf{a}

-\textbf{b}-\textbf{a}

\textbf{a}+7\textbf{b}

\textbf{a}-\textbf{b}

We need to go from point B to point C via point A and point D. We need to go in the opposite direction to vector $4\textbf{b}$ , so we need a negative vector.

We need to go in the opposite direction to vector $\textbf{a}$ , so we need another negative vector. When we have worked out the route, we need to simplify the answer.

Vector Notation Practice Question 4 Explanation Image

\begin{aligned} \overrightarrow{BC}&=\overrightarrow{BA} \ +\ \overrightarrow{AD} \ + \ \overrightarrow{DC}\\\\ &= -4\textbf{b} \ -\ \textbf{a} \ + \ 3\textbf{b}\\\\ &= \ \ -\textbf{b} \ + \ \textbf{a}\\\\ \text{or handwritten as}\\\\ &=\ \ -\underline{b} \ -\ \underline{a} \end{aligned}

2\textbf{a}+3\textbf{b}

12\textbf{a}+3\textbf{b}

-2\textbf{a}+3\textbf{b}

12\textbf{a}-3\textbf{b}

We need to go from point B to point A via points C and D. We need to go in the opposite direction to vector $7\textbf{a}$ , so we need a negative vector. When we have worked out the route, we need to simplify the answer.

\begin{aligned} \overrightarrow{BA}&=\overrightarrow{BC} \ + \ \overrightarrow{CD} \ + \ \overrightarrow{DA}\\\\ &= -7\textbf{a} \ +\ 3\textbf{b} \ + \ 5\textbf{a}\\\\ &= \ \ -2\textbf{a} \ + \ 3\textbf{b}\\\\ \text{or handwritten as}\\\\ &=\ \ -2\underline{a} \ + \ 3\underline{b} \end{aligned}

Vector Notation Practice Question 5 Explanation Image

\textbf{b}-\textbf{c}

\textbf{a}+\textbf{b}+\textbf{c}

2\textbf{a}+\textbf{b}+\textbf{c}

\textbf{b}+\textbf{c}

We need to go from point O to point D. Use the facts about parallel sides to add in more vectors.

\begin{aligned} \overrightarrow{OD}&=\overrightarrow{OE} + \overrightarrow{ED}\\\\ &=\ \textbf{c} \ + \ \textbf{b}\\\\ &= \ \textbf{b} \ + \ \textbf{c}\\\\ \text{or handwritten as}\\\\ &=\ \underline{b} \ + \ \underline{c} \end{aligned}

Vector Notation Practice Question 6 Explanation Image

Vector notation GCSE questions

1. OABC is a trapezium

AB is parallel to OC.

Vector Notation GCSE Question 1

(a) Find, in terms of $\textbf{b}$ , the vector $\overrightarrow{BA}$

(b) Find, in terms of $\textbf{a}$ and $\textbf{b}$ , the vector $\overrightarrow{CA}$

(2 marks)

Show answer

(a)

\overrightarrow{BA}=-5\textbf{b}=-5\underline{b}

(1)

(b)

\overrightarrow{CA}=\textbf{a} \ – \ 3\textbf{b}=\underline{a} \ – \ 3\underline{b}

(1)

2. ABCD is a parallelogram.

The diagonals of the parallelogram intersect at O.

\begin{aligned} \overrightarrow{OA}=\textbf{a}\\ \overrightarrow{OB}=\textbf{b} \end{aligned}

Vector Notation GCSE Question 2

(a) Find, in terms of $\textbf{a}$ , the vector $\overrightarrow{AO}$

(b) Find, in terms of $\textbf{a}$ , the vector $\overrightarrow{CA}$

(3 marks)

Show answer

(a)

\overrightarrow{AO}=-\textbf{a} =-\underline{a}

(1)

(b)

\overrightarrow{CA}=2\textbf{a} =2\underline{a}

(1)

(c)

\overrightarrow{BA}=\textbf{a} \ – \ \textbf{b} =\underline{a} \ – \ \underline{b}

(1)

3. ABCD is a trapezium.

AB is parallel to DC.

Vector Notation GCSE Question 3

(a) Find, in terms of $\textbf{a}$ , the vector $\overrightarrow{AO}$

(b) Find, in terms of $\textbf{a}$ and $\textbf{b}$ , the vector $\overrightarrow{DB}$

(4 marks)

Show answer

(a)

\overrightarrow{AD}=-5\textbf{a} =-5\underline{a}

(1)

(b)

\overrightarrow{DB}=5\textbf{a} \ + \ 9\textbf{b} =5\underline{a} \ + \ 9\underline{b}

(1)

(c)

\overrightarrow{CB}=-7\textbf{b} \ + \ 5\textbf{a} \ + \ 9\textbf{b} =-7\underline{b} \ + \ 5\underline{a} \ + \ 9\underline{b}

For the correct route

(1)

\overrightarrow{CB}=5\textbf{a} \ + \ 2\textbf{b} = 5\underline{a} \ + \ 2\underline{b}

For the correct simplified answer

(1)

Learning checklist

You have now learned how to:

Use vector notation to write vector

Use vector notation to solve a geometry problem

The next lessons are

Did you know?

Vectors are very useful and can be extended beyond GCSE mathematics. Vector analysis is the branch of mathematics that studies vectors.

At GCSE we study two-dimensional vectors, but we can also look at three-dimensional vectors.

In A Level maths cartesian coordinates are also referred to as position vectors when we use a coordinate system as our vector space. In maths a vector is an element of a vector space.

Vectors can also be extended further by learning how to multiply two vectors together using the dot product. This is also known as the scalar product of two vectors. It is possible to multiply vectors and this is known as a cross product. This is also known as the vector product of two vectors.

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.