GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Arithmetic Place value Simplifying fractions

This topic is relevant for:

GCSE Maths Number FDP Percentages

Percentage of an Amount

Percentage Of An Amount

Here we will learn how to find percentage of an amount with and without using a calculator.

There are also percentage of an amount worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is a percentage of an amount?

A percentage of an amount can be calculated by writing the percentage as a decimal or a fraction and then multiplying it by the amount. There are different methods you can use to calculate a percentage of a quantity.

For example, what is 35% of 60?

Method 1: The one percent method

Find 1% first by dividing the amount by 100 and then multiply this by the amount.

\frac{60}{100} \times 35 = 21

Method 2: The decimal multiplier method

Write the percentage as a decimal and then multiply the amount by this decimal.

35\%=0.35

60 \times 0.35=21

Method 3: Using equivalent fractions

Write the percentage as a fraction in its simplest form and then multiply the amount by this fraction.

35\% = \frac{35}{100} =\frac{7}{20}

60 \times \frac{7}{20} = \frac{60\times 7}{20} = \frac{420}{20} =\frac{42}{2}= 21

Alternatively you can calculate the fraction of the quantity like this,

$35\%$ of $60 = \frac{7}{20}$ of $60$

$\frac{1}{20}$ of $60 = 60 \div 20 = 3$

$\frac{7}{20}$ of $60 = 60 \div 20 \times 7 = 21$

Method 4: Building up an answer from simple percentages

Using simple percentages, you can build up to the answer to the question.

This question could be broken down into the following steps.

$10\%$ of $60 = 6$ (To find 10% we divide by 10)

$5\%$ of $60 = 3$ (5% is half of 10%)

$30\%$ of $60 = 18$ (30% is three lots of 10%)

$35\%$ of $60 = 18+3=21$ (30% plus 5%)

Methods 1 and 2 lend themselves to questions where a calculator is allowed. Methods 3 and 4 lend themselves to questions where calculators are not permitted.

What is a percentage of an amount?

Percentage of a quantity

Amount as a percentage

You may be asked to express an amount as a percentage of another.

For example, express 40 as a percentage of 50.

This would mean writing the numbers as a fraction, and multiplying by 100. The first amount is the numerator and the second amount is the denominator.

\frac{40}{50}\times 100=80%

A different question could ask you to write 50 as a percentage of 20. Here it is very important to make sure that the first amount is the numerator and the second amount is the denominator.

\frac{50}{20}\times 100=250%

How to find the percentage of an amount (non-calculator)

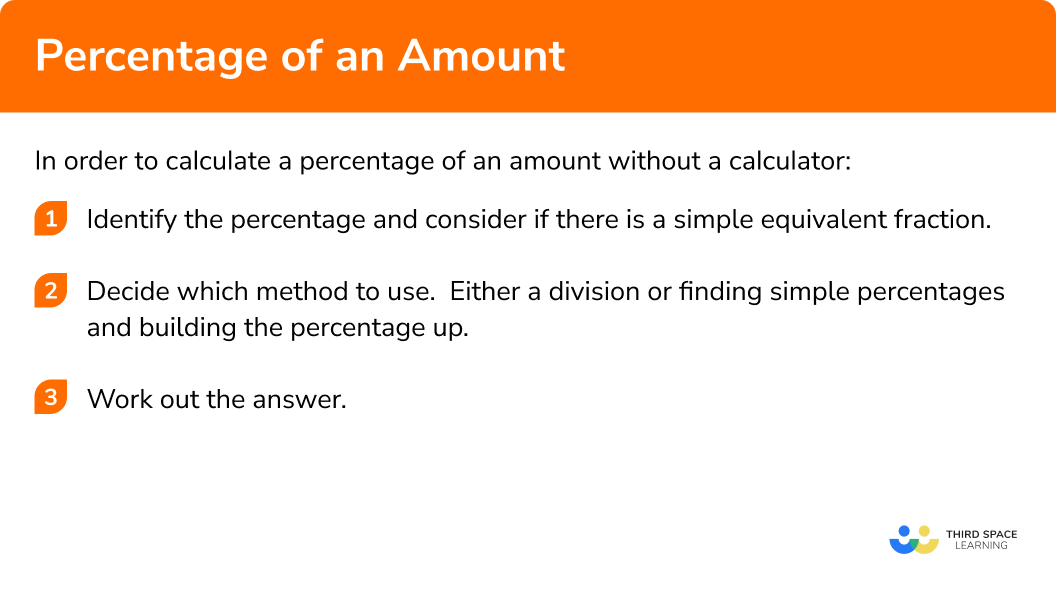
In order to find the percentage of an amount without a calculator:

Identify the percentage and consider if there is a simple equivalent fraction.
Decide which method to use. Either a division or finding simple percentages and building it up.
Work out the answer.

Explain how to calculate a percentage of an amount without a calculator in 3 steps

Percentage of an amount worksheet

Get your free percentage of an amount worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Percentage of an amount worksheet

Get your free percentage of an amount worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Percentage of an amount examples

Example 1: percentage has a simple equivalent fraction

Find 25% of 120 km.

Identify the percentage.

\[25\%=\frac{25}{100}=\frac{1}{4}\]

2 Decide which method to use. Either a division or finding 10%, 1% and building it up.

Since 25% = ¼, to find 25% we can divide by 4.

3 Work out the answer.

\[120\div 4 = 30\]

The answer is 30 km.

Example 2: percentage has a simple equivalent fraction

Find 10% of 450 kg.

Identify the percentage.

\[10\%=\frac{10}{100}=\frac{1}{10}\]

Decide which method to use. Either a division or finding 10%, 1% and building it up.

Since 10% = ⅟₁₀, to find 10% we can divide by 10.

Work out the answer.

\[450\div 10 = 45\]

The answer is 45 kg.

Example 3: percentage can be made up from 10% and 1%

Find 16% of £300.

Identify the percentage.

\[16\%= 10\% + 6\times 1\% \]

Decide which method to use. Either a division or finding 10%, 1% and building it up.

Since 10% = ⅟₁₀, to find 10% we can divide by 10.

\[300\div 10 = 30\]

Then we divide by 10 again to find 1%.

\[30\div 10 = 3\]

Work out the answer.

\[16\%= 10\% + 6\times 1\%\]

Therefore,

16% of 300 is

\[30 + (6\times 3) = 30 + 18 = 48\]

The answer is £48.

Example 4: percentage can be made up from 10% and 1%

Find 23% of £700.

Identify the percentage.

\[23\%= 2\times 10\% + 3\times 1\%\]

Decide which method to use. Either a division or finding 10%, 1% and building it up.

Since 10% = ⅟₁₀, to find 10% we can divide by the denominator 10.

\[700\div 10 = 70\]

Then we divide by 10 again to find 1%.

\[70\div 10 = 7\]

Work out the answer.

\[23\%= 2\times 10\% + 3\times 1\%\]

Therefore,

23% of 700 is

\[(2 × 70) + 3\times 7 = 140 + 21 = 161\]

The answer is £161.

How to find the percentage of an amount (with calculator)

In order to the percentage of an amount with a calculator:

Identify the percentage.
Find the decimal multiplier.
Work out the answer by multiplying the original amount by the decimal multiplier.

Explain how to calculate the percentage of an amount with a calculator in 3 steps

Step by step guide: Percentage Multipliers

Example 5: when you have a calculator

Work out 78% of £411.

Identify the percentage.

\[78\%\]

Find the decimal multiplier.

Let’s find the decimal equivalent of 78%:

\[78\%=\frac{78}{100}=0.78\]

We can calculate this by dividing the numerator of 78 by the denominator of 100.

Writing 78% in its decimal form is 0.78.

Work out the answer by multiplying the original amount by the decimal multiplier.

\[411 \times 0.78=320.58\]

The answer is £320.58.

Example 6: when you have a calculator

Work out 61% of £728.

Identify the percentage.

\[61\%\]

Find the decimal multiplier.

\[61\%=\frac{61}{100}=0.61\]

We can calculate this by dividing the numerator of 61 by a hundred.

Work out the answer by multiplying the original amount by the decimal multiplier.

\[728 \times 0.61=444.08\]

The answer is £444.08.

Common misconceptions

Money and two decimal places

E.g.
An answer of £16.5 should be written as £16.50. There are two digits needed for the pence.

Using a non-calculator method when you have a calculator

If you are asked to find 29% of £391 you can do it by finding 10% and 1% and building it up to find 20% and 9% and adding them together. However it is much more simpler here to use a multiplier.

E.g.
Calculate 29% of £391

\[391 \times 0.29=£ 113.39\]

Dividing by 10 – taking care with the decimal points

E.g.
Find 41% of 53 kg:

10% is:

\[53\div 10 = 5.3\]

1% is:

\[5.3\div 10 = 0.53\]

41% of £53 is:

\[(4 × 5.3) + 0.53 = 21.73\]

The answer is 21.73 kg.

Percentage of an amount is part of our series of lessons to support revision on percentages. You may find it helpful to start with the main percentages lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Practice percentage of an amount questions

\pounds 140

\pounds 28

\pounds 240

\pounds 70

$25\%$ is the same as $\frac{1}{4},$

so we can calculate

\pounds 280 \div 4 = \pounds 70

35kg

7kg

17.5kg

50kg

$50\%$ is the same as $\frac{1}{2},$

so we can calculate

70 \div 2 = 35

40g

24g

16.67g

25g

$10\%$ of $200:$

200 \div 10 = 20

$1\%$ of $200:$

200 \div 100 = 2

\begin{aligned} 12\%&=10\%+(2 \times 1\%)\\\\ &=20+(2 \times 2)\\\\ &=24 \end{aligned}

75km

81km

67km

27km

$10\%$ of $300km:$

300 \div 10 = 30

$1\%$ of $300km:$

300 \div 100 = 3

\begin{aligned} 27\%&=(2 \times 10\%) + (7 \times 1\%)\\\\ &=(2 \times 30) + (7 \times 3)\\\\ &=60+21\\\\ &=81km \end{aligned}

\pounds 404.70

\pounds 404.07

\pounds 12.46

\pounds 570

57\%=\frac{57}{100} = 0.57

£710 \times 0.57 = \pounds 404.70

\pounds 30.43

\pounds 83.79

\pounds 148.57

\pounds 143.20

83\%=\frac{83}{100}=0.83

£179 \times 0.83 = \pounds 148.57

Percentage of an amount GCSE questions

1. Without a calculator,

work out $20\%$ of $600 g.$

(2 marks)

Show answer

for finding $10\%,$

$600 \div 10 = 60$

(1)

for the correct answer

$120 g$

(1)

2. Andy is paid $£1400$ per month. He gets a $2$ % increase in his monthly earnings. How much money will Andy earn per month after the increase?

(2 marks)

Show answer

$2\% = £28,$

$1400 + 28$

(1)

for the correct answer

$£1428$

(1)

3. At a shop T-shirts cost $£4.60$ each. If you buy $20$ or more T-shirts, you can get $10\%$ off the total cost of all of the T-Shirts you have bought.

Work out the total cost of buying $26$ T-shirts.

(3 Marks)

Show answer

$26 \times £4.60 = £119.60$

(1)

$10\%$ of $£119.60=£11.96$ ,

(1)

$£119.60 - £11.96 = 107.64$

(1)

Learning checklist

You have now learned how to:

Interpret percentages as a fraction.

Interpret percentages as a decimal.

Interpret percentage multiplicatively.

Use a calculator to calculate results accurately.

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.