Percentage increase

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Percentages of an amount Percentage to decimal

This topic is relevant for:

GCSE Maths Number FDP Percentages

Percentage Increase

Here is everything you need to know about percentage increase for GCSE maths (Edexcel, AQA and OCR). You’ll learn how to increase a value by a given percentage, use multipliers to calculate percentage increase and work out percentage change.

Look out for the percentage increase and decrease worksheets and exam questions at the end.

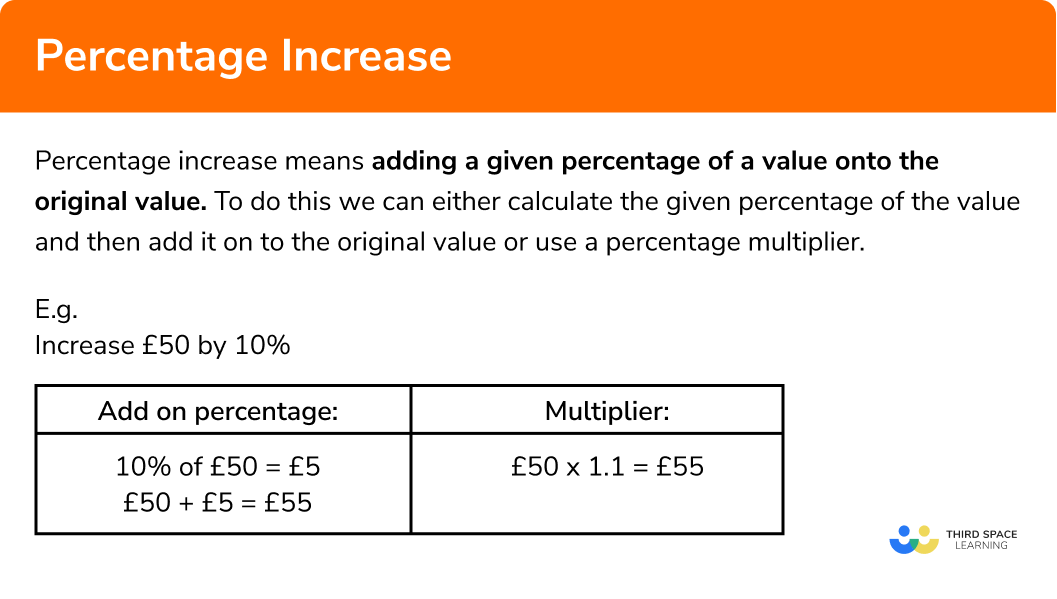
What is percentage increase?

Percentage increase means adding a given percentage of a value on to the original value. To do this we can either calculate the given percentage of the value and then add it on to the original value or use a percentage multiplier.

Explain what percentage increase is?

Percentage increase and decrease worksheet

Get your free percentage increase and decrease worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Percentage increase and decrease worksheet

Get your free percentage increase and decrease worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

How to increase a value by a percentage

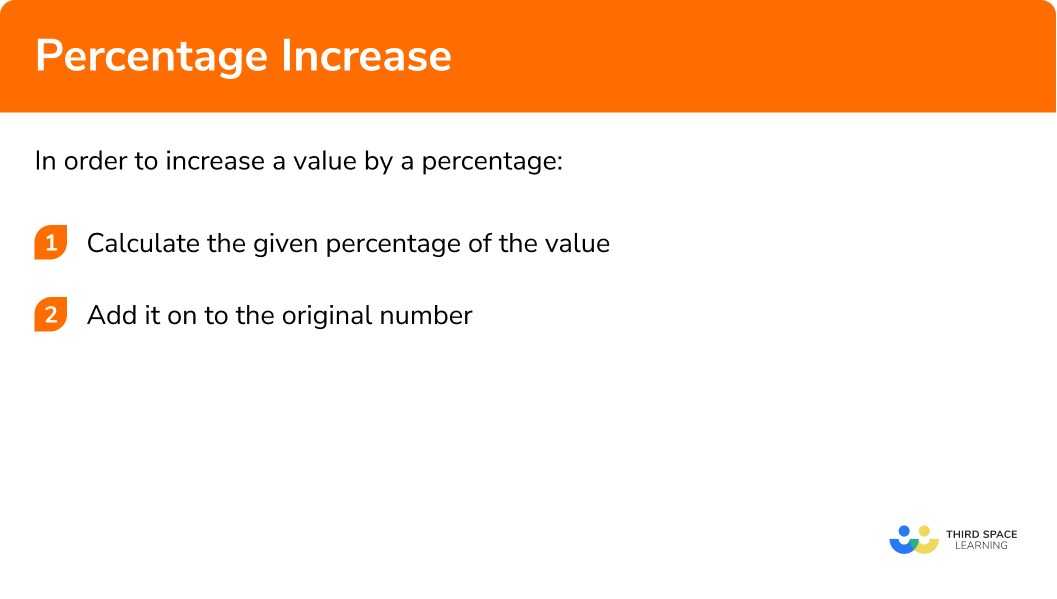
In order to increase a value by a percentage:

Calculate the given percentage of the value.
Add it on to the original number.

What are the 2 steps to increase a value by a percentage?

How to work out percentage increase examples

Example 1: non-calculator

Increase £50 by 40%

Calculate 40% of £50

To do this without a calculator, the easiest way is to calculate 10% (by dividing by 10) and then multiply by 4 to get 40%.

10% of £50 = £5

40% of £50 = £20

2 Add £20 on to the original value

£50 + £20 = £70

Example 2: non-calculator

Increase 400 by 65%

Calculate 65% of 400

10% of 400 = 40

60% of 400 = 240

5% of 400 = 20

65% of 400 = 260

Add 260 on to the original value

400 + 260 = 660

Example 3: using a calculator

Increase 350m by 27%

Calculate 27% of 350m

When using a calculator to calculate a percentage, we divide the value by 100 to find 1% and then multiply by the percentage that we want.

\begin{array}{l} 350 \div 100=3.5 \\ 1\%=3.5\\\\ 3.5 \times 27=94.5 \mathrm{~m}\\ 27\%=94.5 \end{array}

Add 94.5m on to the original value

350m + 94.5m = 444.5m

Example 4: using a calculator

The price of Katrina’s train ticket last year was £72. This year it has increased by 17.5%. Find the price of Katrina’s train ticket this year.

Calculate 17.5% of £72

\[\begin{array}{l} 72 \div 100=0.72 \\ 1\%=0.72\\\\ 0.72 \times 17.5= £ \hspace{1mm} 12.60\\ 17.5\%= £ \hspace{1mm} 12.60 \end{array}\]

Add £12.60 on to the original value

£72 + £12.60 = £84.60

How to increase a value by a percentage using a percentage multiplier

We can increase a value by a percentage using a percentage multiplier. A percentage multiplier is a decimal that is related to the percentage you are trying to find.

Add the percentage we are increasing by to 100%

We add it on to 100% because we are increasing the original value.

2Convert to a decimal

3Multiply the original amount by the decimal

Example 5: using a multiplier

Increase 3200ml by 24%

Here we are calculating a 24 percent increase so add 24% on to 100%

\[100\% + 24\% = 124\%\]

Convert to a decimal. To do this we divide the percentage by 100.

\[124\% = 1.24\]

Multiply 3200 by 1.24

\[3200\times 1.24=3968ml\]

Example 6: using a multiplier

In 2015 Rachel’s monthly rent was £620. Over the course of five years, Rachel’s monthly rent increased by 12.5%. Find Rachel’s monthly rent at the end of the five years.

This is a 12.5 percent increase so we add 12.5% on to 100%

\[100\% + 12.5\% = 112.5\%\]

Convert to a decimal.

\[112.5\% = 1.125\]

Multiply £620 by 1.125

620 × 1.125 = £697.50

Remember to always write money using two decimal places.

How to calculate percentage increase between two values

Given two values, we can calculate the percentage change. This may also be called percentage difference, percentage increase, percentage gain or percentage profit.

We can calculate percentage change using the percentage change formula:

\[\text { Percentage change }=\frac{\text { Change }}{\text { Original }} \times 100\]

The same formula can be used to calculate percentage decrease

Work out how much the value has changed by subtracting the old number from the new number.
Apply the percentage change formula

Example 7: calculating percentage change

The weight of a lamb has increased from 4kg to 5.2kg. Find the percentage increase in the lamb’s weight.

The weight of the lamb has changed from 4kg to 5.2kg. Work out the actual change:

\[5.2 − 4 = 1.2kg\]

Apply the percentage change formula. The change is 1.2kg and the original amount is 4kg.

\begin{array}{l} \text { Percentage change }&=\frac{\text { Change }}{\text { Original }} \times 100 \\\\ \text { Percentage change}&=\frac{1.2}{4} \times 100\end{array}

Percentage change = 30%

The lamb’s weight has increased by 30%.

Example 8: calculating percentage profit

Lucy buys an antique table for £150 and sells it for £185. Calculate Lucy’s percentage profit.

Work out the change in value by subtracting the original price from the new price:

£185 – £150 = £35

Apply the percentage change formula. The change is £35 and the original price is £150.

\[\text { Percentage change }=\frac{35}{150} \times 100\]

Percentage change = 23.3333333..%

The percentage profit is 23.3% to 1 decimal place.

Common misconceptions

Percentages greater than 100%

Believing that percentages cannot be greater than 100%. For percentage increase using multipliers we encounter a lot of percentages greater than 100% as we are adding on to the original 100%.

Converting between percentages and decimals

Incorrectly converting percentages to decimals. The most common mistakes are with single digit percentages (e.g. 5%), multiples of 10 (e.g. 40%) and decimal percentages (e.g. 3.2%)

Remember to divide the percentage by 100 to find the decimal.

E.g.

5% = 0.05,

40% = 0.4,

3.2% = 0.032,

106.5% = 1.065

Using an incorrect value for the denominator in the percentage increase formula

Using the new value instead of the original value for the denominator when calculating percentage change.

Percentage increase is part of our series of lessons to support revision on percentages. You may find it helpful to start with the main percentages lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Percentage increase practice questions

$£540$

$£104$

$£624$

$£640$

$20\%$ of $520$ is $104$ . This can be added to the original amount to find the quantity after the increase.

$3463m$

$4890.6m$

$1470.6m$

$3563m$

$43\%$ of $3420$ is $1470.6$ . This can be added to the original amount to find the quantity after the increase.

$5.32$

$43.32$

$42$

$33.3$

The multiplier we need to use is $1.14$ , so the calculation is $1.14\times38$ .

$172.25kg$

$822.25kg$

$513.83kg$

$676.5kg$

The multiplier we need to use is $1.265$ , so the calculation is $1.265\times650$ .

$55\%$

$155\%$

$220\%$

$110\%$

The amount has increased by $220ml$ . As a percentage of the original amount, this is $\frac{220}{400}\times100$ .

$111\%$

$89\%$

$11\%$

$136.4\%$

The percentage profit is calculated as $\frac{137640-124000}{124000}\times100$ .

Percentage increase GCSE questions

1. (a) Catrin’s salary is $£18000$ . She receives a $3.5\%$ pay rise.

Work out Catrin’s new salary.

(b) How much extra money will Catrin earn each week?

(3 marks)

Show answer

(a)

$3.5\%$ of $\pounds 18000 = \pounds 630$

(1)

$\pounds 18000 + \pounds 630 = \pounds 18630$

(1)

(b)

\pounds 630 \div 52 = \pounds 12.12

(1)

2. In $2010$ the population of the UK was approximately $62220000$ .

By $2020$ it had increased to $66520000$ .

Find the percentage increase in population over these $10$ years.

Give your answer to $2dp$ .

(2 marks)

Show answer

$66520000 - 62220000 = 4300000$

(1)

\frac{4300000}{62220000} \times 100=6.91\%

(1)

3. Thomas bought a box containing $100$ packets of crisps for $£30$ .

He sold $96$ packets of crisps for $50p$ each.

Calculate Thomas’s percentage profit.

(3 marks)

Show answer

96 \times 50 p = \pounds 48

(1)

\pounds 48-\pounds 30=\pounds 18

(1)

\frac{18}{30} \times 100=60 \%

(1)

Learning checklist

You have now learned how to:

Increase a value by a given percentage

Use a percentage multiplier to increase a value by a percentage

Calculate percent increase between two values

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.