Reverse percentages

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Percentages Percentage increase Percentage decrease Proportion

This topic is relevant for:

GCSE Maths Number FDP Percentages

Reverse Percentages

Here we will learn about reverse percentages (or inverse percentages) including how to work backwards to find an original amount given a percentage of that amount or a percentage increase/decrease.

There are also reverse percentages worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What are reverse percentages?

Reverse percentages is a way of working backwards on a percentage problem to find an original amount.

In order to do this:

We can use a calculator to take the percentage given, divide to find 1% and then multiply by 100 to find 100%.
We can also do this without a calculator by using factors of the percentage we have been given.
Sometimes we are given a percentage of an amount and must work out the original value.

We need to remember that the original amount is 100% of the value.

What are reverse percentages?

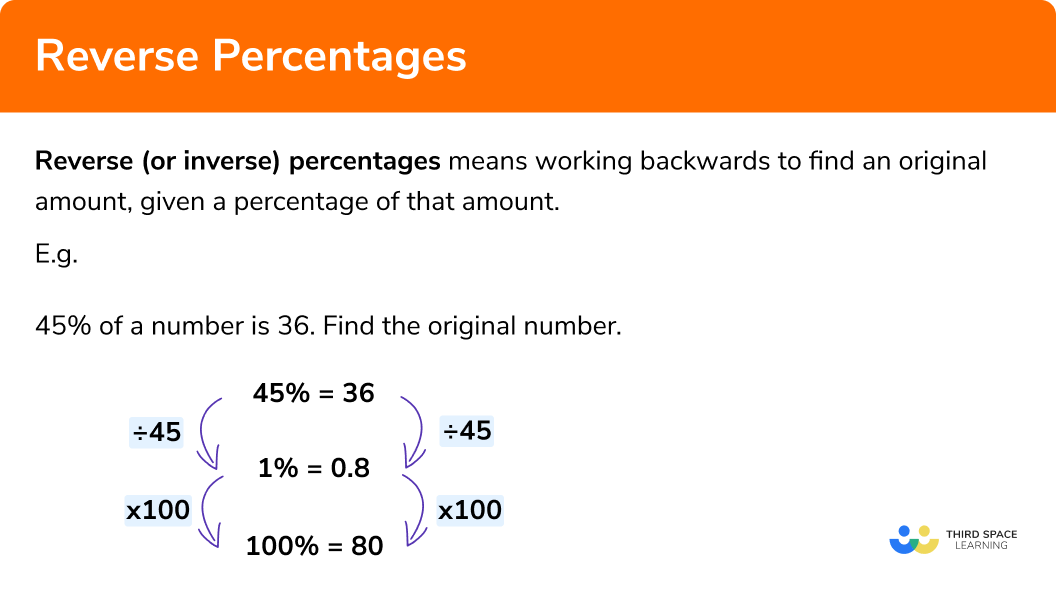
How to use reverse percentages given a percentage of an amount (calculator method)

In order to find the original amount given a percentage of the amount (using a calculator):

Write down the percentage and put it equal to the amount you have been given.
Divide both sides by the percentage.
(e.g. if you have 80%, divide both by 80). This will give you 1%.
Multiply both sides by 100.
This will give you 100%.

Explain how to find the original amount given a percentage of the amount in 3 steps

Reverse percentages worksheet

Get your free reverse percentages worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Reverse percentages worksheet

Get your free reverse percentages worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Reverse percentage examples (calculator method)

Example 1: calculator

45% of a number is 36. Find the original number.

Put the percentage equal to the amount.

45% = 36

2 Divide both sides by the percentage to find 1%.

In this case the percentage is 45%, so divide by 45.

3 Multiply by 100 to find 100%.

The original number was 80.

Example 2: calculator

150% of a number is 690. Calculate the original number.

Put the percentage equal to the amount.

150% = 690

Divide both sides by the percentage to find 1%. In this case the percentage is 150%, so divide by 150.

Multiply by 100 to find 100%.

The original number was 460.

How to use reverse percentages given a percentage of an amount (non-calculator method)

In a situation where we do not have a calculator, we can often simplify the problem by using common factors.
Rather than finding 1%, which might involve a difficult division, we could find 10%, 25%, or any other percentage which is a factor of 100%.

Write down the percentage and put it equal to the amount you have been given.
Identify a common factor of the percentage and 100% (a number which goes in to both).
Use division to find that percentage of your amount.
Use multiplication to find 100%.

Reverse percentages examples (non-calculator method)

Example 3: non-calculator

70% of an amount is 56. Find the original amount.

Put the percentage equal to the amount.

70% = 56

Identify a common factor of 70% and 100%.

Factors of 70: 1, 2, 5, 7, 10, 14, 35, 70

Factors of 100: 1, 2, 4, 5, 10, 20, 25, 50, 100

Here 1, 2, 5 and 10 are all factors of both 70 and 100. We could use any of these but select the one which results in the easiest calculations (usually the highest common factor). In this case we are going to use 10%.

As 10% is a factor of both 70% and 100%, we need to find 10% of our amount. To do this we will divide by 7 because 70% ÷ 7 = 10%.

As we now have 10%, we need to multiply by 10 to find 100%.

The original amount was 80.

Note: In this example, and every example, the method of finding % would also work. The reason that we found 10% here rather than 1% is that 56 ÷ 7 is easier to work out without a calculator than 56 ÷ 70.

Example 4: non-calculator

125% of a number is 350. Find the original value.

Put the percentage equal to the amount.

125% = 350

Identify a common factor of 125% and 100%.

Factors of 125: 1, 5, 25, 125

Factors of 100: 1, 2, 4, 5, 10, 20, 25, 50, 100

Here we are going to use 25%.

As 25 is a factor of both 125% and 100%, we need to find 25% of our amount. To do this we divide by 5 because 125% ÷ 5 = 25%.

As we now have 25%, we need to multiply by 4 to find 100%.

The original amount was 280.

How to use reverse percentages given a percentage increase/decrease

Sometimes, instead of being told a percentage of the amount, we are told what percentage increase or decrease has occurred.
The only difference here compared to what we have already looked at is that we first need to identify what percentage of the original amount we now have.

Identify what percentage of the original amount you now have.
If it has been increased by a percentage, add that percentage onto 100%.
If it has been decreased by a percentage, subtract that percentage from 100%.
Write down the percentage and put it equal to the amount you have been given.
Use either the calculator or non-calculator method to find 100%.

Reverse percentages examples (percentage increase/decrease)

Example 5: percentage increase, calculator

The number of fans attending a football match this week was 12% more than last week. If 728 people attended the match this week, how many attended last week?

This is a percentage increase of 12%.

100% + 12% = 112%

Write down the percentage and put it equal to the amount you have been given.

112% = 728

This is a calculator question, so use the method of finding 1%.

This image has an empty alt attribute; its file name is Reverse-Percentages-9.svg

This image has an empty alt attribute; its file name is Reverse-Percentages-10.svg

The number of fans last week was 650.

Example 6: percentage decrease, calculator

The value of a car has decreased by 16.5% in the last year. If its value now is £5845, find its original price.

This is a percentage decrease of 16.5%.

100% – 16.5% = 83.5%

Write down the percentage and put it equal to the amount you have been given.

83.5% = 5845

Use a calculator to find 1%.

The original price was £7000.

Example 7: percentage increase, non-calculator

A puppy’s weight has increased by 20% to 4.8kg. What was the puppy’s weight before the increase?

This is a percentage increase of 20%.

100% + 20% = 120%

Write down the percentage and put it equal to the amount you have been given.

120% = 4.8

This is a non-calculator question, so use the common factor method.

Factors of 120: 1, 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 30, 40, 60, 120

Factors of 100: 1, 2, 4, 5, 10, 20, 25, 50, 100

There are several common factors here. It would be easiest to use 10% or 20%. Here we are going to use 20%.

The puppy’s original weight was 4kg.

Example 8: percentage decrease, non-calculator

A television is in a 10% sale. The sale price of the television is £450. Find the original price of the television.

This is a percentage decrease of 10%.

100% – 10% = 90%

Write down the percentage and put it equal to the amount you have been given.

90% = 450

This is a non-calculator question, so use the common factor method.

Factors of 90: 1, 2, 3, 5, 6, 9, 10, 15, 30, 45, 90

Factors of 100: 1, 2, 4, 5, 10, 20, 25, 50, 100

Here we are going to use 10%.

The original price of the television was £500.

Common misconceptions

Calculating a percentage and adding it on

A common mistake is to work out the percentage of the number and then add it on.
E.g.
Given 70% of a number, a common error is to calculate 30% of that number to add on to the 70%.
Remember, this does not work as 30% of 70% would not be the same as 30% of the original value.

Not adding/subtracting from 100% when it is a percentage increase/decrease

A common mistake is to use the actual percentage increase/decrease rather than adding/subtracting from 100%.
E.g
If you are told it is a 30% decrease, a common error would be to use 30% instead of 70%.

Reverse percentages is part of our series of lessons to support revision on percentages. You may find it helpful to start with the main percentages lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Practice reverse percentages questions

You may use a calculator for questions 1, 2 and 5.

117

900

360

1166

36\% = 324

Dividing both sides by $36$ gives

1\% = 9

Multiplying both sides by $100$ gives

100\% = 900

3045

2755

2000

1885

145\% = 2900

Dividing both sides by $145$ gives

1\% = 20

Multiplying both sides by $100$ gives

100\% = 2000

126

525

270

350

60\% = 210

Dividing both sides by $3$ gives

20\% = 70

Multiplying both sides by $5$ gives

100\% = 350

44

22

49.5

11

150\% = 33

Dividing both sides by $3$ gives

50\% = 11

Multiplying both sides by $2$ gives

100\% = 22

£ $8000$

£ $7820$

£ $5780$

£ $6785$

The price has been reduced by $15\%$ , so £ $6800$ is equal to $85\%$ of the original price.

85\% = £6800

Dividing both sides by $85$ gives

1\% = 80

Multiplying both sides by $100$ gives

100\% = 8000

221

210

241

218

The number of customers has increased by $10\%$ , so $231$ is equal to $110\%$ of the number from yesterday.

110\% = 231

Dividing both sides by $11$ gives

10\% = 21

Multiplying both sides by $10$ gives

100\% = 210

Reverse percentages GCSE questions:

1. $40\%$ of the children in Rahim’s class walk to school.

$12$ children walk to school.

How many children are in Rahim’s class?

(2 marks)

Show answer

$40\% = 12$

(1)

$10\% = 3$

(1)

$100\% = 30$

(1)

2. In a sale, prices are reduced by $15\%$ .

A phone is reduced by $\pounds 36$ .

Find the original price of the phone.

(3 marks)

Show answer

$15\% = \pounds 36$

(1)

$5\% = \pounds 12$

(1)

$100\% = \pounds 240$

(1)

3. Tony receives a pay increase of $12\%$ .

His new salary is $\pounds 31920$ per annum.

Calculate how much more money he earns each year following the pay increase.

(4 marks)

Show answer

$112\% = \pounds 31920$

(1)

$1\% = \pounds 285$

(1)

$100\% = \pounds 28500$

(1)

$\pounds 31920 − \pounds 28500 = \pounds 3420$

(1)

Learning checklist

You have now learned how to:

Find an amount given a percentage of that amount (calculator and non-calculator)

Calculate reverse percentages involving percentage increase/decrease

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.