GCSE Tutoring Programme
"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."
In order to access this I need to be confident with:
Squares and square roots Cubes and cube roots BIDMAS Rearranging equations TrigonometryThis topic is relevant for:

Inverse Functions
Here we will learn about inverse functions including what an inverse function is, the notation used for an inverse function and how to find an inverse function.
There are also inverse function worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
What are inverse functions?
Inverse functions are functions which reverse or “undo” another function.
To write the inverse of the function f , we use the notation f^{-1} .
We have seen how to use a function machine to work backwards to find the input from a known output. If we were to write the algebraic expression relating to these inverse operations in the correct order, we would have found the inverse function.
To find an inverse function we need to rewrite the function using y as the unknown variable and set the function equal to x. Then we need to rearrange the function to make y the subject and write the function using the inverse function notation.
E.g.
Inverse functions can be used to solve equations or find missing x values on graphs if we know the y value.
Inverse functions are also used when finding an unknown angle in a triangle using trigonometry.
E.g.
When finding a missing angle or solving the equation sin (x)=0.6 , we would need to use the inverse of the sine function, x=sin^{-1}(0.6).
In A level mathematics instead of using sin^{-1}, cos^{-1} and tan^{-1}, the inverse trigonometric functions have different names. We would use arcsin, arccos and arctan.
We also look at when inverse functions can exist. Functions such as quadratics, cubics, sin, cos and tan must have a restricted domain (a limit to which x values are allowed).
We also look at how to find the graph of the inverse function f^{-1} by reflecting the graph of the function f in the line y=x .
What are inverse functions?
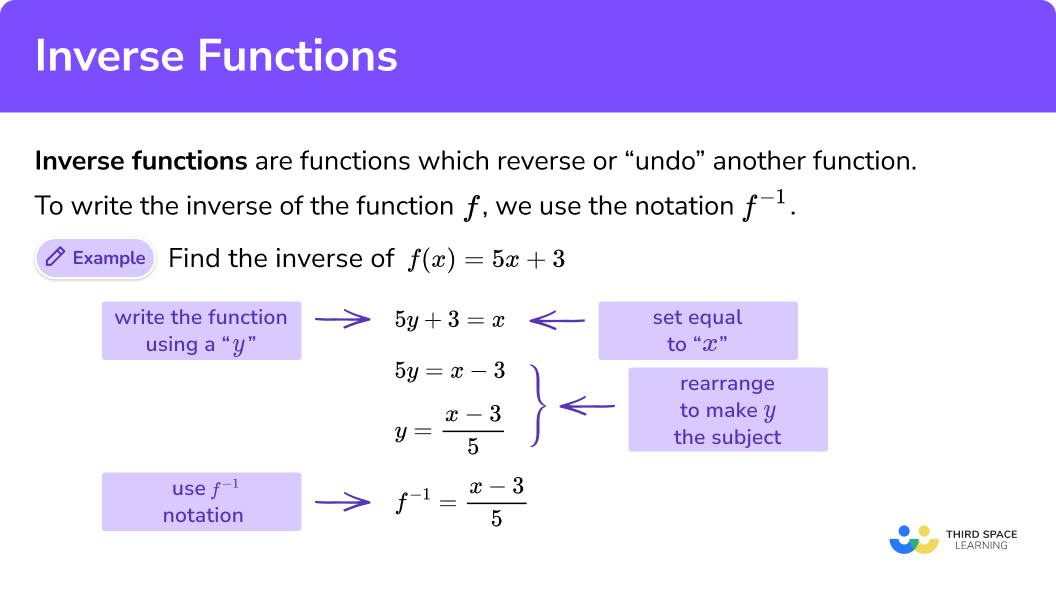
How to find inverse functions
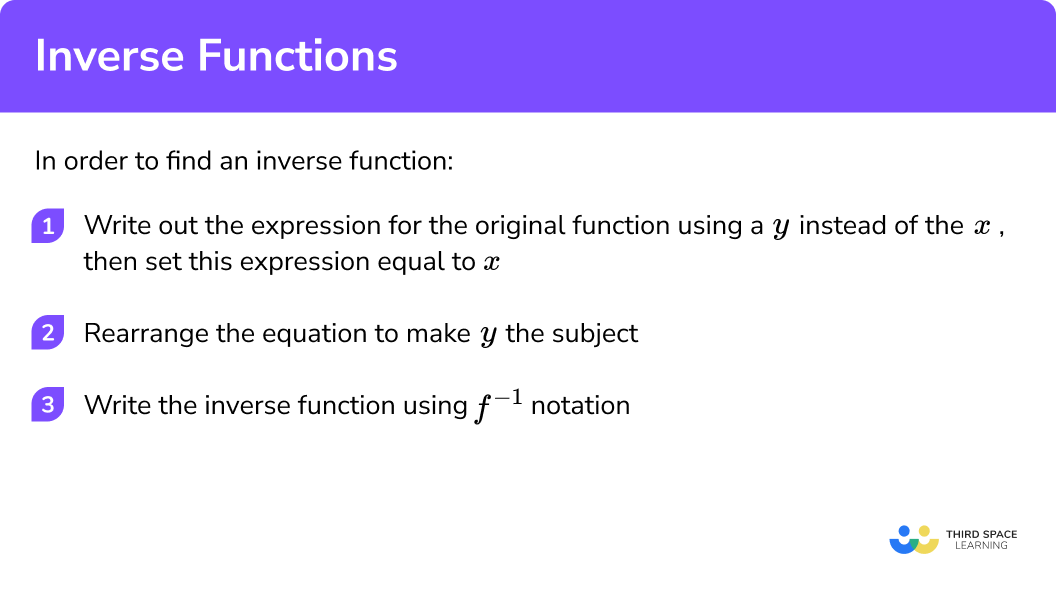
In order to find an inverse function:
- Write out the expression for the original function using a y instead of the x . Set this expression equal to x.
- Rearrange the equation to make y the subject.
- Write your inverse function using the f^{-1} notation.
Explain how to find inverse functions
Inverse functions worksheet

Get your free inverse functions worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Inverse functions worksheet

Get your free inverse functions worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREERelated lessons on functions in algebra
Inverse functions is part of our series of lessons to support revision on functions in algebra. You may find it helpful to start with the main functions in algebra lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:
Inverse functions examples
Example 1: Finding the inverse of a linear function
If f(x)=3x-7 , find f^{-1}(x).
- Write out the expression for the original function using a y instead of the x . Set this expression equal to x.
2Rearrange the equation to make y the subject.
\begin{aligned} & 3y-7=x \\\\ & 3y=x+7 \hspace{2cm} \text{Add } 7 \text{ to both sides of the equation} \\\\ & y=\frac{x+7}{3} \hspace{2cm} \text{Divide both sides by } 3 \end{aligned}3Write your inverse function using the f^{-1} notation.
{{f}^{-1}}\left( x \right)=\frac{x+7}{3}Example 2: Finding the inverse of a linear function
If g(x)=9-\frac{x}{2} find g^{-1}(x) .
Write out the expression for the original function using a y instead of the x . Set this expression equal to x.
Rearrange the equation to make y the subject.
Write your inverse function using the f^{-1} notation.
Use g^{-1} because the original function was g.
{{g}^{-1}}\left( x \right)=18-2x
Example 3: Finding the inverse of a function involving a power
If f(x)=2x^2-5 find f^{-1}(x) .
Write out the expression for the original function using a y instead of the x . Set this expression equal to x.
Rearrange the equation to make y the subject.
An inverse function cannot have two different outputs for one input so we only need the positive square root.
Write your inverse function using the f^{-1} notation.
Example 4: Finding the inverse of a function involving an algebraic fraction
If h(x)=\frac{x-3}{x+2} , find h^{-1}(x) .
Write out the expression for the original function using a y instead of the x . Set this expression equal to x.
Rearrange the equation to make y the subject.
Write your inverse function using the f^{-1} notation.
Common misconceptions
- “
1 overf(x) “
A common error is to think the inverse function will be the reciprocal of the function because of the “power of -1 ” notation.
E.g.
If f(x)=3x, an incorrect expression for f^{-1} would be (3x)^{-1} or \frac{1}{3x} .
Practice inverse function questions
1. If f(x)=4x-9, find f^{-1}(x):




Rearranging the formula, first add 9, then divide by 4.
2. If g(x)=\frac{x}{3}+7, find g^{-1}(x):




Rearranging the formula, first subtract 7, then multiply by 3.
3. If h(x)=5-x, find h^{-1}(x) :




Rearranging the formula, first add y to both sides, then subtract x from both sides.
4. If f(x)=x^3-1, find f^{-1}(x):




Rearranging the formula, first add 1, then cube root.
5. If g(x)=\sqrt{x}+6, find g^{-1}(x) :




Rearranging the formula, first subtract 6, then square and expand the brackets.
6. If h(x)=\frac{2x}{x+3}, find h^{-1}(x) :




See working and use example 4 to help.
\begin{aligned} & \frac{2y}{y+3}=x \\\\ & 2y=x\left( y+3 \right) \\\\ & 2y=xy+3x \\\\ & 2y-xy=3x \\\\ & y\left( 2-x \right)=3x \\\\ & y=\frac{3x}{2-x} \\\\ & {{h}^{-1}}\left( x \right)=\frac{3x}{2-x} \end{aligned}
Inverse functions GCSE questions
1. (a) If f(x)=6x-5, find f^{-1}(x).
(b) If g(x)=3+\sqrt{x}, find g^{-1}(x).
(4 marks)
(a)
Attempt the rearrange formula 6y-5=x
(1)
\frac{x+5}{6}
(1)
(b)
Attempt the rearrange formula 3+\sqrt{y}=x
(1)
(x-3)^2 or x^2-6x+9
(1)
2. If f(x)=3x-2 and g(x)=4x+7
(a) Find f^{-1}(4)
(b) Find when f(x)=g^{-1}(x)
(6 marks)
(a)
Set 3x-2=4 or find f^{-1}(x)=\frac{x+2}{3}
(1)
Answer of 2
(1)
(b)
g^{-1}(x)=\frac{x-7}{4}
(1)
Set equal 3x-2=\frac{x-7}{4}
(1)
Get x’s on one side of the equation 12x-x=-7+8
(1)
Answer of x=\frac{1}{11}
(1)
3. If f(x)=\frac{x+5}{x+3}, find f^{-1}(x).
(4 marks)
x(y+3)=y+5 Multiplying by denominator
(1)
xy-y=5-3x Expand and isolate
(1)
y(x-1)=5-3x y factored out
(1)
f^{-1}(x)=\frac{5-3x}{x-1}
(1)
Learning checklist
You have now learned how to:
- where appropriate, interpret simple expressions as functions with inputs and outputs
- interpret the reverse process as the ‘inverse function’
The next lessons are
Still stuck?
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.