Algebraic fractions

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

In order to access this I need to be confident with:

Fractions Simplifying fractions Adding and subtracting fractions Rearranging equations Solving equations Quadratic equations (factorisation) Quadratic formula Expanding brackets Simultaneous equations

This topic is relevant for:

GCSE Maths Algebra Algebraic Expressions Simplifying Expressions

Algebraic Fractions

Here we will learn about algebraic fractions, including operations with fractions, and solving linear and quadratic equations written in the form of algebraic fractions.

There are also algebraic fractions worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What are algebraic fractions?

Algebraic fractions are fractions that contain at least one variable.

The following algebraic expressions are examples of algebraic fractions:

$x$ is the numerator:

$\quad \quad \quad \frac{x}{12}$

Both the numerator and the denominator contain an $x$ term:

$\quad \quad \quad \frac{x+1}{2x}$

   An expression in    terms of $x$ is the    denominator:

    $\quad \quad \frac{3}{x+1}\$

   Both the numerator    and the    denominator    contain an    expression with $x$ :

    $\quad \quad \frac{3x+4}{2x-5}$

   The numerator is a multiple of $x$ :

    $\quad\quad \quad \quad \frac{2x}{15}$

   The numerator and the denominator are    quadratic expressions:

    $\quad\quad \quad \quad \frac{(x+3)^{2}}{x^{2}-9}$

The main aim of this lesson is to understand how to solve equations that include algebraic fractions.

All the examples above are expressions whereas the examples below are equations as we can find specific values for x for each example to solve the equation.

E.g.

One step equation:

$\quad \quad \frac{x}{12}=4$

A separate constant term:

$\quad \frac{x+1}{2x}+4=x$

   A quadratic    equation:

    $\quad\frac{3}{x+1}=x+5$

   A linear equation:

    $\quad \frac{3x+4}{2x-5}=6$

   A second fraction:

    $\quad \frac{2x}{15}=\frac{5x}{2}$

   Double brackets, difference of two    squares and simultaneous equations:

    $\quad \frac{(x+3)^{2}}{x^{2}-9}=2x-1$

It is important to be able to simplify algebraic fractions into their simplest form. If you need to practice this or need a quick refresher, see the lesson on simplifying algebraic fractions for further information.

Step-by-step guide: Simplifying algebraic fractions

What are algebraic fractions?

$What are algebraic fractions?$

How to solve equations including algebraic fractions

We need to be able to solve equations including algebraic fractions.

Let’s look at a simple example when $\frac{8}{x}=2$ .

Here, the denominator of the fraction contains the variable, so we first need to get the variable out of the denominator.

Rearranging the equation by multiplying both sides by $x$ and then dividing by $2$ , we get the value of $x=4$ .

We can substitute this into the original equation to prove that the answer is correct.

Here, $\frac{8}{4}=2$ so we have the correct answer.

We shall now consider more complicated cases when equations involve algebraic fractions.

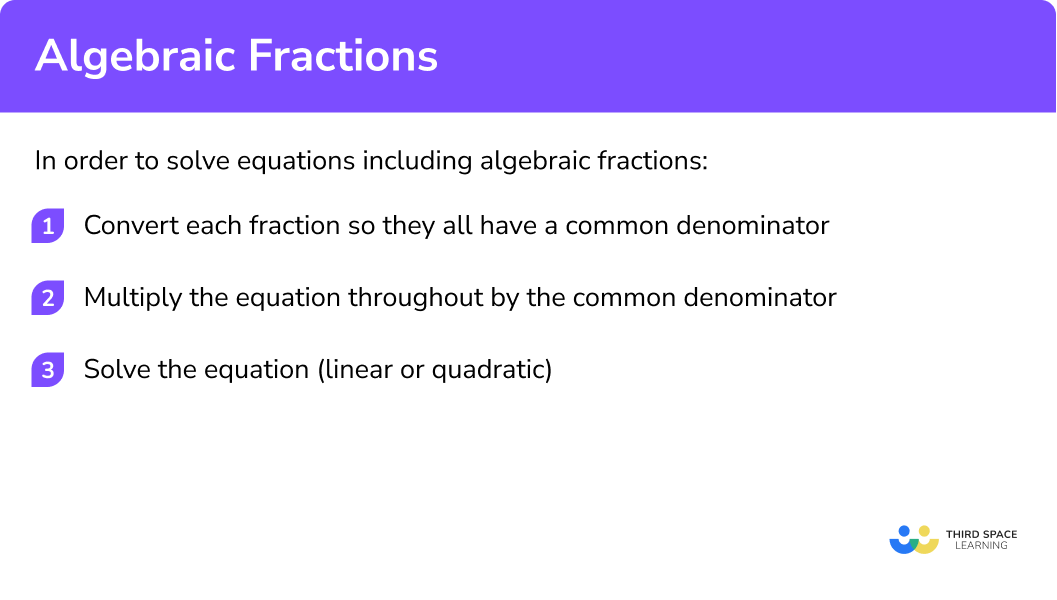
In order to solve equations including algebraic fractions.

Convert each fraction so they all have a common denominator.
Multiply the equation throughout by the common denominator.
Solve the equation (linear or quadratic).

Explain how to solve equations including algebraic fractions

$Explain how to solve equations including algebraic fractions$

$Algebraic fractions worksheet$

Algebraic fractions worksheet

$Algebraic fractions worksheet$

Get your free algebraic fractions worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

$Algebraic fractions worksheet$

Algebraic fractions worksheet

$Algebraic fractions worksheet$

Get your free algebraic fractions worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Algebraic fractions examples

Example 1: Equation with one fraction

Solve the equation

\frac{2x-1}{3}+x=3

Convert each fraction so they all have a common denominator.

Here, we only have one fraction and so we do not need to convert any other term into a fraction.

2Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $3$ (the denominator of the fractional term), we get

Make sure that you multiply every term in the equation by $3$ .

3Solve the equation (linear or quadratic).

You can check your solution by substituting the value for $x$ into the original equation and evaluating it.

Here,

Algebraic fractions examples 1 step 3 (2) 1

Example 2: Equation with two fractions

Solve the equation

\frac{x+1}{2}+\frac{x+3}{5}=6

Convert each fraction so they all have a common denominator.

Here, we have the two fractions with the denominators of $2$ and $5$ . The lowest common multiple of $2$ and $5$ is $10$ and so we can convert the two fractions so that they have the same denominator.

Remember to use brackets to ensure that you multiply the entire numerator by $5$ .

Remember to use brackets to ensure that you multiply the entire numerator by $2$ .

Algebraic fractions examples 2 step 1 (2) 1

We now have the equation

Algebraic fractions examples 2 step 1 (3) 1

Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $10$ (the denominator of the fractional terms), we get

Solve the equation (linear or quadratic).

You can check your solution by substituting the value for $x$ into the original equation and evaluating it.

Here,

Algebraic fractions examples 2 step 3 (2) 1

Example 3: Equation with x in the denominator

Solve the equation

3-\frac{5}{x+1}=1

Convert each fraction so they all have a common denominator.

Here, we have one fraction so we do not need to find a common denominator.

Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $x + 1$ (the denominator of the fractional terms), we get

Solve the equation (linear or quadratic).

You can check your solution by substituting the value for $x$ into the original equation and evaluating it.

Here,

Algebraic fractions examples 3 step 3 (2) 1

Example 4: Equation with three fractions

Solve the equation

\frac{1}{x}+\frac{1}{2x}+\frac{1}{3x}=11

Convert each fraction so they all have a common denominator.

Here, we need to find the lowest common multiple of $x, 2x,$ and $3x$ . As $x$ is the highest common factor, $x \times 1 \times 2 \times 3$ is the lowest common multiple, which is equal to $6x.$ .

So by multiplying the numerator and denominator of each fraction by a constant, we can convert each fraction to have the common denominator of $6x:$ .

We now have an equation which we can immediately simplify.

Algebraic fractions examples 4 step 1 (2) 1

Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $6x$ (the denominator of the fractional terms), we get

Solve the equation (linear or quadratic).

You can check your solution by substituting the value for $x$ into the original equation and evaluating it.

Here,

Algebraic fractions examples 4 step 3 (2) 1

Example 5: Denominators are expressions in terms of x

Solve the equation

\frac{x+1}{x+2}+\frac{3}{x-4}=1

Convert each fraction so they all have a common denominator.

Here, we need to find a common denominator for $(x + 2)$ and $(x - 4)$ .

The easiest way to do this is to multiply the two expressions together.

Top tip: do not expand the brackets too soon as you may be able to simplify the fraction before solving the equation.

By multiplying each fraction by the denominator of the other fraction, we get

We now have an equation which we can immediately simplify.

Algebraic fractions examples 5 step 1 (2) 1

Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $(x+2)(x-4)$ (the denominator of the fractional term), we get

Algebraic fractions - example 5 step 2 1

Solve the equation (linear or quadratic).

Algebraic fractions - example 5 step 3 1

You can check your solution by substituting the value for x into the original equation and evaluating it.

Here,

Algebraic fractions - example 5 step 3 (2) 1

Example 6: Equation including a quadratic

Solve the equation

\frac{9x+4}{x}=-2x

Convert each fraction so they all have a common denominator.

Here, we have a single fraction and so we do not need to find a common denominator.

Multiply the equation throughout by the common denominator.

Multiplying the equation throughout by $x$ (the denominator of the fractional terms), we get

Algebraic fractions - example 6 step 2 1

Solve the equation (linear or quadratic).

Algebraic fractions - example 6 step 3 1

You can check your solution by substituting the value for $x$ into the original equation and evaluating it.

Here, we have two possible solutions for $x$ so we can check both:

Algebraic fractions - example 6 step 3 (2) 1

Common misconceptions

Multiplying the numerator by the denominator

Let us look at example $2$ . When multiplying throughout by $10$ to remove the denominator from each fraction, the numerator has also been multiplied by $10$ .

This means that the fractions have been multiplied by $100$ , instead of $10$ , leaving the next line of work to be incorrect.

Algebraic Fractions Common Misconceptions image 1 1

Ignoring the denominators

When given an equation including an algebraic fraction, if the denominators are ignored the question will be answered incorrectly.

Algebraic Fractions Common Misconceptions image 2 1

Not multiplying all terms by the denominator

When rearranging an equation, the denominator is moved to the other side of the equals sign, instead of each term being multiplied by it. You must remember to multiply throughout by any value, not just the opposite side of the equals sign.

Algebraic Fractions Common Misconceptions image 3 1

Simplifying fractions incorrectly (1)

When adding two fractions, the denominator must be the same. A common misconception for adding two fractions is to add the numerators and the denominators together because this method is emphasised when looking at multiplying fractions.

Algebraic Fractions Common Misconceptions image 4 1

Simplifying fractions incorrectly (2)

Seeing the same term on the numerator and denominator allow the misconception that they can both be cancelled.

Algebraic Fractions Common Misconceptions image 5 1

Practice algebraic fractions questions

x=4

x=1

x=8

x=3

$Algebraic fractions practice questions 1 1$

x=20

x=7.5

x=45

x=22.5

$Algebraic fractions practice questions 2 1$

x=6

x=1.1

x=-\frac{39}{46}

x=0

$Algebraic fractions practice questions 3 1$

x=-1

x=2.5

x=\sqrt{11}

x=0

$Algebraic fractions practice questions 4 1$

x=14

x=\frac{14}{3}

x=-5\pm\sqrt{31}

x=-2

$Algebraic fractions practice questions 5 1$

$Algebraic fractions practice questions 5 (2) 1$

x=-3

x=\frac{23}{3}

x=-1\pm2\sqrt{23}

x=-\frac{23}{3}

$Algebraic fractions practice questions 6 1$

$Algebraic fractions practice questions 6 (2) 1$

Algebraic fractions GCSE questions

1. Arron earns $£40$ per hour. One day, he receives a bonus of $£5$ . He shares this day’s earnings equally with his brother and sister. If he gives away $£190$ , how many hours did Arron work that day?

(2 marks)

Show answer

2(\frac{40x+5}{3})=190

(1)

x=7

(1)

2. Use the quadratic formula to solve the equation

\frac{2x}{x+3} + \frac{5x}{x+2} = 4

(6 marks)

Show answer

$2x(x+2)+5x(x+3)=4(x+3)(x+2)$ or equivalent

(1)

7x^{2}+19x=4x^{2}+20x+24

(1)

3x^{2}-x-24=0

(1)

x=\frac{-1\pm\sqrt{1^{2}-4\times1\times{-24}}}{2\times{3}}

(1)

x=\frac{1\pm{17}}{6}

(1)

$x=3$ or $x=-\frac{8}{3}$

(1)

3. Two shapes given below have the same area. Calculate the value for $x$ .

$Algebraic fractions GCSE questions 3 1$

(7 marks)

Show answer

Area of Triangle $= \frac{2(x+8)}{2}=x+8$

(1)

Area of Square = $\left(\frac{3}{\sqrt{x}}\right)^{2}=\frac{9}{x}$

(1)

x+8=\frac{9}{x}

(1)

x^2+8x-9=0

(1)

(x+9)(x-1)=0

(1)

$x=1$ or $x=-9$

(1)

Conclusion: $x=1$ only

(1)

Learning checklist

You have now learned how to:

use algebraic methods to solve linear equations in 1 variable (including all forms that require rearrangement)

simplify and manipulate algebraic fractions

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Introduction

What are algebraic fractions?

How to solve equations including algebraic fractions

Algebraic fractions worksheet

Algebraic fractions examples

↓

Example 1: Equation with one fraction Example 2: Equation with two fractions Example 3: Equation with x in the denominator Example 4: Equation with three fractions Example 5: Denominators are expressions in terms of x Example 6: Equation including a quadratic

Common misconceptions

Practice algebraic fractions questions

Algebraic fractions GCSE questions

Learning checklist

Next lessons

Still stuck?