GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Area of a rectangle 3D trigonometry Faces, edges and vertices How to calculate surface area

This topic is relevant for:

GCSE Maths Geometry and Measure 3D Shapes Triangular Prism

Surface Area

Surface Area Of A Triangular Prism

Here we will learn about the surface area of a triangular prism and how to calculate it.

There are also volume and surface area of a triangular prism worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is the surface area of a triangular prism?

The surface area of a triangular prism is the total area of all of the faces.

To work out the surface area of a triangular prism, we need to work out the area of each face and add them all together.

Lateral faces are all of the faces of an object excluding the top and the base. For a triangular prism the top and the base are triangles and the lateral faces are rectangular sides. The lateral surface area of a triangular prism is the total area of the rectangular sides

The triangular faces of a triangular prism are congruent (exactly the same) but, unless the triangle is isosceles or equilateral, the rectangles are all different.

E.g.

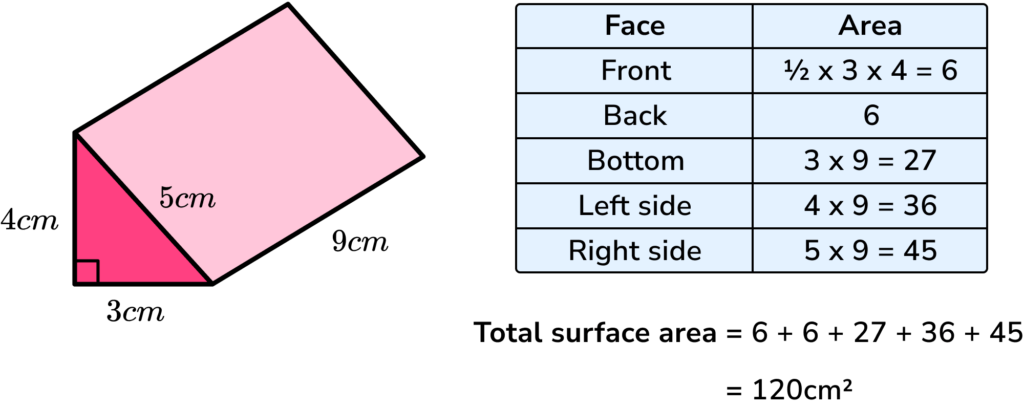
Since it is an area, surface area is measured in square units (e.g. $mm^2, cm^2, m^2$ etc).

What is the surface area of a triangular prism?

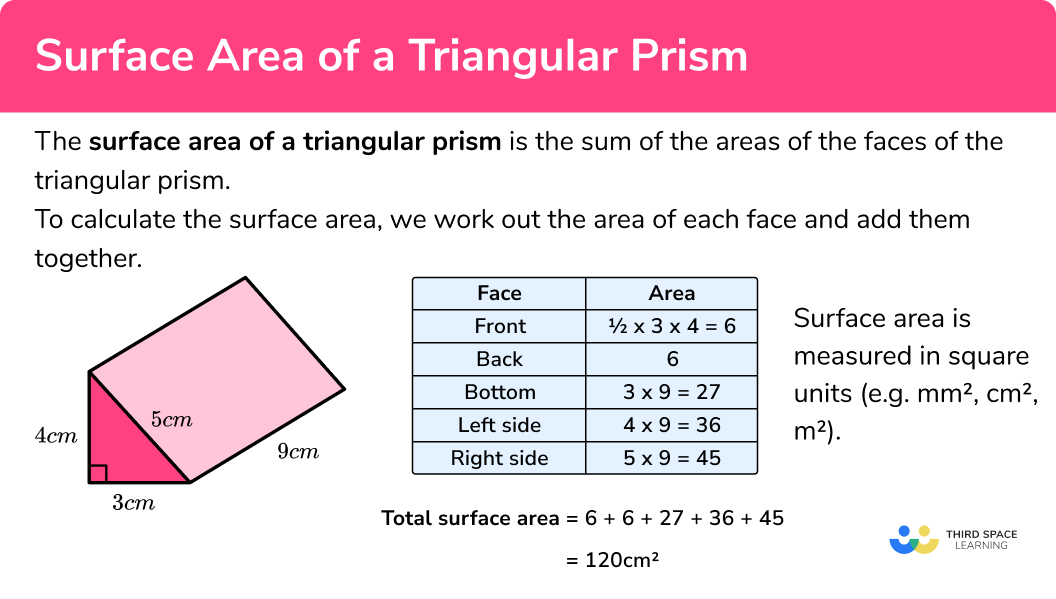
How to calculate the surface area of a triangular prism

In order to work out the surface area of a triangular prism:

Work out the area of each face.
Add the five areas together.
Include the units.

How to calculate the surface area of a triangular prism

Surface area of a triangular prism worksheet

Get your free Surface area of a triangular prism worksheet of 20+ questions and answers. Includes reasoning and applied questions.

COMING SOON

Surface area of a triangular prism worksheet

Get your free Surface area of a triangular prism worksheet of 20+ questions and answers. Includes reasoning and applied questions.

COMING SOON

Surface area of a triangular prism examples

Example 1: finding the surface area of a triangular prism with a right triangle

Work out the surface area of the triangular prism

Work out the area of each face.

The area of the triangle at the front is $\frac{1}{2}\times12\times5 = 30cm^{2}$

The back face is the same as the front face so the area of the back is also $30cm^{2}$ .

The area of the base is $9\times12=108cm^{2}$

The area of the left side is $9\times5=45cm^{2}$

The area of the top is $9\times13=117cm^{2}$

It will make our working clearer if we use a table:

Face	Area
Front	½ × 12 × 12 × 5 = 30
Back	30
Bottom	9 × 12 = 108
Left side	9 × 5 = 45
Top	9 × 13 = 117

2Add the five areas together.

Total surface area: $30 + 30 + 108 + 45 + 117 = 330$

3Include the units.

The measurements on the triangular prism are in cm therefore the total surface area of the triangular prism = $330cm^{2}$ .

Example 2: surface area of a triangular prism with an isosceles triangle

Work out the surface area of the triangular prism

Work out the area of each face.

Face	Area
Front	½ × 6 × 9.5 = 28.5
Back	28.5
Bottom	6 × 14 = 84
Left side	10 × 14 = 140
Right side	10 × 14 = 140

Add the five areas together.

Total surface area: $28.5 + 28.5 + 84 + 140 + 140 = 421$

Include the units.

The measurements on the triangular prism are in mm therefore the total surface area of the triangular prism = $421mm^{2}$ .

Example 3: surface area of a right triangular prism

This prism has a triangular base and rectangular sides. We are told the height of the prism is $9cm$ . We can work out the surface area in exactly the same way, we just adjust the labels we give to each face in our table.

Work out the area of each face.

Face	Area
Top	½ × 6 × 8 = 24
Bottom	24
Front	9 × 10 = 90
Left side	9 × 6 = 54
Right side	9 × 8 = 72

Add the five areas together.

Total surface area: $24 + 24 + 90 + 54 + 72 = 264$

Include the units.

The measurements on the triangular prism are in cm therefore the total surface area of the triangular prism = $264cm^{2}$ .

Example 4: surface area of a triangular prism

Work out the surface area of the triangular prism

Work out the area of each face.

Face	Area
Front	½ × 35 × 12 = 210
Back	210
Bottom	40 × 35 = 1400
Left side	40 × 12 = 480
Top	40 × 37 = 1480

Add the five areas together.

Total surface area: $210 + 210 + 1400 + 480 + 1480 = 3780$

Include the units.

The measurements on the triangular prism are in m therefore the total surface area of the triangular prism = $3780m^{2}$ .

Example 5: surface area of a triangular prism with different units

Work out the surface area of the triangular prism. Give your answer in $mm^{2}$ .

Work out the area of each face.

Some of the measurements here are in cm and some are in mm. Since we have been asked for the answer in $mm^{2}$ , we need to convert all measurements to $mm$ :

$1.4cm = 14mm$ and $2.5cm=25mm$ .

Face	Area
Front	½ × 8 × 14 = 56
Back	56
Bottom	25 × 8 = 200
Left side	25 × 14 = 350
Right side	25 × 16.1 = 402.5

Add the five areas together.

Total surface area: $56 + 56 + 200 + 350 + 402.5 = 1064.5$

Include the units.

The measurements we have used are in mm therefore the total surface area of the triangular prism = $1064.5mm^{2}$ .

Example 6: surface area when there is a missing length

Work out the surface area of the triangular prism. Give your answer in $cm^{2}$ .

Work out the area of each face.

When calculating the surface area we need the length of each side of the triangle.

We are told the base and height of the triangle and the length of the prism but we don’t have the length of the hypotenuse of the triangle.

To work this out we can use the Pythagorean Theorem $a^{2}+b^{2}=c^{2}$ .

\[ 8^{2}+15^{2} = c^{2} \\ 289 = c^{2} \\ \sqrt{289} = c \\ 17 = c \]

Face	Area
Front	½ × 8 × 15 = 60
Back	60
Bottom	9 × 8 = 72
Left side	9 × 15 = 135
Right side	9 × 17 = 153

Add the five areas together.

Total surface area: $60 + 60 + 72 + 135 + 153 = 480$

Include the units.

The measurements we have used are in cm therefore the total surface area of the triangular prism = $480cm^{2}$ .

Common misconceptions

Calculating volume instead of surface area

Volume and surface area are different things – volume tells us the space within the shape whereas surface area is the total area of the faces. To find surface area, work out the area of each face and add them together.

Thinking all of the rectangles have the same area

Usually all of the rectangle have different areas (unless the triangle is isosceles or equilateral).

Using the wrong measurements to work out the area of the triangle faces

In surface area questions, we need to know all three side lengths of the triangle however we only need the base and the height to calculate the area of the triangle

Surface area of a triangular prism is part of our series of lessons to support revision on triangular prism. You may find it helpful to start with the main triangular prism lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Practice surface area of a triangular prism questions

$1200 \mathrm{cm}^{2}$

$1040 \mathrm{cm}^{2}$

$920 \mathrm{cm}^{2}$

$1140 \mathrm{cm}^{2}$

Work out the surface area of each face:

Face	Area
Front	½ × 8 × 15 = 60
Back	60
Bottom	20 × 8 = 160
Left side	20 × 15 = 300
Right side	20 × 17 = 340

\text{Total surface area }=60+60+160+300+340=920\mathrm{cm}^{2}

$720 \mathrm{cm}^{2}$

$672 \mathrm{cm}^{2}$

$546 \mathrm{cm}^{2}$

$576 \mathrm{cm}^{2}$

Work out the surface area of each face:

Face	Area
Front	½ × 12 × 8 = 48
Back	48
Bottom	15 × 12 = 180
Left side	15 × 10 = 150
Right side	15 × 10 = 150

\text{Total surface area }=48+48+180+150+150=576\mathrm{cm}^{2}

$82 \mathrm{cm}^{2}$

$94 \mathrm{cm}^{2}$

$36 \mathrm{cm}^{2}$

$66 \mathrm{cm}^{2}$

Work out the surface area of each face:

Face	Area
Front	½ × 3 × 4 = 6
Back	6
Top	6 × 3 = 18
Left side	6 × 4 = 24
Right side	6 × 5 = 30

\text{Total surface area }=6+6+18+24+30=84\mathrm{cm}^{2}

7.007 \mathrm{cm}^{2}

14.014 \mathrm{cm}^{2}

24.04 \mathrm{cm}^{2}

30.89 \mathrm{cm}^{2}

Work out the surface area of each face.

Face	Area
Front	½ × 2.6 × 4.9 = 6.37
Back	6.37
Bottom	1.1 × 2.6 = 2.86
Left side	1.1 × 4.9 = 5.39
Right side	1.1 × 5.5 = 6.05

\text{Total surface area }=6.37+6.37+2.86+5.39+6.05=27.04\mathrm{cm}^{2}

$15200 \mathrm{cm}^{2}$

$14400 \mathrm{cm}^{2}$

$2448.8 \mathrm{cm}^{2}$

$96000 \mathrm{cm}^{2}$

Notice that some of the measurements are in $m$ and some are in $cm$ . Since we are asked to give the answer in square centimetres, we need to convert all the measurements to $cm$ .

$0.5m = 50cm$ and $0.8m=80cm$ .

Next, Work out the surface area of each face:

Face	Area
Front	½ × 60 × 40 = 1200
Back	1200
Bottom	80 × 60 = 4800
Left side	80 × 50 = 4000
Right side	80 × 50 = 4000

\text{Total surface area }=1200+1200+4800+4000+4000=15200 \mathrm{cm}^{2}

$330 \mathrm{m}^{2}$

$936 \mathrm{m}^{2}$

$1560 \mathrm{m}^{2}$

$660 \mathrm{m}^{2}$

In this question, we are missing the height of the triangle. Since it is a right angled triangle, we can use Pythagoras’ theorem to work out the height:

\begin{aligned} a^{2}+b^{2}&=c^{2}\\ h^{2}+12^{2}&=13^{2}\\ h^{2}+144&=169\\ h^{2}&=169-144\\ h^{2}&=25\\ h&=5 \mathrm{m} \end{aligned}

Next, work out the surface area of each face:

Face	Area
Front	½ × 12 × 5 = 30
Back	30
Bottom	20 × 12 = 240
Top	20 × 13 = 260
Left side	20 × 5 = 100

\text{Total surface area }=30+30+240+260+100=660 \mathrm{m}^{2}

Surface area of a triangular prism GCSE questions

1. Work out the surface area of the triangular prism.

(3 marks)

Show answer

$\frac{1}{2} \times 0.3 \times 0.4 = 0.06$

(1)

$1 \times 0.3=0.1, ~1 \times 0.4 – 0.4,~ 1 \times 0.5 = 0.5$

(1)

$0.06+0.06+0.3+0.3+0.5=1.32 \mathrm{m}^{2}$

(1)

2. A packaging company wants to minimise the amount of packaging they use. Which of these shapes should they choose to make their packaging? Show how you decide.

(5 marks)

Show answer

Prism A: Surface area = $24+24+90+120+150$

(1)

$\text{Surface area }=408 \mathrm{cm}^{2}$

(1)

Prism B: Surface area = $25+25+140+70+156.8$

(1)

$\text{Surface area }=416.8 \mathrm{cm}^{2}$

(1)

They should use shape $A$

(1)

3. A packaging box is made in the following shape

The material used to make the box costs $0.15p$ per $\mathrm{cm}^{2}$ to produce. How much would it cost to make $100$ boxes? Give your answer in pounds.

(5 marks)

Show answer

Surface area = $12+12+88+55+55$

(1)

$\text{Surface area }=222 \mathrm{cm}^{2}$

(1)

$222 \times 0.15 = 33.3$

(1)

$33.3 \times 100=3330 \text{p}$

(1)

$3330p=£33.30$

(1)

Learning checklist

You have now learned how to:

Calculate the surface area of a triangular prism

Use the properties of faces, surfaces, edges and vertices of cubes and cuboids to solve problems in 3-D

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.