Sphere

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Multiplying fractions Substitution Area of a circle Rounding

This topic is relevant for:

GCSE Maths Geometry and Measure 3D Shapes

Sphere

Here we will learn about spheres, including how to find the volume and surface area of a sphere.

There are also sphere worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

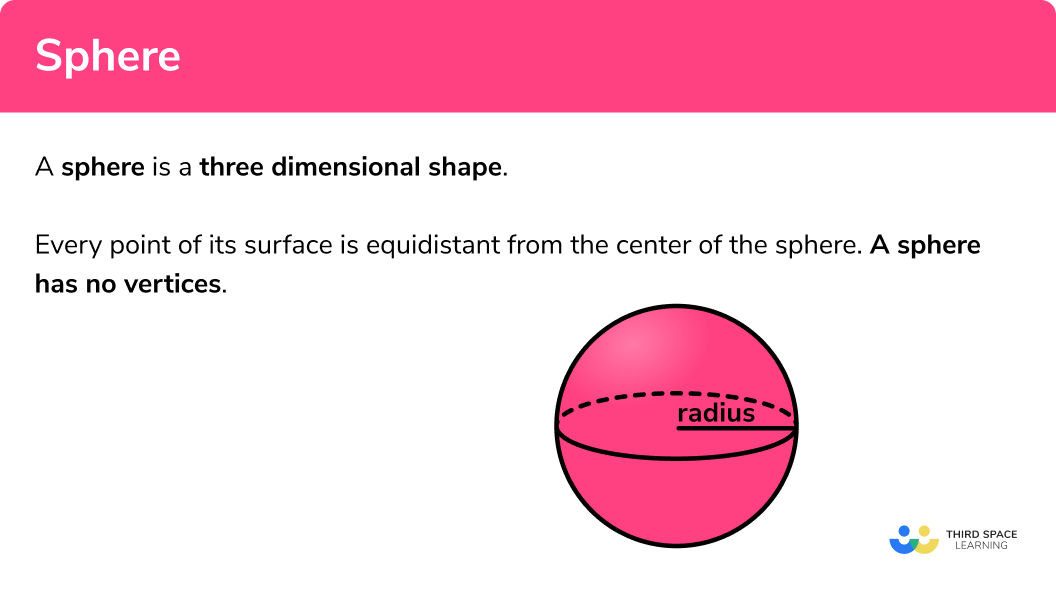
What is a sphere shape?

A sphere shape is a three dimensional shape where every point of its surface is equidistant (the same distance) from the centre of the sphere. The distance from the centre of a sphere to its surface is called the radius. A sphere has no vertices.

A hemisphere is half a sphere.

What is a sphere shape?

Volume of a sphere shape

The volume of a sphere is the amount of space there is inside a sphere.

The formula for the volume of a sphere is:

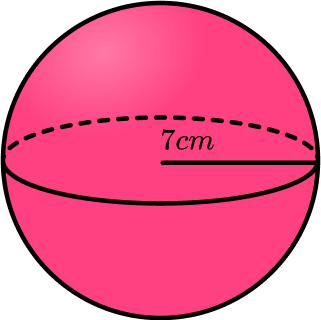
\text{Volume}=\frac{4}{3}\pi{r}^3

E.g. Find the volume of the sphere

This sphere has a radius of $7 \; cm$ .

\begin{aligned} \text{Volume}&=\frac{4}{3} \pi r^3 \\\\ &= \frac{4}{3} \times \pi \times 7^3\\\\ &=\frac{1372}{3}\pi\\\\ &=1436.755... \\\\ &=1440 \ cm^3 \ \text{(3sf)} \end{aligned}

Step-by-step guide: Volume of a sphere

Surface area of a sphere shape

The surface area of a sphere is the area which covers the outer surface of a sphere.

The formula for the surface area of a sphere is:

\text{Surface Area}=4 \pi r^2

E.g. Find the surface area of the sphere

This sphere has a radius of $7 \; cm$ .

\begin{aligned} \text{Surface area}&=4\pi r^2\\\\ &=4 \times \pi \times 7^2\\\\ &=196\pi\\\\ &=615.752...\\\\ &=616 \ cm^2 \ \text{(to 3 sf)} \end{aligned}

Step-by-step guide: Surface area of a sphere

How to calculate the volume or surface area of a sphere

In order to calculate the volume of a sphere or the surface area of a sphere:

Write down the formula.
Substitute the given values.
Work out the calculation
Write the final answer.

How to calculate the volume or surface area of a sphere

Volume and surface area of a sphere worksheet

Get your free sphere shape worksheet of 20+ volume and surface area of a sphere questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Volume and surface area of a sphere worksheet

Get your free sphere shape worksheet of 20+ volume and surface area of a sphere questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Sphere shape examples

Example 1: volume of a sphere

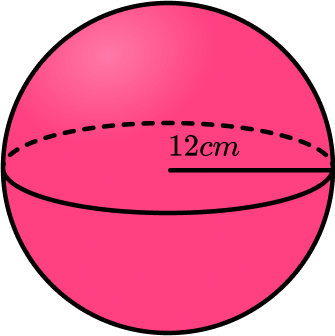
Calculate the volume of a sphere of radius $12 \; cm.$ Give your answer to $3$ significant figures.

Write down the formula for the volume of a sphere.

\text{Volume}=\frac{4}{3} \pi r^3

2Substitute the given values into the formula.

V=\frac{4}{3} \times \pi \times 12^3

3Complete the calculation.

\begin{aligned} &=2304\pi \\\\ &=7238.229... \end{aligned}

4Write the final answer, including the units.

The radius is given in centimetres so the volume will be cubic centimetres.

The volume of the sphere is $7240 \; cm^3 (3 sf)$ .

Example 2: volume of a sphere, given the diameter

Calculate the volume of a sphere with a diameter of $30 \; cm$ . Write your answer in terms of $\pi$ .

Write down the formula for the volume of a sphere.

\text{Volume}=\frac{4}{3} \pi r^3

Substitute the given values into the formula.

The diameter is twice the length of the radius. To calculate the radius, we need to first divide the diameter by $2:$

$30 \div 2=15 \; cm$

The radius is $15 \; cm.$ Substituting this into the formula for the volume of a sphere, we have

$V=\frac{4}{3} \times \pi \times 15^3$

Complete the calculation.

V=4500\pi

Write the final answer, including the units.

The radius is given in centimetres so the volume will be cubic centimetres.

The volume of the sphere is $4500\pi \; cm^3$ .

Example 3: volume of a hemisphere

Calculate the volume of a hemisphere of radius $4 \; cm$ . Write your answer to $2$ decimal places.

Write down the formula for the volume of a sphere.

\text{Volume}=\frac{4}{3} \pi r^3

Substitute the given values into the formula.

V=\frac{4}{3} \times \pi \times 4^3

Complete the calculation.

V=268.0825...

But this is for a whole sphere. We need a hemisphere, so we need to divide by $2.$

$268.0825…\div 2=134.041...$

Write the final answer, including the units.

The radius is given in centimetres so the volume will be cubic centimetres.

The volume of the hemisphere is $134.04 \; cm^3 \; (to \; 2 dp).$

Example 4: surface area of a sphere

Calculate the surface area of a sphere with radius $7 \; cm$ . Write your answer to $1$ decimal place.

Write down the formula for the surface area of a sphere.

\text{Surface Area }=4\pi{r}^{2}

Substitute the given values into the formula.

Here we know the radius of the sphere is $7 \; cm$ so we substitute $r=7$ into the formula for the surface area.

$SA=4\times\pi\times{7}^{2}$

Complete the calculation.

\begin{aligned} SA&=196\pi\\\\ &=615.7521601… \end{aligned}

Write the final answer, including the units.

The radius is measured in centimetres so the surface area is measured in square centimetres $(cm^2)$ , with the solution rounded to $1$ decimal place.

The surface area of the sphere is $615.8 \; cm^2$ .

Example 5: surface area of a sphere given the diameter – in terms of π

Find the surface area of a sphere with the diameter of $20 \; cm.$ Leave your answer in terms of $\pi$ .

Write down the formula for the surface area of a sphere.

\text{Surface Area }=4\pi{r}^{2}

Substitute the given values into the formula.

The diameter of the sphere is $20\;cm$ and so we need to use this to calculate the radius and hence the surface area of the sphere.

$20 \div 2=10$

The radius of the sphere is $10\;cm.$

We now know the radius of the sphere is $10\;cm$ so we substitute $r=10$ into the formula for the surface area.

$SA=4\times\pi\times{10}^{2}$

Complete the calculation.

SA=400\pi

Write the final answer, including the units.

The radius is measured in centimetres so the surface area is measured in square centimetres $(cm^2)$ , with the solution in terms of $\pi$ .

The surface area of the sphere is $400\pi \;cm^2$ .

Example 6: total surface area of a hemisphere

Find the total surface area of a hemisphere with a radius $4 \; cm.$ Write your answer to $3$ significant figures.

Write down the formula for the surface area of a sphere.

\text{Surface Area }=4\pi{r}^{2}

For the surface area of a hemisphere we need half of the surface area of a sphere and add it on to the area of the flat circular base. The formula can be adapted.

$\text{Total Surface Area}=\frac{1}{2}\times 4\pi r^2 + \pi r^2$

Substitute the given values into the formula.

\text{Total SA}=2 \times \pi \times 4^2+\pi \times 4^2

Complete the calculation.

\begin{aligned} \text{Total SA}&=48\pi\\\\ &=150.796… \end{aligned}

Write the final answer, including the units.

The radius is measured in centimetres so the surface area is measured in square centimetres $(cm^2)$ , with the solution rounded to $3$ significant figures.

The surface area of the sphere is $151 \; cm^2 \; (to \; 3 sf)$ .

Common misconceptions

Using the correct formula

There are several formulas that can be used, so we need to match the correct formula to the correct context.

Rounding

It is important to not round the answer until the end of the calculation. This will mean your final answer is accurate.

Make sure you have the correct units

For area we use square units such as $cm^2, m^2, km^2.$

For volume we use cube units such as $cm^3, m^3, km^3.$

Using the radius or the diameter

It is a common error to mix up radius and diameter. Remember the radius is half of the diameter.

Practice sphere shape questions

333.04 \; cm^3

330 \; m^3

58.09 \; cm^3

232.35 \; cm^3

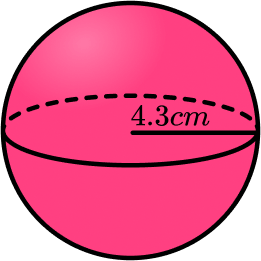
We are finding the volume of a sphere so we substitute the value of the radius $r=4.3$ into the formula $V=\frac{4}{3}\pi{r}^{3}$

\begin{aligned} \text{Volume}&=\frac{4}{3} \pi r^3 \\\\ &= \frac{4}{3} \times \pi \times 12^3\\\\ &=333.0381428… \\\\ &=333.04\text{cm}^{3} \ \text{(2dp)} \end{aligned}

432\pi \; cm^3

1944\pi \; m^3

7776\pi \; cm^3

5832\pi \; cm^3

We are finding the volume of a sphere so we substitute the value of the radius into the formula $V=\frac{4}{3}\pi{r}^{3}$ . As the diameter is twice the length of the radius, $r=36 \div 2=18 \;cm.$

\begin{aligned} \text{Volume}&=\frac{4}{3} \pi r^3 \\\\ &= \frac{4}{3} \times \pi \times 18^3\\\\ &=7776\pi \\\\ &=7776\pi \; cm^3 \end{aligned}

362.3 \; cm^3

998.3 \; m^3

499.2 \; cm^3

120.8 \; cm^3

We are finding the volume of a hemisphere, so we need to calculate half of the volume of a sphere using the radius $r=6.2 \; cm,$ and the formula: $V=(\frac{4}{3}\pi{r}^3)\div{2}.$

\begin{aligned} V&=(\frac{4}{3}\pi{r}^3)\div{2}\\\\ &=(\frac{4}{3}\times\pi\times{6.2}^3)\div{2}\\\\ &=499.152996…\\\\ &=499.2\text{ cm}^3 \end{aligned}

270\text{ cm}^2

407.72\text{ cm}^2

66.48\text{ cm}^2

265.90\text{ cm}^2

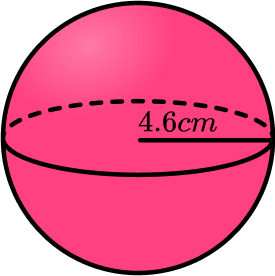
We are finding the surface area of a sphere, so we substitute the value of the radius $r=4.6$ into the formula $SA=4\pi r^2.$

\begin{aligned} \text{Surface area}&=4\pi r^2\\\\ &=4 \times \pi \times 4.6^2\\\\ &=265.9044022…\\\\ &=265.90 \text{ cm}^2 \ \text{(2dp)} \end{aligned}

1024\pi\text{ mm}^2

64\pi\text{ mm}^2

256\pi \text{ mm}^2

2144\pi\text{ mm}^2

We are finding the surface area of a sphere, so we need to calculate the value of the radius using the diameter, then substitute the value of the radius into the formula for the surface area.
$r=16 \div 2=8 \; mm$

The radius $r=8 \; mm.$

\begin{aligned} \text{Surface area}&=4\pi r^2\\\\ &=4 \times \pi \times 8^2\\\\ &=256\pi \ \text{mm}^2 \end{aligned}

573.4 \text{ cm}^2

764.5 \text{ cm}^2

191.1 \text{ cm}^2

993.9 \text{ cm}^2

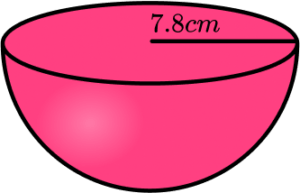
We need to find the curved surface area by finding half of the surface area of a sphere. Then we add on the area of the circular top.

\begin{aligned} \text{Total surface area}&=3\pi r^2\\\\ &=3 \times \pi \times{7.8}^2\\\\ &=573.4034911…\\\\ &=573.4 \text{ cm}^2 \ \text{(1dp)} \end{aligned}

Sphere shape GCSE questions

\text{Surface area of a sphere}=4 \pi r^2

\quad \quad \quad \text{Volume of a sphere}=\frac{4}{3} \pi r^3

1. Here is a sphere with a radius $8.4 \; cm.$

Sphere GCSE Question 1

Calculate the volume of the sphere.

Write your answer to $1$ decimal place.

(2 marks)

Show answer

V=\frac{4}{3} \times \pi \times 8.4^3

(1)

2482.71271…=2482.7 \; cm^3

(1)

2. A tennis ball has a radius of $3.4 \; cm.$ Each tennis ball is covered in felt. Calculate the area of the felt required to cover the ball to the nearest square millimetre.

Sphere GCSE Question 4.1

(4 marks)

Show answer

3.4 \; cm=34 \; mm

(1)

SA=4\times \pi \times{34}^2

(1)

14526.72443\dots

(1)

14527 \; mm^2

(1)

3. A container is a hemisphere with a diameter of $70 \; cm.$

Sphere GCSE Question 3

Sand fills the container at a rate of $5000 \; cm^3$ per minute.

How long does it take to fill the container?

Give your answer to the nearest minute.

(3 marks)

Show answer

(\frac{4}{3} \times \pi \times 35^3) \div{2} = 89797.19002…

(1)

89797.19002… \div{5000}=17.959438…

(1)

$17.959438\dots = 18$ minutes

(1)

4. A sphere has a surface area of $1000 \; m^2.$

Calculate its volume.

Give your answer to $3$ significant figures.

(3 marks)

Show answer

r=\sqrt{\frac{1000}{4\pi}}=8.920620581…

(1)

\frac{4}{3}\times \pi \times8.920620581^3=2973.540194…

(1)

2973.540194…=2970 \; m^3 \; (3sf)

(1)

Learning checklist

You have now learned how to:

Find the volume of a sphere

Find the surface area of a sphere

How to solve problems involving spheres

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.