GCSE Tutoring Programme
"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."
In order to access this I need to be confident with:
3D shapes Faces, edges and vertices Area of a quadrilateral Substitution Rearranging formulae Changing the subject of a formulaThis topic is relevant for:

Square Based Pyramid
Here we will learn about square based pyramids, including what they are and what features they have.
There are also square based pyramid worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
What is a square based pyramid?
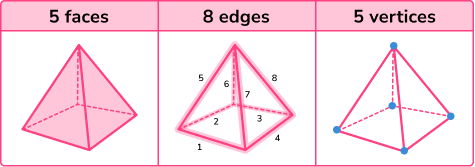
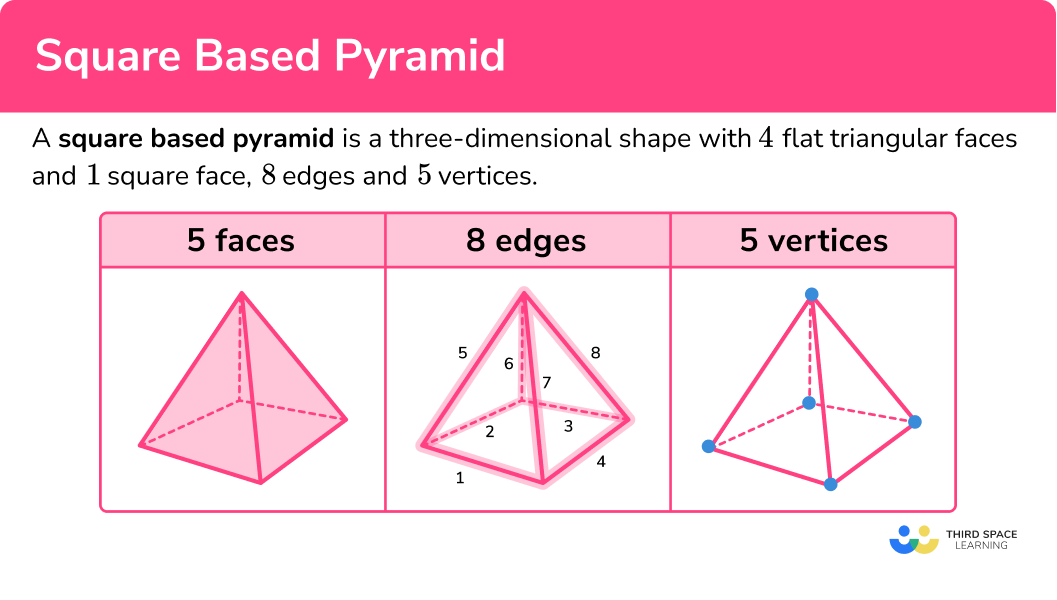
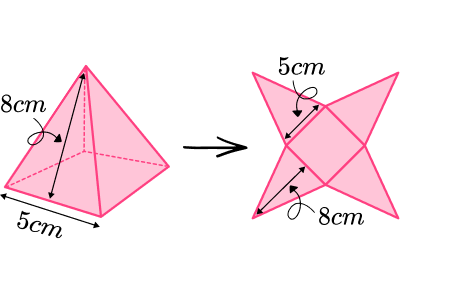
A square based pyramid is a three-dimensional shape with 4 flat triangular faces and 1 square face, 8 edges and 5 vertices.
It is sometimes called pentahedron because it has 5 faces.
A pyramid is a polyhedra with a flat base and triangular sides which meet at the apex. The flat base can be any polygon. There are different types of pyramids such as a triangular pyramid (known as a tetrahedron), a rectangular pyramid or even a hexagonal pyramid or a pentagonal pyramid.

What is a square based pyramid?
Net of a square based pyramid
The net of a square based pyramid is made of 4 triangles and a square.

Volume of a square based pyramid
The volume of a square based pyramid can be found by using the formula
\text{Volume}=\cfrac{1}{3}\times \text{area of base} \times \text{height}.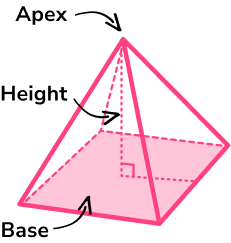
The pyramid height should be perpendicular to its base.
This can be written as
V=\cfrac{1}{3}Bh ,
where
- V represents the volume of the pyramid,
- B represents the area of the base of the pyramid,
- h represents the perpendicular height of the pyramid.
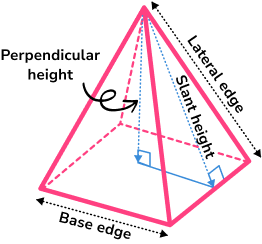
You may not be given the perpendicular height. You may need to apply Pythagoras’ theorem to find it if you are given the slant height or the lateral edge length.
Surface area of a square based pyramid
The surface area of a square based pyramid is calculated by finding the area of each of the faces and adding them together.
For example,
Find the surface area of the square based pyramid.
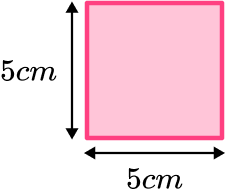
Area of a square = 5 \ cm \times 5 \ cm=25 \ cm^2
Area of a triangle = \cfrac{5 \ cm \ \times \ 8 \ cm}{2}=20 \ cm^2
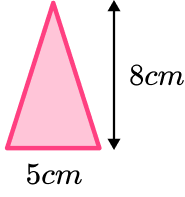
There are 4 triangular faces so multiply 20 \ cm^2 by 4 to give 80 \ cm^{2}.
Total surface area = 25 \ cm^{2}+80 \ cm^{2}=105 \ cm^2
Right square pyramid
A right square pyramid is a square based pyramid where the apex is directly above the centre of the square base, forming a right-angled triangle. The other 4 faces are isosceles triangles.
Oblique square pyramid
An oblique square pyramid is a square based pyramid where the apex is NOT directly above the centre of the square base.
How to calculate the volume of a square based pyramid
In order to calculate the volume of a square based pyramid:
- Calculate the area of the base.
- Substitute values into the formula and solve.
- Write the answer, including the units.
Explain how to calculate the volume of a square based pyramid

Volume and surface area of pyramids worksheet (includes square based pyramid)

Get your free square based pyramid worksheet of 20+ volume and surface area of pyramids questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Volume and surface area of pyramids worksheet (includes square based pyramid)

Get your free square based pyramid worksheet of 20+ volume and surface area of pyramids questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREESquare based pyramid examples
Example 1: calculating the volume given the height
Find the volume of the square based pyramid.
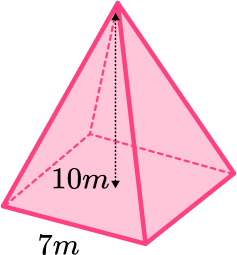
- Calculate the area of the base.
The area of the base can be found by squaring the base side.
7^2=492Substitute values into the formula and solve.
The volume of a square based pyramid can be found by using the formula,
\begin{aligned} V&=\cfrac{1}{3}Bh \\\\ V&=\cfrac{1}{3}\times 49\times 10 \\\\ V&=163.333… \end{aligned}3Write the answer, including the units.
The volume is 163.3 \ m^3 (to 1 dp).
Example 2: calculating the volume not given the height
Find the volume of the square based pyramid. The apex is directly above the centre of the square base.

Calculate the area of the base.
The area of the base can be found by squaring the base edge.
8^2=64
Substitute values into the formula and solve.
The volume of a square based pyramid can be found by using the formula,
V=\cfrac{1}{3}Bh.
We do not know the perpendicular height. We have been given the lateral edge length. We will need to apply Pythagoras’ theorem twice to find the perpendicular height. The value 4 comes from using the midpoint of the base edge to make right angled triangles.
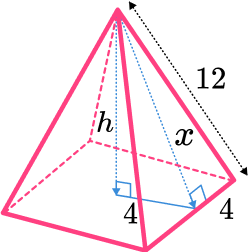
First we find the slant length from the lateral edge length.
x=\sqrt{12^2-4^2}=8\sqrt{2}
Then we can find the perpendicular height of the square based pyramid.
h=\sqrt{(8\sqrt{2})^2-4^2}=4\sqrt{7}
\begin{aligned} V&=\cfrac{1}{3}Bh \\\\ V&=\cfrac{1}{3}\times 64\times 4\sqrt{7} \\\\ V&=225.770… \end{aligned}
Write the answer, including the units.
The volume is 225.8 \ cm^3 (to 1 dp).
Example 3: calculating the height given the volume
The volume of the square based pyramid is 740 \ cm^{3}. Find the perpendicular height h.
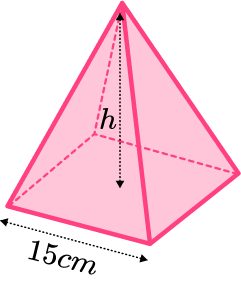
Calculate the area of the base.
The area of the base can be found by squaring the base edge.
15^2=225
Substitute values into the formula and solve.
The formula for the volume of the square based pyramid can be used to find the perpendicular height h.
\begin{aligned} V&=\cfrac{1}{3}Bh \\\\ 740&=\cfrac{1}{3}\times 225\times h \\\\ h&=\cfrac{740\times 3}{225} \\\\ h&=9.8666… \end{aligned}
Write the answer, including the units.
The perpendicular height h is 9.87 \ cm (to 3 sf).
Example 4: calculating the length of the base given the volume
The volume of the square based pyramid is 570 \ cm^{3}. Find the base edge.
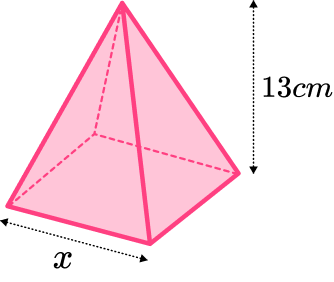
Calculate the area of the base.
The area of the base can not be found yet. But, when we find it we can square root it to find the length of the base (the base edge).
Substitute values into the formula and solve.
The formula for the volume of the square based pyramid can be used to find the base area.
\begin{aligned} V&=\cfrac{1}{3}Bh \\\\ 570&=\cfrac{1}{3}\times B\times 13 \\\\ h&=\cfrac{570\times 3}{13} \\\\ h&=131.5384… \end{aligned}
We can then square root the base area to find the length of the base.
\sqrt{131.5384…}=11.469…
Write the answer, including the units.
The length of the base is 11.5 \ cm (to 3 sf).
Example 5: working out the total surface area of a square pyramid
Find the total surface area of this square based pyramid.
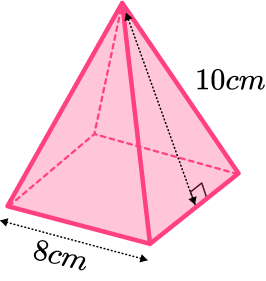
Calculate the area of the base.
The area of the base can be found by squaring the base edge.
8^2=64
Substitute values into the formula and solve.
The area of the triangular faces needs to be found. This is the lateral surface area.
We can find the area of one triangular face and then multiply it by four. We have the slant height which can be used to find the area of one triangular face. The triangular faces are all congruent isosceles triangles.
\begin{aligned} A&=\cfrac{1}{2}bh \\\\ A&=\cfrac{1}{2}\times 8\times 10 \\\\ A&=40 \end{aligned}
We can then multiply the area of one triangular face by 4 and add the area of the square base to find the total surface area.
4\times 40+64=224
Write the answer, including the units.
The total surface area is 224 \ cm^{2}.
Example 6: working out the total surface area using Pythagoras’ theorem
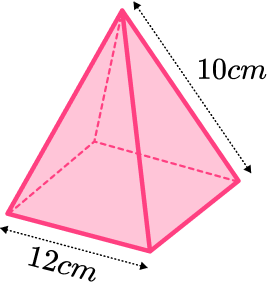
Calculate the area of the base.
The area of the base can be found by squaring the base edge.
12^2=144
Substitute values into the formula and solve.
The area of the triangular faces needs to be found. This is the lateral surface area.
We can find the area of one triangular face and then multiply it by four. We need to find the slant height which can be used to find the area of one triangular face. The triangular faces are all congruent isosceles triangles.
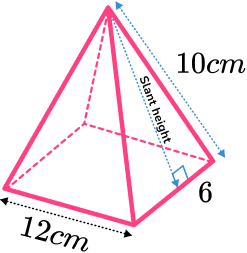
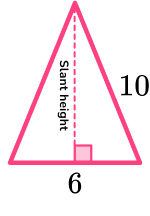
Using Pythagoras’ theorem, we can find perpendicular height of the triangle.
\text{slant height}=\sqrt{10^2-6^2}=8
The area of the triangle is then found.
\text{Area}=\cfrac{1}{2}bh=\cfrac{1}{2}\times 12\times 8=48
We can then multiply the area of one triangular face by 4 and add the area of the square base to find the total surface area.
4\times 48+144=336
Write the answer, including the units.
The total surface area is 336 \ cm^{2}.
Common misconceptions
- The height is the perpendicular height
The height of the pyramid needs to be the perpendicular height. This is the height that is at a right-angle to the base.
- Using the correct units
Remember to use cubic units for volume such as cm^3 or m^{3}. Square units are for area, for example cm^2 or m^{2}.
- Use the correct volume formula
There are many different volume formulas in maths. Make sure that you use the correct one to work out the volume of a pyramid.
\text{Volume}=\cfrac{1}{3}\times \text{area of base} \times \text{height}Or
V=\cfrac{1}{3}Bh- Be accurate
When there are two or more steps in your workings, do not round your workings. Only round at the end of your solution so that your answer is accurate.
- Take care with rounding
At the end of the question, make sure you round your answer to the correct number of decimal places or significant figures.
Related lessons
Volume of a pyramid is part of our series of lessons to support revision on pyramids. You may find it helpful to start with the main pyramid lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:
Practice square based pyramid questions
1. How many faces does a square based pyramid have?




A square based pyramid has 5 faces. One is the square base and the other 4 are triangles which are the lateral faces.
2. Which of these nets are for a square based pyramid?








A square based pyramid has 5 faces. One is a square, the base. The other faces are triangles which need to have the same slant height so that they meet together at the apex.
3. Find the volume of this square based pyramid.
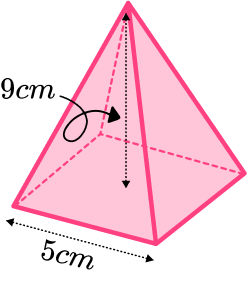




We can work out the volume of a pyramid by using the formula
V=\cfrac{1}{3}Bh.
The base area can be found by squaring the side length of the base square.
5^2=25
We can substitute the values we have been given into the formula and work out the volume.
V=\cfrac{1}{3}\times 25 \times 9=75
So the volume is 75 \ cm^{3}.
4. Find the volume of this square based pyramid.
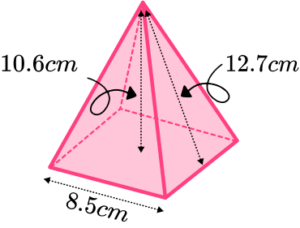




We can work out the volume of a pyramid by using the formula
V=\cfrac{1}{3}Bh.
The base area can be found by squaring the side length of the base square.
8.5^2=72.25
We can substitute the values we have been given into the formula and work out the volume. We need to make sure that we use the perpendicular height.
V=\cfrac{1}{3}\times 72.25 \times 10.6=255.28…
So the volume is 255 \ cm^{3} (to 3 sf).
5. The volume of a square based pyramid is 165 \ cm^{3}. The side length of the square is 9.2. Find the perpendicular height of the square based pyramid. Give your answer correct to 3 significant figures.




We use the formula for volume of a pyramid
V=\cfrac{1}{3}Bh.
The base area can be found by squaring the side length of the base square.
9.2^2=84.64
We can substitute the values we have been given into the formula.
165=\cfrac{1}{3}\times 84.64 \times h
We can reverse this to find the base area.
h=\cfrac{80\times 3}{9.7}=5.8482…
So the perpendicular height is 5.85 \ cm when rounded to 3 significant figures.
6. Find the total surface area of the square based pyramid. The apex is directly above the centre of the square base. Give your answer correct to 3 significant figures.
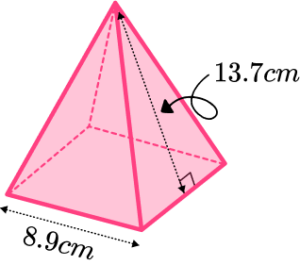




A triangular based pyramid has 5 faces. The square base and 4 triangular faces. Since the apex is directly above the centre of the square base, all 4 triangles are congruent.
Area of square base,
8.9^2=79.21.
Area of triangle face,
A=\cfrac{1}{2}bh=\cfrac{1}{2}\times 8.9\times 13.7=60.965
Total surface area of square pyramid,
79.21+4\times 60.965=323.07 .
So the total surface area is 323 \ cm^2 (to 3 sf).
Square based pyramid GCSE questions
1. Circle the correct diagram which shows the plan view of a square based pyramid.

(1 mark)
D
(1)
2. (a) How many edges does a square based pyramid have?
(b) How many vertices does a square based pyramid have?
(2 marks)
(a) 8
(1)
(b) 5
(1)
3. The diagram shows a square based pyramid.
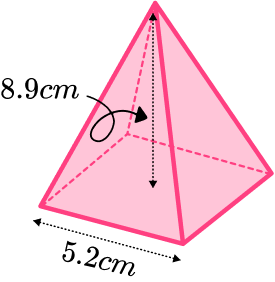
Calculate the volume of this pyramid.
Give your answer correct to 3 significant figures.
Give the correct units for your answer.
(4 marks)
Area of base is 5.2^{2}=27.04 .
(1)
Volume of pyramid is \cfrac{1}{3} \times 27.04 \times 8.9=80.2186…
(1)
80.2(1)
cm^3(1)
4. An equilateral square pyramid is a square based pyramid with equal lengths.
All the lengths are 10 \ cm.
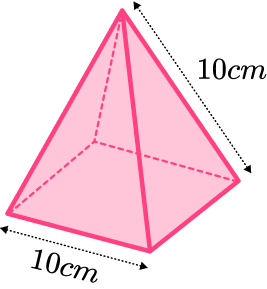
Find the total surface area of the equilateral square based pyramid.
Give your answer as an exact value.
(5 marks)
Area of base is 10^{2}=100 .
(1)
Height of triangle face 5\sqrt{3}
Or sin(60)=\cfrac{\sqrt{3}}{2}
(1)
Area of one triangle is 25\sqrt{3} .
(1)
4 \times 25\sqrt{3}(1)
100+100\sqrt{3} \ cm^2(1)
Learning checklist
You have now learned how to:
- Recognise a square based pyramid and its features
- Calculate the volume of a square based pyramid
- Solve problems involving the volume
- Find the total surface area of a square based pyramid
The next lessons are
Still stuck?
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.