GCSE Tutoring Programme
"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."
In order to access this I need to be confident with:
3D shapes Area of polygons Substitution Solving equations Rearranging formulae Changing the subject of a formula Rounding decimals Significant figuresThis topic is relevant for:

Volume Formula
Here we will learn about volume formulas, including the formula for calculating the volume of 3D shapes and how to use the volume formula to solve problems.
There are also worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
What is a volume formula?
A volume formula is a formula which is used to calculate the volume of a 3D shape. Volume is the amount of space there is inside a shape.
To calculate the volume of an object in three dimensions, we can use the various volume formulas.
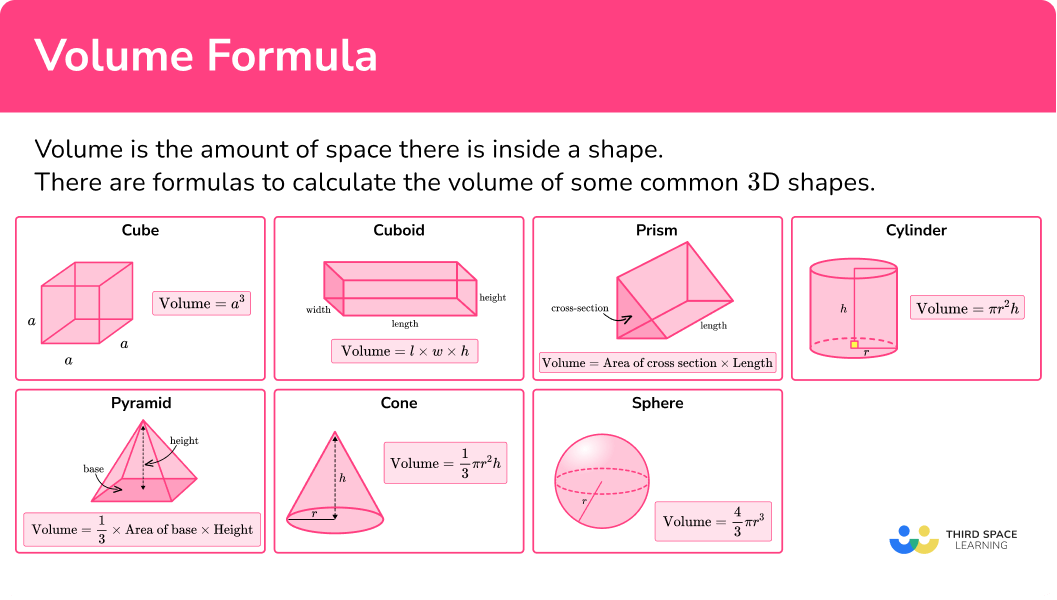
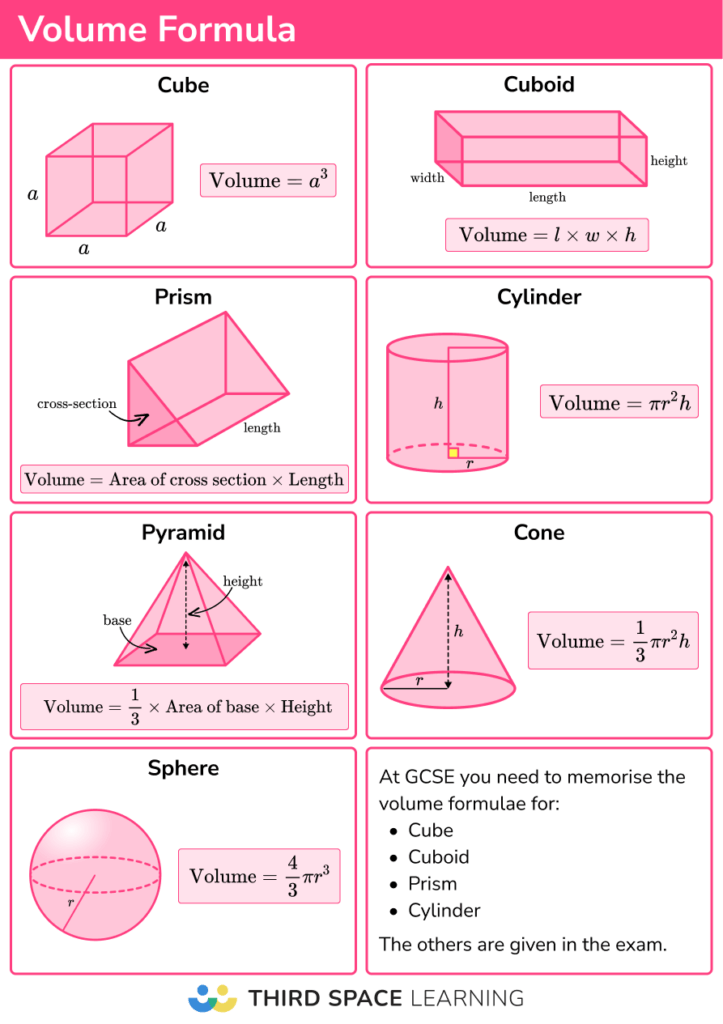
| Cube | 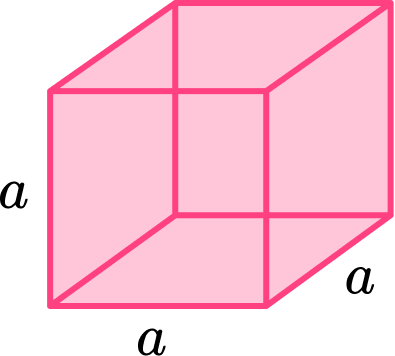 | |
| Cuboid (or rectangular prism) | 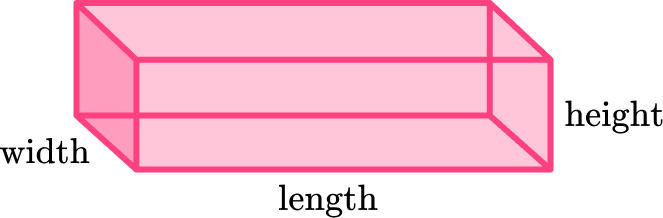 | |
| Prism | 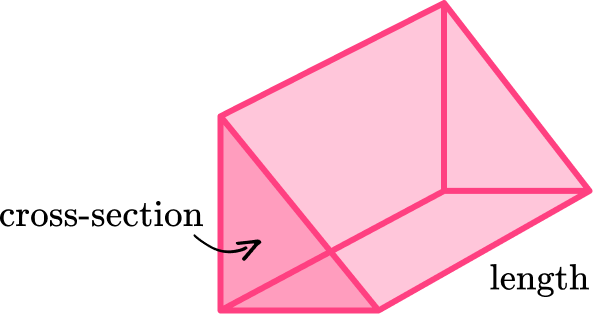 | |
| Cylinder | 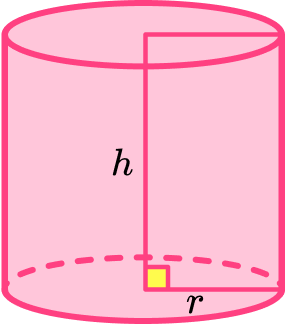 | |
| Pyramid |  | |
| Cone | 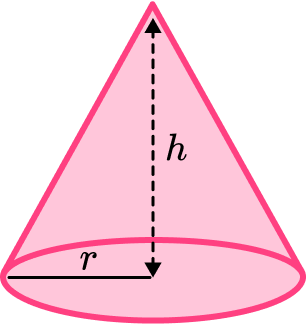 | |
| Sphere | 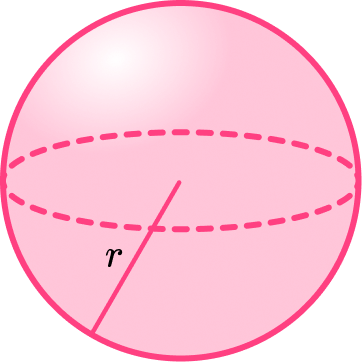 |
The formulae for the volume of a cone and the volume of a sphere are given to you for GCSE maths. You need to learn the other formulae.
Volume is measured in cubic units.
- Metric units examples
(cubic centimetres),
(cubic metres),
(cubic millimetres).
- Imperial units examples
cubic feet,
cubic inches.
Volume can also be described using units of capacity such as millilitres, litres, pints or gallons.
What is a volume formula?
Download our free volume formula poster to focus your revision!
How to use volume formula
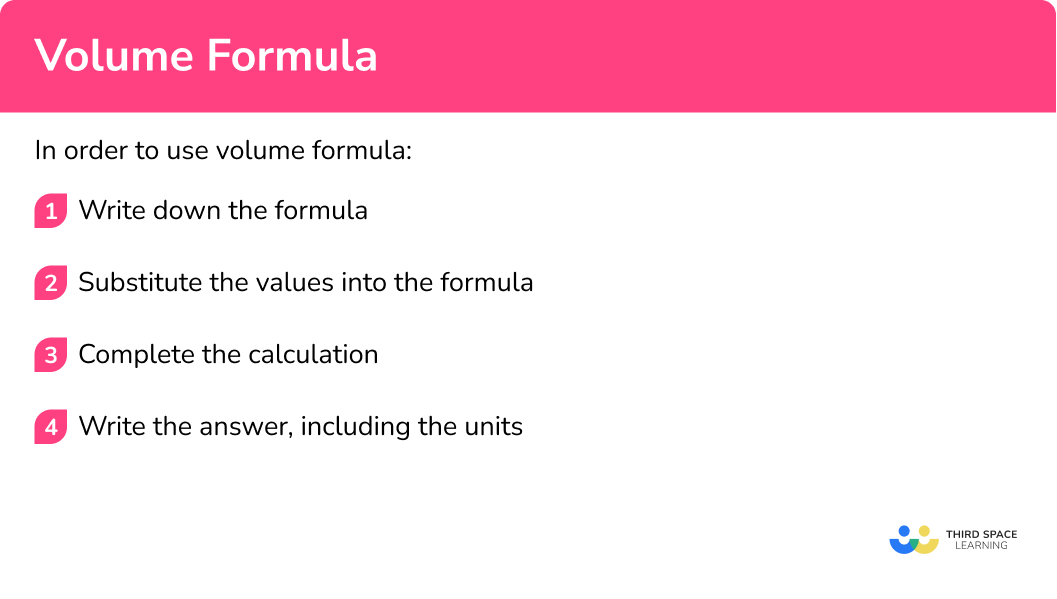
In order to use volume formula:
- Write down the formula.
- Substitute the values into the formula.
- Complete the calculation.
- Write the answer, including the units.
Explain how to use volume formula
Volume worksheet (includes volume formula)

Get your free volume formula worksheet of 20+ volume questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Volume worksheet (includes volume formula)

Get your free volume formula worksheet of 20+ volume questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREERelated lessons on how to calculate volume
Volume formula is part of our series of lessons to support revision on how to calculate volume and 3D shapes. You may find it helpful to start with the main 3D shapes lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:
Volume formula examples
Example 1: volume of a cylinder
Calculate the volume of the cylinder below.
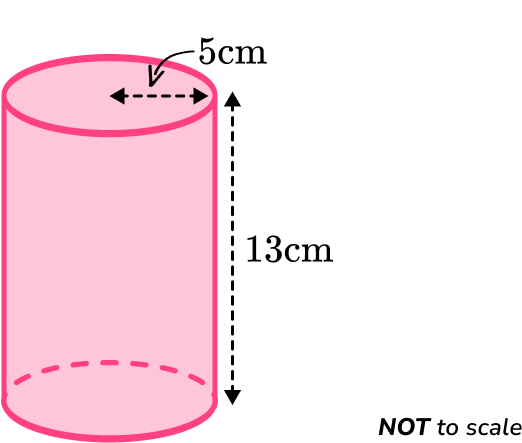
- Write down the formula.
The 3D shape is a cylinder. The formula you need to use is
2Substitute the values into the formula.
We need to calculate the area of a circle (the circular base) and then multiply it by the height of the shape.
The values you need to substitute into the formula are the radius of the base and the height of the shape
3Complete the calculation.
4Write the answer, including the units.
The dimensions of the cylinder were given in centimetres, so the volume (the amount of three-dimensional space inside the shape) will be in cubic centimetres
(to the nearest integer).
Example 2: volume of a sphere
Calculate the volume of a sphere with diameter
Write down the formula.
The 3D shape is a sphere. The formula you need to use is
Substitute the values into the formula.
You need to substitute the value of the radius of the sphere into the formula. You need to divide the diameter by to calculate the radius
Complete the calculation.
Write the answer, including the units.
The dimensions of the sphere were given in millimetres, so the volume of the sphere will be in cubic millimetres
(to significant figures).
Example 3: missing dimension
The volume of the cuboid is
Calculate the value of

Write down the formula.
The 3D shape is a cuboid. The formula you need to use is
This formula is very similar to the formula for the volume of a cube.
Substitute the values into the formula.
The values you need to substitute into the formula are and
For the height you can use
The length the width and the height of the cuboid are interchangeable, so it doesn’t matter which dimension or or
Complete the calculation.
Write the answer, including the units.
The dimensions of the cuboid were given in centimetres and the volume was given in cubic centimetres
Example 4: missing dimension
The volume of the square pyramid is cuboid is
Calculate the height of the pyramid.

Write down the formula.
The 3D shape is a pyramid. The formula you need to use is
Substitute the values into the formula.
First you need to work out the area of the square base,
The values you need to substitute into the formula are and
For the height you can use
Complete the calculation.
Write the answer, including the units.
The dimensions of the pyramid were given in centimetres and the volume was given in cubic centimetres
Example 5: volume of a compound shape
This shape is made from a cuboid and a square based pyramid. Calculate the volume of the 3D shape.
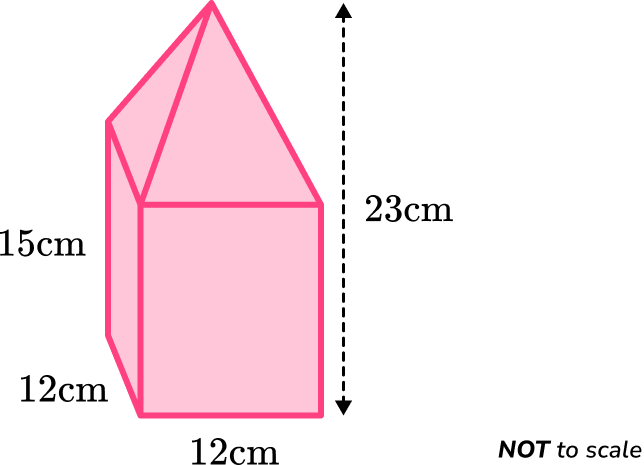
Write down the formula.
The 3D shape is made from a cuboid and a pyramid. We can find the volume of each piece and then add the two volumes together. The formulas you need to use are,
| Cuboid | Pyramid |
|---|---|
Substitute the values into the formula.
The values you need to substitute into the formulae are,
| Cuboid | Pyramid |
|---|---|
| and
| The area of the base is The height of the pyramid is |
Complete the calculation.
| Cuboid | Pyramid |
|---|---|
The volume of the shape is
Write the answer, including the units.
The dimensions of the 3D shape were given in centimetres, so the volume will be in cubic centimetres
Example 6: volume of a compound shape
This shape is made from a cylinder and a cone. Calculate the volume of the 3D shape.
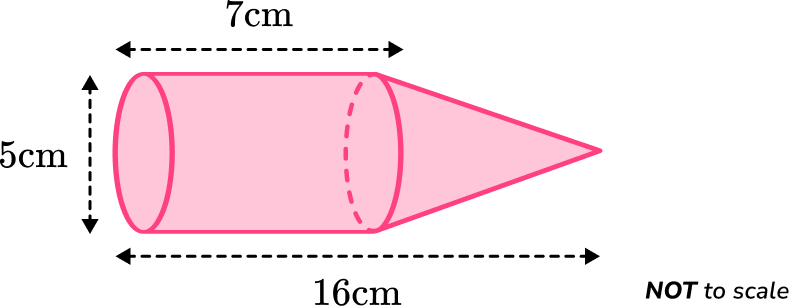
Write down the formula.
The 3D shape is made from a cuboid and a pyramid. We can find the volume of each piece and then add the two volumes together. The formulas you need to use are,
| Cylinder | Cone |
|---|---|
Substitute the values into the formula.
You need to divide the diameter by to calculate the radius. The values you need to substitute into the formulae are
| Cylinder | Cone |
|---|---|
| and | The height of the cone needs to be calculated by subtracting the length of the whole shape from the length of the side of the cylinder. |
Complete the calculation.
| Cylinder | Cone |
|---|---|
The volume of the shape is
Write the answer, including the units.
The dimensions of the 3D shape were given in centimetres, so the volume will be in cubic centimetres
(in terms of )
or
(to decimal place)
Common misconceptions
- Missing/incorrect units
You should always include units in your answer.
Volume is measured in cubic units (for example, ).
- Calculating with different units
You need to make sure all measurements are in the same units before calculating the volume. (For example, you can’t have some measurements in centimetres and some in metres).
- Make sure you get the correct formula
Be careful to make sure you use the correct formula for the volume of the three-dimensional shape. Volume formulas can be easily confused with each other and with formulas for calculating the surface area of a three-dimensional object.
- Don’t round off too early
It is important to not round decimals until the end of the calculation. Rounding too early can result in an inaccurate answer.
- Leave your answer in the correct form
It is important to leave your answer in terms of if asked to.
Practice volume formula questions
1. Calculate the volume.
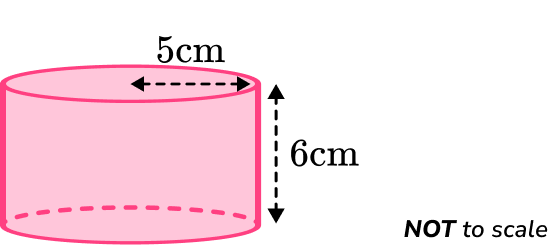




The volume formula to calculate the volume of the cylinder is
You need to substitute in the values and
The volume of the cylinder is
2. Calculate the volume of this pyramid.
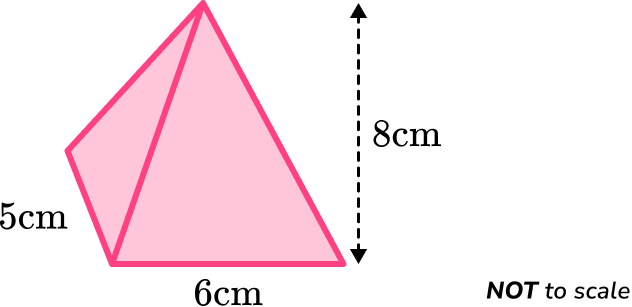




The volume formula to calculate the volume of the pyramid is
You need to find the area of the base of the pyramid.
The volume of the pyramid is
3. The volume of this Calculate the length of the missing side.
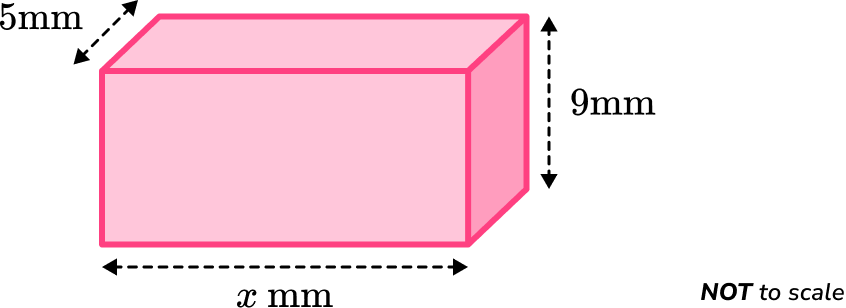




The volume formula to calculate the volume of the cuboid is
You need to substitute in the values given, using for the unknown length into the volume formula.
The missing length is
The missing length of the cuboid is
4. The volume of a cylinder is The height of the cylinder is Find the radius of the cylinder.




The volume formula to calculate the volume of the cylinder is
You need to substitute in the values given into the formula
The workings for the missing length are
The radius of the cylinder is
5. Calculate the volume of the shape below.





The volume of the cuboid is
Or you can take one side of the cube and cube it.
The volume of the pyramid is
The total volume can be found by adding the two volumes together.
6. Calculate the volume of the shape below. Give your answer to significant figures.
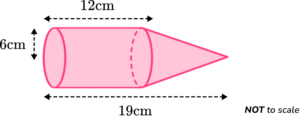




The volume of the cylinder is
The volume of the cone is
The total volume can be found by adding the two volumes together.
Volume formula GCSE questions
1. Shown below is a prism.
The cross-sectional area is
The prism has length
Find the volume of the prism.
Include the units.
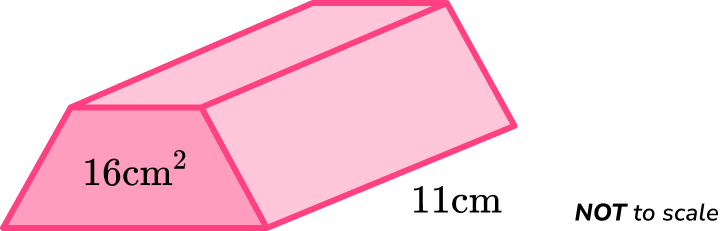
(3 marks)
(1)
(1)
(1)
2. (a) Shown below is a cuboid.
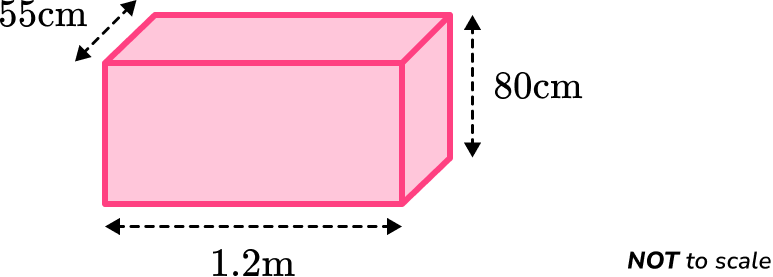
The dimensions of the cuboid are and
Find the volume of the cuboid.
Give your answer in cubic metres.
(b) The cuboid is filled with water.
You are given that
Calculate the volume of water needed to fill the cuboid.
Give your answer in litres.
(5 marks)
(a)
or
(1)
(1)
(1)
(b)
(1)
(1)
3. (a) The volume of a cylinder is
The radius of the cylinder is
Calculate the height of the cylinder.
Give your answer to significant figures.
(b) The cylinder is made from pine wood.
Pine wood has a density of
Given that .
Calculate the mass of the cylinder.
Give your answer in kilograms.
(3 marks)
(a)
(1)
(1)
(1)
(b)
(1)
(1)
(1)
4. The diagram shows a hollow pipe. It is made from a cylinder with diameter and height with a cylindrical hole of diameter and height
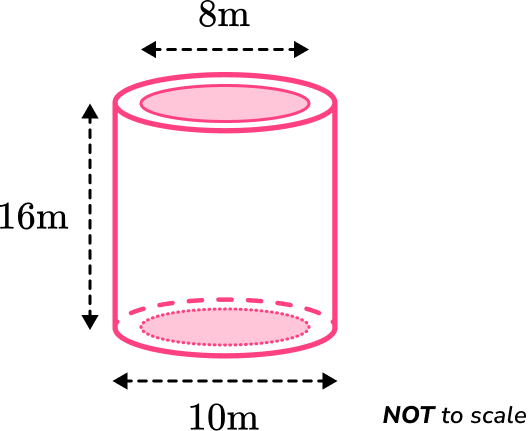
Work out the volume of the hollow pipe.
Leave your answer in terms of Include the units.
(5 marks)
or
(1)
or
(1)
(1)
(1)
(1)
5. The volume of a sphere is
Calculate the diameter of the sphere.
Give your answer correct to significant figures.
(4 marks)
(1)
(1)
(1)
(1)
Learning checklist
You have now learned how to:
- Use a volume formula to calculate the volume of a 3D shape
- Use a volume formula to find a missing dimension of a shape given its volume
- Solve problems involving volume using a volume formula
The next lessons are
Still stuck?
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.