Area of a trapezium

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Types of quadrilaterals Metric conversions Area of a rectangle Area of a parallelogram

This topic is relevant for:

GCSE Maths Geometry and Measure Area

Area Of A Trapezium

Here we will learn about finding the area of a trapezium, including compound area questions, questions with missing side lengths and questions involving unit conversion.

There are also area of a trapeziumworksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is the area of a trapezium?

The area of a trapezium is the amount of space inside the trapezium. It is measured in units squared ( $cm^{2}, m^{2}, mm^{2}$ etc.)

A trapezium (also known as a trapezoid) is a quadrilateral with exactly one pair of parallel sides. The parallel sides are indicated using small arrows.

The plural of trapezium is trapezia or trapeziums.

An isosceles trapezium is a trapezium where the base angles are equal and therefore the left and right side lengths are also equal.

The area of a trapezium is calculated by:

Adding the parallel sides
Dividing by $2$
Multiplying by the height.

This can also be summarised with the following formula:

$\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$

Where $a$ and $b$ are the parallel sides

Where $h$ is the perpendicular height of the trapezium

What is the area of a trapezium?

How to find the area of a trapezium

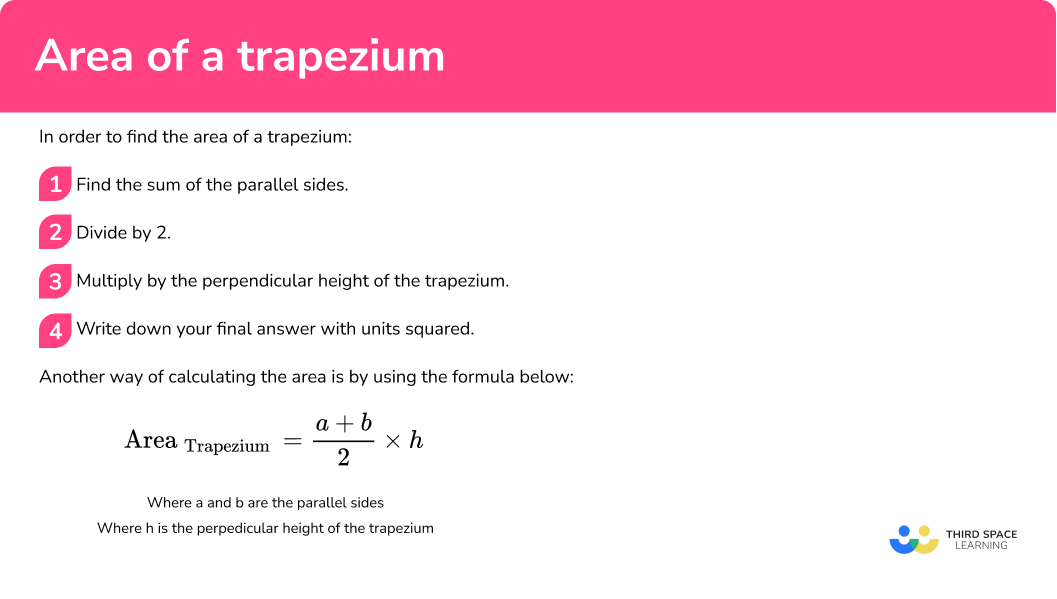
In order to find the area of a trapezium

Find the sum of the parallel sides.
Divide by $2$ .
Multiply by the perpendicular height of the trapezium.
Write down your final answer with units squared.

Another way of calculating the area is by using the formula below:

$\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$

Where $a$ and $b$ are the parallel sides

Where $h$ is equal to the perpendicular height of the trapezium

How to find the area of a trapezium

Area of a trapezium worksheet

Get your free Area of a trapezium worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Area of a trapezium worksheet

Get your free Area of a trapezium worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on area

Area of a trapezium is part of our series of lessons to support revision on area. You may find it helpful to start with the main area lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Area of a trapezium examples

Example 1: finding area given the parallel side lengths and height

Find the area of the trapezium below:

Find the sum of the parallel sides.

$4+6=10$

2Divide by 2.

$10\div2=5$

3Multiply by the perpendicular height of the trapezium.

In this case the perpendicular height is $3$ .

$5\times3=15$

4Write your final answer with units squared.

$\text { Area }=15 \mathrm{~m}^{2}$

Example 2: finding the area of a trapezium requiring converting units

Find the area of the trapezium below:

Find the sum of the parallel sides.

We have two different units for the parallel sides so we must change the measures to a common unit.

In this case it is easier to convert to metres since the height is also in metres.

There are $100cm$ in a metre, so if we divide the top side of the trapezium by $100$ we get $9.5m$

So now if we add the sides:

$9.5+14=23.5$

Divide by 2.

$23.5\div2=11.75$

Multiply by the perpendicular height of the trapezium.

In this case the perpendicular height is $8$ .

$11.75\times8=94$

Write your final answer with units squared.

$\text { Area }=94 m^{2}$

Example 3: worded question

Sarah is looking to design the flower bed below at the front of her house. She would like to cover the entire flower bed with rose bushes. Each bush needs $1.5m^{2}$ to allow the roots to spread out evenly. What is the maximum number of rose bushes she can plant on her flower bed?

Find the sum of the parallel sides.

$4.5+1.5=6$

Divide by 2.

$6\div2=3$

Multiply by the perpendicular height of the trapezium.

In this case the perpendicular height is $3$ .

$3\times3=9$

Write your final answer with units squared.

$\text { Area }=9 m^{2}$

Now to answer the actual question, since each rose bush needs $1.5$ square metres to grow, we need to divide $1.5$ into the total area which is $9$ .

$9\div1.5 = 6$

Sarah can plant a maximum of $6$ rose bushes in her garden.

Example 4: area of compound shapes

Find the area of the shaded region below:

Find the sum of the parallel sides.

Before we can do this step we need to split the compound shape into individual shapes we can calculate the area of. In this case we can split the hexagonal shape at the top into two trapeziums and have a rectangle at the bottom as shown:

Now if we take one trapezium, we know one length is $8m$ , the length of the other parallel side is $6m$ because that is the length of the rectangle and which is connected to the side.

$8+6=14$

*We calculated the height as $4.5m$ because the height of both trapeziums combined is $9m$ so to get the height of one trapezium we divide $9$ by $2$ to get $4.5$ .

Divide by 2.

$14\div2=7$

Multiply by the perpendicular height of the trapezium.

In this case the perpendicular height is $4.5$ .

$7\times4.5=31.5$

Write your final answer with units squared.

$\text { Area }_{\text {Trapezium }}=31.5 \mathrm{~m}^{2}$

We have to now double this area as there are $2$ trapeziums and then add the area of rectangle.

\[\begin{aligned} \text { Area }_{\text {Rectangle }} &=l \times w \\ \text { Area }_{\text {Rectangle }} &=6 \times 3 \\ \text { Area }_{\text {Rectangle }} &=18 \mathrm{~m}^{2} \end{aligned}\]

\[\begin{aligned} \text { Area }_{\text {Compound Shape }} &=31.5\times 2+18 \\ &=81 \mathrm{~m}^{2} \end{aligned}\]

Example 5: calculating height given the area

The area of the trapezium below is $128m^{2}$ . Calculate the value of $h$ in the trapezium below:

Rearrange the area of a trapezium formula

To find the height of the trapezium let us start with the formula for the area of a trapezium and rearrange it to solve for height (h).

$\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$

First we need to move the $2$ to the other side of the equal sign. Division changes to multiplication.

$2 A=(a+b) \times h$

Next we need to move the $a+b$ to the other side of the equal sign. Multiplication changes to division.

$\frac{2 A}{(a+b)}=h$

The new formula for the height of the trapezium is:

$h=\frac{2 A}{(a+b)}$

Substitute in the known values

We can then substitute our known values:

\[\begin{aligned} h &=\frac{2(128)}{(12+4)} \\ h &=16 \mathrm{~m} \end{aligned}\]

Example 6: calculating base length given the area

The area of a trapezium is $322$ square millimetres and its height is $14mm$ . If the length of one of the parallel sides is $27mm$ , what is the length of the other parallel side?

Let’s start out by sketching out a trapezium using what is given in the question:

Rearrange the area of a trapezium formula

To find the length of the other parallel side $‘a’$ let us start with the formula for the area of a trapezium and rearrange it to solve for $a$ .

$\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$

First we need to multiply both sides of the formula by $2$ .

$2 A=(a+b) \times h$

Next we need to divide both sides of the formula by $‘h’$ .

$\frac{2 A}{h}=a+b$

Then we can then subtract $‘b’$ from both sides of the formula.

$\frac{2 A}{h}-b=a$

The new formula for $a$ is:

$a=\frac{2 A}{h}-b$

Substitute in the known values

We can then substitute our known values:

\[\begin{aligned} &a=\frac{2(322)}{14}-27 \\ &a=19 \mathrm{~mm} \end{aligned}\]

Common misconceptions

Using incorrect units for the answer

A common error is to forget to include squared units when asked to calculate area.

Forgetting to convert measures to a common unit

Before calculating the area of a trapezium, pupils must look at the units given in the question. If different units are given e.g. length = $4m$ and width = $3cm$ pupils must convert them either both to $cm$ or both to $m$ .

Using length of the non-parallel sides when calculating area and not the height

Sometimes in a question we are given additional lengths which are not needed in our calculations. Sometimes we are given the length of the non-parallel sides of the trapezium. We must be careful to not mistake these measurements for height.

Practice area of a trapezium questions

20.5m^{2}

36m^{2}

67.5m^{2}

47.5m^{2}

Add the parallel sides $9$ and $7$ together. Divide the sum by $2$ . Times by the perpendicular height of $4.5$ .

5085cm^{2}

5085m^{2}

2055.6cm^{2}

2055.6m^{2}

Convert $0.68m$ into $cm$ . Add the $45cm$ and $68cm$ together. Divide by $2$ . Multiply by $90$ .

42.5

10

11

165

Add the parallel sides $11$ and $6$ together. Divide the sum by $2$ . Times by the perpendicular height of $5$ . Divide by $4$ .

224m^{2}

84.5m^{2}

44m^{2}

55m^{2}

Find the area of the rectangle by multiplying its length times width. $(5$ x $11)$ . Split the hexagon in half and find the area of each trapezium. To do this add the parallel sides ( $11$ and $15$ ), divide by $2$ , and multiply by the perpendicular height which in this case is $6.5$ . As there are two congruent trapeziums, multiply the area of the trapezium by $2$ and add on the area of the rectangle to get the compound area.

8.5m^{2}

60m^{2}

16m

8.5m

Start with the area of a trapezium formula $\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$ and rearrange to get the formula $h=\frac{2 A}{(a+b)}$ . Substitute the values of the area and the parallel sides and solve for the height.

55mm

13mm

45mm

14mm

Start with the area of a trapezium formula $\text { Area }_{\text {Trapezium }}=\frac{a+b}{2} \times h$ and rearrange to get the formula $a=\frac{2 A}{h}-b$ . Make sure to isolate a by moving the other variables to the other side of the equal sign and changing to the opposite sign. Substitute the known values and solve for the length of the parallel side.

Area of a trapezium GCSE questions

1. Find the area of the trapezium below.

(3 marks)

Show answer

Finding sum of parallel sides $(12+18=30)$

(1)

Dividing by $2$ or multiplying by height

(1)

$75m^{2}$ (cao with units)

(1)

2. (a) Calculate the value of $x$ in the trapezium below.

(b) The above trapezium is enlarged by a scale factor of $2$ . What is the area of the new trapezium?

(7 marks)

Show answer

(a)
Evidence of adding the parallel sides $11+17 = 28$

(1)

Dividing by $2$

(1)

$70\div14$ seen

(1)

$5m$ seen (cao including units)

(1)

(b)
$2^{2}$ oe seen

(1)

$70\times4$ seen

(1)

280m^{2}

(1)

3. A painter is looking to paint his deck. Below is a blueprint of the deck. A can of paint costs $£5.50$ and covers an area of $1.5cm^{2}$ . How much would it cost to paint the entire deck?

(5 marks)

Show answer

Adding parallel sides of trapezium and dividing by $2$ or multiplying by height

(1)

$50cm^{2}$ seen as area of trapezium

(1)

$\frac{1}{2}bh$ seen or correct values substituted or $24cm^{2}$ seen with some calculations

(1)

Evidence of adding areas or dividing total are by $1.5$ or multiplying by $£5.50$

(1)

£271.33

(1)

Learning checklist

You have now learned how to:

Calculate and compare the area of trapeziums including using standard units

Calculate the area of trapeziums and related composite shapes

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Introduction

What is the area of a trapezium?

How to find the area of a trapezium

Area of a trapezium worksheet

Area of a trapezium examples

↓

Example 1: finding area given the parallel side lengths and height Example 2: finding the area of a trapezium requiring converting units Example 3: worded question Example 4: area of compound shapes Example 5: calculating height given the area Example 6: calculating base length given the area

Common misconceptions

Practice area of a trapezium questions

Area of a trapezium GCSE questions

Learning checklist

Next lessons

Still stuck?