GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Identifying parts of a circle

Irrational numbers

Rounding decimals Rounding to significant figures Squares and square roots

This topic is relevant for:

GCSE Maths Geometry and Measure Area

Pi R Squared

𝝅r² (Pi R Squared)

Here we will learn about using the formula $\pi r^2$ (pi r squared) to calculate the area of a circle given the radius, diameter or the circumference.

There are also worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is pi r squared?

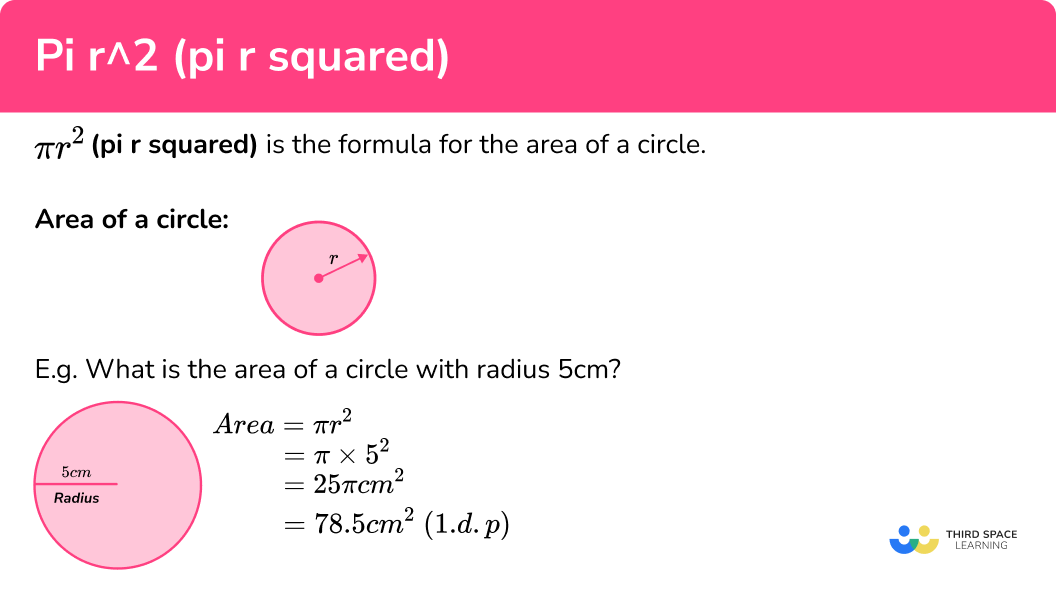
Pi r squared is the formula for the area of a circle.

This is because there is a specific relationship between the radius ( $r$ ) of a circle and its area.

Area of a circle formula:

\pi \times r\times r

we usually simplify this to

Area= \pi r^2

E.g.

What is the area of a circle with radius $5cm$ ? Give your answer to one decimal place.

Squaring the radius of $5$ gives $25.$ Then multiply pi by this value to work out the area of the circle.

\begin{aligned} \text { Area } &=\pi r^{2} \\\\ &=\pi \times 5^{2} \\\\ &=25 \pi \mathrm{cm}^{2} \\\\ &=78.5 \mathrm{~cm}^{2}(1 . \mathrm{d} . \mathrm{p}) \end{aligned}

What is pi r squared?

What is pi?

$\pi$ (pronounced pi) is a greek letter that represents the ratio of the circumference of a circle to its diameter.

For all circles if you divide the length of the circumference by the length of the diameter you get the value $\pi$ .

Note: $\pi$ is an irrational number which means it cannot be written as a fraction. It is a non recurring decimal and has an approximate value of $3.1415\dots$

In GCSE you should use the $\pi$ button on your Casio calculator when working with .

To get this you need to press [SHIFT][ $\times 10^x$ ], see below.

Sometimes the question may ask you to give the answer ‘in terms of $\pi$ ’.

E.g.

$3 \times \pi = 3 \pi$ (this is an answer in terms of pi)

$5 \times \pi = 5 \pi$ (this is an answer in terms of pi)

$17 \times \pi = 53.407...$ (this is an answer not in terms of pi)

How to use pi r squared

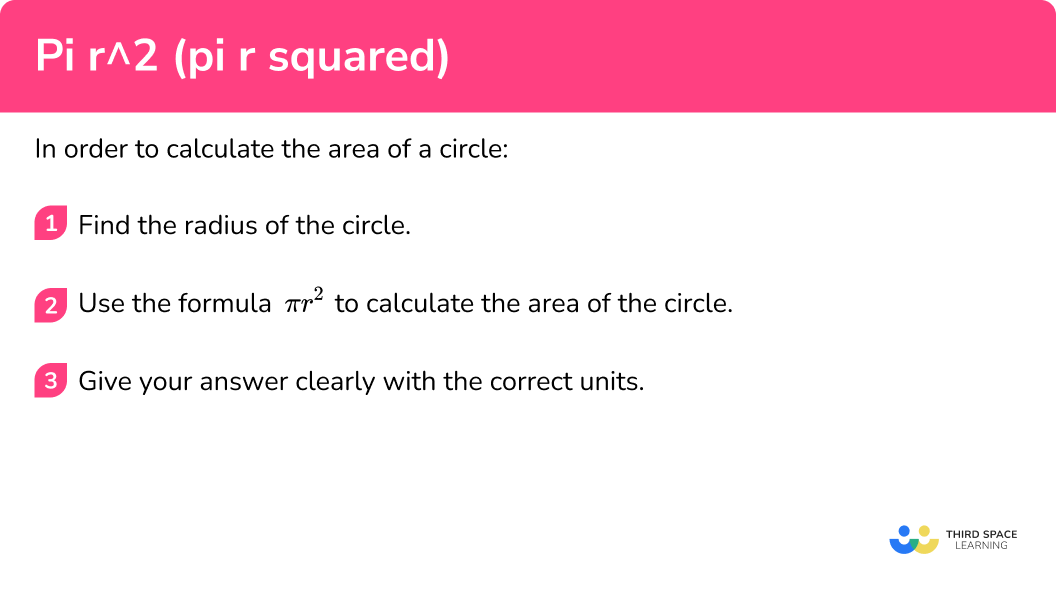
In order to calculate the area of a circle:

Find the radius of the circle.
Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.
Give your answer clearly with the correct units.

How to use pi r squared

Area of a circle worksheet using pi r squared

Get your free area of a circle using worksheet of 20+ questions and answers. Includes reasoning and applied questions using pi r squared.

DOWNLOAD FREE

Area of a circle worksheet using pi r squared

Get your free area of a circle using worksheet of 20+ questions and answers. Includes reasoning and applied questions using pi r squared.

DOWNLOAD FREE

Pi r squared examples

Example 1: calculating the area of the circle given the radius

Find the radius of the circle.

The radius is given in the question

Radius $= 7cm$

2Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times7\times 7 \\\\ &49\pi \\\\ &=153.93904... \end{aligned}

Remember the question asks you to round your answer to ‘ $2$ decimal places’

153.94

3Give your answer clearly with the correct units.

153.94cm^2

Example 2: calculating the area of the circle given the diameter

Find the radius of the circle.

The question gives you the diameter which is twice the radius.

Diameter $= 20mm$

Radius $= 20mm \div 2$

Radius $= 10mm$

Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times10\times 10 \\\\ &100\pi \\\\ &=314.159... \end{aligned}

Remember the question asks you to round your answer to ‘ $1$ decimal place’

$314.2$

Give your answer clearly with the correct units.

314.2mm^2

Example 3: calculating the area of the circle, given the radius, answer in terms of 𝝅?

Find the radius of the circle.

The radius is given in the question

Radius $= 18m$

Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times18\times 18 \\\\ &324\pi \end{aligned}

Remember the question asks you to give your answer to ‘in terms of $\pi$ ’. Therefore you leave the answer in the form $324 \pi$

Give your answer clearly with the correct units.

324\pi \; m^2

Example 4: calculating the area of the circle given the diameter

Find the radius of the circle.

The question gives you the diameter which is twice the radius.

Diameter $= 210km$

Radius $= 210km \div 2$

Radius $= 105km$

Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times105\times 105 \\\\ &11025\pi \end{aligned}

Remember the question asks you to give your answer to ‘in terms of $\pi$ ’. Therefore you leave the answer in the form $11025 \pi$

Give your answer clearly with the correct units.

11025\pi \; km^2

Example 5: calculating the area of the circle given the circumference of a circle

A circle has a circumference of $12cm.$

Calculate its area.

Give your answer to $2$ decimal places.

Find the radius of the circle.

The question gives you the circumference of the circle which is equal to $2\pi r$

This means you can find the radius of the circle from the circumference, see below:

Circumference $= 2\pi r$

Circumference $= 12$

$\begin{aligned} &12 = 2\pi r \quad \quad \quad \text{ Divide both sides by } 2\pi \\\\ &\frac{12}{2\pi}= r \\\\ &1.9099=r \end{aligned}$

Notice how $r$ has been rounded to more decimal places than the question requires so you do not cause a rounding error.

Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times1.9099\times 1.9099 \\\\ &11.4596 \end{aligned}

Remember the question asks you to give your answer to ‘ $2$ decimal place’

$11.46$

Give your answer clearly with the correct units.

11.46cm^2

Example 6: calculating the area of a semicircle given the diameter

Find the radius of the circle.

The question gives you the diameter which is twice the radius.

Diameter $= 200m$

Radius $= 200m \div 2$

Radius $= 100m$

Use the formula $\bf{\pi r^2}$ to calculate the area of the circle.

\begin{aligned} &\pi r^2 \\\\ &\pi \times r\times r \\\\ &\pi \times 100\times 100 \\\\ &10000\pi \end{aligned}

Remember the question asks you to give your answer to ‘in terms of $\pi$ ’. Therefore you leave the answer in the form $10000 \pi$

$10000\pi$

This is the area of a whole circle with a diameter of $200m.$ You only want the area of a semicircle.

A semicircle has half the area of a full circle so you need to divide your answer by two. Remember to keep it in terms of $\pi$ .

$\begin{aligned} &10000 \pi \div 2 \\\\ &5000\pi \end{aligned}$

Remember the question asks you to give your answer to ‘in terms of $\pi$ ’. Therefore you leave the answer in the form $5000 \pi$

Give your answer clearly with the correct units.

5000 \pi \; m^2

Common misconceptions

Not using the radius

You must have the radius to find the area of a circle from the formula.

A question may not give you the radius directly and so we need to use the information given to find the radius first.

Not including the correct units

When working with area you must always give the correct units squared

E.g.

$cm^2, \; m^2, \; km^2$ etc.

Not rounding correctly

These questions often involve rounding. You must only round at the end of the question and to the specified number of decimal places.

Not giving answer in terms of $\pi$

Sometimes the question may ask you to give the answer ‘in terms of $\pi$ ’. This means you do not give the numerical answer that is produced when you multiply it by $\pi$ .

E.g.

$6 \times \pi = 6\pi$ (this is an answer in terms of pi)

$6 \times \pi = 18.8495592…$ (this answer is not in terms of pi)

Misuse of calculator

Ensure you know how to correctly use the $\pi$ button on your calculator .

Degrees and radians

We can also measure angles in radians, however at GCSE we will always measure angles in degrees.

Practice pi r squared questions

6cm

3cm

12cm

6\pi cm

The diameter of the circle is twice the size of the radius. Therefore to find the radius you can divide the diameter by $2.$

6cm \div 2 = 3cm

\pi d

2\pi r

\pi r^2

\pi r

\pi \times r\times r

we usually simplify this to

\text{Area } =\pi r^2

4\pi cm^2

4\pi cm

12.6cm

12.6 cm^2

\text{Area of a circle } =\pi r^2

$\pi \times 2 \times 2$ is equal to $12.566...$

This answer is correctly rounded to $1$ decimal place and has the correct units

9\pi cm^2

324\pi cm^2

18\pi cm^2

81\pi cm^2

\text{Area of a circle } =\pi r^2

\pi \times 9 \times 9

81 \pi

This answer is correctly given in terms of $\pi$

16\pi cm^2

64\pi cm^2

8\pi cm^2

2\pi cm^2

$8cm =$ diameter

$4cm =$ radius

\text{Area of a circle } = \pi r^2

\pi \times 4 \times 4

16 \pi

This answer is correctly given in terms of $\pi$

314 cm^2

314.1 cm^2

314.159 cm^2

100\pi cm^2

\text{Area of a circle } = \pi r^2

$\pi \times 10 \times 10$ is equal to $314.1592654...$

This answer is correctly rounded to $1$ decimal place and has the correct units

Pi r squared GCSE questions

1. The radius of a circle is $4.5 cm$

Work out the area of the circle

Give your answer correct to $3$ significant figures

(3 marks)

Show answer

$\pi \times 4.5 \times 4.5$ or $63.617\dots$

(1)

63.6

(1)

cm^2

(1)

2. The radius of a circle is $19.1 m$

Work out the area of the circle

Give your answer correct to $2$ decimal places

(3 marks)

Show answer

$\pi \times19.1 \times 19.1$ or $1146.0844\dots$

(1)

1146.08

(1)

m^2

(1)

3. The diameter of a circle is $18cm$

Work out the area of the circle

Give your answer in terms of $\pi$

(3 marks)

Show answer

\pi \times9\times 9

(1)

81\pi

(1)

cm^2

(1)

4. The area of a circle is $49 \pi \; cm^2$

Calculate the radius of the circle

(3 marks)

Show answer

49\pi = \pi r^2

(1)

49= r^2

(1)

r=7

(1)

Learning checklist

You have now learned how to:

Identify and apply circle definitions and properties, including: centre, radius, chord, diameter, circumference

Know and apply the formulae

\pi r^2

Give answers in terms of

\pi

Calculate area of

2

D shapes including circles and semicircles

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.