Perimeter of a sector

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Parts of a circle Arc length Solving equations Cosine rule Rounding

This topic is relevant for:

GCSE Maths Geometry and Measure 2D Shapes Circles, Sectors & Arcs Sector Of A Circle

Perimeter Of A Sector

Here we will learn about calculating the perimeter of a sector including how to find the perimeter of a sector with a given angle and radius, and how to find the radius of a circle when given the perimeter of the sector.

There are also perimeter of a sector worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is the perimeter of a sector?

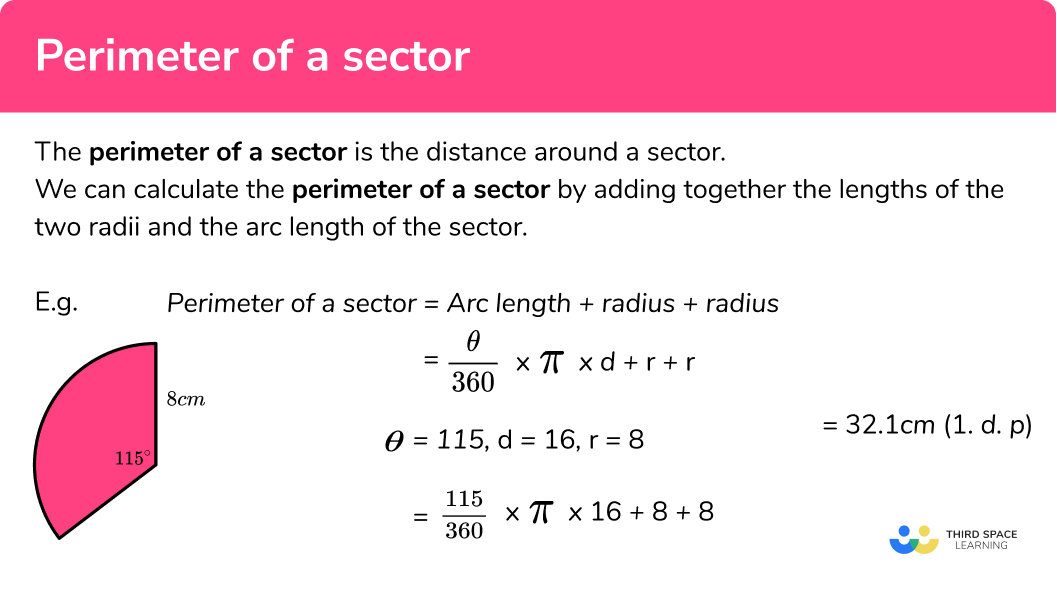
The perimeter of a sector is the distance around a sector.

We can calculate the perimeter of a sector by adding together the lengths of the two radii and the arc length of the sector.

E.g.

Calculate the perimeter of the below sector to $1$ decimal place:

Arc length formula:

$\text{Arc length} = \frac{\theta}{360} \times \pi\times d$

$\theta$ = Angle of the sector

$r$ = Radius of the circle

$\text{Arc length} = \frac{\theta}{360} \times 2\times\pi \times r$

$\theta$ = Angle of the sector

$r$ = Radius of the circle

Here angle $\theta = 115^o$ and $r = 8$ , so

$\begin{aligned} \text { Arc length }&=\frac{115}{360} \times 2 \times \pi \times 8 \\\\ &=16.05702912 \ldots \end{aligned}$

To calculate the perimeter of the sector we need to add the arc length to the lengths of the two radii:

Perimeter of a sector formula:

$\begin{aligned} \text { Perimeter of a sector }&= \text{Arc length + radius + radius}\\\\ &=16.05702912 + 8 + 8\\\\ &=32.05702912\ldots\\\\ &=32.1 \;cm \; (1.d.p) \end{aligned}$

What is the perimeter of a sector?

How to find the perimeter of a sector

In order to find the perimeter of a sector:

Find the length of the diameter/radius.
Find the size of the angle creating the arc of the sector.
Find the arc length of the sector.
Add together the arc length and the two radii.
Clearly state your answer.

Explain how to find the perimeter of a sector

Arcs and sectors worksheet (including perimeter of a sector)

Get your free worksheet of 20+ questions and answers. Includes reasoning and applied questions on the perimeter of a sector.

DOWNLOAD FREE

Arcs and sectors worksheet (including perimeter of a sector)

Get your free worksheet of 20+ questions and answers. Includes reasoning and applied questions on the perimeter of a sector.

DOWNLOAD FREE

Perimeter of a sector is part of our series of lessons to support revision on sector of a circle and circles, sectors and arcs. You may find it helpful to start with the main circles, sectors and arcs lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Perimeter of a sector examples

Example 1: calculate the perimeter of a sector (quadrant)

Calculate the perimeter of a sector of the sector.

Give your answer to $3$ decimal places.

Find the length of the diameter/radius.

Radius $= 6cm$

2Find the size of the angle creating the arc of the sector.

Angle $= 90^o$ . Shown by the symbol of the right angle.

3Find the arc length of the sector.

$\text{Arc length } = \frac{\theta}{360} \times 2\times\pi \times r$

$\begin{aligned} &=\frac{90}{360} \times 2\times\pi \times 6 \\\\ &=3\pi \end{aligned}$

4Add together the arc length and the two radii.

Arc length: $3\pi \; cm$

Radius: $6 \; cm$

$\text{Total perimeter of sector } = 3\pi + 6 +6 \; cm$

$\text{Total perimeter of sector } = 3\pi + 12 \; cm$

5 Clearly state your answer.

The question asked you to round your answer to $3$ decimal places

$\text{Perimeter of sector} = 3\pi + 12 \; cm$

$\text{Arc length} = 21.424777..cm$

$\text{Arc length} = 21.425 \; cm$

Remember the perimeter of a sector is a measure of distance and therefore the units are not squared.

Example 2: calculate the perimeter of a sector (semi circle)

Calculate the perimeter of a sector of the semicircle shown below.

Give your answer in terms of $\pi.$

Find the length of the diameter/radius.

Diameter $= 24 \; cm$

Find the size of the angle creating the arc of the sector.

Angle $= 180^o$ . This is because the shape shown is a semi circle. Therefore, the angle of the straight line is $180$ degrees.

Find the arc length of the sector.

$\text{Arc length } = \frac{\theta}{360} \times \pi\times d$

$\begin{aligned} &=\frac{180}{360} \times \pi\times 24 \\\\ &=12\pi \end{aligned}$

Add together the arc length and the two radii.

Arc Length: $12\pi \; cm$

Diameter: $24 \; cm$

Radius: $12 \; cm$

$\text{Total perimeter of sector } = 12\pi + 12 +12 \; cm$

$\text{Total perimeter of sector } = 12\pi + 24 \; cm$

Clearly state your answer.

The question asks you to give your answer in terms of pi

$\text{Perimeter of sector } = 12\pi + 24 \; cm$

This answer is in terms of pi

Example 3: calculate the perimeter of a sector (with an angle given)

Calculate the perimeter of a sector of the sector shown below.

Give your answer to $3$ significant figures.

Find the length of the diameter/radius.

Radius $= 5.5cm$

Find the size of the angle creating the arc of the sector.

Angle $= 117^o$

Find the arc length of the sector.

$\text{Arc length } = \frac{\theta}{360} \times 2\times\pi \times r$

$\begin{aligned} &=\frac{117}{360} \times 2\times\pi \times 5.5 \\\\ &=\frac{143}{40} \pi \end{aligned}$

Add together the arc length and the two radii.

Arc Length: $\frac{143}{40} \pi \; cm$

Radius $5.5 \; cm$

$\text{Total perimeter of sector } = \frac{143}{40} \pi + 5.5 +5.5 \; cm$

$\text{Total perimeter of sector } = \frac{143}{40} \pi + 11 \; cm$

Clearly state your answer.

The question asked you to round your answer to $3$ significant figures

$\text{Perimeter of sector } = \frac{143}{40} \pi + 11 \; cm$

$\text{Perimeter of sector } = 22.2311...cm$

$\text{Perimeter of sector } = 22.2 \; cm$

Example 4: calculate the perimeter of a sector (the angle is not given)

Calculate the perimeter of a sector of the sector AOB below;

The length of the radius (OB) is $9cm.$

The length of a chord (AB) is $10cm.$

Give your answer to $1$ decimal place.

Find the length of the diameter/radius.

Radius $= 19 \; cm$

Find the size of the angle creating the arc of the sector.

In this example you are not given the angle of the sector, you need to calculate it first.

Here you can use the triangle created by the two radii and the chord to find the angle (see below):

Perimeter of a sector total Example 4 Step 2

We will need to use the cosine rule to find the angle.

$a^2=b^2+c^2-2bcCos(A)$

$A$ is the angle you are trying to find. You can therefore use the rearranged cosine rule to find the angle.

$\begin{aligned} \operatorname{Cos} A&=\frac{b^{2}+c^{2}-a^{2}}{2 b c} \\ \operatorname{Cos} A&=\frac{19^{2}+19^{2}-20^{2}}{2 \times 19 \times 19} \\ \operatorname{Cos} A&=\frac{161}{361} \\ A&=\operatorname{Cos}^{-1}\left(\frac{161}{361}\right) \\ A&=63.51^{\circ} \end{aligned}$

The size of the angle creating the sector (made by the two radii) is $63.5^o$ .

Find the arc length of the sector.

As you know the radius you can use the formula which has $‘r’$ as a variable.

$\text{Arc length } = \frac{\theta}{360} \times 2\times\pi \times r$

$\begin{aligned} &=\frac{63.5}{360} \times 2\times\pi \times 9 \\\\ &=21.057... \end{aligned}$

Add together the arc length and the two radii.

Arc Length: $21.057 \; cm$ Notice how you round this to more than the final answers required. This is to avoid rounding errors.

Radius $19 \; cm$

$\text{Total perimeter of sector } = 21.057 + 19 + 19 \; cm$

$\text{Total perimeter of sector } = 59.057 \; cm$

Clearly state your answer.

The question asked you to round your answer to $1$ decimal place.

$\text{Arc length } = 59.057 \; cm$

$\text{Arc length } = 59.1 \; cm$

How to solve problems involving the perimeter of a sector

Sometimes you may be given the perimeter of a sector and asked to find a property of the circle e.g the radius.

In this case you need to ‘reverse’ the process.

Clearly state which of the properties you know and do not know.
Create an equation for the perimeter of the sector and substitute in the value you know.
Solve the equation to find the unknown property.
Clearly state your answer.

Solving problems involving perimeter of a sector examples

Example 5: finding the radius given the perimeter of a sector

The sector below has a perimeter of $20cm$ and an angle of $125^o.$

Calculate the length of $x.$

Give you answer to $2$ decimal places.

Clearly state which of the properties you know and do not know.

Radius $= \; x$

Angle of sector $= \; 125^o$

Perimeter of a sector $= \; 20cm$

Arc length $= \; \frac{125}{360} \times 2\times\pi \times x$

Arc length $= \; \frac{25x}{36} \times\pi$

Create an equation for the perimeter of the sector and substitute in the value you know.

Perimeter of sector = Arc length + radius + radius

$\begin{aligned} 20&=\frac{25}{36} \pi x+x+x \hspace{2.6cm} \text{Substitute in values } \\\\ 20&=\frac{25}{36} \pi x+2 x \hspace{3cm} \text{Simplify } x+x \end{aligned}$

Solve the equation to find the unknown property.

$\begin{aligned} 20&=\frac{25}{36} \pi x+2 x \hspace{3cm} \text{Simplify } x+x \\\\ 20&=x\left(\frac{25}{36} \pi+2\right) \hspace{2.5cm} \text{Take } x \text{ out as a factor} \\\\ 20&=x(4.18166) \\\\ 20 \div 4.18166&=x \hspace{4.3cm} \text{Make } x \text{ the subject}\\\\ 4.783 \ldots&=x \end{aligned}$

Clearly state your answer.

The question asks you to give your answer to $2$ decimal places

$x=4.783...$

$x=4.78$

Example 6: finding the angle of a sector given the perimeter of a sector

The sector below has an perimeter $62cm$ and a radius of $18.$

Calculate the length of $x.$

Give you answer to $2$ decimal places.

Clearly state which of the properties you know and do not know.

Radius $= \; 18$

Angle of sector $= \; x^o$

Perimeter of a sector $= \; 62cm$

$\begin{aligned} \text { Arc length }&=\frac{x}{360} \times 2 \times \pi \times 18 \\\\ \text { Arc length }&= \frac{1}{10} \pi x \end{aligned}$

Create an equation for the perimeter of the sector and substitute in the value you know.

Perimeter of sector = Arc length + radius + radius

$\begin{aligned} 62&=\frac{1}{10} \pi x+18+18 \hspace{2.3cm} \text{Substitute in values } \\\\ 62&=\frac{1}{10} \pi x+36 \hspace{3cm} \text{Simplify} \\\\ 26&=\frac{1}{10} \pi x \end{aligned}$

Solve the equation to find the unknown property.

$\begin{aligned} 26&=\frac{1}{10} \pi x \\\\ 26 \div \frac{\pi}{10}&=x \hspace{4.8cm} \text{Make } x \text{ the subject}\\\\ 82.76057&=x \end{aligned}$

Clearly state your answer.

The question asks you to give your answer to $2$ decimal places

$x=82.76057...$

$x=82.76$

Common misconceptions

Finding the length of the arc not the perimeter of the sector

You must remember that the perimeter of the sector is the combined length of the arc and the two radii.

Units

Remember the perimeter is a length and therefore the units will not be squared or cubed. Square units are for area and cubed units are for volume.

Practice perimeter of a sector questions

Circumference

Sector

Arc

Minor arc

A sector with an angle of $360º$ is a full circle.

Therefore, the perimeter of the sector is the whole length of the outside of the circle which is called the circumference.

$2$ radii

The arc only

The arc and one radius

The arc and two radii

The perimeter of a shape is the distance around a two-dimensional shape.

Therefore, the perimeter of a sector is the combined length of the arc and two radii.

$5\pi+20 \; cm$

$5\pi \; cm$

$25\pi \; cm^2$

$35.7 \; cm$

Total perimeter of sector = Length of arc + radius + radius

Total perimeter of sector = $5\pi + 10 +10 \; cm$

Total perimeter of sector = $5\pi + 20 \; cm$

This answer is in terms of pi

$51.4 \; cm$

$10\pi \; cm$

$10\pi + 20 \; cm^2$

$10\pi + 20 \; cm$

Total perimeter of sector = Length of arc + radius + radius

Total perimeter of sector = $10\pi + 10 +10 \; cm$

Total perimeter of sector = $5\pi + 20 \; cm$

This answer is in terms of pi

$107.4 \; cm$

$192\pi \; cm^2$

$24\pi +32 \; cm$

$24\pi \; cm$

Total perimeter of sector = Length of arc + radius + radius

Total perimeter of sector = $24\pi + 16 +16 \; cm$

Total perimeter of sector = $5\pi + 32 \; cm$

This answer is in terms of pi

$30.0 \; cm$

$21.99 \; cm$

$14\pi \; cm^2$

$7\pi +8 \; cm$

Total perimeter of sector = Length of arc + radius + radius

Total perimeter of sector = $7\pi + 4 +4 \; cm$

Total perimeter of sector = $7\pi + 8 \; cm$

Total perimeter of sector = $29.9911 \; cm$

Total perimeter of sector = $30.0 \; cm$

Perimeter of a sector GCSE questions

1. The diagram shows a sector of a circle with centre $O$ .

The radius of the circle is $13cm.$

The angle of the sector is $150^o.$

Perimeter of a sector total GCSE Question 1

Calculate the perimeter of a sector, give your answer correct to $3$ significant figures

(4 marks)

Show answer

$\frac{150}{360} \times 2\times\pi \times 13 \quad$ or $\quad 34.0339$

(1)

$“34.0339” + 13 + 13$

(1)

$60.0339$

(1)

$60.0$

(1)

2. The diagram shows a sector of a circle with centre $O$ .

The radius of the circle is $6.5cm.$

The acute angle $AOB$ is $70^o$

Perimeter of a sector total GCSE Question 2

Calculate the perimeter of a sector, give your answer correct to $3$ significant figures.

(4 marks)

Show answer

$\frac{290}{360} \times 2\times\pi \times 6.5 \quad$ or $\quad 32.899$

(1)

$“32.899” + 13 + 13$

(1)

$58.899$

(1)

$58.9$

(1)

3. The diagram shows a sector of a circle with centre $O$ ..

The radius of the circle is $4.9cm.$

The acute angle $AOB$ is $60^o$

Perimeter of a sector total GCSE Question 3

Calculate the perimeter of a sector, give your answer correct to $2$ significant figures.

(4 marks)

Show answer

$\frac{60}{360} \times 2\times\pi \times 4.9 \quad$ or $\quad 5.1312$

(1)

$“5.1312” + 4.9 + 4.9$

(1)

$14.9312$

(1)

$14.93$

(1)

4. Below is a sector of a circle with an perimeter of a sector of $60cm$ and a radius of $10cm$

Find the size of the angle labelled $x.$

Give you answer correct to $3$ significant figures.

Perimeter of a sector total GCSE Question 4

(5 marks)

Show answer

Correct attempt to form an equation for the perimeter of a sector

$60=\frac{x}{360} \times 2 \times \boldsymbol{\pi} \times 10 + 20$

(1)

Attempt to solve equation for $x$

$40 =\frac{x}{18} \pi$

$40 \div \frac{\pi}{18} = x$

(2)

$229.18$

(1)

$229$

(1)

5. The sector below has a perimeter of a sector of $30cm.$

Calculate the length of $x.$

Give your answer to $2$ decimal places

Perimeter of a sector total GCSE Question 5

(5 marks)

Show answer

Correct attempt to form an equation for the perimeter of a sector

30=\frac{135}{360} \times 2 \times \boldsymbol{\pi} \times x +2x

(1)

Attempt to solve equation for $x$

\begin{aligned} &30=\frac{3}{4} \pi x + 2x \\\\ &30 =x(\frac{3}{4} \pi + 2x ) \\\\ &30 =x(4.356) \\\\ \end{aligned}

(2)

6.8867

(1)

6.89

(1)

Learning checklist

You have now learned how to:

Identify and apply circle definitions and properties, including: centre, radius, chord, diameter, circumference

Identify and apply circle definitions and properties, including tangent, arc, sector and segment

Calculate the length of an arc

Calculate the perimeter of a sector

Calculate a property of a circle given the perimeter of a sector

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.