Collecting like terms

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Addition and subtraction

Negative numbers

BIDMAS

This topic is relevant for:

GCSE Maths Algebra Simplifying Expressions

Collecting Like Terms

Here we will learn about simplifying algebraic expressions by collecting like terms.

There are also collecting like terms worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

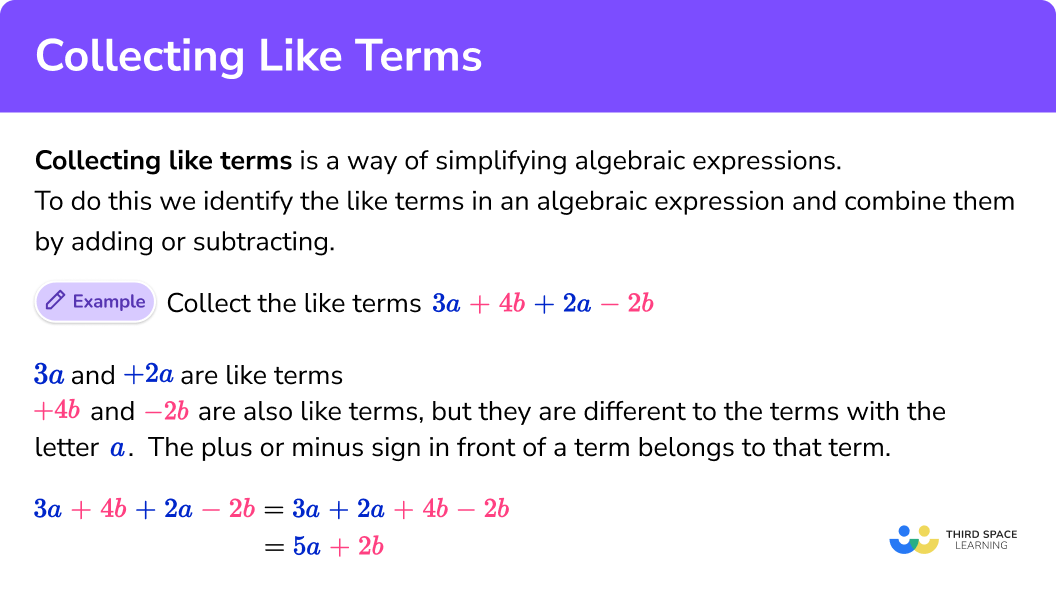
What do we mean by collecting like terms?

Collecting like terms is a way of simplifying algebraic expressions. It is also known as combining like terms.
To do this we identify the like terms in an algebraic expression and combine them by adding or subtracting.

See also: Simplifying expressions

E.g.
This is an expression with 4 terms:

\[3a +4b + 2a -2b\]

3a and +2a are like terms (they have the same letter).
+4b and -2b are also like terms, but they are different to the terms with the letter a.
The plus or minus sign in front of a term belongs to that term.

\[\begin{align*} 3a +4b + 2a – b &= 3a + 2a+ 4b – b\\ &=5a+2b\\ \end{align*}\]

This expression cannot be simplified any more as the 5a and the +2b are unlike terms.

What do we mean by collecting like terms?

How to collect like terms

In order to simplify algebraic expressions by collecting like terms:

Identify the like terms
Group the like terms
Combine the like terms by adding or subtracting

Explain how to collect like terms

Collecting like terms worksheet

Get your free Collecting like terms worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Collecting like terms worksheet

Get your free Collecting like terms worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Collecting like terms examples

Example 1: one type of like term

Simplify:

\[ 3x+2x +4x \]

Identify the like terms

All the terms involve an x. They are all the same type of term.

\[ 3x+2x +4x \]

2Group the like terms

The like terms are already grouped together.

\[3x+2x +4x \]

3Combine the like terms by adding or subtracting

\[3x+2x +4x =9x\]

The final answer is

\[9x\]

Example 2: one type of like term

Simplify:

\[ 6a-3a +4a \]

Identify the like terms

All the terms involve an a. They are all the same type of term.

\[ 6a-3a +4a\]

Group the like terms

The like terms are already grouped together.

\[6a-3a +4a \]

Combine the like terms by adding or subtracting

\[6a-3a +4a=7a \]

Because

\[6-3+4=7\]

The final answer is:

\[7a\]

Example 3: two types of like terms

Simplify:

\[7a+4b+2a+3b\]

Identify the like terms

The terms involving a are like terms (7a and +2a)
The terms involving b are like terms (+4b and +3b)
The plus (or minus) sign belongs to the term after it.

Group the like terms

\[7a+4b+2a+3b=7a+2a+4b+3b\]

Combine the like terms by adding or subtracting

\[\begin{align*} 7a+4b+2a+3b &=7a+2a+4b+3b\\ &=9a+7b\\ \end{align*}\]

Because

\[7+2=9\]

And

\[4+3=7\]

The final answer is:

\[9a+7b\]

Example 4: two types of like terms

Simplify the linear expression:

\[5x+4y-3x+5y\]

Identify the like terms

The terms involving x are like terms (5x and -3x)
The terms involving y are like terms ( +4y and +5y)
The plus or minus sign belongs to the term after it.

Group the like terms

\[5x+4y-3x+5y=5x-3x+4y+5y\]

Combine the like terms by adding or subtracting

\[\begin{align*} 5x+4y-3x+5y &=5x-3x+4y+5y\\ &=2x+9y\\ \end{align*}\]

Because

\[5-3=2\]

And

\[4+5=9\]

The final answer is

\[2x+9y\]

Example 5: three types of like terms

Simplify the algebraic terms:

\[2c-5d+4+3c+7d+2\]

Identify the like terms

The terms involving c are like terms (2c and +3c)
The terms involving d are like terms (-5d and +7d)
The terms that are only numbers are known as constants (+4 and +2)
The plus or minus sign belongs to the term after it.

Group the like terms

\[ 2c-5d+4+3c+7d+2= 2c+3c-5d+7d+4+2\]

Combine the like terms by adding or subtracting

\[\begin{align*} 2c-5d+4+3c+7d+2 &=2c+3c-5d+7d+4+2\\ &=5c+2d+6\\ \end{align*}\]

Because

\[2+3=5\]

And

\[-5+7=2\]

And

\[4+2=6\]

The final answer is

\[5c+2d+6\]

Example 6: three types of like terms

Simplify:

\[-6x+5y+4+2x-3y-7\]

Identify the like terms

The terms involving x are like terms (-6x and +2x)
The terms involving y are like terms (+5y and -3y)
The terms that are only numbers are known as constants (+4 and -7)
The plus or minus sign belongs to the term after it.

Group the like terms

\[ -6x+5y+4+2x-3y-7= -6x+2x+5y-3y+4-7\]

Combine the like terms by adding or subtracting

\[\begin{align*} -6x+5y+4+2x-3y-7 &=-6x+2x+5y-3y+4-7\\ &=-4x+3y-3\\ \end{align*}\]

Because

\[-6+2=-4\]

And

\[5-3=2\]

And

\[4-7=-3\]

The final answer is

\[-4x+2y-3\]

Example 7: variables with exponents

Simplify:

\[8x^{2}+5x-4x^{2}+7x\]

Identify the like terms

The terms involving x² are like terms (8x $^{2}$ and -4x $^{2}$ )
The terms involving x are like terms (+5x and +7x)
The plus or minus sign belongs to the term after it.

Group the like terms

\[8x^{2}+5x-4x^{2}+7x = 8x^{2}-4x^{2}+5x+7x\]

Combine the like terms by adding or subtracting

\[\begin{align*} 8x^{2}+5x-4x^{2}+7x &= 8x^{2}-4x^{2}+5x+7x\\ &=4x^{2}+12x\\ \end{align*}\]

Because

\[8-4=4\]

And

\[5+7=12\]

The final answer is

\[4x^{2}+12x\]

Example 8: variables with exponents

Simplify:

\[6x^{2}y-2x^{2}+4x^{2}y-5x^{2}\]

Identify the like terms

The terms involving x²y are like terms (6x $^{2}$ y and +4x $^{2}$ y)
The terms involving x² are like terms (-2x $^{2}$ and -5x $^{2}$ )
The plus or minus sign belongs to the term after it.

Group the like terms

\[6x^{2}y-2x^{2}+4x^{2}y-5x^{2}=6x^{2}y+4x^{2}y-2x^{2}-5x^{2}\]

Combine the like terms by adding or subtracting

\[\begin{align*} 6x^{2}y-2x^{2}+4x^{2}y-5x^{2}&=6x^{2}y+4x^{2}y-2x^{2}-5x^{2}\\ &=10x^{2}y-7x^{2}\\ \end{align*}\]

Because

\[6+4=10\]

And

\[-2-5=-7\]

The final answer is

\[10x^{2}y-7x^{2}\]

Common misconceptions

No coefficient (number) seen in front of a term

If there is no coefficient (number) seen in front of a term then the coefficient is 1, but we do not write the number 1.

\[x+4x=1x+4x=5x\]

When asked to simplify an algebraic expression it is possible for the answer to be zero

It is possible for all the terms to be cancelled out and the answer is zero.

E.g.

\[5x+3y-5x+4y=7y\]

The terms involving x have cancelled out. We do not write 0x.

Terms must be exactly the same to be “like terms”

Terms involving y and y² are unlike terms and cannot be collected together by adding or subtracting.

\[3y+4y^2+2y=5y+4y^2\]

This has been simplified as far as possible.

The order of the terms in the final answer is not important

The order of the terms is not critical as long as the plus and minus signs are with the correct term.

So:

\[3m+4n=4n+3m\]

And:

\[-2x+5y=5y-2x\]

Practice collecting like terms questions

9x

11x

8x

9xxx

4x+2x+3x=9x

Because:

4+2+3=9

5a^3

9a^3

9a

5a

3a-2a+4a=5a

Because:

3-2+4=5

7c+7d

3c+7d

7c-d

10cd

\begin{aligned} 5c+3d-2c+4d &=5c-2c+3d+4d\\ &=3c+7d\\ \end{aligned}

3x+3y

11x+7y

3x+7y

6xy

\begin{aligned} 7x-2y-4x+5y &=7x-4x-2y+5y\\ &=3x+3y\\ \end{aligned}

5m+5n+11

6m+8n+7

21mn

5m+7n+7

\begin{aligned} 2m+3n+1+4m+5n+6 &=2m+3n+1+4m+5n+6\\ &=6m+8n+7\\ \end{aligned}

4p+6q+8

8p+6q+8

4p-2q+8

8p-2q+8

\begin{aligned} 6p-4q+5-2p+2q+3 &=6p-2p-4q+2q+5+3\\ &=4p-2q+8\\ \end{aligned}

Collecting like terms GCSE questions

1. Simplify: $9a-4a+3a$

(1 mark)

Show answer

$8a$ i

(1)

2. Simplify: $7x+5y-2x+3y$

(2 marks)

Show answer

for either $5x$ or $8y$

(1)

$5x+8y$ i

(1)

3. The diagram shows a pentagon. It has one line of symmetry.

Collecting like terms GCSE question 3 1

$AE$ = $5x$
$AB$ = $2x+3$
$BC$ = $x+2$

Write an expression for the perimeter in its simplest form.

(3 marks)

Show answer

$CD$ = $x+2$
$DE$ = $2x+3$
for using symmetry to find one of the sides $CD$ or $DE$

(1)

Perimeter is: $5x+2x+3+x+2+2x+3+x+2$
for adding the $5$ sides together

(1)

for simplifying and writing the final answer: $11x+10$

(1)

Learning checklist

You have now learned how to:

Simplify algebraic expressions by collecting like terms

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Introduction

What is collecting like terms?

How to collect like terms

Collecting like terms worksheet

Collecting like terms examples

↓

Example 1: one type of like term Example 2: one type of like term Example 3: two types of like terms Example 4: two types of like terms Example 5: three types of like terms Example 6: three types of like terms Example 7: variables with exponents Example 8: variables with exponents

Common misconceptions

Practice collecting like terms questions

Collecting like terms GCSE questions

Learning checklist

Next lessons

Still stuck?