Equations

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Algebraic notation Simplify algebraic expressions Substitution

Polynomials

This topic is relevant for:

GCSE Maths Algebra

Equations

Here we will learn about equations, including solving equations, linear equations, quadratic equations, simultaneous equations and rearranging equations.

There are also equations worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What are equations?

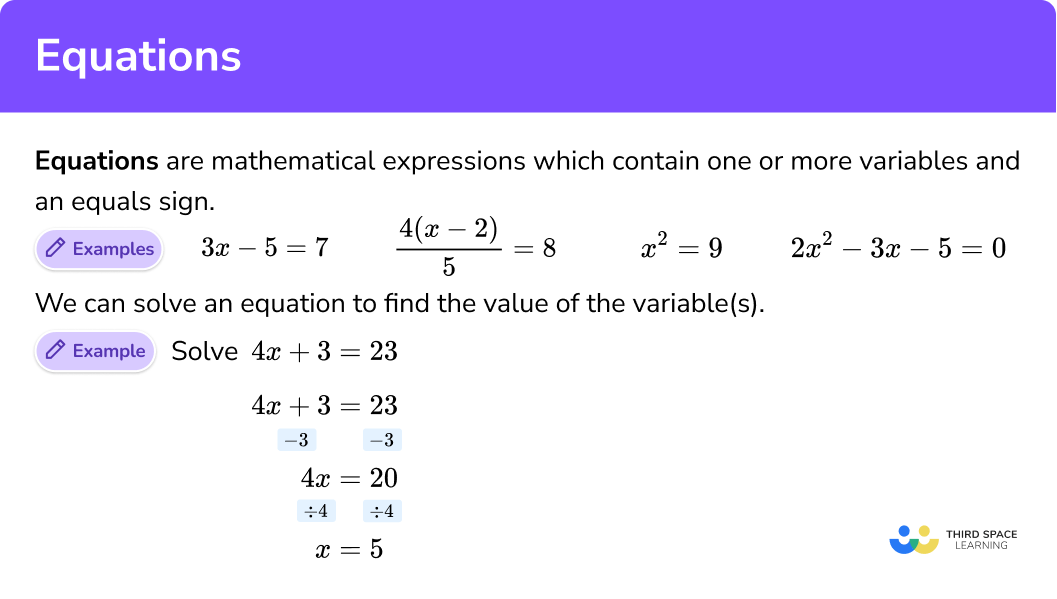
Equations are mathematical expressions which contain a variable and an equals sign.

We can solve different types of equations to find the value of the variable.

E.g.

\begin{aligned} &3x-5=7 \\\\\\ &\frac{4(x-2)}{5}=8 \\\\\\ &x^2=9 \\\\\\ &2x^2-3x-5=0 \end{aligned}

What are equations?

What are simultaneous equations?

Simultaneous equations are a pair of equations with two variables. They can be solved to find a pair of values which make both equations true at the same time.

Step-by-step guide: Simultaneous equations

E.g. Linear simultaneous equations

\begin{aligned} x+y&=10\\\\ x-y&=4 \end{aligned}

E.g. Quadratic simultaneous equations

y=x^2-6x+8

y=2x+1

What is rearranging equations?

Rearranging equations means we change the subject of the equation to display it in a different way.

Step-by-step guide: Rearranging equations

E.g.

The subject of the following equation is currently $y$ .

y=3x+2

We can rearrange the mathematical equation to make $x$ the subject:

x=\frac{y-2}{3}

Sometimes an equation is a formula. This is when it is used to solve a specific problem.

E.g.

Here is the formula to work out the area of a circle:

A=\pi r^2

It could be rearranged to find the radius if we are given the area:

r=\sqrt{\frac{A}{\pi }}

Equations worksheet

Get your free equations worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Equations worksheet

Get your free equations worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Equations and identities

Equations and identities look very similar, but are two different things.

An identity is true for all values of the variable, whereas the equation is true for only certain values.

For example, the equation:

3x+5=14

can be solved, so it is true only when $x=3$ .

However the identity:

3(x+2)\equiv3x+6

It can not be solved. The left-hand side always equals the right-hand side for all values of $x$ .

At A-level you will be introduced to a variety of trigonometric identities to enable you to prove trigonometric expressions such as each pythagorean identity (derived from the pythagoras theorem) or the double angle identities.

Identity sign

Algebraic identities use the ‘≡’ symbol. It is like an equals sign =, but it means ‘identical to’.

Iteration

Iteration is a process in which the approximate solution is determined using an iterative formula and a known starting number. When using iteration to calculate a root of an equation, the values converge towards a fixed point.

Step-by-step guide: Iteration

Solving equations

Types of equations

Linear equations

Simple linear equations are solved by using a “balancing method” by applying the inverse operation to both sides of the equation such as addition, subtraction, multiplication, division or using powers and square roots. They can be checked by substituting the answer back into the original equation.

Step-by-step guide: Linear equations

Linear equations – one unknown

E.g.

Solve: $3x+4=16$

Linear equations – unknowns on both sides

E.g.

Solve: $4x+7=2x+12$

Linear equations – with brackets

E.g.

Solve: $3(x-5)=18$

Equations with fractions

E.g.

Solve: $\frac{5}{x}=10$

In order to solve the equation you need to multiply both sides by the denominator.

Simple equations – with powers and roots

Whilst these are NOT linear equations they are included here as the method used to solve them is very similar.

E.g.

Solve: $4x^2+1=35$

Step-by-step guide: Equations with fractions

Quadratic equations

Quadratic equations are solved by using different methods. They can be checked by substituting the answer back into the original equation.

Step-by-step guide Quadratic equations

Quadratic equations – by factorising

We factorise the quadratic and then solve each factor being equal to $0$ , one at a time.

E.g.

Solve: $x^2-5x+6=0$

$\begin{aligned} x^2 -5x+6 &= 0\\\\ (x-2)(x-3) &= 0 \\\\ \end{aligned}$

$\begin{aligned} x-2&=0 \quad \text{or} \quad x-3=0\\\\ x&=2 \quad \text{or} \quad x=3 \\\\ \end{aligned}$

Step-by-step guide: Solving quadratic equations by factorising

Quadratic equations – quadratic formula

We can use the quadratic formula to solve any quadratic equation of the form

$ax^2+bx+c$

The quadratic formula is: $x=\frac{-b \pm \sqrt{b^{2}-4 a c}}{2 a}$

E.g.

Solve: $3x^2-4x-5=0$

Give your answers to $2$ decimal places

$\begin{aligned} &3x^{2}-4x-5=0\\\\ x&=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\\\\ a&=3, \quad b=-4, \quad c=-5\\\\ x&=\frac{-(-4)\pm\sqrt{(-4)^2-4(3)(-5)}}{2(3)}\\\\ x&=\frac{4\pm\sqrt{76}}{6} \\\\ x&=2.1196... \quad \text{or} \quad x=-0.78629...\\\\ x&=2.12 \quad \text{or} \quad x=-0.79 \end{aligned}$

Step-by-step guide: Quadratic formula

Quadratic equations example – completing the square

E.g.

Solve: $x^2-4x-5=0$

$\begin{aligned} x^2 -4x-5 &= 0\\\\ x^2-4x&=5\\\\ (x-2)^2 -4&=5\\\\ (x-2)^2 &= 9\\\\ x-2 &= \pm 3\\\\ x&=2\pm 3\\\\ x=5 \quad &\textrm{or} \quad x=-1 \end{aligned}$

Step-by-step guide: Completing the square

Quadratic equations example – graphically

E.g.

Using the graph of $y=x^2-6x+8$ , solve: $x^2-6x+8=0$

The solutions are to be found where $y=0$

$x=2 \quad \textrm{or} \quad x=4$

Step-by-step guide: Solving quadratic equations graphically

Simultaneous equations

Simultaneous linear equations

E.g.

Solve these simultaneous equations:

$\begin{aligned} 2x+3y&=14\\\\ 6x-y&=12 \end{aligned}$

Equations - Simultaneous linear equations 3a

Step-by-step guide: Simultaneous equations

Quadratic simultaneous equations

E.g.

Solve these simultaneous equations:

$\begin{aligned} y&=x^2-6x+8\\\\ y&=2x+1 \end{aligned}$

\[\begin{aligned} x^2-6x+8&=2x+1\\ x^2-8x+7&=0\\ (x-7)(x-1)&=0\\ \\ x=7 \quad &\text{or} \quad x=1\\ \\ \text{substitue} \quad x=7 \quad \text{into} \quad y&=2x+1\\ y&=2(7)+1=15\\ \\ \text{substitue} \quad x=1 \quad \text{into} \quad y&=2x+1\\ y&=2(1)+1=3\\ \\ \text{solutions are} \quad x=7,\quad y=15& \quad \text{or} \quad x=1, \quad y=3\\ \end{aligned}\]

Step-by-step guide: Quadratic simultaneous equations

Step-by-step guide: Quadratic simultaneous equations

Solve simultaneous equations graphically

E.g.

Draw graphs to solve the simultaneous equations:

$\begin{aligned} 2x+y=5 \\\\ y=2x-1 \end{aligned}$

Draw the graphs on the same axes and see where they intersect.

Equations - Simultaneous linear equations 3c

The two straight lines intersect at $(1.5, 2),$ so the solution is $x=1.5$ and $y=2$

Step-by-step guide: Solving simultaneous equations graphically

Rearranging equations

Example:

Make $t$ the subject of: $v=u+at$

\begin{aligned} &v=u+at \\\\ &v-u=at\\\\ &\frac{v-u}{a}=t\\\\ &\text{Therefore} \\\\ &t= \frac{v-u}{a} \end{aligned}

Step-by-step guide: Make $x$ the subject

Common misconceptions

Solutions to equations are not always positive whole numbers

Solutions to equations can be positive integers (whole numbers), but they can also be negative. They can also be decimals or fractions.

Don’t try to just write down the answer

When working with equations, take each step one at a time. Keep your workings neat and tidy so you are more likely to be correct.

If you multiply both sides (or divide) make sure that you do this to each and every term

When you are working with an equation and you need to multiply (or divide), it is easy to make a mistake. Make sure you apply the multiplication to every term.

For example:

Each term on both sides is multiplied by $3$

\begin{aligned} \frac{x}{3}&=4x-5\\\\ x&=12x-15 \end{aligned}

Practice equations questions

x=5

x=10

x=6

x=9

\begin{aligned} 4x+10&=30\\\\ 4x&=20\\\\ x&=5 \end{aligned}

x=4

x=5

x=6

x=3

\begin{aligned} 2(x+5)&=3x+4\\\\ 2x+10&=3x+4\\\\ 10&=x+4\\\\ 6&=x\\\\ \text{therefore} \quad x&=6 \end{aligned}

x=31

x=25

x=27

x=29

\begin{aligned} \frac{x-4}{3}&=7\\\\ x-4&=21\\\\ x&=25 \end{aligned}

x=2 \quad \text{or} \quad x=4

x=8 \quad \text{or} \quad x=6

x=-8 \quad \text{or} \quad x=-6

x=-2 \quad \text{or} \quad x=-4

\begin{aligned} x^2+6x+8&=0\\\\ (x+2)(x+4)&=0\\\\ x=-2 \quad \text{or} \quad x&=-4 \end{aligned}

x=4 \quad \text{and} \quad y=2

x=4 \quad \text{and} \quad y=1

x=3 \quad \text{and} \quad y=1

x=3 \quad \text{and} \quad y=2

\begin{aligned} 2x+5y&=13 \quad [1]\\ x+2y&=6 \quad [2]\\ \\ [1]\times2 \quad 4x+10y&=26 \quad [3]\\ [2]\times5 \quad 5x+10y&=30 \quad[4]\\ \hline [4]-[3] \qquad \qquad x&=4\\ \\ \text{substitute} \quad x=4 \quad \text{into} \quad[2]\\ 4+2y&=6\\ 2y&=2\\ y&=1\\ \\ \text{solution is} \quad x=4 \quad \text{and} \quad y=1 \end{aligned}

h=V-\pi r^2

h=\frac{\pi V}{r^2}

h=\frac{V}{\pi r^2}

h=\sqrt{\frac{V}{\pi r}}

\begin{aligned} V &= \pi r^2 h\\\\ \frac{V}{\pi} &= r^2h\\\\ \frac{V}{\pi r^2} &=h\\\\ \text{therefore} \quad h&= \frac{V}{\pi r^2} \end{aligned}

Equations GCSE questions

1. Solve:

3x-4=12

(2 marks)

Show answer

3x=16

(1)

x=5\frac{1}{3}

(1)

2. Solve the simultaneous equation.

\begin{aligned} 2x+y&= 15\\\\ x-y &= 6 \end{aligned}

(3 marks)

Show answer

Add the 2 equations

2x+x=15+6

(1)

\begin{aligned} 3x&= 21\\\\ x &= 7 \end{aligned}

(1)

\begin{aligned} 2x+y&= 15\\\\ 2(7)+y &= 15\\\\ 14+y&=15\\\\ y&=1 \end{aligned}

(1)

3. Solve:

x^2-x-20=0

(3 marks)

Show answer

\begin{aligned} x^2-x-20&=0\\\\ (x\pm a)(x\pm b) &= 0 \end{aligned}

(1)

(x-5)(x+4)=0

(1)

$x=5 \quad \text{or} \quad x=-4$

(1)

4. Rearrange the equation to make $x$ the subject:

y=\frac{x}{4}-5

(2 marks)

Show answer

\begin{aligned} y&=\frac{x}{4}-5\\\\ y+5&=\frac{x}{4} \end{aligned}

(1)

\begin{aligned} 4(y+5)&=x\\\\ x&=4(y+5) \end{aligned}

(1)

Learning checklist

You have now learned how to:

Use algebraic methods to solve linear equations in

1

variable

Rearrange formulae to change the subject

To find approximate solutions of simultaneous linear equations

Solve quadratic equations {including those that require rearrangement} algebraically by factorising

Solve quadratic equations by completing the square

Solve quadratic equations by using the quadratic formula

Solve quadratic equations – find approximate solutions using a graph

Solve

2

simultaneous linear equations in

2

variables algebraically

Solve

2

simultaneous equations in

2

variables – one linear and one quadratic algebraically

Solve

2

simultaneous equations in

2

variables – find approximate solutions using a graph

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.