3D Pythagoras

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Substitution Squares and square roots Solving equations Rounding 3D shapes Pythagoras’ Theorem

This topic is relevant for:

GCSE Maths Geometry and Measure Pythagoras Theorem

3D Pythagoras

Here we will learn about 3D Pythagoras including how to apply Pythagoras’ theorem in three dimensional situations.

There are also 3D Pythagoras worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is 3D Pythagoras?

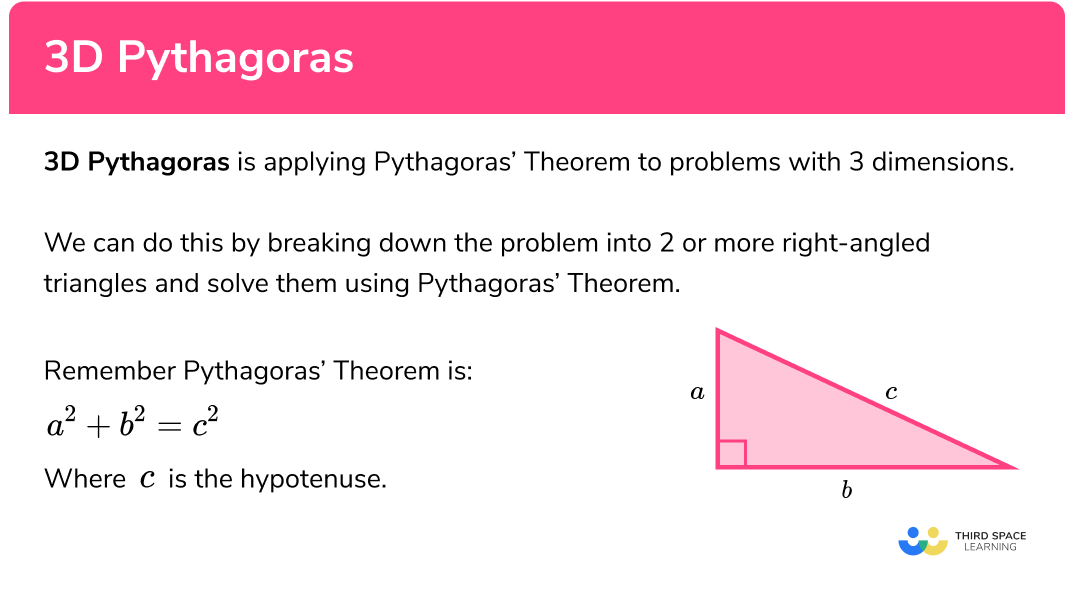
3D Pythagoras is applying Pythagoras’ theorem to problems with 3 dimensions.

We can do this by breaking down the problem into 2 or more right-angled triangles and solve them using Pythagoras’ theorem.

Remember Pythagoras’ theorem is:

\[a^2+b^2=c^2\]

Where c is the hypotenuse.

See also: Pythagoras theorem

What is 3D Pythagoras?

3D Pythagoras worked example

Let’s take a look at an example:

We are going to find the length AG in the cuboid ABCDEFGH.

We can see that we can make a right angled triangle ACG which we can use to work out AG.

In order to use Pythagoras’ theorem we need to know two sides of the triangle. So in order to work out the longest side AG we first need to work out one of the shorter sides AC.

Let’s call this side x and redraw this triangle.

We can see that the side we have labeled x forms the diagonal line of the base of the cuboid.

We can see that the triangle ABC is a right angled triangle so we can use Pythagoras’ theorem to calculate x.

\[AC=\sqrt{10^2 + 4^2} = 2\sqrt{29} = 10.7703…\]

So,

\[AG=\sqrt{10.7703…^2 + 6^2} = \sqrt{152}=2\sqrt{38} = 12.328…\]

So the required length is 12.3 cm (correct to 1 decimal place).

3D Pythagoras for cuboids

We can also use a special 3D Pythagoras’ Theorem for cuboids where x, y, z are the sides of a cuboid and s is the longest diagonal in the cuboid.

\[x^2+y^2+z^2=s^2\]

E.g

\[\begin{aligned} &s^{2}=10^{2}+4^{2}+6^{2} \\ &s=\sqrt{10^{2}+4^{2}+6^{2}} \\ &s=12.3 \mathrm{~cm} \quad(1 . \mathrm{d} \cdot \mathrm{p}) \end{aligned}\]

If angles and lengths are involved in the problem, we may also need to use trigonometry.

See also: Trigonometry

How to solve problems using 3D Pythagoras

In order to solve problems in three-dimensions using Pythagoras’ theorem:

Identify the useful right angled triangle(s).
Apply Pythagoras’ Theorem.
Finalise your answer.

How to solve problems using 3D Pythagoras

3D Pythagoras worksheet

Get your free 3D Pythagoras worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

3D Pythagoras worksheet

Get your free 3D Pythagoras worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

3D Pythagoras examples

Example 1: cones

Find the slant length of the cone with height 10 cm and radius 8 cm. Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

We can draw a diagram of the cone and look for the right-angled triangle. The slant length, l, is the hypotenuse of the right-angled triangle with the radius and the height being the adjacent sides to the right-angle.

2Apply Pythagoras’ Theorem.

\[\begin{align*} a^2 + b^2 &= c^2 \\ 8^2 + 10^2 &= l^2\\ l^2 &= 8^2+10^2\\ l^2 &= 64 + 100\\ l^2 &=164\\ l &= \sqrt{164} \\ \end{align*}\]

3Finalise your answer.

\[l = \sqrt{164}=12.8062…\]

The slant length of the cone is 12.8 cm (to 1 decimal place).

This is useful as we need the slant length in order to find the curved surface area of a cone.

Example 2: cones

Find the height of the cone with radius 12 cm and slant length 17 cm. Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

Apply Pythagoras’ Theorem.

\[\begin{align*} a^2 + b^2 &= c^2 \\ h^2 + 12^2 &= 17^2\\ h^2 &= 17^2-12^2\\ h^2 &= 289 -144\\ h^2 &=145\\ h &= \sqrt{145} \\ \end{align*}\]

Finalise your answer.

\[h = \sqrt{145}=12.04159…\]

The height of the cone is 12.0 cm (to 1 decimal place).

This is useful as we need the height in order to find the volume of a cone.

Example 3: cuboids

Here is a cuboid ABCDEFGH. Find the length AG. Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

Apply Pythagoras’ Theorem.

\[AC=\sqrt{20^2 + 7^2} =\sqrt{449} = 21.1896…\]

So,

\[AG=\sqrt{21.1896…^2 + 11^2} = \sqrt{570} = 23.87467…\]

Finalise your answer.

\[AG = \sqrt{570}=23.87467…\]

The length AG is 23.9 cm (to 1 decimal place)

Alternatively we could have done this in one step:

\[AG=\sqrt{20^2 + 7^2 + 11^2}=\sqrt{570}=23.87467…\]

Example 4: cuboids

Here is a cuboid ABCDEFGH. Find the length BH. Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

Apply Pythagoras’ Theorem.

\[BD=\sqrt{5.6^2 + 8.1^2} = 9.84733…\]

So,

\[AG=\sqrt{9.84733…^2 + 12.3^2} = 15.75626…\]

Finalise your answer.

\[AG=15.75626…\]

The length AG is 15.8 cm (to 1 decimal place).

Alternatively we could have done this in one step:

\[AG=\sqrt{8.1^2 + 5.6^2 + 12.3^2}=15.75626…\]

Example 5: pyramids

The diagram shows a square-based pyramid ABCDX.

\[AB=12 cm\]

\[AX=BX=CX=DX=17 cm\]

The point M is the midpoint of AB.

Calculate the length MX.

Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

Triangle ABX is an isosceles triangle. MX and AB are perpendicular.

Apply Pythagoras’ Theorem.

\[MX=\sqrt{17^2 – 6^2} = 15.90597…\]

Finalise your answer.

\[MX=15.90597…\]

The length MX is 15.9 cm (to 1 decimal place).

This is useful as we need the height of the triangles in order to find the surface area of a pyramid.

Example 6: pyramids

The diagram shows a square-based pyramid ABCDX.

\[AB=20 cm\]

\[AX=BX=CX=DX=30 cm\]

M is the centre of the square base ABCD.

Calculate the length MX.

Give your answer correct to 1 decimal place.

Identify the useful right angled triangle(s).

Apply Pythagoras’ Theorem.

\[AC=\sqrt{20^2 + 20^2} =\sqrt{800} = 20\sqrt{2}=28.28427…\]

So,

\[AM=\frac{1}{2}AC=10\sqrt{2}=14.14213…\]

\[MX=\sqrt{30^2 – 14.14213…^2} = 10\sqrt{7} = 26.45751…\]

Finalise your answer.

\[MX = 10\sqrt{7}=26.45751…\]

The length MX is 26.5 cm (to 1 decimal place).

This is useful as we need the height of the pyramid in order to find the volume of a pyramid.

Common misconceptions

Three dimensions can be difficult to visualise

It can be tricky to identify where right angles are on a 2D diagram of a 3D shape. You may find it useful to use a box to help you to visualise it, such as an old tissue box or a shoe box.

Use all of the digits on the calculator screen

It is very easy to make a mistake at the end with rounding. Make sure you use all of the digits on the calculator screen and only round your final answer at the end of the question. Rounding too soon will lose accuracy.

Lengths of sides do not have to be whole numbers

Lengths can be decimals, fractions or even irrational numbers such as surds
E.g.
$\sqrt{2}$ .

Practice 3D Pythagoras questions

18.0 cm

18.1 cm

11.2 cm

11.1 cm

l=\sqrt{15^2 + 10^2}=18.02775…

12.9 cm

13.0 cm

29.8 cm

29.9 cm

l=\sqrt{23^2 – 19^2}=12.96148…

13.1 cm

12.4 cm

12.5 cm

13.2 cm

AG=\sqrt{10^2 + 3^2 + 8^2}=\sqrt{173}=13.1529…

20.2 cm

20.3 cm

12.4 cm

12.5 cm

BH=\sqrt{7^2 + 6^2 + 18^2}=\sqrt{409}=20.22374…

17.2 cm

14.9 cm

17.3 cm

15.0 cm

MX=\sqrt{18^2 – 5^2}=\sqrt{299}=17.29161…

41.3 cm

26.5 cm

26.4 cm

41.2 cm

AC =\sqrt{40^2 + 40^2} = \sqrt{3200}= 40\sqrt{2} = 56.5685…

So,
$AM=\frac{1}{2}AC=20\sqrt{2}=28.28427…\\ MX=\sqrt{50^2 – 28.28427…^2} = 10\sqrt{17} = 41.2310…$

3D Pythagoras GCSE questions

1. Rectangle ABCD is the horizontal base of a triangular prism ABCDEF.
$AE = BE\\ AB = 10 cm\\ AE = 17 cm\\ BC = 25 cm$

Work out the volume of the prism. Give your answer correct to $3$ significant figures.

(5 marks)

Show answer

$h^2=17^2 - 5^2$
for starting to use Pythagoras to find the height

(1)

$h=\sqrt{17^2 – 5^2} = 2\sqrt{66} = 16.24807…$
for finding the height of the triangle

(1)

$Area = \frac{1}{2}\times 10 \times 2\sqrt{66} = 10\sqrt{66}=81.24038…$
for finding the area of the triangle

(1)

$Volume = 10\sqrt{66} \times 25 = 2031.0096…$
for finding the volume of the triangular prism

(1)

$Volume = 2030 cm^3$
for the final volume

(1)

2. The diagram shows a box in the shape of a cuboid ABCDEFGH.

AB = 12.5 cm\\ AE = 6.7 cm\\ BC = 3.8 cm

A string runs diagonally across the box from D to F.

Calculate the length of the string DF.
Give your answer correct to $3$ significant figures.

(3 marks)

Show answer

$DB=\sqrt{12.5^2 + 3.8^2} = 13.06483…$
for using Pythagoras to find DB

(1)

$DF=\sqrt{13.06483…^2 + 6.7^2} = 14.68264…$
for using Pythagoras to find DF

(1)

$DF = 14.7 cm$
for the final length

(1)

3. A cone has a radius of $10 cm$ .
The curved surface area of the cone is $800 cm^2$ .
Find the volume of the cone.
Give your answer correct to $3$ significant figures.

(5 marks)

Show answer

$\pi \times 10 \times l=800$
for setting up the equation to find the slant length

(1)

$l=\frac{800}{10\pi}=\frac{80}{\pi}$
for finding the slant length

(1)

$h=\sqrt{(\frac{80}{\pi})^2-10^2}=23.419128…$
for finding the height

(1)

$V=\frac{1}{3}\times \pi \times 10^2 \times 23.419128… = 2452.445…$
for finding the volume

(1)

$V=2452.445\dots = 2450 cm^3$
insert for the final answer

(1)

Learning checklist

You have now learned how to:

Use and apply Pythagoras’ theorem to solve problems in three dimensions

Solve multi-step problems

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.