Exterior angles of a polygon

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

2D shapes Angles on a straight line Angles in a triangle Polygons Regular polygon Irregular polygon

This topic is relevant for:

GCSE Maths Geometry and Measure Angles Angles In Polygons

Exterior Angles Of A Polygon

Here is everything you need to know about exterior angles in polygons for GCSE maths (Edexcel, AQA and OCR). You’ll learn how to calculate the sum of exterior angles for a polygon, a single exterior angle and use this knowledge to solve problems.

At the end you’ll find worksheets based on Edexcel, AQA and OCR GCSE exam style questions, along with further guidance on where to go next if you’re still stuck.

What are exterior angles?

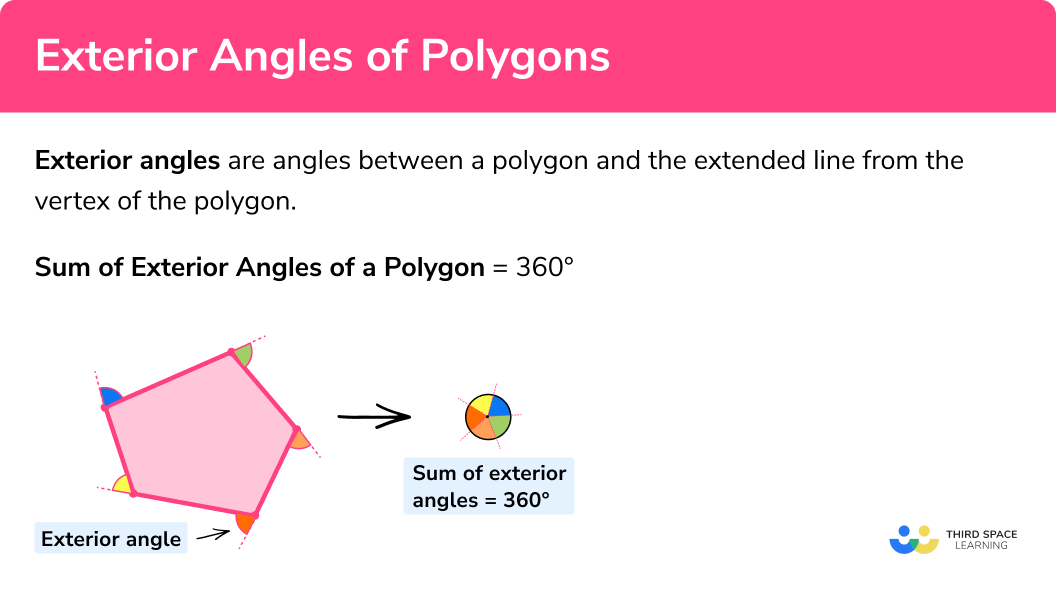
Exterior angles are angles between a polygon and the extended line from the vertex of the polygon.

Sum of exterior angles of a polygon = 360º

Interior and exterior angles form a straight line – they add to 180º.

Check out our lessons on interior angles of polygons and sum of the interior angles to find out more.

What are exterior angles?

Keywords

Polygon:
A polygon is a two dimensional shape with at least three sides, where the sides are all straight lines.

Regular & irregular polygons:
A regular polygon is where all angles are equal size and all sides are equal length
E.g. a square.
An irregular polygon where all angles are not equal size and/or all sides are not equal length
E.g. a trapezium

How to solve problems involving exterior angles

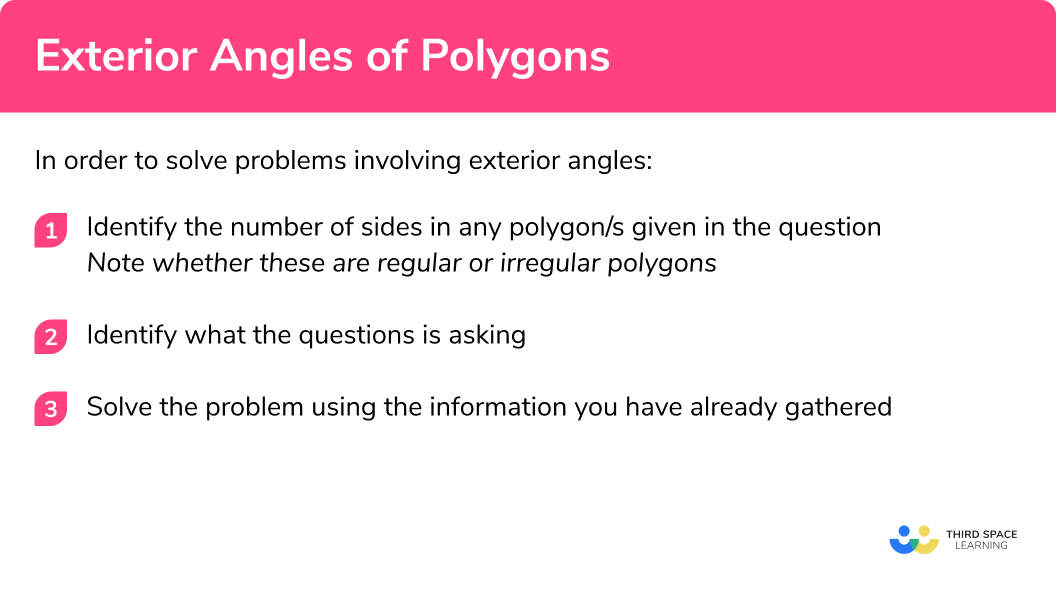
In order to solve problems involving exterior angles following these steps:

Identify the number of sides in any polygon/s given in the question.
Note whether these are regular or irregular polygons.
Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$
Solve the problem using the information you have already gathered with use of the formulae interior angle $\textbf{+}$ exterior angle $\, \textbf{=} \; \bf{180^{\circ}}$ and Sum of interior angles $\, \textbf{=} \; \bf{(n-2) \times 180^{\circ}}$ if required

How to solve problems involving exterior angles.

Interior and exterior angles worksheet (includes exterior angles of a polygon)

Get your free interior and exterior angles worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Interior and exterior angles worksheet (includes exterior angles of a polygon)

Get your free interior and exterior angles worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on angles in polygons

Exterior angles of a polygon is part of our series of lessons to support revision on angles in polygons. You may find it helpful to start with the main angles in polygons lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Exterior angles of polygons examples

Example 1: finding the size of a single exterior angle for a regular polygon

Calculate the size of a single exterior angle for a regular hexagon.

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular shapes.

A hexagon has 6 sides.

Regular – therefore all exterior angles are equal.

2Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

The size of one exterior angle.

We know the sum of exterior angles for a polygon is 360°.

3Solve the problem using the information you have already gathered with use of the formulae interior angle $\textbf{+}$ exterior angle $\, \textbf{=} \; \bf{180^{\circ}}$ and Sum of interior angles $\, \textbf{=} \; \bf{(n-2) \times 180^{\circ}}$ if required

\[360 \div 6 = 60\]

The size of each exterior angle is 60º.

Example 2: finding an exterior angle given an interior angle for an irregular polygon

An irregular octagon has one interior angle of size 130º. What is the size of the adjacent exterior angle?

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular polygons.

8 sides and irregular – (irregular octagon).

Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

As adjacent means next to we are being asked to find the size of the exterior angle which is on an straight line with the interior angle.

Solve the problem using the information you have already gathered with use of the formulae interior angle $\textbf{+}$ exterior angle $\, \textbf{=} \; \bf{180^{\circ}}$ and Sum of interior angles $\, \textbf{=} \; \bf{(n-2) \times 180^{\circ}}$ if required

We know that angles on a straight line add to 180º, so if the interior angle is 130º then the exterior angle will be 50º.

Example 3: interior + exterior angle = 180º

Calculate angle x.

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular polygons.

6 sides – irregular hexagon.

The interior angles of a hexagon add to 720 degrees.

Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

Find the exterior angle x.

It is an irregular polygon so the exterior angles are not all equal.

We can work out the missing interior angle of the polygon.

\begin{aligned} 150+120+90+160+130+a&=720 \\\\ 650+a&=720 \\\\ a&=70 \end{aligned}

The interior angle + the exterior angle must equal 180º

Therefore 70 + exterior angle = 180º

So x = 110º.

Example 4: finding the number of sides given the exterior angle of a regular polygon

An exterior angle of a regular polygon is 20º. How many sides does the polygon have?

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular polygons.

Unknown number of sides.

Regular polygon – therefore all exterior angles are equal.

Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

We need to find the number of sides.

We know the sum of the exterior angles is 360º and we know that each exterior angle is equal because it is a regular polygon.

\begin{aligned} 20 \times \text { number of sides }&=360\\\\ 20n&=360 \\\\ n&=18 \end{aligned}

Therefore the polygon has 18 sides.

Example 5: finding the number of sides given the interior angle of a regular polygon

The size of each interior angle of a regular polygon is 150º. How many sides does the polygon have?

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular polygons.

Unknown number of sides.

Regular polygon – therefore all exterior angles are equal.

Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

We need to find the number of sides.

We know the sum of the exterior angles is 360º and we know that each exterior angle is equal because it is a regular polygon.

We also know that the sum of an interior and an exterior angle is 180º.

If the interior angle is 150º then the exterior angle will be 30º.

The number of sides can therefore be calculated by 360 ÷ 30 = 12

The polygon has 12 sides.

Example 6: multi step problem involving interior and exterior angles

The size of each interior angle of a regular polygon is 11 times the size of each exterior angle. Work out the number of sides the polygon has.

Identify the number of sides in any polygon/s given in the question. Note whether these are regular or irregular polygons.

Unknown sides.

Regular polygon – therefore each exterior angle is equal.

Identify what the question is asking recalling that the Sum of exterior angles $\, \textbf{=} \; \bf{360^{\circ}}$

Number of sides of the polygon.

Other Information we know:

Total of Exterior Angles = 360º

Interior + Exterior Angle = 180º

11 × Interior Angle = Exterior Angle

We will call each of the interior angles x.

Since ‘11 × Interior Angle = Exterior Angle’ we can call each exterior angle 11x.

Therefore

\begin{aligned} x+11 x&=180 \\\\ 12 x&=180 \\\\ x&=15 \end{aligned}

The size of one exterior angle is 15º.

The number of sides of the polygon is 360 ÷ 15 = 24

The Polygon has 24 sides.

Common misconceptions

Misidentifying the exterior angle

E.g.

The exterior angle of a triangle is the angle between the side and the extension of an adjacent side.

Here the interior angle (internal angle) is 60º, so the exterior angle (external angle) must be 120^º.

Exterior Angles of Polygons Common Misconceptions

Miscounting the number of sides

Misidentifying if a polygon is regular or irregular

Incorrectly assuming all the angles are the same size

Misidentifying which angle the question is asking you to calculate

Practice exterior angles of a polygon questions

90^{\circ}

60^{\circ}

180^{\circ}

270^{\circ}

Exterior angles of a polygon add up to $360$ . A regular quadrilateral has $4$ interior angles equal in size, so the four exterior angles are equal.

This means we can divide $360$ by $4$ to get the solution.

45^{\circ}

60^{\circ}

40^{\circ}

135^{\circ}

Exterior angles of a polygon add up to $360$ . A regular octagon has $8$ interior angles equal in size, so the eight exterior angles are equal.

This means we can divide $360$ by $8$ to get the solution.

90^{\circ}

40^{\circ}

140^{\circ}

280^{\circ}

Exterior angles of a polygon add up to $360$ . A regular nonagon has $9$ interior angles equal in size, so the nine exterior angles are equal.

This means we can divide $360$ by $9$ to get the solution.

 $12$  sides

 $20$  sides

 $30$  sides

 $32$  sides

Exterior angles of a polygon add up to $360$ .

This means we can divide $360$ by $12$ to get the solution.

 $12$  sides

 $20$  sides

 $18$  sides

 $16$  sides

Exterior angles of a polygon add up to $360$ .

This means we can divide $360$ by $20$ to get the solution.

 $125^{\circ}$

 $40^{\circ}$

 $55^{\circ}$

 $140^{\circ}$

The four known exterior angles will be $55^{\circ}$ , since angles on a straight line sum to $180$ . This means the fifth exterior angle will be $140^{\circ}$ because exterior angles add up to $360$ .

Using angles on a straight line once more means that the missing angle is $40^{\circ}$ .

Exterior angles of a polygon GCSE questions

1. A regular polygon has $15$ sides. Calculate the size of one exterior angle.

(1 mark)

Show answer

$360 \div 15 = 24$

$= 24^{\circ}$

(1)

2. (a) The diagram below shows part of a regular polygon. Calculate the size of the exterior angle of the polygon.

Exterior Angles of Polygons Exam Question 2a

(b) Work out how many sides this polygon has.

(3 marks)

Show answer

(a)

$180 - 162=18$

$= 18^{\circ}$

(1)

(b)

$360 \div 18$

(1)

$20$

(1)

3. Shown below are parts of two regular polygons.

Exterior Angles of Polygons Exam Question 3

Polygon A has $9$ sides and an exterior angle of $x.$

Polygon B has an interior angle of $3x.$

How many sides does polygon B have?

(4 marks)

Show answer

$360 \div 9 = 40$

(1)

$x = 40, 3x = 120$

(1)

Polygon B: Interior angle is $120^{\circ}$ , exterior angle is $60^{\circ}$

(1)

$360 \div 60 = 6$

(1)

Learning checklist

You have now learned how to:

Use conventional terms for geometry e.g. exterior angle

Derive a formula for the total of exterior angles for a polygon and consequently calculate the sum of exterior angles for a regular polygon

Solve problems involving interior and exterior angles

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.