Types of numbers

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Arithmetic Negative numbers Sequences Fractions to decimals

This topic is relevant for:

GCSE Maths Number

Types Of Numbers

Here we will learn about different types of numbers and how to solve problems using them.

There are also types of numbers worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What are types of numbers?

Types of numbers are different sets of numerical values.

To determine the type of number, you must use the definition to see whether the number belongs to the set.

Understand number definitions and terms

A number is a count or measurement.

We write or talk about numbers using numerals such as $‘6’.$ We need to know the different types of numbers to be able to solve problems.

Positive and negative numbers

Any number above zero is a positive number and any number below zero is a negative number.

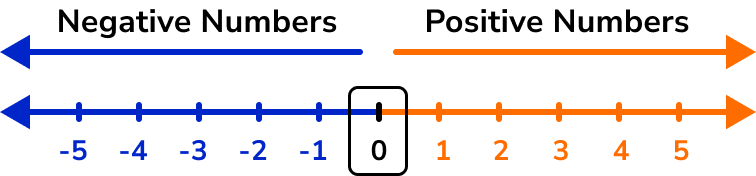
Real numbers

All numbers on a number line are real numbers.

Real numbers can also be classified as rational or irrational.

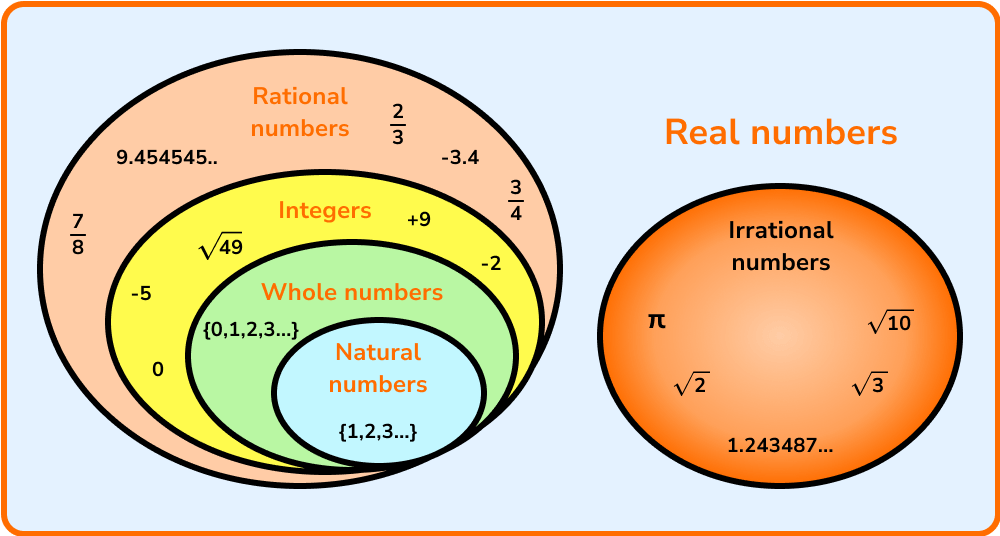
Rational and irrational numbers

We can make a rational number by dividing an integer by an integer.

Irrational numbers are the real numbers that cannot be expressed in fraction form.

Step-by-step guide: Rational numbers

Natural numbers are positive integers (including $0$ ). We can also call these ‘counting’ numbers’.

For example,

Even and odd

Even numbers are divisible by $2$ without remainders; they end in $0, 2, 4, 6$ or $8.$

Odd numbers are not divisible by $2$ without remainders and end in $1, 3, 5, 7$ or $9.$

For example,

Decimals

We can recognise decimal numbers because they have a whole number and a fractional part. The decimal point separates the whole number from the fractional part.

For example,

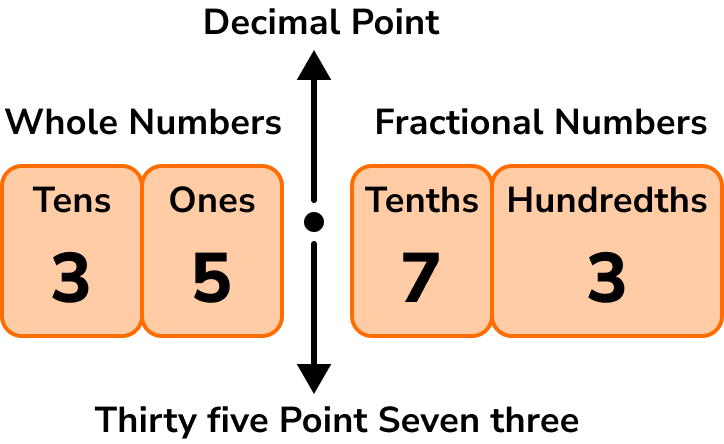
Step-by-step guide: Decimals

Prime numbers and composite numbers

A prime number is a number which is only divisible by $1$ and itself. We can define composite numbers as whole numbers that have more than two factors.

For example,

$3 = 3 \times 1,$ so $3$ is prime.

$6= 3 \times 2,$ or $6= 1 \times 6. \ 6$ has more than two factors, so $6$ is composite.

We also need to recognise the properties of some other ‘special’ numbers.

Step-by-step guide: Prime numbers

Square numbers

Square numbers are the product of a number that has been multiplied by itself.

We can show square numbers using a pattern of dots in a square.

For example,

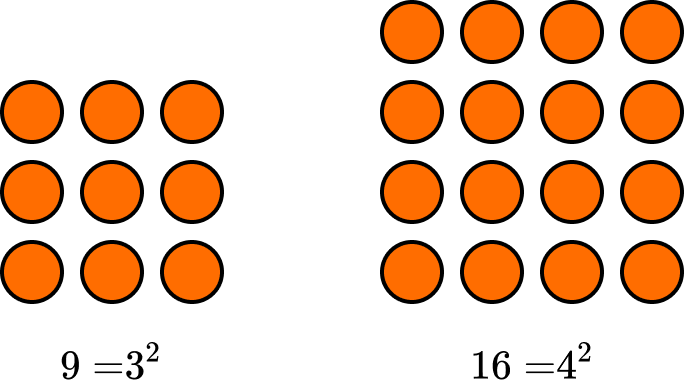
Step-by-step guide: Squares and square roots

Cube numbers

A Cube number is the product when a number has been multiplied by itself and then itself again. We can show cube numbers using 3D cubes.

For example,

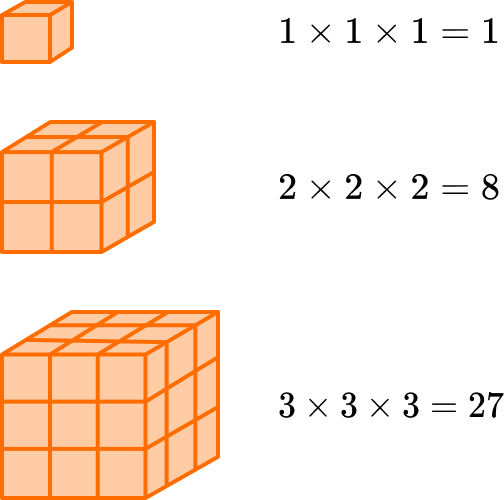
Step-by-step guide: Cube and cube roots

Triangular numbers

A Triangular number is a number that can be shown using a pattern of dots in an equilateral triangle. We can find a triangular number by cumulatively adding natural numbers.

For example,

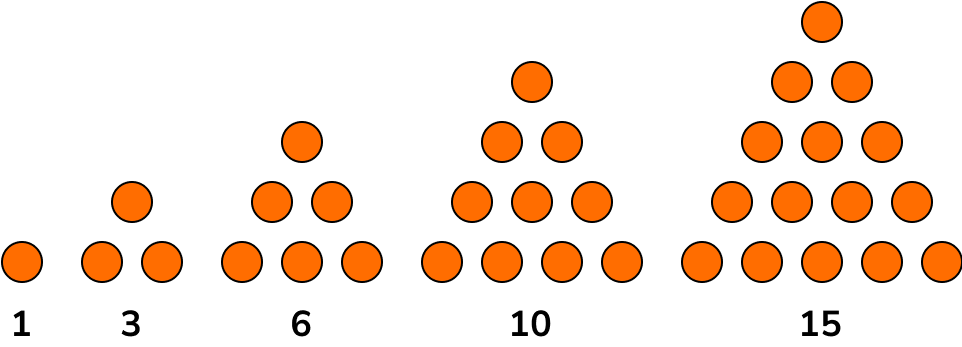
Step-by-step guide: Triangular numbers

Reciprocal maths

A reciprocal of a number is its multiplicative inverse. This means that if you multiply a number by its reciprocal the answer is $1.$

To find a reciprocal you divide $1$ by the number.

For example, the reciprocal of $3$ is $\frac{1}{3}.$

Step-by-step guide: Reciprocal maths (coming soon)

What are types of numbers?

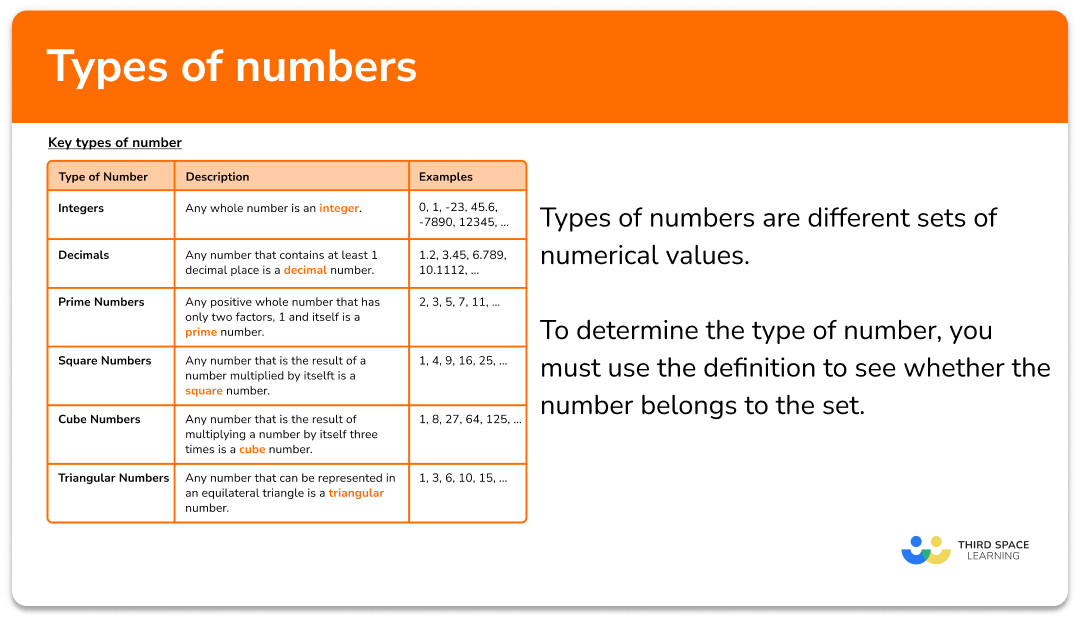
Key types of number

Below is a description of each of these types of numbers, along with a few examples.

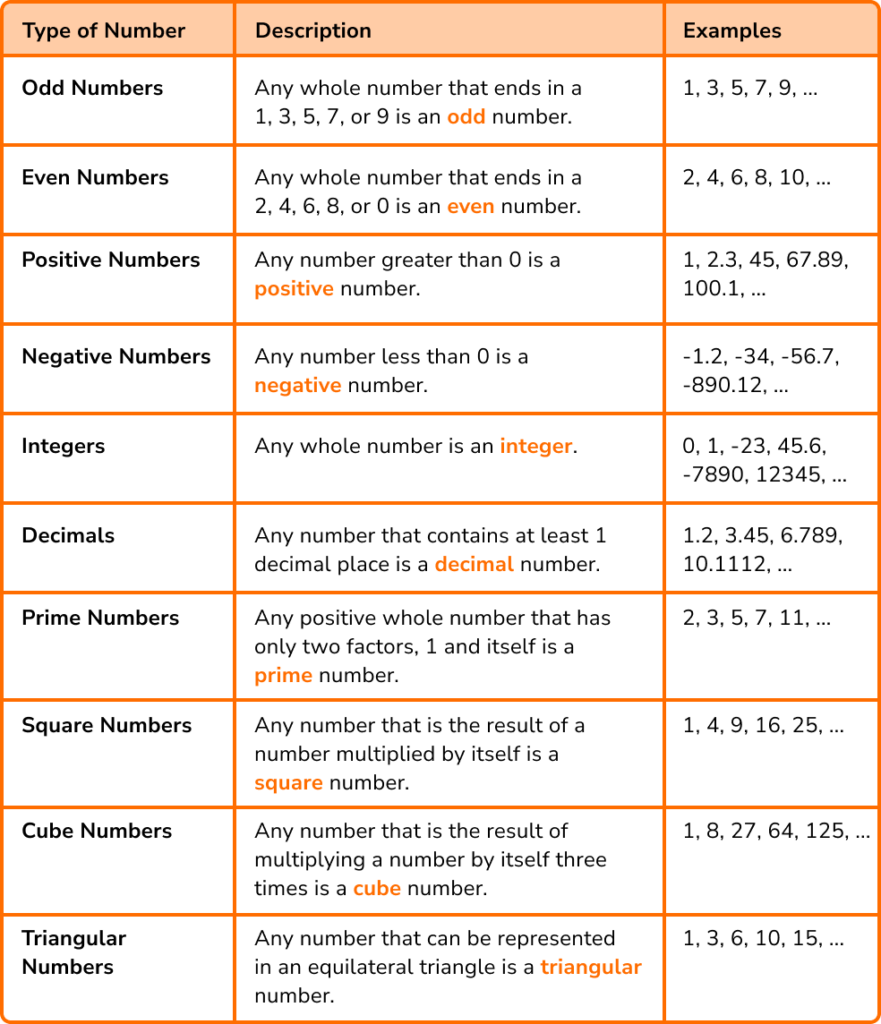
Other types of number

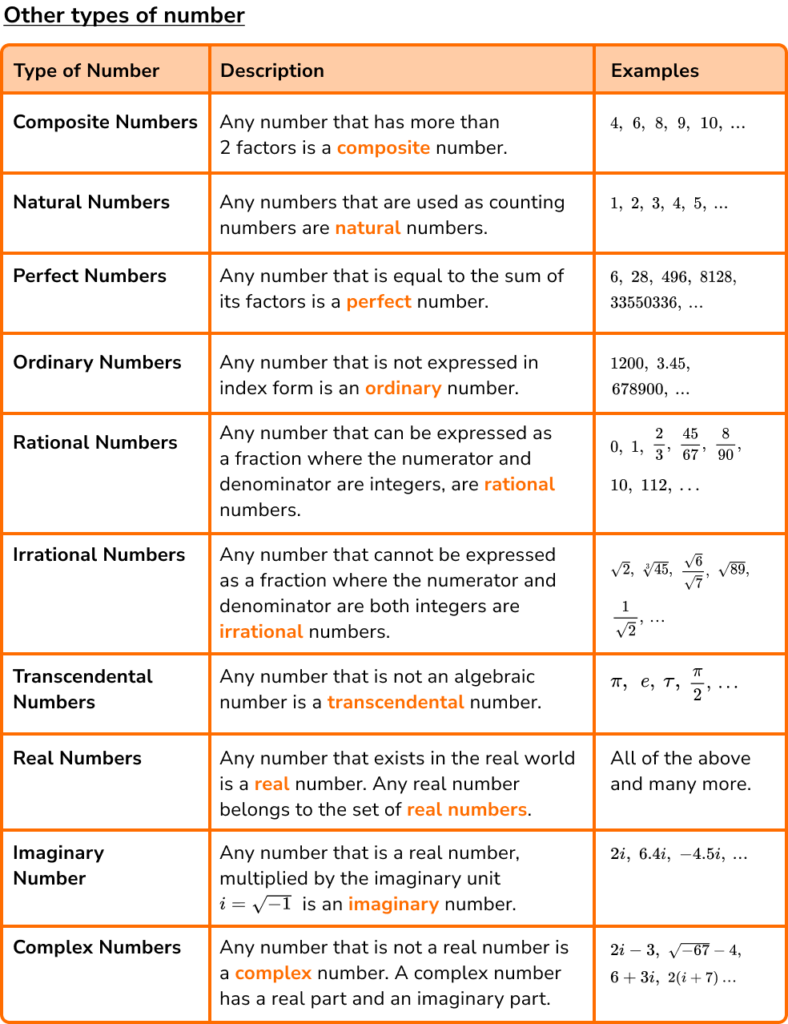
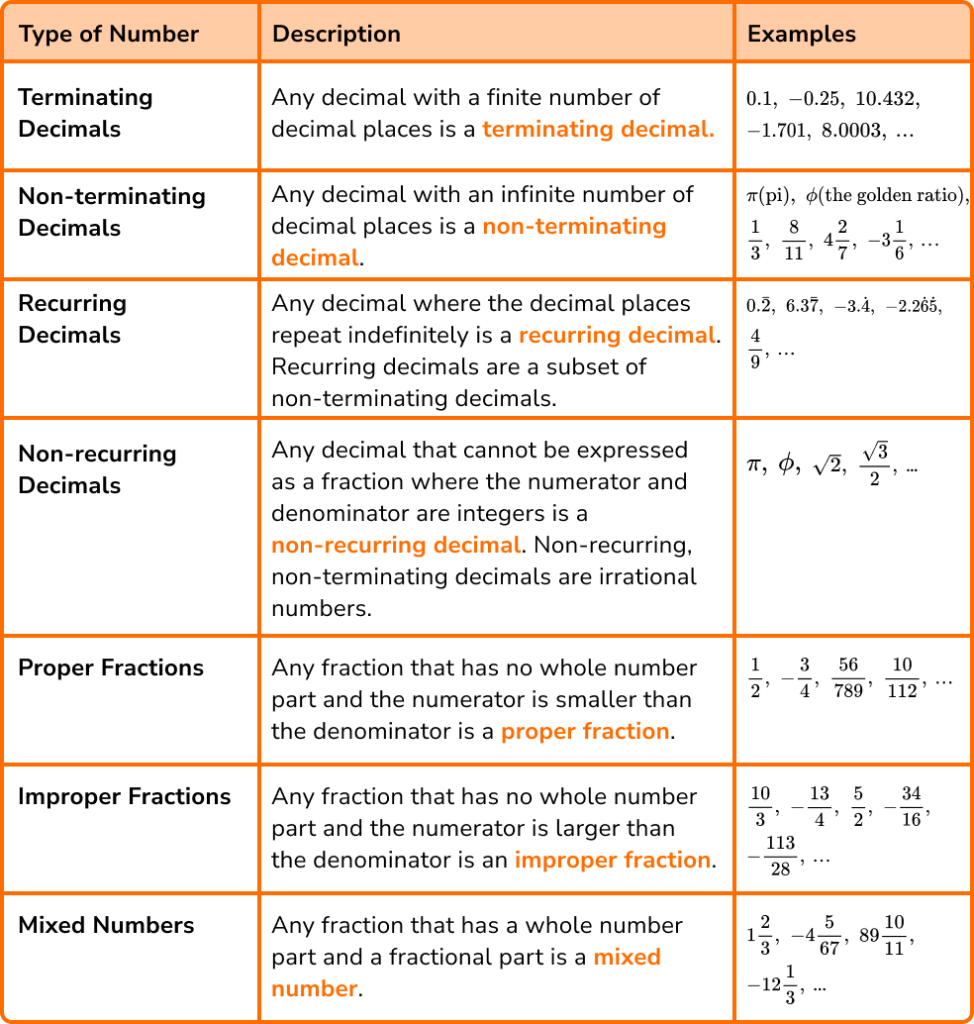
Fractions and decimals

There are also a few specific types of numbers involving fractions and decimals that you are expected to understand. Below is a table outlining these different types of fractions and decimals.

How to determine the type of a number

In order to determine the type of a number:

Recall the definition of the type of number needed.
Show that the number does (not) fit the definition.
Conclude your answer.

Explain how to determine the type of a number

Types of numbers worksheet

Get your free types of numbers worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Types of numbers worksheet

Get your free types of numbers worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Types of number examples

Example 1: square numbers

Show that the number $36$ is a square number.

Recall the definition of the type of number needed.

The definition of a square number is any number that is the result of a number multiplied by itself.

2Show that the number does (not) fit the definition.

36=6 \times 6

3Conclude your answer.

$36$ is a square number because it can be calculated by multiplying $6$ by itself.

Example 2: prime numbers

Determine whether the number $23$ is a prime number.

Recall the definition of the type of number needed.

The definition of a prime number is any positive whole number that has only two factors, $1$ and itself.

Show that the number does (not) fit the definition.

The factors of $23$ are $1$ and $23.$

Conclude your answer.

$23$ is a prime number as it only has two factors, $1$ and $23$ (itself).

Example 3: rational numbers

Show that $\sqrt{3}\times\sqrt{27}$ is a rational number.

Recall the definition of the type of number needed.

The definition of a rational number is any number that can be expressed as a fraction where the numerator and denominator are integers.

Show that the number does (not) fit the definition.

Completing the calculation, we have

$\sqrt{3}\times\sqrt{27}=\sqrt{81}=9.$

Conclude your answer.

$\sqrt{3}\times\sqrt{27}=9,$ which is a rational number as $9$ can be written as a fraction where the numerator and denominator are integers, $\frac{9}{1}.$

Step-by-step guide: Surds

Example 4: recurring decimal

Show that $\frac{2}{9}$ is a recurring decimal.

Recall the definition of the type of number needed.

The definition of a recurring decimal is any decimal where the decimal places repeat indefinitely.

Show that the number does (not) fit the definition.

We need to calculate $2 \div 9$ to show that the decimal repeats itself. Using long division, we have

This means that $\frac{2}{9}=0.22…=0.\dot{2}.$

Conclude your answer.

$\frac{2}{9} =0.\dot{2},$ which is a recurring decimal.

Step-by-step guide: Recurring decimals to fractions

Example 5: mixed number

Write $\frac{21}{4}$ as a mixed number.

Recall the definition of the type of number needed.

The definition of a mixed number is any fraction that has a whole number part and a fractional part. The fractional part is a proper fraction.

Show that the number does (not) fit the definition.

Currently we have an improper fraction. To convert this to a mixed number, we need to calculate how many times the denominator goes into the numerator and determine the remainder.

$21\div{4}=5$ remainder $1,$ and so the whole number part of the mixed number is $5.$

The fractional part of the mixed number has a numerator of $1,$ and as we are dividing by $4,$ the denominator stays as $4.$

So we have $\frac{21}{4}=5\frac{1}{4}.$

Conclude your answer.

$\frac{21}{4}=5\frac{1}{4}$ as a mixed number.

Example 6: cube number in index form

The number $592704=2^{6}\times{3}^{3}\times{7}^{3}.$ Show that $592704$ is a cube number.

Recall the definition of the type of number needed.

The definition of a cube number is any number that is the result of multiplying a number by itself three times.

Show that the number does (not) fit the definition.

Using laws of indices, the cube root of $592704$ is the same as

$\begin{aligned} 592704&=(2^{6}\times{3}^{3}\times{7}^{3})^{\frac{1}{3}}\\\\ &=2^{\frac{6}{3}}\times{3}^{\frac{3}{3}}\times{7}^{\frac{3}{3}}\\\\ &=2^{2}\times{3}\times{7}\\\\ &=4\times{3}\times{7}\\\\ &=84 \end{aligned}$

Conclude your answer.

The cube root of $592704$ is $84,$ so $592704$ is a cube number $(84^{3}).$

Common misconceptions

One type of number is another (mixed up definitions)

It is very common that definitions get mixed up, for example, a recurring decimal is the opposite of a terminating decimal. The set of numbers that are not terminating decimals are non-terminating decimals. Within that set is the subset of recurring decimals.

All recurring decimals are non-terminating, but not all non-terminating decimals are recurring (think of $\pi$ and $\frac{1}{9}$ ).

Categorising zero as a natural number

Zero is not a natural number because these numbers are used as counting numbers and so the first natural number is $1.$

Mixing up squares, cubes and their roots

The square root of a number is written as the value squared, for example, $\sqrt{9}=3^2$ which is incorrect. The square root of $9$ is $3$ because $3 \times 3=9.$

Not recognising whole numbers as rational numbers

Rational numbers are numbers that can be expressed as a fraction where the numerator and the denominator are integers. The number $3$ is rational because we can express $3$ as the fraction $\frac{3}{1}.$

Fractions cannot contain decimals

They can, they just look mean! Take the fraction $\frac{2.3}{4.6}.$ At first glance, this does not look pleasant but by using equivalent fractions, we can multiply both the numerator and denominator by $10$ to get $\frac{23}{46}.$

A common factor of both of these numbers is $23$ and so this is equivalent to $\frac{1}{2}.$ When we simplify fractions, we deliberately avoid decimals, because the fractions become more complicated.

Practice types of numbers questions

Natural Number

Recurring Decimal

Proper Fraction

Complex Number

$22$ is a natural number because any number that is used as a counting number is a natural number.

Composite Number

Mixed Number

Imaginary Number

Terminating Decimal

$2.43$ is a terminating decimal because any decimal with a finite number of decimal places is a terminating decimal.

Improper Fraction

Perfect Number

Irrational Number

Exponent

$\frac{\sqrt{2}}{5}$ is an irrational number because the numerator is not an integer and the definition of an irrational number is any number that cannot be expressed as a fraction where the numerator and denominator are both integers.

Triangular Number

Cube Number

Square Number

Ordinary Number

$2^{8}\times{3}^{6}\times{5}^{12}$ is a square number because all of the exponents are even.

\sqrt{2^{8}\times{3}^{6}\times{5}^{12}}=(2^{8}\times{3}^{6}\times{5}^{12})^{\frac{1}{2}}=2^{4}\times{3}^{3}\times{5}^{6}

Ordinary Number

Perfect Number

Prime Number

Transcendental Number

The factors of $28$ are, $1, 2, 4, 7, 14,$ and $28.$

$1+2+4+7+14=28$ and so $28$ is a perfect number.

Complex Number

Irrational Number

Non-terminating Decimal

Square Number

Completing the calculation we have

\frac{\sqrt{25}\times\sqrt{4}}{0.025}=\frac{\sqrt{100}}{0.025}=\frac{10}{0.025}=400.

$400=20\times{20}$ and so $\frac{\sqrt{25}\times\sqrt{4}}{0.025}$ is a square number.

Types of number GCSE questions

1. Below is a table showing the base $10$ values for some roman numerals.

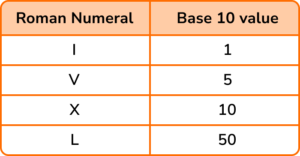
Types of numbers gcse question 1

(a) Given that $23$ is written as $XXIII,$ and $49$ is written as $IL,$ what is the value of $49-23$ in roman numerals?

(b) What type of number is your answer to part (a) in base $10?$ Circle all that may apply.

Positive Integer Terminating Decimal

Rational NumberNatural NumberPrime Number

(3 marks)

Show answer

(a)

$49-23=26$ which is written as $XXVI$ .

(1)

(b)

Any two of the following: Positive Integer, Rational Number, Natural Number.

(1)

All three.

(1)

2. Below is a list of five numbers.

3.\dot{3} \quad \quad \quad \frac{2}{7} \quad \quad \quad 0.43 \quad \quad \quad 4\frac{1}{2} \quad \quad \quad \frac{\pi}{2}

(a) Which number listed above is an irrational number?

(b) List all of the numbers above that are recurring decimals.

(2 marks)

Show answer

(a)

\frac{\pi}{2}

(1)

(b)

$3.\dot{3}$ and $\frac{2}{7}$ .

(1)

3. (a) Show that $\sqrt{2}(\sqrt{2} \times \sqrt{4})$ is a square number.

(b) Show that $\frac{3}{8} \times 16$ is a perfect number.

(5 marks)

Show answer

(a)

$\sqrt{2}(\sqrt{2} \times 2)$ or $\sqrt{2} \times 2\sqrt{2}$

(1)

2 \times \sqrt{4}=2 \times 2=4

(1)

(b)

\frac{3}{8} \times 16=3 \times 2=6

(1)

Factors of $6: 1, 2, 3, 6$

(1)

1+2+3=6

(1)

Learning checklist

You have now learned how to:

Understand and use the vocabulary of different types of numbers

Solve problems by reasoning using types of numbers

The next lessons are

Beyond GCSE

For GCSE Mathematics, the discriminant of the quadratic formula $b^{2}-4ac$ has $3$ possible conditions that determine the number and type of roots of a quadratic equation of the form $ax^{2}+bx+c=0.$ We say that for

$b^{2}-4ac>0,$ we have two distinct, real roots
$b^{2}-4ac=0,$ we have one repeated, real root
$b^{2}-4ac<0,$ there are no real solutions.

For the third type $b^{2}-4ac<0,$ the solutions are complex numbers. This is covered in detail at A Level.

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.