1. Complete the table of values to generate the coordinates for a linear graph with the equation $ y=4x+2. $ <img class="alignnone size-medium wp-image-155798" src="https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-Graph-Practice-question-1-300x51.png" alt="Linear Graph Practice question 1" width="300" height="51" srcset="https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-Graph-Practice-question-1-300x51.png 300w, https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-Graph-Practice-question-1.png 544w" sizes="(max-width: 300px) 100vw, 300px" />

2. Complete the table of values to generate the coordinates for a linear graph with the equation $ y=2x-1. $ <img class="alignnone size-medium wp-image-155803" src="https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-graph-practice-question-2-300x51.png" alt="Linear graph practice question 2" width="300" height="51" srcset="https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-graph-practice-question-2-300x51.png 300w, https://thirdspacelearning.com/wp-content/uploads/2023/02/Linear-graph-practice-question-2.png 543w" sizes="(max-width: 300px) 100vw, 300px" />

Linear graph

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Coordinates maths Substitution Arithmetic Reciprocal maths

This topic is relevant for:

GCSE Maths Algebra Types Of Graphs Straight Line Graphs

Linear Graph

Here we will learn about linear graphs, including plotting linear graphs, using the equation of a straight line and parallel and perpendicular lines.

There are also linear graph worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is a linear graph?

A linear graph is a straight line graph which represents a linear relationship between two variables. Linear graphs help us to illustrate how the relationship between these variables changes over time.

For example, Lewis buys a pay as you go phone. There is a standard monthly fee of $£5$ for the SIM card, and then Lewis will pay $50p$ for every text message he sends. This can be represented as a linear graph.

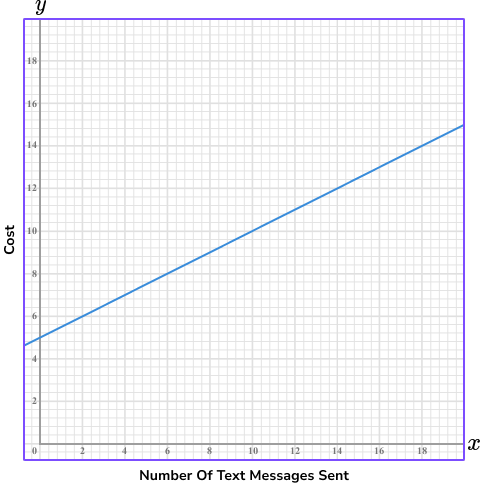
The standard monthly fee of $£5$ is the $y$ -intercept and the $50p$ charge per text message is the gradient (slope) of the line.

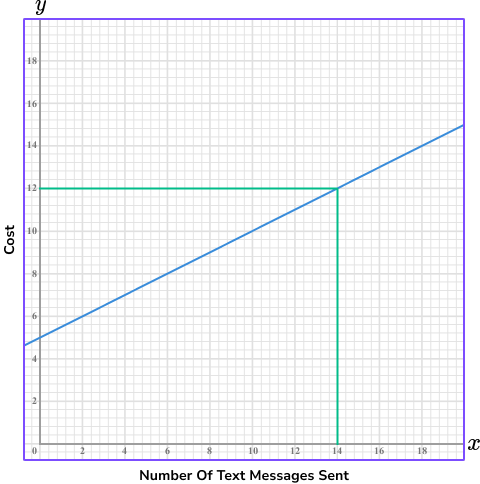
From this graph, we can predict Lewis’ monthly phone bill using the number of text messages he sends.

For example, if Lewis sends $14$ text messages, he will pay a total of $£12.$

What is a linear graph?

Drawing linear graphs

In order to draw linear graphs (also known as graphs of linear equations) we need to generate pairs of coordinates to plot onto a set of axes.

To generate these we substitute given $x$ values into the equation of the linear graph to work out the corresponding $y$ values. Once we have generated the coordinates, we plot these as a graph.

For example,

y=x+2.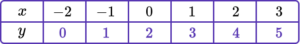

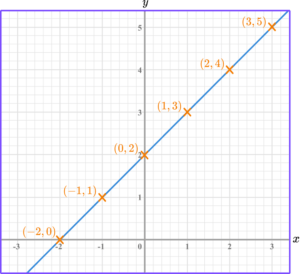
Step-by-step guide: Equation of a line

How to draw a linear graph

In order to draw a linear graph:

Substitute the $\textbf{x}$ coordinate into the equation of a straight line.
Plot the paired $\textbf{x}$ and $\textbf{y}$ coordinates onto the axes.
Join the points with a straight line.

Explain how to draw a linear graph

Straight line graph worksheet (includes linear graph)

Get your free linear graph worksheet of 20+ straight line graph questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Straight line graph worksheet (includes linear graph)

Get your free linear graph worksheet of 20+ straight line graph questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on straight line graphs

Linear graph is part of our series of lessons to support revision on straight line graphs. You may find it helpful to start with the main straight line graphs lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Linear graph examples

Example 1: drawing linear graphs

Draw the graph of the equation $y=3x+1$ for all values of $x$ from $-2$ to $2.$

Substitute the $\textbf{x}$ coordinate into the equation of a straight line.

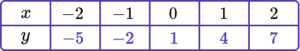
2Plot the paired $\textbf{x}$ and $\textbf{y}$ coordinates onto the axes.

3Join the points with a straight line.

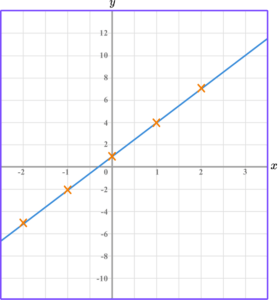
Example 2: drawing linear graphs

Draw the graph of the equation $y=2x-3$ for all values of $x$ from $-2$ to $2.$

Substitute the $\textbf{x}$ coordinate into the equation of a straight line.

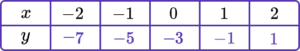
Plot the paired $\textbf{x}$ and $\textbf{y}$ coordinates onto the axes.

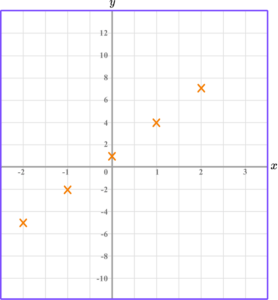
Join the points with a straight line

Example 3: drawing linear graphs

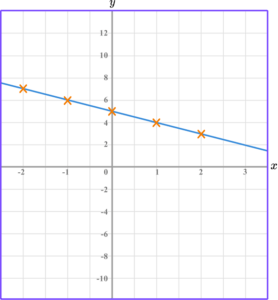
Draw the graph of the equation $y=5-x$ for all values of $x$ from $-2$ to $2.$

Substitute the $\textbf{x}$ coordinate into the equation of a straight line.

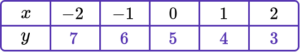
Plot the paired $\textbf{x}$ and $\textbf{y}$ coordinates onto the axes.

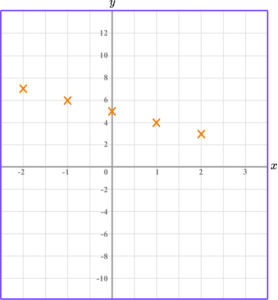
Join the points with a straight line

Gradient of a linear graph

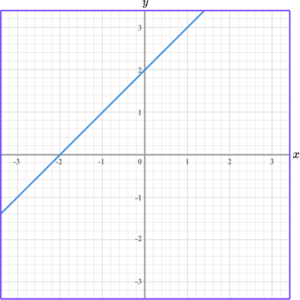
The gradient of a linear graph tells us how steep the line is. The higher the gradient, the steeper the line. If the gradient is positive, the line goes up, from left to right. If the gradient is negative, the line goes down, from left to right.

For example, the linear graph below has a positive gradient. It moves in an upward direction, from left to right.

Linear Graph Gradient of a linear graph example 1

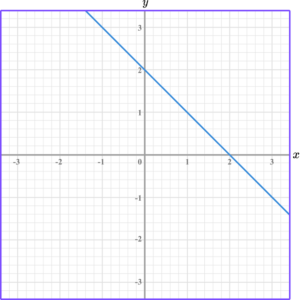
This linear graph has a negative gradient. It moves in a downwards direction, from left to right.

Linear Graph Gradient of a linear graph example 2

Step-by-step guide: Gradient of a line

How to find the gradient from a linear graph

In order to find the gradient from a linear graph:

Select two points on the line that occur on the corners of two grid squares.
Sketch a right angle triangle and label the change in $\textbf{y}$ and the change in $\textbf{x}.$
Divide the change in $\textbf{y}$ by the change in $\textbf{x}$ to find $\textbf{m}.$

Explain how to find the gradient from a linear graph

Gradient of a linear graph examples

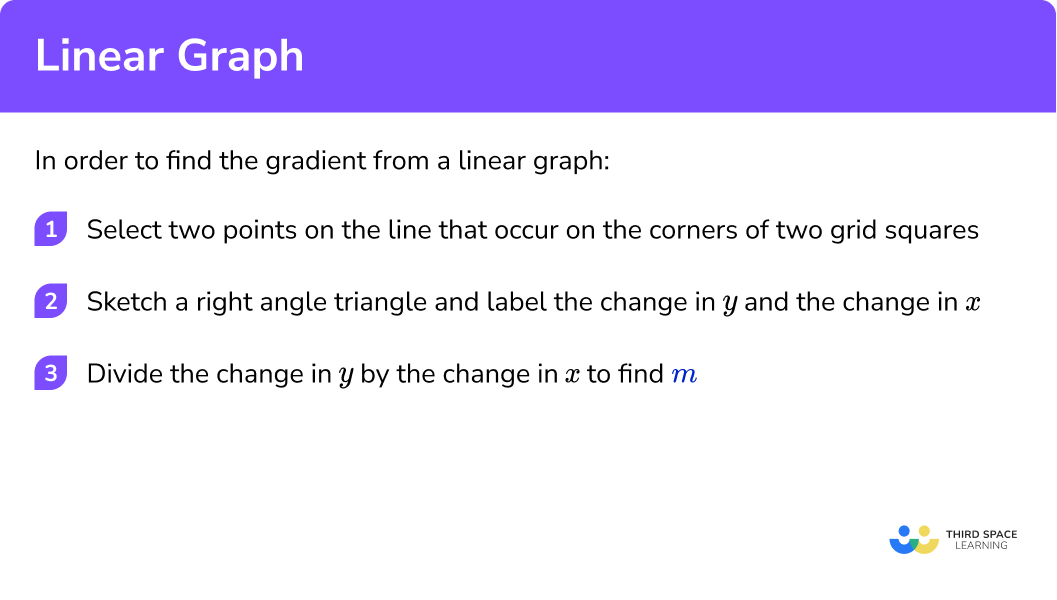
Example 4: gradient of a positive linear graph

Find the gradient of the linear graph below.

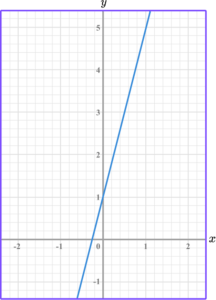
Select two points on the line that occur on the corners of two grid squares.

Find two coordinates, that are integers (whole numbers), and lie on the straight line.

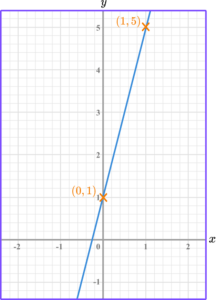
Sketch a right angle triangle and label the change in $\textbf{y}$ and the change in $\textbf{x}.$

The change in $y$ is $y_2-y_1=5-1=4.$

The change in $x$ is $x_2-x_1=1-0=1.$

Divide the change in $\textbf{y}$ by the change in $\textbf{x}$ to find $\textbf{m}.$

The formula for the gradient of a linear graph is

$\text { gradient }=\frac{y_2-y_1}{x_2-x_1}.$

So, we do the change in $y \ \div$ change in $x.$

For this linear graph, the gradient is $4 \div 1=4.$

Example 5: gradient of a negative linear graph

Find the gradient of the linear graph below.

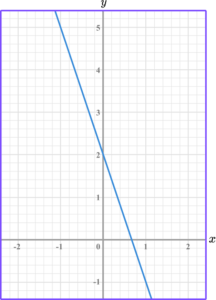
Select two points on the line that occur on the corners of two grid squares.

Find two coordinates, that are integers (whole numbers), and lie on the straight line.

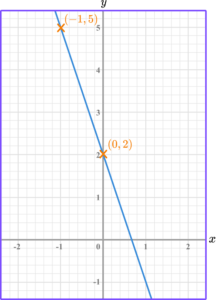
Sketch a right angle triangle and label the change in $\textbf{y}$ and the change in $\textbf{x}.$

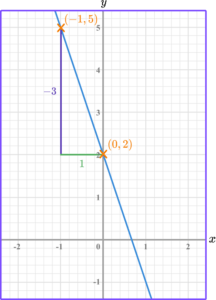
The change in $y$ is $y_2-y_1=2-5=-3.$

The change in $x$ is $x_2-x_1=0--1=1.$

Divide the change in $\textbf{y}$ by the change in $\textbf{x}$ to find $\textbf{m}.$

The formula for the gradient of a linear graph is

$\text { gradient }=\frac{y_2-y_1}{x_2-x_1}.$

So, we do the change in $y \ \div$ change in $x.$

For this linear graph, the gradient is $-3 \div 1=-3.$

Equation of a linear graph

A linear graph is a visual representation of a linear function. All linear graphs can be written as linear equations. These equations take the general form

y=mx+c.

Where $m$ is the gradient of the line and $c$ is the $\textbf{y}$ -intercept.

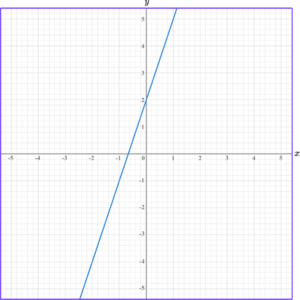
For example,

y=3x+2.

Here we can see that the gradient is $2,$ and the $y$ -intercept is at $+2.$

The graph of this equation would look like this,

Step-by-step guide: Equation of a line

How to find the equation of a linear graph

In order to find the equation of a linear graph:

Calculate the gradient of the line.
State the $\textbf{y}$ -intercept of the straight line.
Write the equation of the line in the form $\bf{y=mx+c.}$

Explain how to find the equation of a linear graph

Finding the equation of a linear graph examples

Example 6: finding the equation of a linear graph

Write the equation of the linear graph below in the form $y=mx+c.$

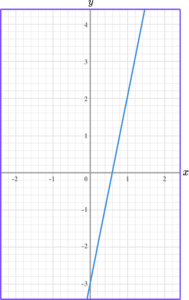
Calculate the gradient of the line.

The formula for the gradient of a linear graph is

$\text { gradient }=\frac{y_2-y_1}{x_2-x_1}$

So, we do the change in $y \ \div$ change in $x.$

For this linear graph, the gradient is $5 \div 1=5.$

State the $\textbf{y}$ -intercept of the straight line.

The $y$ -intercept is where the linear graph crosses the $y$ -axis.

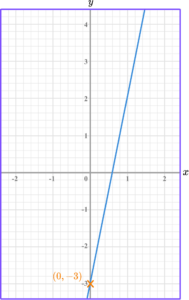
In this instance, the linear graph crosses the $y$ -axis at $-3.$

Write the equation of the line in the form $\bf{y=mx+c.}$

Equations of linear graphs take the general form $y=mx+c.$

Where $m$ is the gradient of the line and $c$ is the $y$ -intercept.

Hence, the equation of the line is $y=5x-3.$

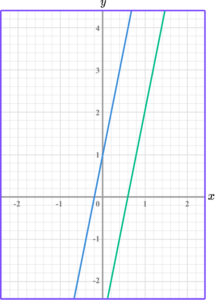
Parallel and perpendicular lines

Parallel lines are lines that travel in exactly the same direction, remaining equidistant from each other, therefore never meet. The gradients of parallel lines are equal.

For example,

Linear Graph Parallel and perpendicular lines example 1

Perpendicular lines are lines that intersect at $90 \degree$ to each other. The gradients of perpendicular lines are the negative reciprocals of each other. In other words, they multiply together and give a product of $-1.$

For example,

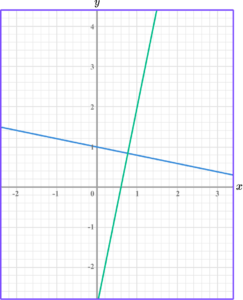
Linear Graph Parallel and perpendicular lines example 2

Step-by-step guide: Parallel and perpendicular lines

How to identify lines parallel or perpendicular to a linear graph

In order to identify lines that are parallel or perpendicular to a linear graph:

Calculate the gradient of both lines.
Compare the gradients.
State whether the lines are parallel, perpendicular or neither.

Explain how to identify lines parallel or perpendicular to a linear graph

Identifying parallel and perpendicular examples

Example 7: identifying parallel lines

Show that the linear graphs below are parallel.

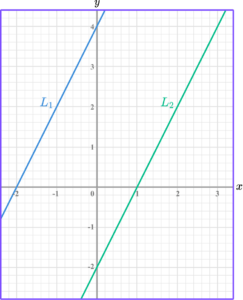
Calculate the gradient of both lines.

The gradient of $L_1$ is $4 \div 2=2.$

The gradient of $L_2$ is $4 \div 2=2.$

Compare the gradients.

Both lines have the same gradient, $2.$

State whether the lines are parallel, perpendicular or neither.

Hence, lines $L_1$ and $L_2$ are parallel.

Example 8: identifying perpendicular lines

Show that the linear graphs below are perpendicular.

Calculate the gradient of both lines.

The gradient of $L_1$ is $4 \div 1=4.$

The gradient of $L_2$ is $-1 \div 4=-\frac{1}{4}.$

Compare the gradients.

The gradients are not the same, so the lines are not parallel.

$4 \times -\frac{1}{4}=-1$

State whether the lines are parallel, perpendicular or neither.

Hence, lines $L_1$ and $L_2$ are perpendicular.

Common misconceptions

Confusing $\textbf{x}$ and $\textbf{y}$ coordinates

When plotting coordinates, they are in the form $(x, \ y).$

For example, the coordinate $(4, \ 3)$ would be plotted at the point where $4$ on the $x$ -axis and $3$ on the $y$ -axis intersect.

Recognising algebraic notation

When substituting values to generate the coordinates for plotting linear graphs, remember $3x = 3 \times x.$ Inversely, $\frac{x}{3}=x \div 3.$

Practice linear graph questions

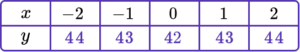
Linear Graph Practice question 1 a

Linear Graph Practice question 1 b

Linear Graph Practice question 1 c Correct Answer

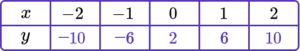
Linear Graph Practice question 1 d

$y=4x+2$ is the equation of the linear graph.

Substituting the $x$ values into the equation, generates the corresponding $y$ values.

\begin{aligned} &y=(4 \times -2)+2=-6 \\\\ &y=(4 \times -1)+2=-2 \\\\ &y=(4 \times 0)+2=2 \\\\ &y=(4 \times 1)+2=6 \\\\ &y=(4 \times 2)+2=10 \end{aligned}

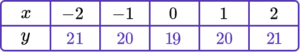
Linear graph practice question 2 a

Linear graph practice question 2 b Correct Answer

Linear graph practice question 2 c

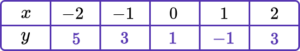
Linear graph practice question 2 d

$y=2x-1$ is the equation of the linear graph.

Substituting the $x$ values into the equation, generates the corresponding $y$ values.

\begin{aligned} &y=(2 \times -2)-1=-5\\\\ &y=(2 \times -1)-1=-3 \\\\ &y=(2 \times 0)-1=-1 \\\\ &y=(2 \times 1)-1=1 \\\\ &y=(2 \times 2)-1=3 \end{aligned}

\frac{1}{6}

4

\frac{1}{4}

6

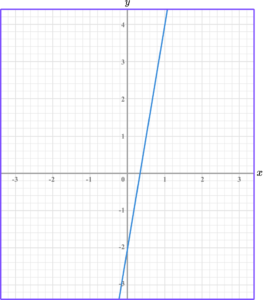
The change in $y$ is $y_2-y_1=4- -2=6.$

The change in $x$ is $x_2-x_1=1-0=1.$

The formula for the gradient of a linear graph is

\text { gradient }=\frac{y_2-y_1}{x_2-x_1}.

So, we do the change in $y \ \div$ change in $x.$

For this linear graph, the gradient is $6 \div 1=6.$

y=3x-4

y=-3x-4

y=4x-3

y=\frac{1}{3}x-4

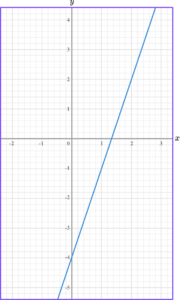
The $y$ -intercept is $-4.$

The gradient is $3.$

The equation of the linear graph is $y=3x-4.$

y=5x-2

y=2x+5

y=2x-5

y=5x+2

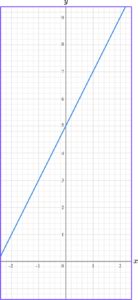
The $y$ -intercept is $5.$

The gradient is $2.$

The equation of the linear graph is $y=2x+5.$

y=6x+10

y=x+6

y=x-1

y=2x-1

Parallel lines have the same gradient. The gradient of the linear graph $y=6x-1$ is $6,$ so the equation which is parallel from the line is $y=6x+10.$

Linear graph GCSE questions

1. (a) Complete the table of values for $y=2x+5.$

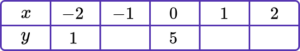
Linear graph GCSE question 1 a

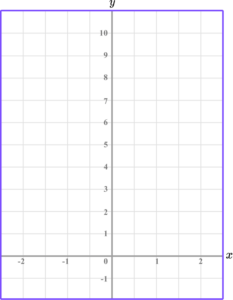
(b) On the grid, draw the graph of $y=2x+5$ for values of $x$ from $-2$ to $2.$

Linear graph GCSE question 1 b

(4 marks)

Show answer

(a)

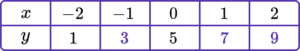
Linear graph GCSE question 1 a answer

$1$ correct value.

(1)

All $3$ correct values.

(1)

(b)

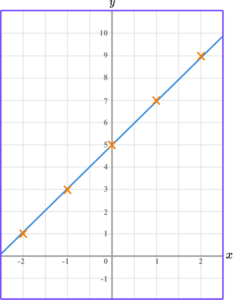
Linear graph GCSE question 1 b answer

All coordinates plotted correctly.

(1)

Straight line drawn connecting all coordinates.

(1)

2. Write the equation of the linear graph below in the form $y=mx+c.$

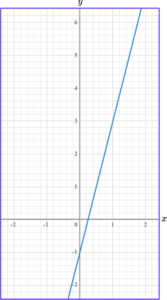
Linear graph GCSE question 2

(3 marks)

Show answer

Gradient identified as $4.$

(1)

$y$ -intercept identified as $-1.$

(1)

y=4x-1

(1)

3. Write down the equation of a line parallel to $y=3x+2.$

(1 mark)

Show answer

Any equation written in the form $y=mx+c$ starting $y=3x.$

(1)

Learning checklist

You have now learned how to:

Plot linear graphs

Identify the gradient of a linear graph

Find the equation of a linear graph

Identify parallel and perpendicular lines

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.