Congruent triangles

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

2D shapes Angles in a triangle Pythagoras’ theorem Coordinates maths

This topic is relevant for:

GCSE Maths Geometry and Measure Congruence And Similarity

Congruent Triangles

Here we will learn about congruent triangles, including how to identify them and prove the congruence of triangles.

There are also congruent triangles worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

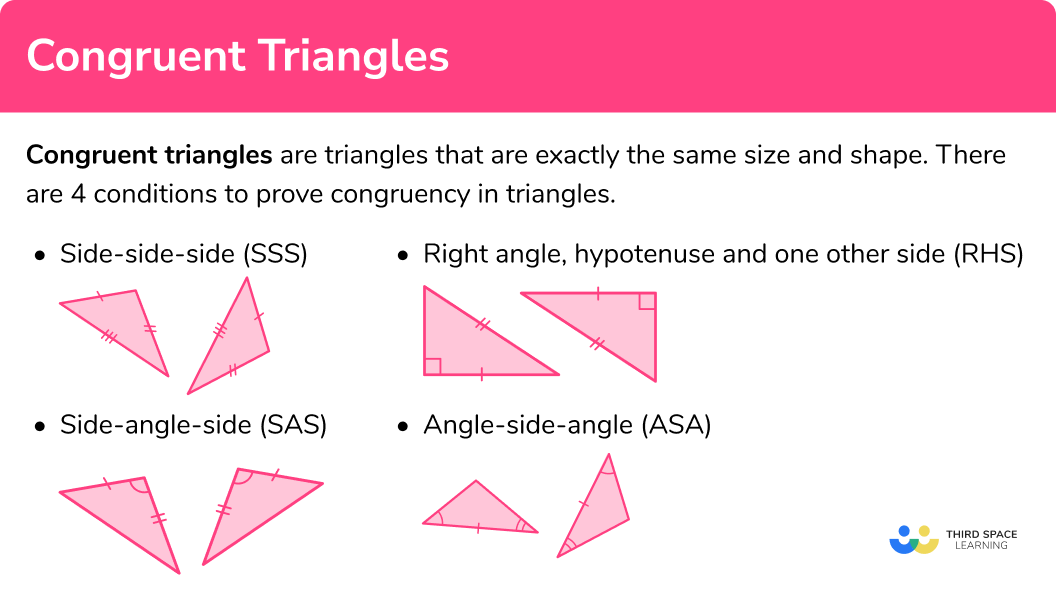
What are congruent triangles?

Congruent triangles are triangles that are exactly the same size and shape.

There are $4$ conditions to prove congruency in triangles.

See also: Congruent shapes

Side-side-side (SSS)

When two triangles have all three sides the same, they are congruent triangles.

The second triangle may be a rotation or a mirror image of the first triangle (or both).

Right angle, hypotenuse and one other side (RHS)

When two triangles are right-angled triangles and have the hypotenuse and one of the shorter sides the same, they are congruent triangles.

The second triangle may be a rotation or a mirror image of the first triangle (or both). The third side would also be identical and this can be checked using Pythagoras’ theorem.

Side-angle-side (SAS)

When two triangles have two sides and the included angle the same, they are congruent triangles. The included angle is the angle in between the two sides.

The second triangle may be a rotation or a mirror image of the first triangle (or both).

Angle-side-angle (ASA)

When two triangles have two angles and the included side the same, they are congruent triangles. The included side is the side in between the two angles.

The second triangle may be a rotation or a mirror image of the first triangle (or both).

This can also be known as angle-angle-side (AAS) as if two angles in a triangle are known, the third angle can be worked out using the angle fact that the sum of interior angles in a triangle is $180°$ .

What are congruent triangles?

Keywords

Congruent is used to describe shapes such as quadrilaterals or polygons which are exactly the same shape and the same size.

Similar is used to describe shapes such as quadrilaterals or polygons which are the same shape, but different sizes. A scale factor is involved.

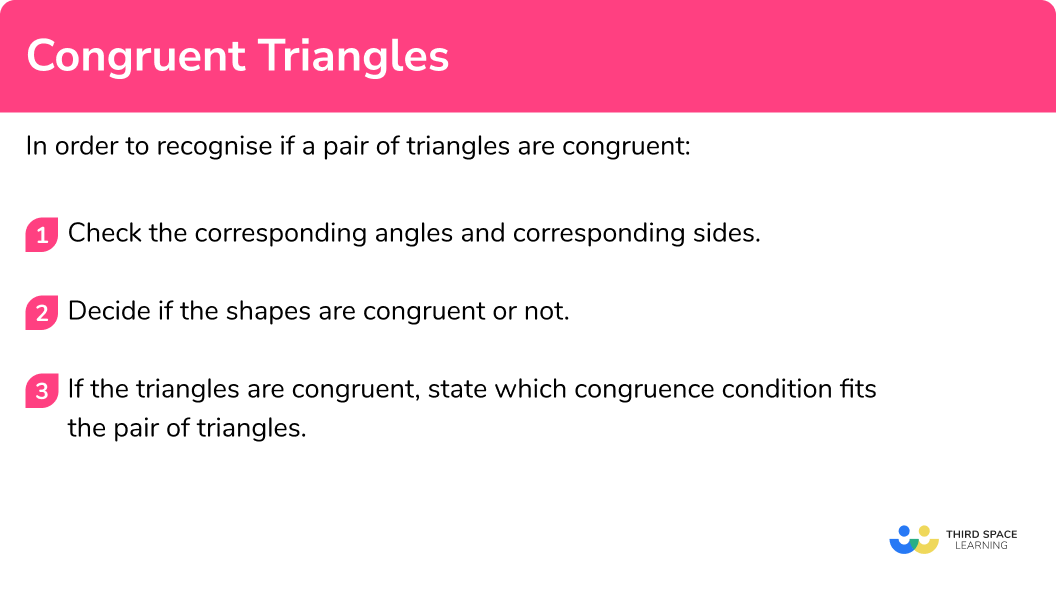
How to recognise congruent triangles

In order to recognise if a pair of triangles are congruent:

Check the corresponding angles and corresponding sides.
Decide if the shapes are congruent or not.
If the triangles are congruent, state which congruence condition fits the pair of triangles.

How to recognise congruent triangles

Congruent triangles worksheet

Get your free congruent triangles worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Congruent triangles worksheet

Get your free congruent triangles worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on congruence and similarity

Congruent triangles is part of our series of lessons to support revision on congruence and similarity. You may find it helpful to start with the main congruence and similarity lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Congruent triangles examples

Example 1: recognise congruent triangles

Decide whether this pair of triangles are congruent. If they are congruent, state why:

Check the corresponding angles and corresponding sides.

Both triangles have sides $5cm$ and $7cm$ . They both have an angle of $95°$ .

2Decide if the shapes are congruent or not.

The $95°$ angles are in a corresponding position. The triangles are mirror images of each other. The triangles are congruent.

3If the triangles are congruent, which congruence condition fits the pair of triangles.

The triangles are congruent with the condition side-angle-side (SAS).

Example 2: recognise congruent triangles

Decide whether this pair of triangles are congruent. If they are congruent, state why:

Check the corresponding angles and corresponding sides.

Both triangles have sides $6.3cm, 8.1cm$ and $10.2cm$ .

Decide if the shapes are congruent or not.

The triangles are the same shape and the same size – they are congruent.

If the triangles are congruent, which congruence condition fits the pair of triangles.

The triangles are congruent with the condition side-side-side (SSS).

Example 3: recognise congruent triangles

Decide whether this pair of triangles are congruent. If they are congruent, state why:

Check the corresponding angles and corresponding sides.

Both triangles have sides $8cm$ .

Both triangles have a $50°$ angle.

But their second angles are different.

Decide if the shapes are congruent or not.

The triangles look like they are different shapes BUT the third angle can be worked out.

Using the angle fact that the sum of interior angles of a triangle is $180°$ we can work out the missing angle in both triangles.

The $8cm$ side is in between the $30°$ and $50°$ angles in both triangles. The triangles are the same shape and the same size.

They are congruent triangles.

If the triangles are congruent, which congruence condition fits the pair of triangles.

The triangles are congruent with the condition angle-side-angle (ASA).

Example 4: recognise congruent triangles

Decide whether this pair of triangles are congruent. If they are congruent, state why:

Check the corresponding angles and corresponding sides.

Both triangles are right-angled triangles.

They both have a short side of $9cm$ .

But the hypotenuse of each triangle is different.

Decide if the shapes are congruent or not.

The triangles look like they are the same shape, but they are not. The triangles are not congruent.

If the triangles are congruent, which congruence condition fits the pair of triangles.

The triangles are not congruent.

The right angle, hypotenuse and one other side (RHS) condition was close to being satisfied, but not quite.

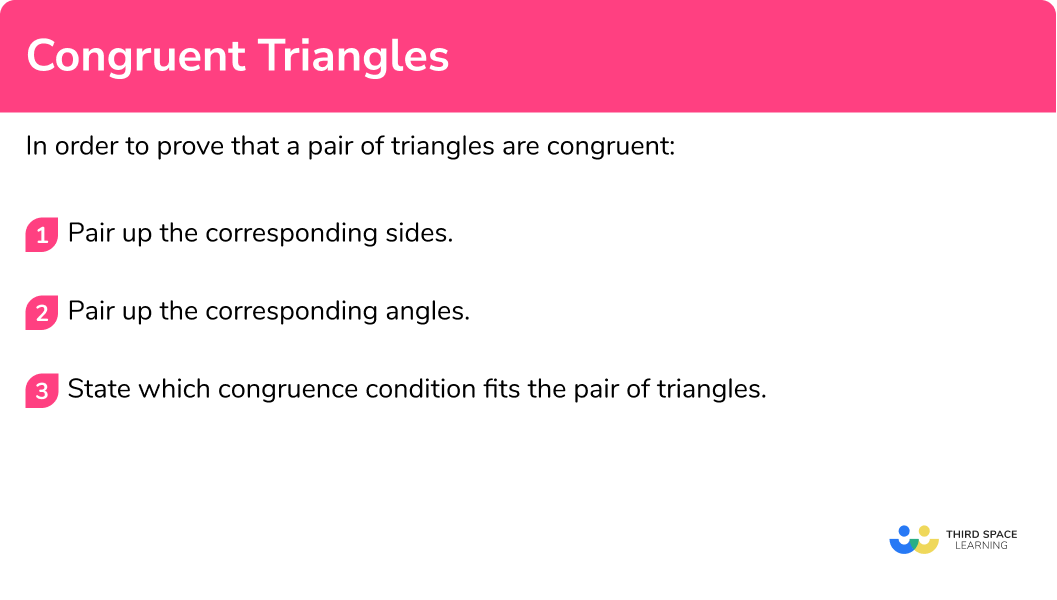
How to prove congruent triangles

In order to prove that a pair of triangles are congruent:

Pair up the corresponding sides.
Pair up the corresponding angles.
State which congruence condition fits the pair of triangles.

How to prove congruent triangles

Proving congruent triangles examples

Example 5: prove triangles are congruent

Prove that triangle $ABC$ is congruent to triangle $DEF$ .

Pair up the corresponding sides.

State which sides are identical, here there are two pairs of corresponding sides.

\[\begin{align*} AB &= EF \\ AC &= DE\\ \end{align*}\]

Pair up the corresponding angles.

State which angles are identical, here there is one pair of equal angles.

You need to use the correct notation.

$\text{angle} \ CAB = \text{angle} \ DEF$

State which congruence condition fits the pair of triangles.

Triangle $ABC$ is congruent to triangle $DEF$ because they fit the side-angle-side (SAS) condition.

Example 6: prove triangles are congruent

Prove that triangle $ABD$ is congruent to triangle $BCD$

Pair up the corresponding sides.

State which sides are identical, here there is one pair of corresponding sides. It is the common side.

$BD$ is common

Pair up the corresponding angles.

State which angles are identical, here there are two pairs of equal angles.

You need to use the correct notation.

\[\begin{align*} \text{angle} \ ABD &= \text{angle} \ BDC\\ \text{angle} \ ADB &= \text{angle} \ CBD\\ \end{align*}\]

State which congruence condition fits the pair of triangles.

Triangle $ABD$ is congruent to triangle $BCD$ because they fit the angle-side-angle (ASA) condition.

Common misconceptions

AAA – all three angles

AAA – all three angles being equal is not a condition for triangle congruence. These two triangles have identical angles, but the second triangle is an enlargement of the first triangle. They are similar triangles not congruent triangles.

E.g.

Remember – triangles can be congruent but rotations or mirror images

The second triangle may be a rotation or a mirror image of the first shape (or both). The triangle may still be congruent.

Use the correct notation

If we wanted to write about the $50°$ angle we shouldn’t just call it Angle $B$ .

It is much better to use the notation angle $ABC$ (or angle $CBA$ ).

For exam questions, check how many marks it is worth

Some exam questions ask you to explain why two triangles are congruent and are only worth one mark, here you only need to state the congruence condition (RHS, SSS, SAS or ASA).

If the exam question asks you to prove that two triangles are congruent and are worth several marks. You will need to match up the $3$ pairs of equal sides/angles and state the congruence condition.

Give details for proof questions (higher)

Some questions asking to prove that two triangles are congruent may need more explanations in the details. For example you may need to use an angle fact, such as, “alternate angles are equal”.

Practice congruent triangles questions

SAS

SSS

RHS

ASA

The triangles are right-angled triangles. They have the same hypotenuse and the same short side.

RHS

SSS

ASA

SAS

The triangles have two identical sides and an identical included angle.

RHS

SSS

ASA

SAS

The triangles have two identical angles and an identical included side.

RHS

SSS

SAS

ASA

The triangles have three identical sides.

SAS
Because
$\begin{aligned} AB = EF \\ AC = DF\\ \text{angle} \ CAB= \text{angle} \ DEF\\ \end{aligned}$

SAS
Because
$\begin{aligned} AB = EF \\ AC = DF\\ \text{angle} \ A= \text{angle} \ E\\ \end{aligned}$

SAS
Because
$\begin{aligned} AB = EF \\ BC = DE\\ \text{angle} \ CAB= \text{angle} \ DEF\\ \end{aligned}$

SAS
Because
$\begin{aligned} AB = EF \\ AC = DF\\ \text{angle} \ ABC= \text{angle} \ DEF\\ \end{aligned}$

The correct sides need to be paired up. The correct notation needs to be used for the angles.

SAS
Because
$\begin{aligned} AB = EF \\ \text{angle} \ CAB= \text{angle} \ DFE\\ \text{angle} \ BAC= \text{angle} \ DEF\\ \end{aligned}$

ASA
Because
$\begin{aligned} AB = EF \\ \text{angle} \ CAB= \text{angle} \ DFE\\ \text{angle} \ CBA= \text{angle} \ DEF\\ \end{aligned}$

ASA
Because
$\begin{aligned} AB = DE \\ \text{angle} \ CAB= \text{angle} \ EFD\\ \text{angle} \ CBA= \text{angle} \ DEF\\ \end{aligned}$

ASA
Because
$\begin{aligned} AB = EF \\ \text{angle} \ A= \text{angle} \ F\\ \text{angle} \ B= \text{angle} \ E\\ \end{aligned}$

The correct sides need to be paired up. The correct notation needs to be used for the angles.

Congruent triangles GCSE questions

1. Explain why these two triangles are congruent.

(1 mark)

Show answer

Side-angle-side
(correct answer only)

(1)

2. Prove that triangle $ABC$ is congruent to triangle $DEF$ .

(4 marks)

Show answer

Angle $EDF = 43^{\circ}$
For finding the angle $EDF$

(1)

$AC=DE$
For matching up the identical sides

(1)

$\begin{aligned} \text{angle} \ BAC= \text{angle} \ DEF\\ \text{angle} \ BCA= \text{angle} \ EDF\\ \end{aligned}$
For matching up the identical angles

(1)

Triangle $ABC$ is congruent to Triangle $DEF$ because angle-side-angle (ASA)
For giving the correct condition

(1)

3. $ABCD$ is a rectangle.
$AC$ is the diagonal of the rectangle.
Prove triangle $ABC$ is congruent to triangle $ACD$ .

(4 marks)

Show answer

Angle $ABC$ = angle $ADC = 90^{\circ}$
For identifying the right angles

(1)

The hypotenuse is AC and is common to both triangles
For identifying the hypotenuse

(1)

Side $AD$ = side $BC$ (as opposite sides of a rectangle are equal)
For identifying a pair of equal sides

(1)

Triangle $ABC$ is congruent to Triangle $DEF$ because right angle, hypotenuse, side (RHS)
For identifying the right angles

(1)

Learning checklist

You have now learned how to:

Identify congruent triangles and the condition of congruence

Prove two triangles are congruent

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.