Enlargement

Here we will learn about enlargement, including how to enlarge a 2D shape by a scale factor and how to describe an enlargement in detail.

There are also enlargement worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is enlargement?

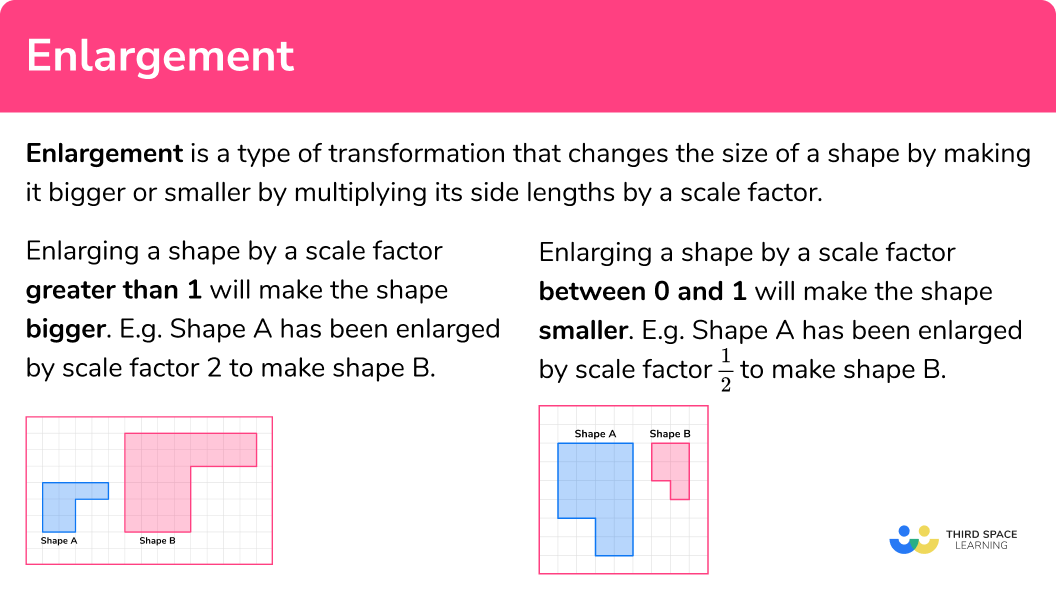
Enlargement is a type of transformation that changes the size of a shape by making it bigger or smaller by multiplying its side lengths by a scale factor.

Enlargements have real life functions, such as changing the size of photographic prints or pictures in documents.

• Enlarging a shape by a scale factor greater than 1 will make the shape bigger.

E.g.

Shape A has been enlarged by scale factor 2 to make shape B. 

The corresponding angles are identical but each side in shape B is double the size of the original shape.

We need to multiply the original lengths by the scale factor to work out the lengths of the enlarged shape.

• Enlarging a shape by a scale factor between 0 and 1 will make the shape smaller.
  
  This is often written as a fraction and is known as a fractional scale factor.

E.g.

Shape A has been enlarged by scale factor \frac{1}{2} to make shape B.

The corresponding angles are identical but each side in shape B is half the size of the original shape.

The object is the name of the original shape. The image is the name of the shape after it has been translated. When an object is enlarged the object and the image are similar shapes.

What is enlargement?

Centre of enlargement

The centre of enlargement places the enlargement in a specific place.

To use a centre of enlargement we need to draw straight lines from the centre of enlargement through the vertices of the original shape. These are called ray lines.

E.g.

Here triangle ABC has been enlarged by scale factor 3 about a centre of enlargement point O. 

The new triangle is labelled A’B’C’. 

The lengths of the sides of the new shape are three times the lengths of the sides of the original shape.

The pairs of corresponding sides are parallel lines. 

The angles in the two shapes are the same. 

The triangles are similar triangles.

Step-by-step guide: Centre of enlargement

Step-by-step guide: Scale factor

How to use enlargement

Points to consider:

1. The side lengths of the shape are enlarged depending on the scale factor
2. To work out the length scale factor – compare corresponding sides
3. If there is not a centre of enlargement, the enlarged shape can be drawn anywhere on the grid
4. If there is a centre of enlargement, ray lines can help to make sure the enlarged shape in the correct position on the grid

Enlargement maths examples

Example 1: use a scale factor to enlarge a shape

Enlarge the shaded shape by scale factor 2 .

Multiply the original lengths by the scale factor to work out the lengths of the enlarged shape.  It is easier to start with horizontal or vertical lines.

Step-by-step guide: Scale factor (coming soon)

Example 2: calculating a scale factor

Shape A has been enlarged to make shape B. Calculate the scale factor.

To calculate the scale factor we need to divide an enlarged length by the corresponding original length.

Example 3: with a centre of enlargement on a grid 

Enlarge the shaded shape with scale factor 3 about the point.

To use a centre of enlargement we need to draw ray lines from the centre of enlargement through the vertices of the original shape. Use the ray lines to help you enlarge the shape.

Step-by-step guide: Centre of enlargement

Example 4: with a centre of enlargement on a coordinate grid 

Enlarge the shaded shape by scale factor 2 about the point (1,2)

Plot the centre of enlargement on the coordinate grid. Then draw ray lines from the centre of enlargement through the vertices of the original shape. Use the ray lines to help you enlarge the shape.

Example 5: find a centre of enlargement

Shape A has been enlarged to make shape B. Find the centre of enlargement.

Find pairs of corresponding vertices and draw ray lines going through the points.

Example 6: negative scale factor (higher)

Enlarge the shaded shape with scale factor -2 about the point.

There are also negative scale factors in the higher GCSE only.  These are an extension of positive scale factors.

Draw ray lines from the centre of enlargement through the vertices of the original shape. Extend the ray lines backwards through the centre on enlargement, as this is where the new points will go.

Common misconceptions

• Accuracy

Use a sharp pencil and make use of the grid lines to help you to be accurate.

• Diagonal lines

Diagonal lines can be tricky to enlarge, so it is best to use horizontal and vertical lines.

• The origin

The origin of a coordinate grid has the coordinates (0,0) .  It is commonly denoted as O.   It is used often as the centre of enlargement.

• Describe a single transformation

If you are asked to give a single transformation – make sure it is a single transformation, not 2 or more.  Also make sure that you state the type of transformation and give full details.

E.g.

For enlargements state scale factor and the coordinates of the centre of enlargement.

The next lessons are

• Pythagoras’ theorem
• Trigonometry
• Loci and construction
• Standard form

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.