High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Addition and subtractionCoordinate grid
Integers Magnitude of a vectorAdding vectors
Here you will learn about adding vectors, including what it is and how to do it.
Students will first learn about adding vectors as part of the number system in high school.
What is adding vectors?
Adding vectors is adding one vector to another vector. This is sometimes known as a vector sum.
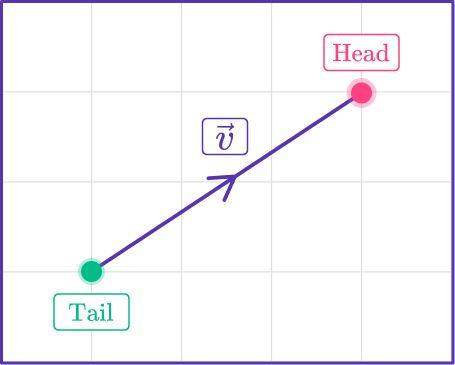
As vectors can be located anywhere in a space, the start of the vector is called the tail, and the end of the vector is called the head. The direction of the vector is therefore from the tail to the head and is indicated using an arrow through the middle of the vector.
Here, the arrow of vector \vec{v}=\langle{3,2}\rangle points from the tail to the head of the vector.
![[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Algebra-check-for-understanding-quiz-listing-image-.png)
[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)
![[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Algebra-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 8 students’ understanding of algebra. 10+ questions with answers covering a range of 6th and 8th grade algebra topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Algebra-check-for-understanding-quiz-listing-image-.png)
[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)
![[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Algebra-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 8 students’ understanding of algebra. 10+ questions with answers covering a range of 6th and 8th grade algebra topics to identify areas of strength and support!
DOWNLOAD FREELet’s look at some examples of vectors.
For the addition of vectors, \vec{a}+\vec{b}, place the tail of vector \vec{b} onto the head of vector \vec{a}.

Adding the vectors forms the new vector \vec{a}+\vec{b}. This is drawn as a straight line from the tail of \vec{a} to the head of \vec{b}.
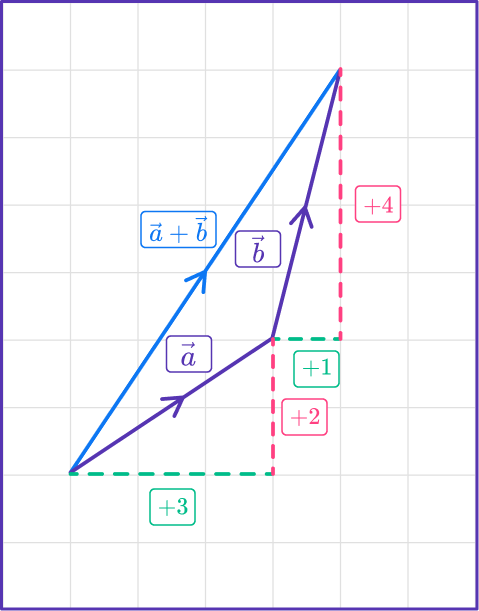
Now you can identify the components of the new vector. Notice they form the base and height of a right triangle, in which the vector is the hypotenuse.
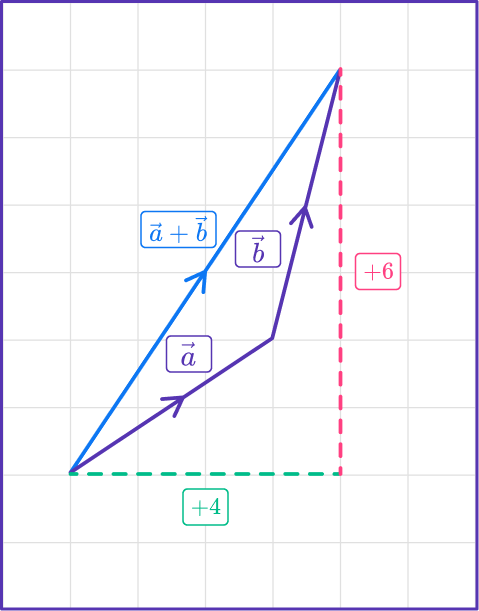
Here, the horizontal x component of the vector \vec{a}+\vec{b} is 4 right and the vertical y component is 6 up. So \vec{a}+\vec{b}=\langle{4,6}\rangle.
Though it can be helpful, when finding a vector sum you do not have to draw a diagram. You can just add the vector components. First, add the x components (first numbers) and then add the y components (second numbers).
\begin{aligned}\vec{a}+\vec{b}&=\langle{3,2}\rangle+\langle{1,4\rangle} \\\\ &=\langle{3+1,2+4}\rangle \\\\ &=\langle{4,6}\rangle \end{aligned}The new vector that results from adding two given vectors is known as the resultant vector.
The addition of vectors is commutative which means that the order in which you add the vectors is not important. In other words we will get the same result if you add the second vector to the first vector.
\vec{a}+\vec{b}=\vec{b}+\vec{a}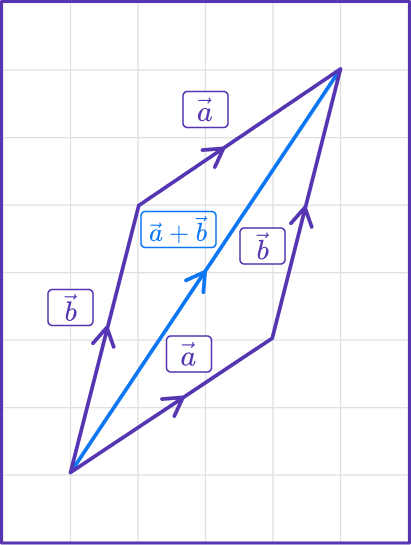
The diagram is a parallelogram. The resultant vector is the diagonal of the parallelogram.
Note: This page covers only 2 -dimensional vectors.
What is adding vectors?
Common Core State Standards
How does this relate to high school math?
- The Number System (HSN.VM.B.4a)
Add vectors end-to-end, component-wise, and by the parallelogram rule. Understand that the magnitude of a sum of two vectors is typically not the sum of the magnitudes.
- The Number System (HSN.VM.B.4b)
Given two vectors in magnitude and direction form, determine the magnitude and direction of their sum.
How to add vectors
In order to add vectors:
- Add the \textbf{x} components.
- Add the \textbf{y} components.
- Write the resultant vector.
Adding vectors examples
Example 1: add vectors graphically
Add the given vectors, \langle{2,4}\rangle+\langle{5,1}\rangle, shown on the coordinate plate.
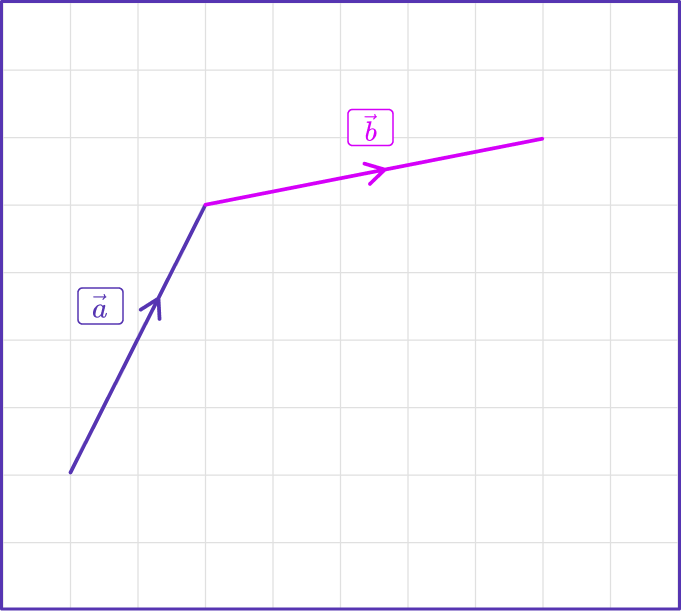
Notice the direction of the vectors is positive.
- Add the \textbf{x} components.
Connect the tail of vector \vec{a} to the head of vector \vec{b} to show the resultant vector \vec{a}+\vec{b}.
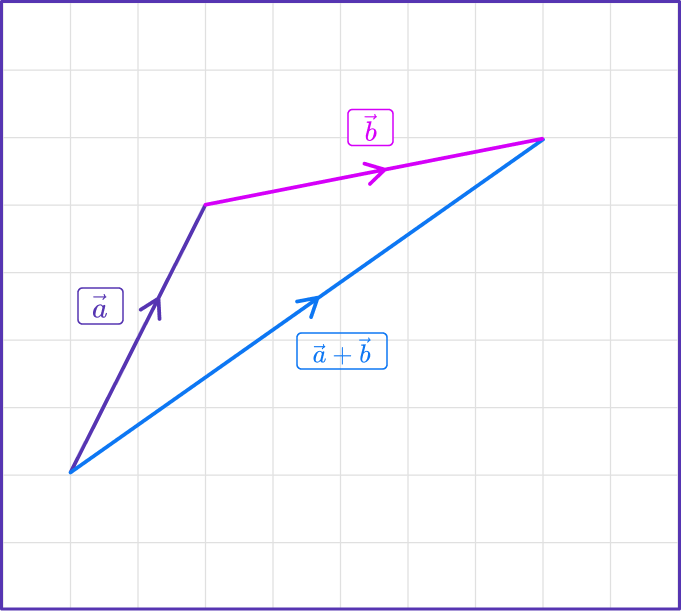
Look at the horizontal change from the tail of the first vector to the head of the second vector.

The horizontal x component is 7 right, or 7.
2Add the \textbf{y} components.
Look at the vertical change from the tail of the first vector to the head of the second vector.
The vertical y component is 5 up, or 5.
3Write the resultant vector.
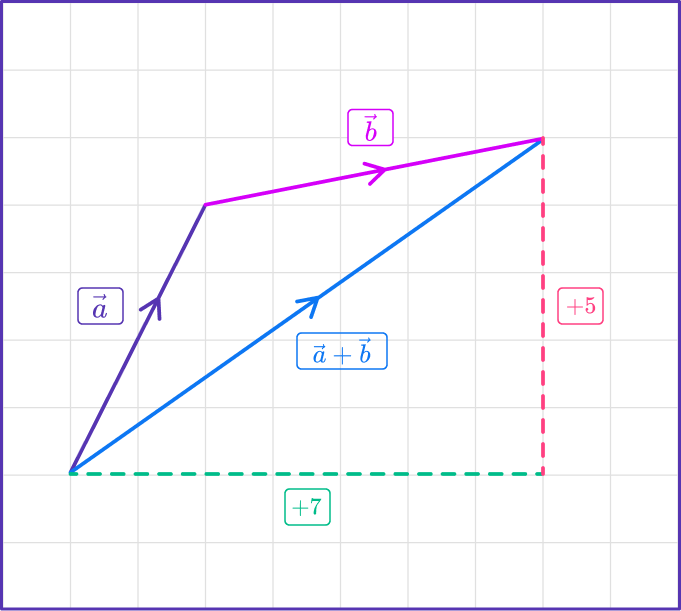
\langle{7,5}\rangleExample 2: add vectors graphically
Write the resultant vector \vec{a}+\vec{b} in the form \langle{x,y}\rangle.
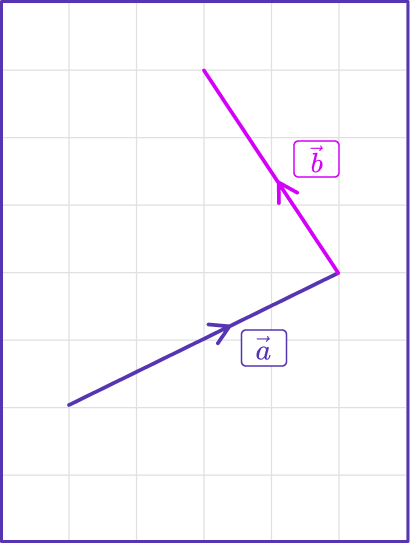
Add the \textbf{x} components.
Draw a straight line connecting the tail of \vec{a} to the head of \vec{b} and draw an arrow to show the direction of the vector \vec{a}+\vec{b}.
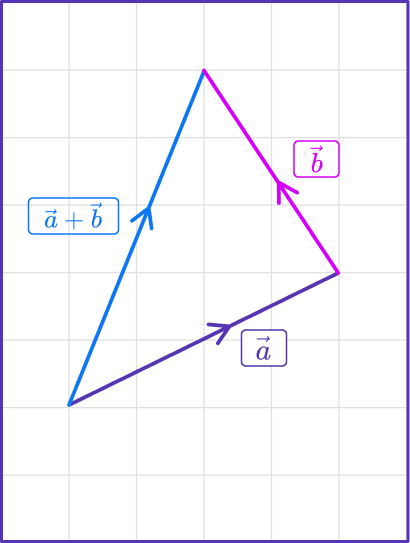
Look at the horizontal change from the tail of the first vector to the head of the second vector.
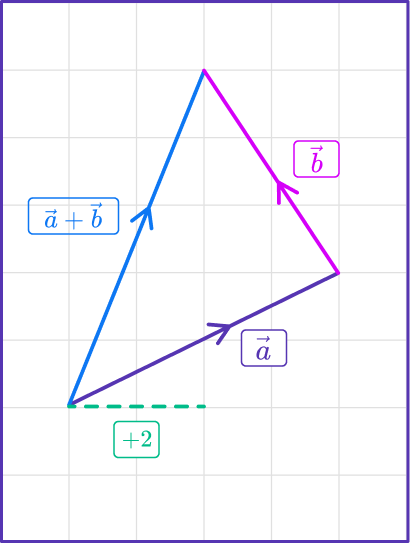
Add the \textbf{y} components.
Look at the vertical change from the tail of the first vector to the head of the second vector.
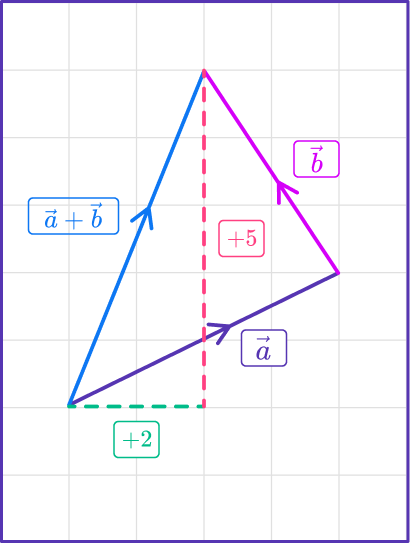
Write the resultant vector.
Example 3: add three vectors
Add the given vectors: \langle{8,2}\rangle+\langle{7,1}\rangle+\langle{3,0}\rangle
Add the \textbf{x} components.
Add the first numbers of the original vectors:
8+7+3=18
Add the \textbf{y} components.
Add the second numbers of the original vectors:
2+1+0=3
Write the resultant vector.
Write the two answers in the component form of a vector:
\langle{8,2}\rangle+\langle{7,1}\rangle+\langle{3,0}\rangle=\langle{18,3}\rangle
Example 4: add vectors including negative numbers
Add the given vectors: \langle{- \, 5,- \, 2}\rangle+\langle{2,- \, 4}\rangle
Add the \textbf{x} components.
Add the first numbers of the original vectors:
- \, 5+2=- \, 3
Add the \textbf{y} components.
Add the second numbers of the original vectors:
- \, 2+(- \, 4)=- \, 2-4=- \,6
Write the resultant vector.
Write the two answers in the component form of a vector:
\langle{- \, 5,- \, 2}\rangle+\langle{2,- \, 4}\rangle=\langle{- \, 3,- \, 6}\rangle
Example 5: add vectors including a scalar
Let \vec{a}=\langle{7,3}\rangle and \vec{b}=\langle{- \, 3,- \, 4}\rangle. Calculate 2\vec{a}+\vec{b} writing your answer in the component form of the vector.
Add the \textbf{x} components.
The vector 2\vec{a} is the same as \vec{a}+\vec{a} so 2\vec{a}+\vec{b} is the same as \vec{a}+\vec{a}+\vec{b}.
Add the first numbers of the original vectors:
7+7+(- \, 3)=14-3=11
Add the \textbf{y} components.
Add the second numbers of the original vectors:
3+(- \, 4)+(- \, 4)=3-4-4=- \, 5
Write the resultant vector.
Write the two answers in the component form of the vector:
2\vec{a}+\vec{b}=\langle{11,- \, 5}\rangle
Example 6: add vectors including 0
Let \vec{a}=\langle{- \, 3,- \, 1}\rangle,~\vec{b}=\langle{- \, 2,4}\rangle and \vec{c}=\langle{5,- \, 3}\rangle. Find \vec{a}+\vec{b}+\vec{c}. Write your answer in the component form.
Add the \textbf{x} components.
Add the first numbers of the original vectors:
- \, 3+(- \, 2)+5=0
Add the \textbf{y} components.
Add the second numbers of the original vectors:
- \, 1+4-3=0
Write the resultant vector.
Write the two answers in the component form of the vector:
\langle{- \, 3,- \, 1}\rangle+\langle{- \, 2,4}\rangle+\langle{5,- \, 3}\rangle=\langle{0,0}\rangle
Teaching tips for adding vectors
- Instead of jumping to the rule, let students explore adding vectors using the head-to-tail method on coordinate system graphs. This will help them understand why the rule for adding vectors work and in turn help them have a deeper understanding of vectors.
- Make sure that students understand the vocabulary for the components of a vector. Terms such as tail (starting point), head (end point), magnitude and direction should be clearly defined for students.
Easy mistakes to make
- Incorrectly adding negative integers
Mistakes can easily be made when adding a positive number and a negative number. Make sure that students’ negative numbers skills are well practiced before teaching vectors. For example, 3+(- \, 4)=3-4=- \, 1
- Corresponding components with the wrong axes
The horizontal components are related to the x -axis and the vertical components y -axis. Show students how this connects to their knowledge of the coordinate grid.
- Confusing scalars, vectors and matrices
Since this is an introductory topic, students may easily confuse terms. Clearly give examples of each and correct students when they use these terms incorrectly.
Related vectors lessons
- Component form of a vector
- Subtracting vectors
- Vector multiplication
- Vector practice problems
Practice adding vectors questions
1. Add the given vectors, \vec{a}=\langle{3,3}\rangle and \vec{b}=\langle{5,3}\rangle, shown on the coordinate plane.
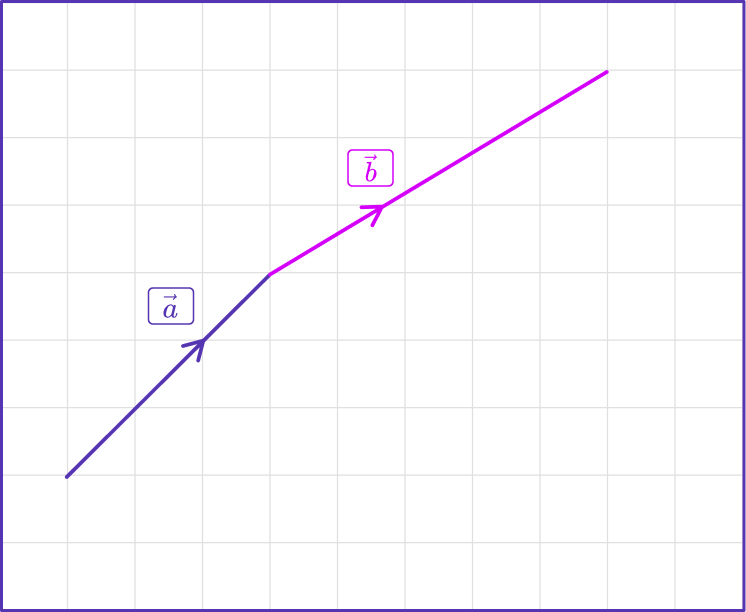




Adding the vectors forms the new vector \vec{a}+\vec{b}.

Calculate the horizontal and vertical component of the vector by counting squares:
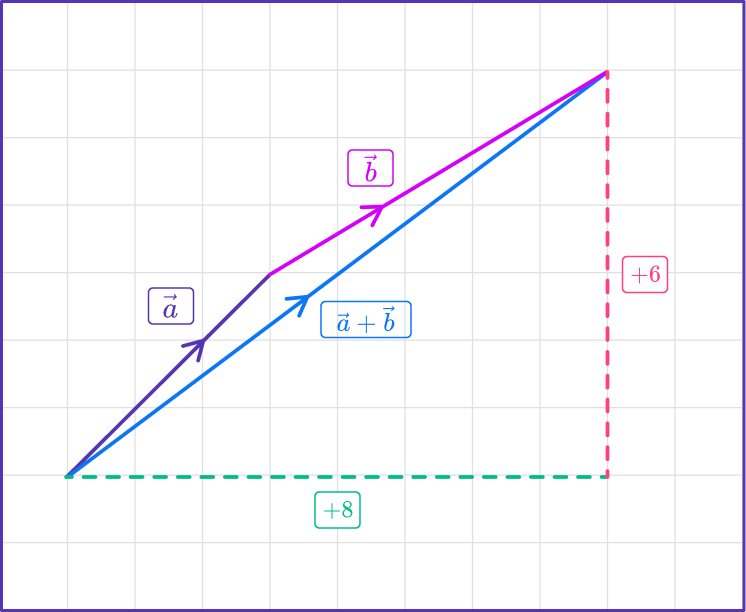
The resultant vector is \langle{8,6}\rangle.
2. Find the resultant vector of \langle{5,1}\rangle+\langle{3,6}\rangle, shown on the coordinate plane. Write your answer in the component form of the vector.
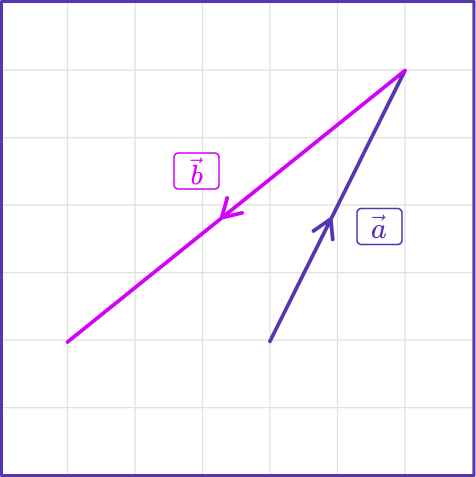




Connect the tail of \vec{a} to the head of \vec{b}.
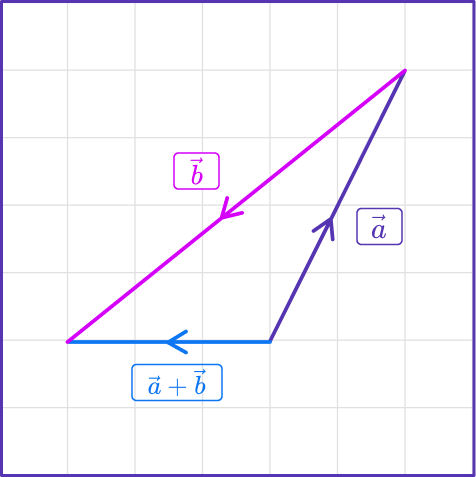
Look at the horizontal and vertical change in vector \vec{a}+\vec{b}.
The horizontal component is – \, 3.
The vertical component is 0.
The resultant vector is \langle{- \, 3,0}\rangle.
3. Add the given vectors, \langle{0,4}\rangle+\langle{- \, 4,0}\rangle, shown on the coordinate plane.





Adding the vectors forms the new vector \vec{a}+\vec{b}.
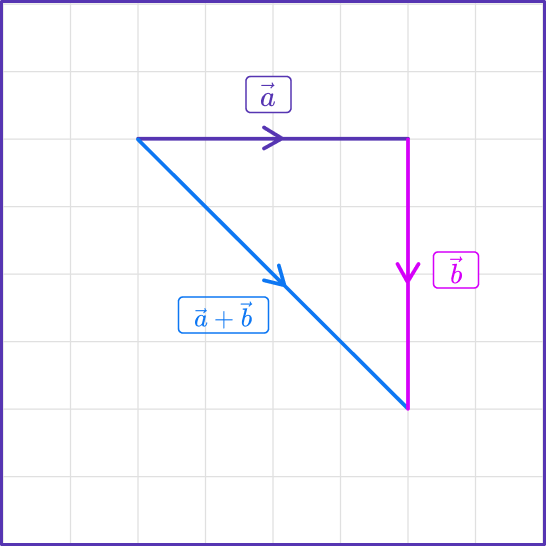
Look at the horizontal and vertical change from the tail of the first vector to the head of the second vector.
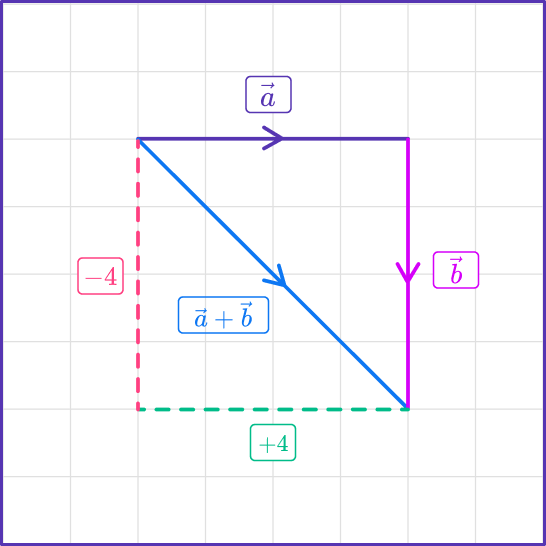
The horizontal component is 4.
The vertical component is – \, 4.
The resultant vector is \langle{4,- \, 4}\rangle.
4. Add vector \vec{r} and vector \vec{s}.
\vec{r}=\langle{3,2}\rangle
\vec{s}=\langle{1,5}\rangle
Write the resultant vector in the component form.




5. Calculate the resultant vector
\langle{4,5}\rangle+\langle{1,- \, 3}\rangle+\langle{- \, 2,1}\rangle




6. Let \vec{a}=\langle{1,4}\rangle and \vec{b}=\langle{2,- \, 3}\rangle. Calculate \vec{a}+2\vec{b}.




Adding vectors FAQs
Subtracting vectors is similar, but instead of adding the components, you subtract them.
Derived from the Pythagorean theorem, you square the components of the vector and then take their square root.
Yes, though the examples on this page only show the sum of two vectors, any number of vectors can be added.
Vectors that have a magnitude of 1.
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!