High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Parts of a circle Types of angles Straight angle 2D shapesInterior and exterior angles of polygons
Congruence and similarity
Tangent of a circle
Here you will learn about the circle theorems involving tangents of a circle, including their application, proof, and using them to solve more difficult problems.
Students will first learn about the tangent of a circle as part of geometry in high school.
What is the tangent of a circle?
A tangent of a circle is a straight line that touches the circumference of the circle at a single point. This point is called the point of tangency.
- Diagram 1 – The angle between a tangent and radius is 90 degrees.
- Diagram 2 – Tangents which meet at the same point are equal in length.
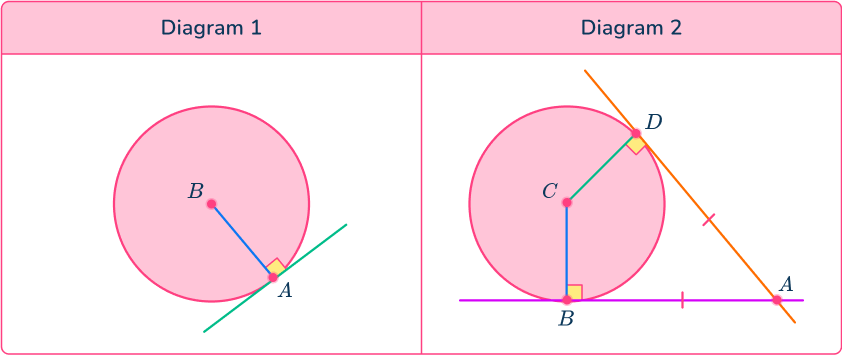
In diagram 1 above, the tangent meets the circle at point A (the point of tangency), which is perpendicular to the radius of the circle at that point .
In diagram 2, two tangents meet the circle at two different points (B and D) and they intersect at point A.
If the points B and D are linked by a chord, AB and AD are the same length, so ABD is an isosceles triangle.
If the points B and D join to the center of the circle C, they form a kite ABCD. This means that you have the two circle theorems:
Isosceles triangle

Kite
![[FREE] Common Core Practice Tests (Grades 3 to 6)](https://thirdspacelearning.com/wp-content/uploads/2023/10/common-core-practice-tests.png)
[FREE] Common Core Practice Tests (Grades 3 to 6)
![[FREE] Common Core Practice Tests (Grades 3 to 6)](https://thirdspacelearning.com/wp-content/uploads/2023/10/common-core-practice-tests.png)
Prepare for math tests in your state with these Grade 3 to Grade 6 practice assessments for Common Core and state equivalents. 40 multiple choice questions and detailed answers to support test prep, created by US math experts covering a range of topics!
DOWNLOAD FREE![[FREE] Common Core Practice Tests (Grades 3 to 6)](https://thirdspacelearning.com/wp-content/uploads/2023/10/common-core-practice-tests.png)
[FREE] Common Core Practice Tests (Grades 3 to 6)
![[FREE] Common Core Practice Tests (Grades 3 to 6)](https://thirdspacelearning.com/wp-content/uploads/2023/10/common-core-practice-tests.png)
Prepare for math tests in your state with these Grade 3 to Grade 6 practice assessments for Common Core and state equivalents. 40 multiple choice questions and detailed answers to support test prep, created by US math experts covering a range of topics!
DOWNLOAD FREEKey parts of a circle needed for these theorems
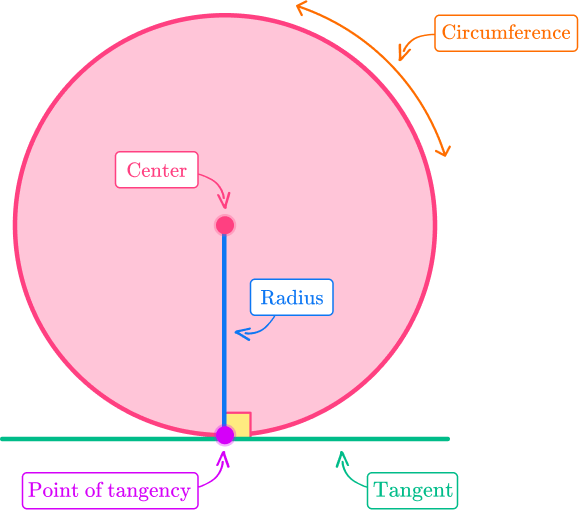
- The radius of a circle is the distance from the center to the circumference of the circle. The radius is half of the diameter.
- The center of the circle is a point that is located in the middle of a circle.
- The circumference of the circle is the distance around the edge of the circle.
Proving that if two tangents meet, they are the same length
To be able to prove this theorem, you do not need to know any other circle theorem. You just need to be confident with angles in a triangle. You also need to understand congruence.

What is the tangent of a circle?

Common Core State Standards
How does this relate to high school math?
- High school: Geometry – Circles (HS.G.C.A.4)
Construct a tangent line from a point outside a given circle to the circle.
How to use the tangent theorems
In order to use the tangent of a circle:
- Locate the key parts of the circle for the theorem.
- Use other angle facts to determine the remaining angle(s) made with the tangent.
- Use the tangent theorem to state the other missing angle.
Tangent of a circle examples
Example 1: standard diagram
Points A, B, and C are on the circumference of a circle with point O as the center. ( This can also be called circle O.) \, DE is a tangent at point A. Calculate the size of angle BAD.

- Locate the key parts of the circle for the theorem.
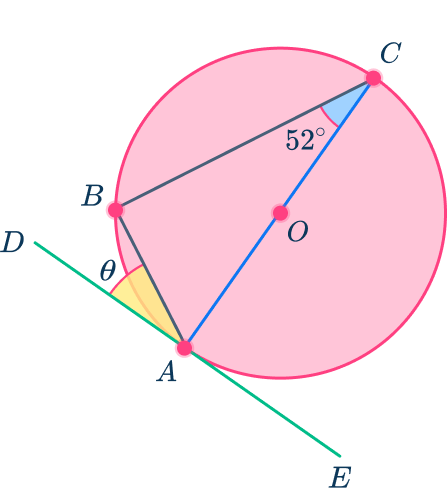
Here you have:
- Angle BCA=52^{\circ}
- AC is the diameter
- DE is a tangent
- Angle BAD=\theta
2Use other angle facts to determine the remaining angle(s) made with the tangent.
As AC is a diameter and the angle in a semicircle is 90^{\circ}, angle ABC=90^{\circ}. As angles in a triangle total 180^{\circ},
\begin{aligned} \text{ Angle } CAB&=180-(90+52) \\\\ &=38^{\circ} \end{aligned}
3Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle BAD\text{:}
\begin{aligned}BAD&=90-38 \\\\ &=52^{\circ} \end{aligned}Example 2: angles in the same segment
A, B, C, and D are points on the circumference of a circle with center O. \, AC and BD intersect at point G. \, EF is a tangent at point C and is parallel to BD. Calculate the size of angle BCF.
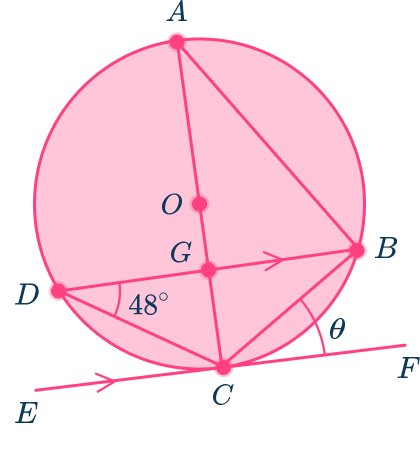
Locate the key parts of the circle for the theorem.
Here you have:
- Angle BDC=48^{\circ}
- AC is the diameter
- EF is a tangent
- Angle BCF=\theta
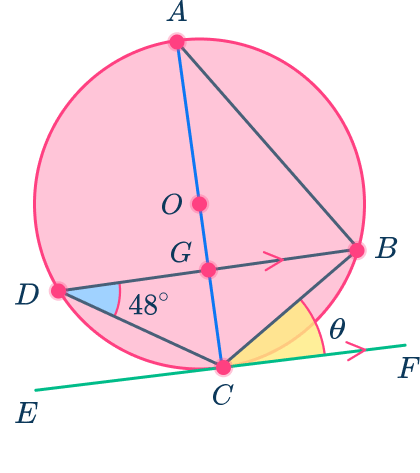
Use other angle facts to determine the remaining angle(s) made with the tangent.
Angles in the same segment are equal, so angle BDC= angle BAC=48^{\circ}. As AC is a diameter and angles in a semicircle are 90^{\circ}, angle ABC=90^{\circ}.
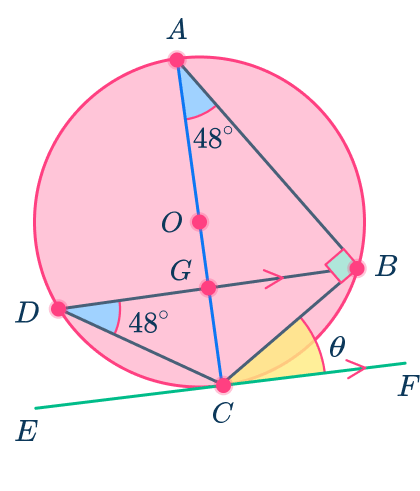
Angles in a triangle total 180^{\circ} so you can now calculate angle ACB\text{:}
\begin{aligned}ACB&=180-(90+48) \\\\ &=42^{\circ} \end{aligned}
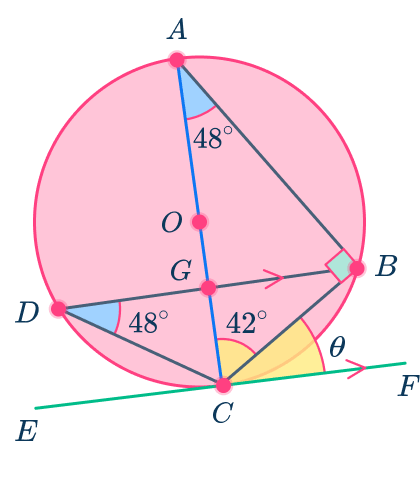
Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle BCF\text{:}
Example 3: angles at the center
A circle with center O has three points on the circumference, A, B, and C. The tangent DE passes through point C. Calculate the size of angle BCE.

Locate the key parts of the circle for the theorem.
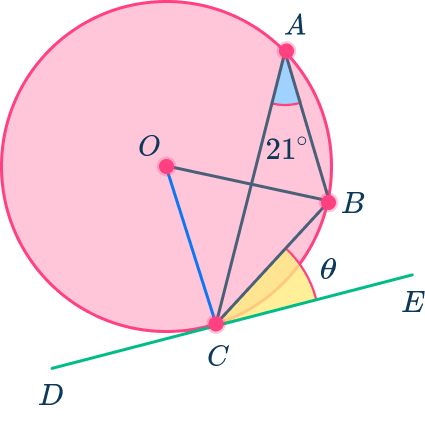
Here you have:
- Angle BAC=21^{\circ}
- OC is the radius
- DE is a tangent
- Angle BCE=\theta
Use other angle facts to determine the remaining angle(s) made with the tangent.
The angle at the center is twice the angle at the circumference and so angle BOC=21\times{2}=42^{\circ}. Triangle OBC is isosceles because OB and OC are radii.
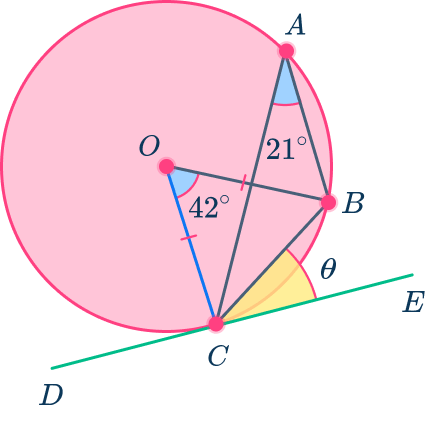
As OBC is an isosceles triangle, you can calculate the size of angle OCB\text{:}
\begin{aligned}OCB&=(180-42)\div{2} \\\\ &=69^{\circ} \end{aligned}
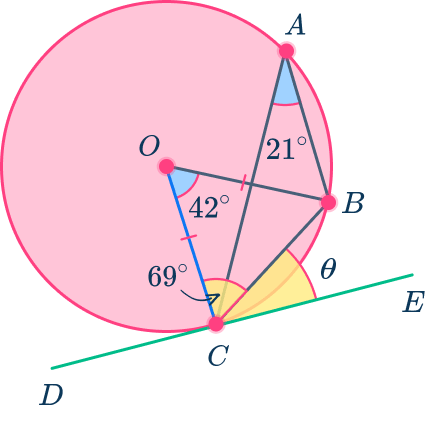
Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle BCE\text{:}
Example 4: tangent of a circle
B, C, and D are points on the circumference of a circle with center O. \, AE and AF are tangents to the circle. Calculate the size of angle DBF.
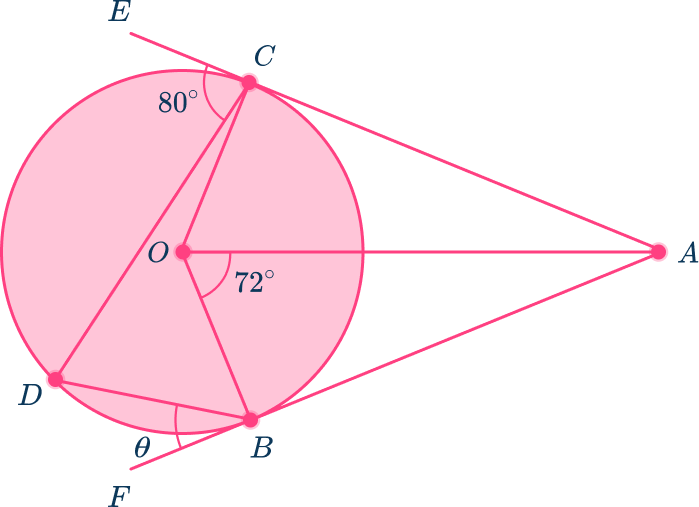
Locate the key parts of the circle for the theorem.
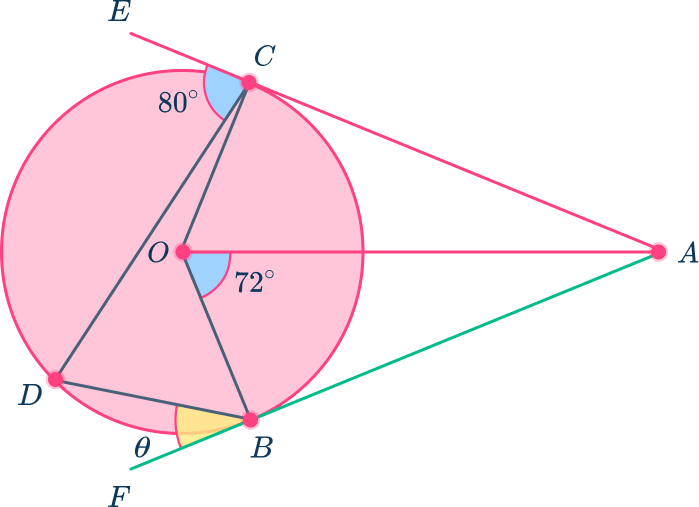
Here you have:
- Angle DCE=80^{\circ}
- OB is the radius
- AF is a tangent
- Angle OBD=\theta
Use other angle facts to determine the remaining angle(s) made with the tangent.
You need to find a way of calculating angle OBD as this angle added to \theta is equal to 90^{\circ}. \, COBD is a quadrilateral, so if you can calculate all of the angles within this quadrilateral, you can then find angle DBF.
ABOC is a kite because tangents that meet at the same point are equal and the two other sides OB and OC are radii, so they are the same length.
The line OA bisects the angle BOC and so you can state that the angle COA=72^{\circ} as it is the same size as angle AOB.
As both tangents meet the radius at 90 degrees, angles ACO and ABO are also equal to 90^{\circ}.
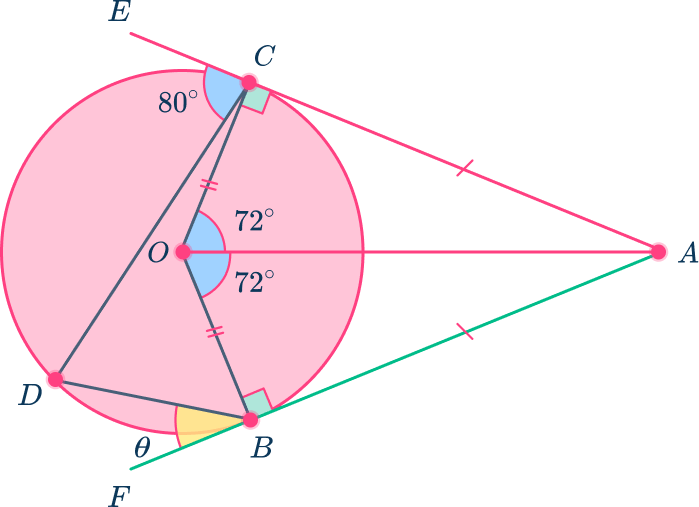
The angle at the center is twice the angle at the circumference and so as the angle at the center would equal 144^{\circ} \, (72+72=144^{\circ}), the angle BDC is half this angle and so angle BDC=72^{\circ}.
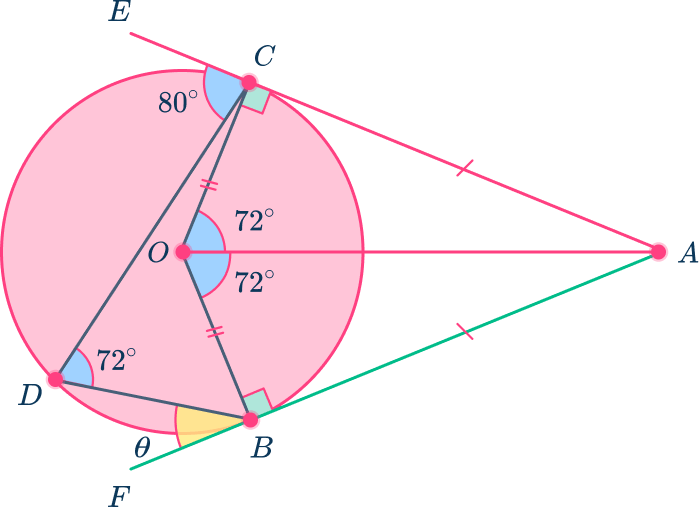
You can also calculate the size of the angle OCE because the angle between the tangent and the radius is 90^{\circ}. This means angle OCD=90-80=10^{\circ}.
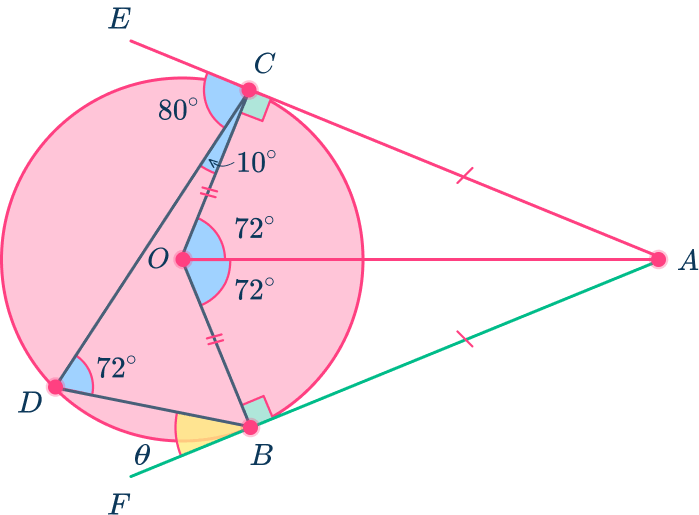
As angles around a point total 360^{\circ}, you can say that the reflex angle BOC is equal to:
As angles in a quadrilateral total 360^{\circ}, you can calculate the size of angle OBD as COBD is a quadrilateral.
\begin{aligned}OBD&=360-(10+216+72) \\\\ &=62^{\circ} \end{aligned}
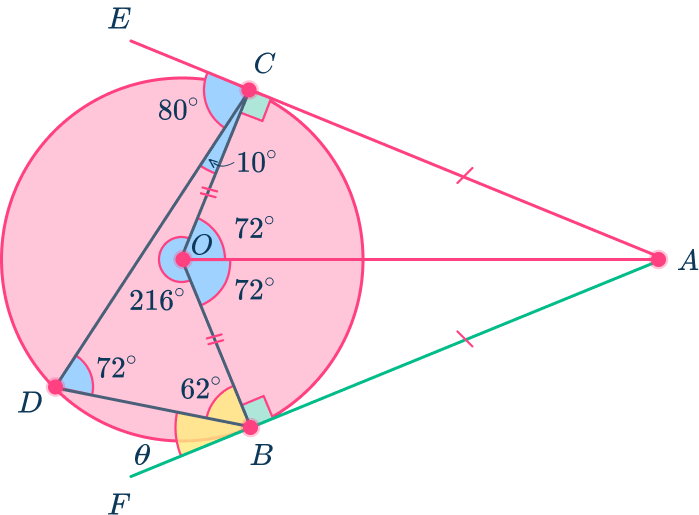
Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle DBF\text{:}
Example 5: alternate segment theorem
The triangle ABC is inscribed in a circle with center O. \, DE is a tangent at point A. Calculate the size of the angle OAC.
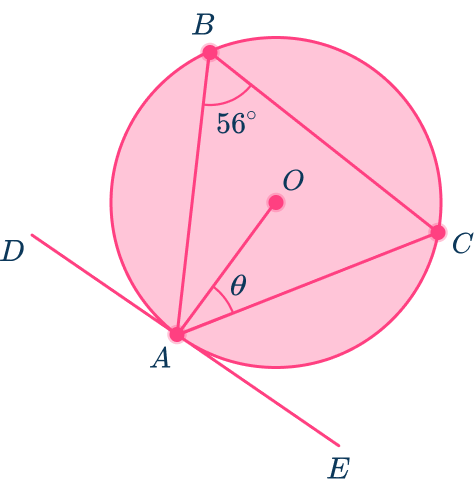
Locate the key parts of the circle for the theorem.

Here you have:
- Angle ABC=56^{\circ}
- OA is a radius
- DE is a tangent
- The angle OAC=\theta
Use other angle facts to determine the remaining angle(s) made with the tangent.
The alternate segment theorem states that the angle made with the tangent and a chord is equal to the angle in the alternate segment. This means that the angle CAE is equal to the angle ABC, so CAE=56^{\circ}.
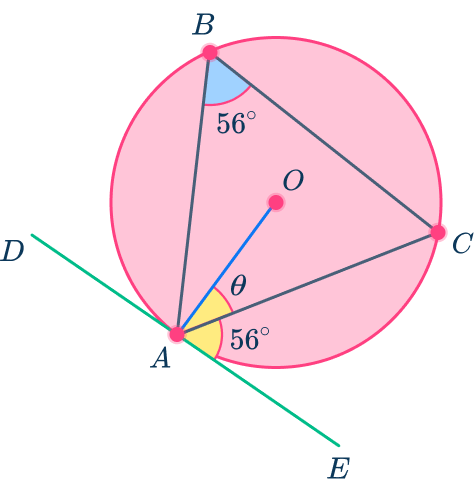
Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle OAC\text{:}
Example 6: complex diagram
ABCD is an arrowhead inscribed inside a circle with center C. The two tangents EF and GH meet at external point P. Calculate the size of angle FPG.

Locate the key parts of the circle for the theorem.
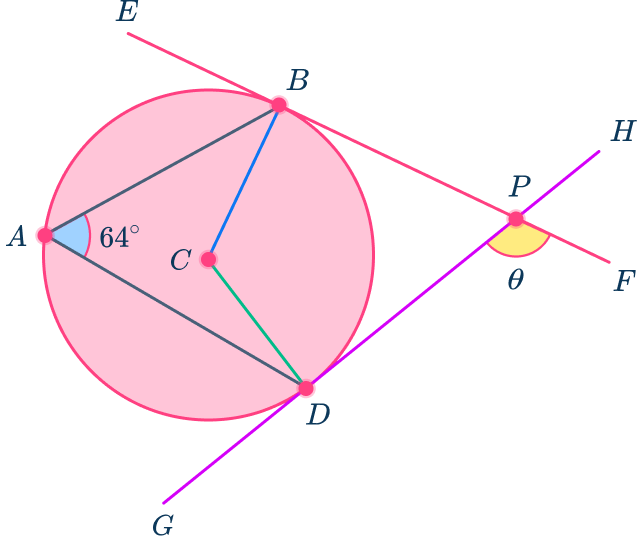
Here you have:
- Angle BAD=64^{\circ}
- BC and CD are radii
- EF and GH are tangents
- Angle FPG=\theta
Use other angle facts to determine the remaining angle(s) made with the tangent.
The angle at the center is twice the angle at the circumference so the angle BCD is twice the size of angle BAD. \, BCD=64\times{2}=128^{\circ}.
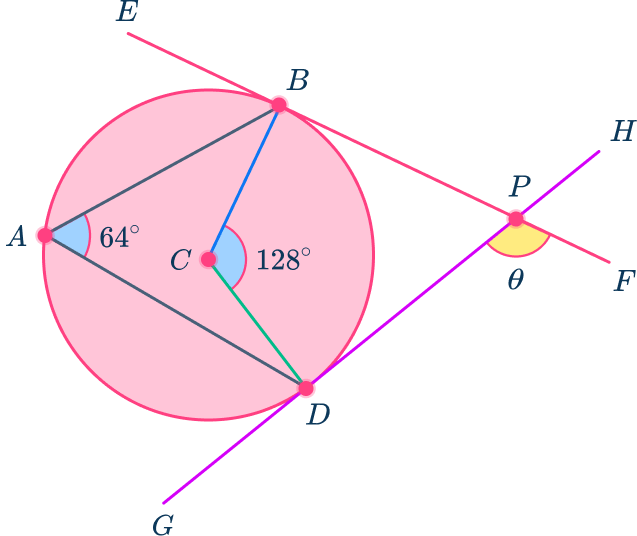
Use the tangent theorem to state the other missing angle.
As the angle between the tangent and the radius is 90^{\circ}, you can now calculate angle BPD which is on a straight line with FPG\text{:}
As angles on a straight line total 180^{\circ},
Teaching tips for tangent of a circle
- Provide worksheets with real-world examples where tangents are encountered, such as the point where a road meets a circular roundabout or the point where a billiard ball strikes the cushion of a table. Relating abstract concepts to tangible situations enhances understanding.
- Start with simple practice questions and gradually increase complexity. This approach enhances critical thinking and problem-solving skills.
- Highlight connections between tangents and other mathematical concepts, such as the Pythagorean theorem (Pythagoras’ theorem), similar triangles, and trigonometry.
Easy mistakes to make
- Misrepresenting the angle between the tangent and the radius
Either through miscalculation or an assumption, the angle between the tangent and the radius is not 90^{\circ} because “it doesn’t look like it”, it must be proven.
- Misusing the alternate segment theorem
There are cases when the Alternate Segment Theorem is used to describe the angle at the tangent or the angle in the alternate segment at the circumference but neither is true.
Take Example 5 above. The angle OAC is assumed to be equal to 56^{\circ} whereas angle CAE is equal to 56^{\circ}.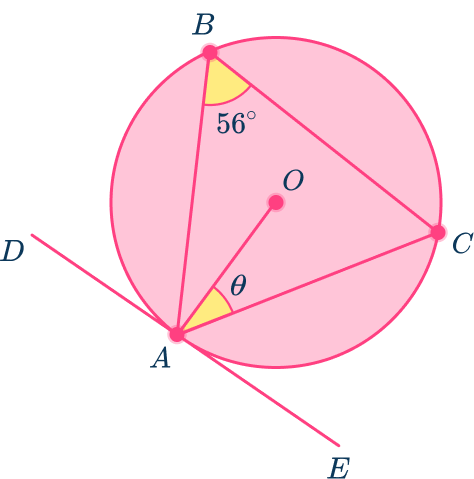
- Thinking the angle is double or half the opposing angle
The kite that is formed when two tangents meet has two angles of 90^{\circ} and 90^{\circ} because they meet the radius at 90^{\circ}.
The other two angles are assumed to be double or half whereas they should total 180^{\circ} (it is a unique case for the cyclic quadrilateral).
In the example below, the angle COB is correctly equal to 144^{\circ} as 72\times{2}=144^{\circ}. The angle CAB is incorrectly equal to 144\div{2}=72^{\circ}.
This is incorrect because angles in a quadrilateral should total 360^{\circ}, whereas shape ABOC has a total of 90+90+144+72=396^{\circ}. The only case when this is correct is when ABOC is a square.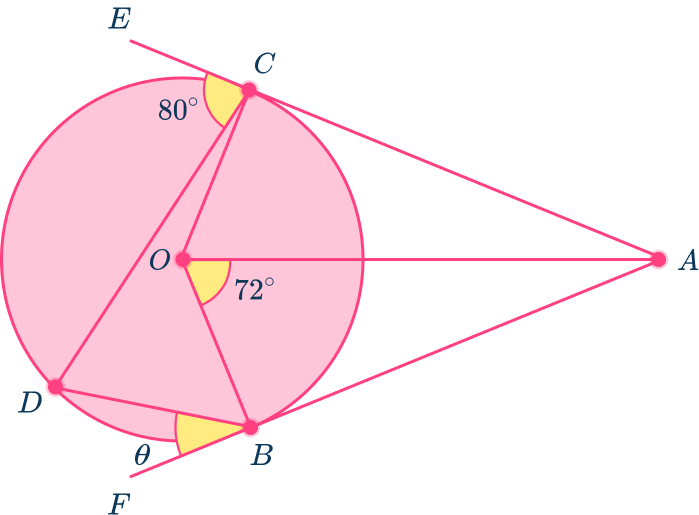
Related circle theorems lessons
- Circle theorems
- Central angle
- Chords of a circle
- Subtended
- Circle chord theorems
Practice tangent of a circle questions
1. The right triangle ABC is inscribed in the circle with center O. The line DE is a tangent of the circle with the point of contact at A. Angle BAD=78^{\circ}. Calculate the size of the angle ACB.





- BAC=90-78=12^{\circ} \, ( the tangent meets the radius at 90^{\circ})
- ABC=90^{\circ} \, ( angles in a semicircle are 90^{\circ})
- ACB=180-(90+12)=78^{\circ} \, ( angles in a triangle sum to 180^{\circ})
2. A, B, C, and D are points on the circumference of a circle with center O. \, AC and BD are endpoints of two perpendicular lines with AC passing through the center of the circle. EF is a tangent to the circle at point C. Calculate the size of the angle BCF.





- DBC=68^{\circ} \, (BD is parallel to EF as BD and AC are perpendicular)
- BGC=90^{\circ} \, ( chord of a triangle)
- BCG=180-(90+68)=22^{\circ}
- 90-22=68^{\circ} \, ( the tangent meets the radius at 90^{\circ})
3. The triangle ABC is inscribed into a circle with center O. The line DE is a tangent at the point C. The angle BCE= \theta. Calculate the size of angle BCE.
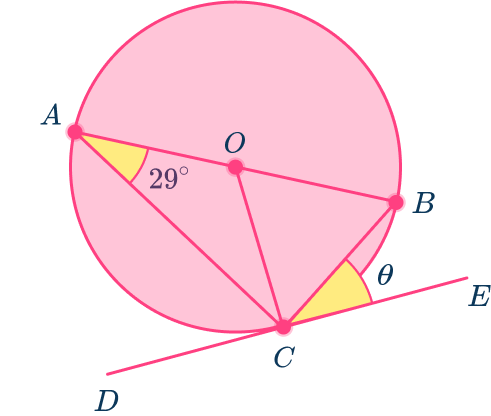




- OCA=29^{\circ} \, ( triangle OAC is isosceles as OA=OC)
- ABC=90^{\circ} \, ( angles in a semicircle)
- OCB=90-29=61^{\circ}
- BCE=90-61=29^{\circ} \, ( the tangent meets the radius at 90^{\circ})
4. A circle with center O has three points on the circumference, B, C, and D. The lines AE and AF are tangents to the circle at points B and C. The tangents meet at the point A. Angle BAC=40^{\circ}. Calculate the size of the angle ODC.
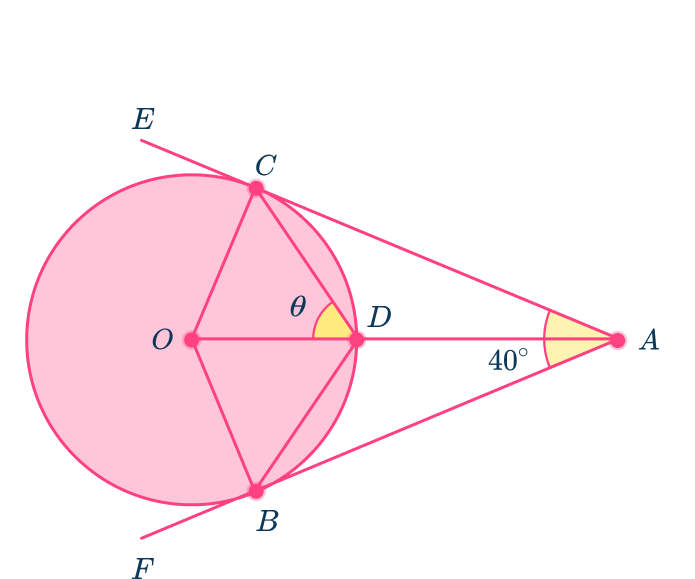




- OAC=40\div{2}=20^{\circ} \, (OA bisects the angle BAC)
- ACO=90^{\circ} \, ( angles in a semicircle)
- AOC=180-(90+20)=70^{\circ} \, ( angles in a triangle)
- OCD is an isosceles triangle as OC=OD
- ODC=(180-70)\div{2}=55^{\circ} \, ( angles in an isosceles triangle)
5. A circle with center O has three points on the circumference, A, B, and C. The line DE is a tangent to the circle at the point A. Angle BAD=78^{\circ}. Calculate the size of the angle ABC.
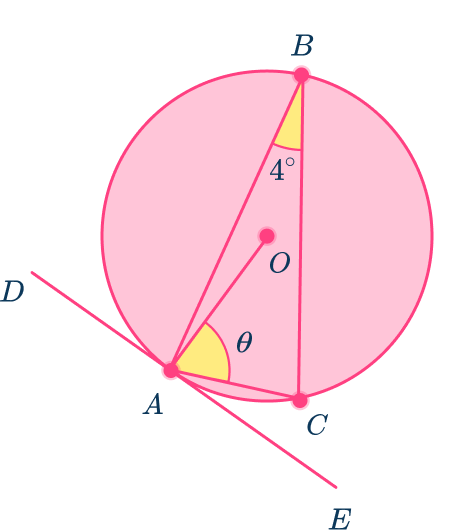




- EAC=4^{\circ} \, ( alternate segment theorem)
- OAC=90^{\circ} \, ( angles in a semicircle are 90^{\circ})
- OAC=90-4=86^{\circ}
6. A, B, and D are points on the circle with center C. The tangents EF and GH intersect at the point P at an angle of 112^{\circ}. Calculate the size of angle BAD.
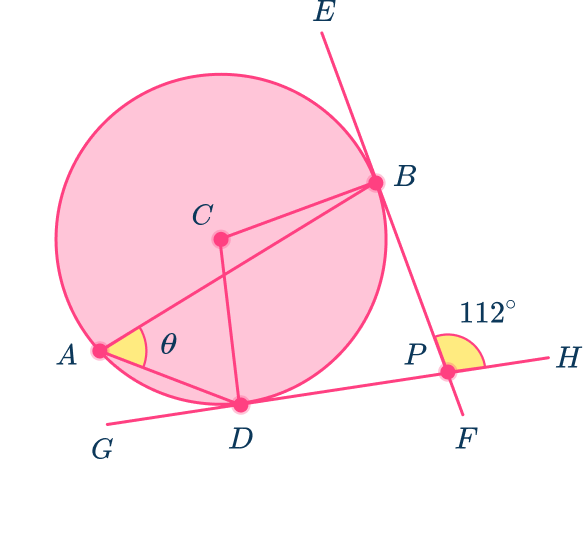




- BPD=180-112=68^{\circ} \, ( angles on a straight line total 180^{\circ})
- CDP=CBP=90^{\circ} \, ( the tangent meets the radius at 90^{\circ})
- BCD=360-(90+90+68)=112^{\circ} \, ( angles in a quadrilateral total 360^{\circ})
- BAD=112\div{2}=56^{\circ} \, ( angle at the center is twice the angle at the circumference)
Tangent of a circle FAQs
A tangent to a circle is a straight line that touches the circle at exactly one point, without crossing it. This single point where the circle touches the line is called the point of tangency.
The Tangent-Secant Theorem states that if a tangent and a secant are drawn from a point outside a circle, then the square of the length of the tangent segment is equal to the product of the lengths of the whole secant segment and its external segment.
If you know the distance from the point to the center of the circle (d) and the radius of the circle (r), the length of the tangent segment can be found using the Pythagorean theorem: Length of tangent =\sqrt{d^2-r^2}
To find the point of tangency, you need the coordinates of the point and the equation of the circle. The point of tangency will satisfy both the equation of the circle and the equation of the tangent line.
A tangent is a straight line that touches a circle at exactly one point. A chord is a line segment with both endpoints on the circumference of the circle.
The next lessons are
- Prism shape
- Types of data
- Averages and range
- Equation of a circle
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!