High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Adding and subtracting fractions Multiplying and dividing fractions Substitution Rearranging equationsChanging the subject
SOHCAHTOA The Pythagorean TheoremTrig identities
Here you will learn about trigonometric identities, including recognizing and working with key trigonometric identities, as well as applying algebraic skills to simplify the identities.
Students first learn how to work with trigonometric identities in Algebra II and expand that knowledge through Precalculus.
What are trigonometric identities?
Trigonometric identities are mathematical equations that involve trigonometric functions, like sine, cosine, and tangent and they are true for all values of the variables involved.
An identity is an equation that is always true.
For example, when expanding the algebraic expression 2(x+1) using the distributive property you get 2 x+2. These two functions of x are equivalent so you can write the statement: 2(x+1) \equiv 2 x+2.
The same is true for trigonometric ratios and expressions.
Let’s take a look at several equivalent expressions that are trigonometric identities.
Using the right triangle:
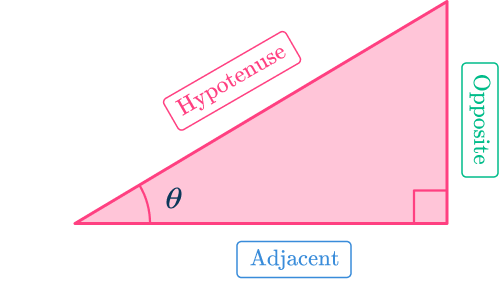
Where O= Opposite side, A= Adjacent side, and H= Hypotenuse for the right-angled triangle with respect to angle \theta (theta). The following relationships are considered trigonometric identities because they are equivalent, equal or identical.
- \sin(\theta)=\cfrac{O}{H}
- \cos(\theta)=\cfrac{A}{H}
- \tan(\theta)=\cfrac{O}{A}
Where O= Opposite side, A= Adjacent side, and H= Hypotenuse for the right-angled triangle with respect to angle \theta (theta).
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 9 to 12 students’ understanding of Trigonometry. 15+ questions with answers covering a range of 9th to 12th grade trigonometry topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 9 to 12 students’ understanding of Trigonometry. 15+ questions with answers covering a range of 9th to 12th grade trigonometry topics to identify areas of strength and support!
DOWNLOAD FREELet’s take a look at another trigonometric relationship that is equivalent, equal, or identical, hence making it a trigonometric identity.
\tan (\theta)=\cfrac{\sin (\theta)}{\cos (\theta)}This relationship can be proven true using the same right triangle. Substitute \sin \theta with \cfrac{O}{H} and \cos \theta with \cfrac{A}{H}.
\tan (\theta)=\cfrac{\sin \theta}{\cos \theta}=\cfrac{\cfrac{O}{H}}{\cfrac{A}{H}}=\cfrac{O}{A}This proves that \tan (\theta)=\cfrac{O}{A} which also equals \cfrac{\sin \theta}{\cos \theta} meaning that \tan (\theta)=\cfrac{\sin \theta}{\cos \theta} is always a true relationship so it is a trigonometric identity.
Let’s take a look at another trigonometric relationship that is equivalent, equal, or identical, hence making it a trigonometric identity.
\sin ^2(\theta)+\cos ^2(\theta)=1.This relationship can be proven true using a right triangle. Notice how values are assigned to each of the sides. Using the numerical values might be easier to see the relationship.

In this case, \sin \theta=\cfrac{3}{5} and \cos \theta=\cfrac{4}{5} so, let’s substitute those values in for \sin \theta and \cos \theta.
\begin{aligned} \sin ^2(\theta)+\cos ^2(\theta)&=1 \\\\ \left(\cfrac{3}{5}\right)^2+\left(\cfrac{4}{5}\right)^2&=1 \\\\ \cfrac{9}{25}+\cfrac{16}{25}&=1 \\\\ \cfrac{25}{25}&=1 \\\\ 1&=1 \end{aligned}This proves that \sin ^2(\theta)+\cos ^2(\theta)=1 is a true relationship meaning that it is equivalent, equal, or identical so it is a trigonometric identity.
\sin ^2(\theta)+\cos ^2(\theta)=1 is known as a Pythagorean theorem identity because you use the Pythagorean theorem to prove it true.
Both, \tan (\theta)=\cfrac{\sin \theta}{\cos \theta} and \sin ^2(\theta)+\cos ^2(\theta)=1 are identities that you can use to prove other identities.
What are trigonometric identities?

Common Core State Standards
How does this apply to high school math?
- High School Functions – Trigonometric Functions (HSF-TF.C.8)
Prove the Pythagorean identity \sin ^2(\theta)+\cos ^2(\theta)=1 and use it to find \sin(\theta), \, \cos(\theta), or \tan(\theta) given \sin(\theta), \, \cos(\theta), or \tan(\theta) and the quadrant of the angle.
How to prove trigonometric identities
In order to prove trigonometric identities:
- Choose one side of the identity to simplify.
- Substitute values using the trigonometric ratios or another trigonometric identity.
- Simplify the identity.
Trigonometric identities examples
Example 1: the relationship between sin, cos and tan
Prove that the trigonometric identity is true:
\cfrac{\sin \theta)}{\cos(\theta)}=\tan(\theta)- Choose one side of the identity to simplify.
In this case, use the left hand side of the identity to simplify.
2Substitute values using the trigonometric ratios or another trigonometric identity.
Using the relationships of the sides of a right triangle, you know that:
\sin (\theta)=\cfrac{O}{H}, \, \cos (\theta)=\cfrac{A}{H}, and \tan (\theta)=\cfrac{O}{A}
The equation can be rewritten to be:
\cfrac{\cfrac{O}{H}}{\cfrac{A}{H}}=\tan \theta3Simplify the identity.
Use the rules of division of fractions:
\begin{aligned}\cfrac{\cfrac{O}{H}}{\cfrac{A}{H}}&=\tan \theta \\\\ \cfrac{O}{H} \div \cfrac{A}{H}&=\tan \theta \\\\ \cfrac{O}{H} \times \cfrac{H}{A}&=\tan \theta \\\\ \cfrac{O}{A}&=\tan \theta \\\\ \tan \theta&=\tan \theta \end{aligned}Both sides of the equation are identical, meaning that the identity is proven to be true.
Example 2: the relationship between sin, cos and tan
Verify the identity:
\sin \theta \cot \theta \sec \theta=1Choose one side of the identity to simplify.
In this case, choose the left hand side of the identity to simplify.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the trigonometric ratios established from SOHCAHTOA, you know that:
\begin{aligned} \sin \theta&=\cfrac{O}{H} \\\\ \cot \theta&=\cfrac{A}{O} \\\\ \sec \theta&=\cfrac{H}{A} \end{aligned}
So, the identity can be rewritten to be:
\left(\cfrac{O}{H}\right)\left(\cfrac{A}{O}\right)\left(\cfrac{H}{A}\right)=1
Simplify the identity.
Using the rule for multiplying fractions,
\begin{aligned} \left(\cfrac{O}{H}\right)\left(\cfrac{A}{O}\right)\left(\cfrac{H}{A}\right)&=1 \\\\ \cfrac{O A H}{O A H}&=1 \\\\ 1&=1 \end{aligned}
The identity is identical on both sides of the equal sign, meaning that the identity is verified.
Example 3: the relationship between sin, cos and tan
Verify the identity:
\cot \theta=\cfrac{\cos \theta}{\sin \theta}Choose one side of the identity to simplify.
In this case, choose the right hand side of the identity to simplify.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the trigonometric ratios established from SOHCAHTOA, you know that:
\begin{aligned}& \cos \theta=\cfrac{A}{H} \\\\
& \sin \theta=\cfrac{O}{H} \end{aligned}
So, the identity can be rewritten to be:
\cot \theta=\cfrac{\cfrac{A}{H}}{\cfrac{O}{H}}
Simplify the identity.
Using the rule for division of fractions,
\begin{aligned}\cot \theta & =\cfrac{\cfrac{A}{H}}{\cfrac{O}{H}} \\\\
\cot \theta & =\cfrac{A}{H} \div\cfrac{O}{H} \\\\
\cot \theta & =\cfrac{A}{H} \times \cfrac{H}{O} \\\\
\cot \theta & =\cfrac{A}{O} \end{aligned}
We know that the cotangent is the reciprocal of the tangent.
So, if the \tan \theta=\cfrac{O}{A} then it’s true that the \cot \theta=\cfrac{A}{o}
So,
\begin{aligned}& \cot \theta=\cfrac{A}{o} \\\\
& \cot \theta=\cot \theta \end{aligned}
The identity is verified to be true.
Example 4: the relationship between sin, cos and tan
Verify the identity:
\cfrac{\cot \theta \sec \theta}{\csc \theta}=1Choose one side of the identity to simplify.
Choose the left side of the identity to simplify.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the trigonometric ratios established from SOHCAHTOA, you know that:
\begin{aligned}& \cot \theta=\cfrac{A}{O} \\\\
& \sec \theta=\cfrac{H}{A} \\\\
& \csc \theta=\cfrac{H}{O} \end{aligned}
So, the identity can be rewritten as:
\cfrac{\left(\cfrac{A}{O}\right)\left(\cfrac{H}{A}\right)}{\cfrac{H}{O}}=1
Simplify the identity.
Using the rule for multiplication and division of fractions simplify,
\begin{aligned} \cfrac{\left(\cfrac{A}{O}\right)\left(\cfrac{H}{A}\right)}{\cfrac{H}{O}}&=1 \\\\ \cfrac{\cfrac{H}{O}}{\cfrac{H}{O}}&=1 \\\\ 1&=1 \end{aligned}
The identity is verified to be true.
Example 5: the relationship between sin, cos and tan
Verify the identity:
\sin x \sec x=\tan xChoose one side of the identity to simplify.
In this case, choose the left hand side of the identity to simplify.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the trigonometric ratios established from SOHCAHTOA, you know that:
\begin{aligned} \sin \theta&=\cfrac{O}{H} \\\\ \sec \theta&=\cfrac{H}{A} \end{aligned}
So, the identity can be rewritten as:
\left(\cfrac{O}{H}\right)\left(\cfrac{H}{A}\right)=\tan x
Simplify the identity.
From SOHCAHTOA, you know that \tan x=\cfrac{O}{A}
\begin{aligned} \cfrac{O}{A}&=\tan x \\\\ \tan x&=\tan x \end{aligned}
The trigonometric identity is verified to be true.
Example 6: using Pythagoras’ theorem to prove a trigonometric identity
Verify the identity:
\sin ^2(\theta)+\cos ^2(\theta)=1Choose one side of the identity to simplify.
In this case, simplify the left hand side of the identity.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the trigonometric ratios established from SOHCAHTOA, you know that:
\sin \theta=\cfrac{O}{H}
\cos \theta=\cfrac{A}{H}
Rewrite the identity to be:
\left(\cfrac{O}{H}\right)^2+\left(\cfrac{A}{H}\right)^2=1
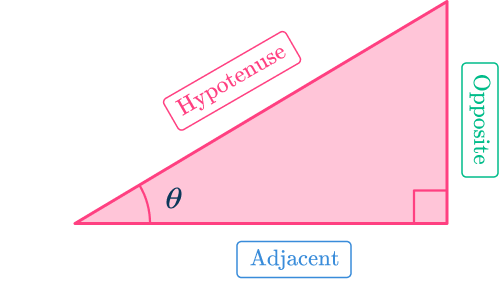
Simplify the identity.
From the right triangle and using Pythagorean theorem, you know that A^2+O^2=H^2 .
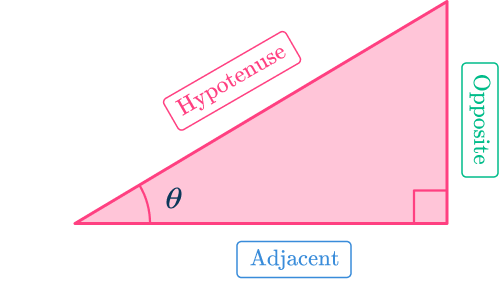
So, you can substitute A^2+O^2 with H^2 .
\begin{aligned} \cfrac{O^2+A^2}{H^2}&=1 \\\\ \cfrac{H^2}{H^2}&=1 \\\\ 1&=1 \end{aligned}
The identity is verified to be true.
Example 7: proving identities using exact trig values
Verify the identity \cos(60)=1-\sin(30) using the given right triangle.
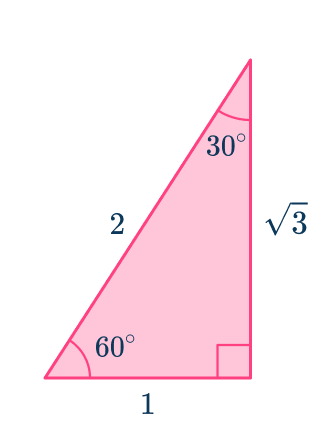
Choose one side of the identity to simplify.
In this case, choose the right hand side of the identity to simplify.
Substitute values using the trigonometric ratios or another trigonometric identity.
Using the right triangle and SOHCAHTOA, the following relationship is true.
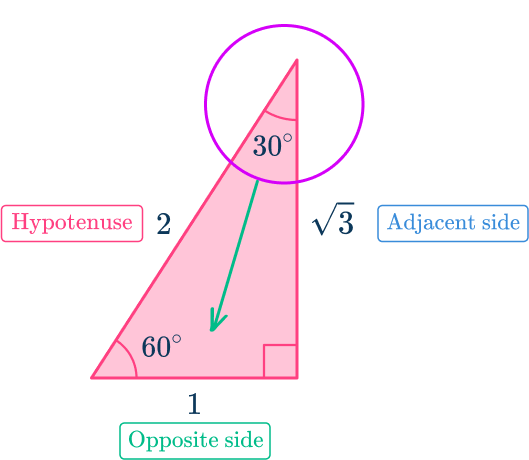
\sin (30)=\cfrac{O}{H}=\cfrac{1}{2}
Rewrite the identity to be:
\cos (60)=1-\cfrac{1}{2}
Simplify the identity.
From the triangle and SOHCAHTOA, \cfrac{1}{2} is equal to \cos (60).
\begin{aligned} \cos (60)&=\cfrac{1}{2} \\\\ \cos (60)&=\cos (60) \end{aligned}
The identity is verified to be true.
Teaching tips for trigonometric identities
- Have students investigate trig identities using inquiry based platforms such as Desmos.
- Instead of having students practice problems with worksheets, have them engage in collaborative learning activities such as jigsaw tasks, scavenger hunts, and/or gallery walks.
- Engage students with team game-playing to review skills with platforms such as Blooket and Quizziz.
Easy mistakes to make
- Treating an identity like an equation
You do not solve an identity by balancing both sides of the equal sign like an equation. An identity is a statement where two expressions or functions are mathematically identical. For example, 3x+6\equiv{3}(x+2) because when it is factored, the expression becomes 3(x+2).
- Mixing up the right triangle trigonometric ratios
It is key to remember the three trig ratios that are written as the mnemonic SOHCAHTOA. This way you will not confuse the relationships between the sides and the angles.
\begin{aligned}\sin \theta & =\cfrac{O}{H} \\\\ \cos \theta & =\cfrac{A}{H} \\\\ \tan \theta & =\cfrac{O}{A} \\\\ \csc \theta & =\cfrac{H}{O} \text { (reciprocal of the sine) } \\\\ \sec \theta & =\cfrac{H}{A} \text { (reciprocal of the cosine) } \\\\ \cot \theta & =\cfrac{A}{O} \text { (reciprocal of the tangent) } \end{aligned}
Related trigonometry lessons
- Trigonometry
- Trig functions
- How to find the exact values of a trig function
- Sin cos tan
- Law of sines
- Trig formula for area of a triangle
- Trig formulas
- Law of cosines
- Trig table
Practice trigonometric identities questions
1. Which of the following trigonometric identities is mathematically correct?




From SOHCAHTOA, you know that \sin(\theta)=\cfrac{O}{H} and \cos(\theta)=\cfrac{A}{H},
This means that:
\sin(\theta)\div\cos(\theta)=\cfrac{O}{H} \div \cfrac{A}{H}=\cfrac{O}{H}\times \cfrac{H}{A}=\cfrac{OH}{HA}=\cfrac{O}{A}=\tan(\theta)
2. Which of the following trigonometric identities is mathematically correct?




Using the trigonometric ratios from SOHCAHTOA, you know that \cos \theta=\cfrac{A}{H} and \csc \theta=\cfrac{H}{O}
Rewrite the trigonometric identity to be:
\left(\cfrac{A}{H}\right)\left(\cfrac{H}{O}\right)=\cot \theta
Simplify the left hand side of the identity,
\left(\cfrac{A}{O}\right)=\cot \theta
From SOHCAHTOA, the cotangent is the reciprocal of the tangent. So, if \tan \theta=\cfrac{O}{A} then it is true that \cot \theta=\cfrac{A}{o}
So,
\begin{aligned}& \left(\cfrac{A}{O}\right)=\cot \theta \\\\ & \cot \theta=\cot \theta \end{aligned}
You can also use the identity that \csc \theta=\cfrac{1}{\sin \theta} because the sine is the reciprocal of the cosecant which means you can rewrite the identity to be:
\begin{aligned} \cos \theta \times \cfrac{1}{\sin \theta}&=\cot \theta \\\\ \cfrac{\cos \theta}{\sin \theta}&=\cot \theta \end{aligned}
3. Which of the following trigonometric functions is identical to \cfrac{\tan(\theta)}{\sin(\theta)}?




Using the right triangle ratios from SOHCAHTOA, you know that
\sin(\theta)=\cfrac{O}{H}, \, \tan(\theta)=\cfrac{O}{A}, and \cos(\theta)=\cfrac{A}{H},
\tan(\theta)\div\sin(\theta) is equivalent to
\begin{aligned}=&\cfrac{O}{A}\div\cfrac{O}{H} \\\\ =&\cfrac{O}{A}\times\cfrac{H}{O} \\\\ =&\cfrac{OH}{AO} \\\\ =&\cfrac{H}{A} \\\\ =&\cfrac{1}{\cos(\theta)} \end{aligned}
\begin{aligned}& \cfrac{\tan \theta}{\sin \theta}=\cfrac{1}{\cos \theta} \\\\ & \cfrac{1}{\cos \theta}=\cfrac{1}{\cos \theta} \end{aligned}
4. Which solution is identical to 2\cos(45)\times\sin(45)?
Use the triangle below to help you.
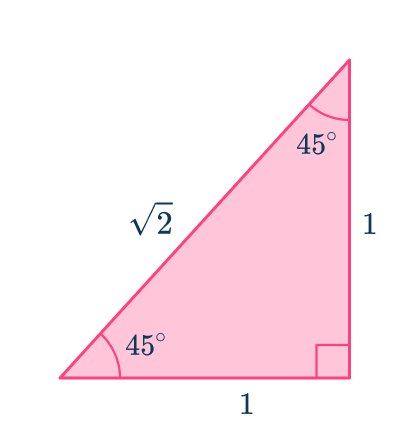




Using the right triangle and the ratios from SOHCAHTOA,
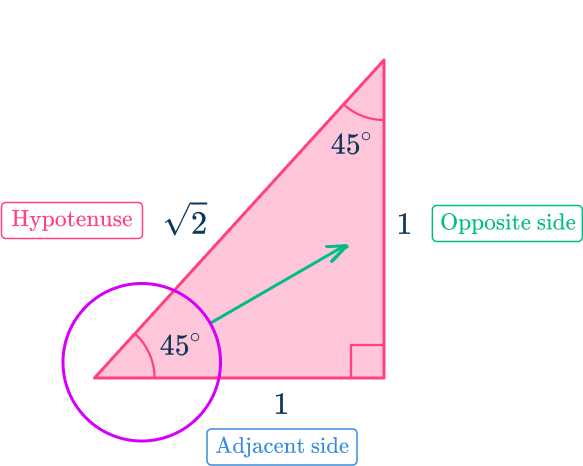
\begin{aligned}&\begin{aligned}& \cos \theta=\cfrac{A}{H} \\\\ & \cos 45=\cfrac{1}{\sqrt{2}} \end{aligned} \\\\ &\begin{aligned}& \sin \theta=\cfrac{O}{H} \\\\ & \sin 45=\cfrac{1}{\sqrt{2}} \end{aligned} \end{aligned}
\begin{aligned}& \cfrac{\tan \theta}{\sin \theta}=\cfrac{1}{\cos \theta} \\\\ & \cfrac{1}{\cos \theta}=\cfrac{1}{\cos \theta} \end{aligned}
Rewrite the identity to be:
\begin{aligned}& 2\left(\cfrac{1}{\sqrt{2}}\right) \times 2\left(\cfrac{1}{\sqrt{2}}\right)=4\left(\cfrac{1}{\sqrt{4}}\right) \\\\ & 4\left(\cfrac{1}{\sqrt{4}}\right)=4 \times \cfrac{1}{2}=\cfrac{4}{2}=2\end{aligned}
This verifies that
2=2
5. Select the expression that is identical to \cfrac{\cos \theta \tan \theta}{\sin \theta}.




Using the ratios from SOHCAHTOA, you know that:
\begin{aligned}\cos \theta & =\cfrac{A}{H} \\\\ \tan \theta & =\cfrac{A}{O} \\\\ \sin \theta & =\cfrac{O}{H} \end{aligned}
Rewrite the identity to be:
\left(\cfrac{A}{H} \times \cfrac{O}{A}\right) \div \cfrac{O}{H}
Using rules for multiplying and dividing fractions,
\cfrac{O}{H} \times \cfrac{H}{O}=\cfrac{O H}{O H}=1
Another way to solve it would be to use the identity that
\tan \theta=\cfrac{\sin \theta}{\cos \theta}
Rewrite the identity to be:
\begin{aligned}& \cfrac{\cos \theta \times \cfrac{\sin \theta}{\cos \theta}}{\sin \theta} \\\\ & \cfrac{\sin \theta}{\sin \theta}=1 \end{aligned}
6. Select the expression that is identical to \cfrac{\sin(\theta)}{\sqrt{1-\sin^{2}(\theta)}}?




Using the Pythagorean identity, \sin ^2 \theta+\cos ^2 \theta=1
The identity can be rearranged by solving for \cos \theta\text{:}
\begin{aligned}& \cos ^2 \theta=1-\sin ^2 \theta \\\\ & \cos \theta=\sqrt{1-\sin ^2 \theta} \end{aligned}
Rewrite the identity to be:
\cfrac{\sin \theta}{\cos \theta}
You know from SOHCAHTOA that
\begin{aligned}& \sin \theta=\cfrac{O}{H} \\\\ & \cos \theta=\cfrac{A}{H} \end{aligned}
So, \cfrac{\cfrac{O}{H}}{\cfrac{A}{H}}=\cfrac{O}{H} \div \cfrac{A}{H}=\cfrac{O}{H} \times \cfrac{H}{A}=\cfrac{O}{A}
\cfrac{O}{A}=\tan \theta
But, \cfrac{\sin \theta}{\cos \theta}=\tan \theta is an identity that can be used to verify other identities.
Trigonometric identities FAQs
No, you can use other relationships to help verify identities such as secant being the reciprocal of cosine, cotangent being the reciprocal of the tangent, etc.
Yes, as you continue to learn about trig identities, there are other fundamental trigonometric identities that you will learn how to verify and use, cofunction identities, sum identities, difference identities, product identities, half-angle identities, and double angle identities. They are also referred to as double-angle formulas, half-angle formulas, difference formulas, and sum formulas.
Yes, the unit circle is helpful when verifying identities because it can be used as a base for proving identities. The unit circle has a radius of 1 and special right triangles can be formed within it where the radius of 1 is the hypotenuse of the triangles.
In order to solve trigonometric equations, you may have to apply fundamental trigonometric identities and the unit circle to find the radian measure of the angles in the equation.
Yes, the sine and cosine are complementary.
The next lessons are
- Circle math
- Sectors, arcs and segments
- Circle theorems
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!