High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Sin cos tan SOHCAHTOA Solving equations Rearranging equationsLaw of sines
Here is everything you need to know about the Law of Sines. You will learn what it is and how to use it to find the missing sides and angles of a triangle.
Students first learn how to work with the law of sines in geometry and expand that knowledge as they progress through algebra 2 and precalculus.
What is the law of sines?
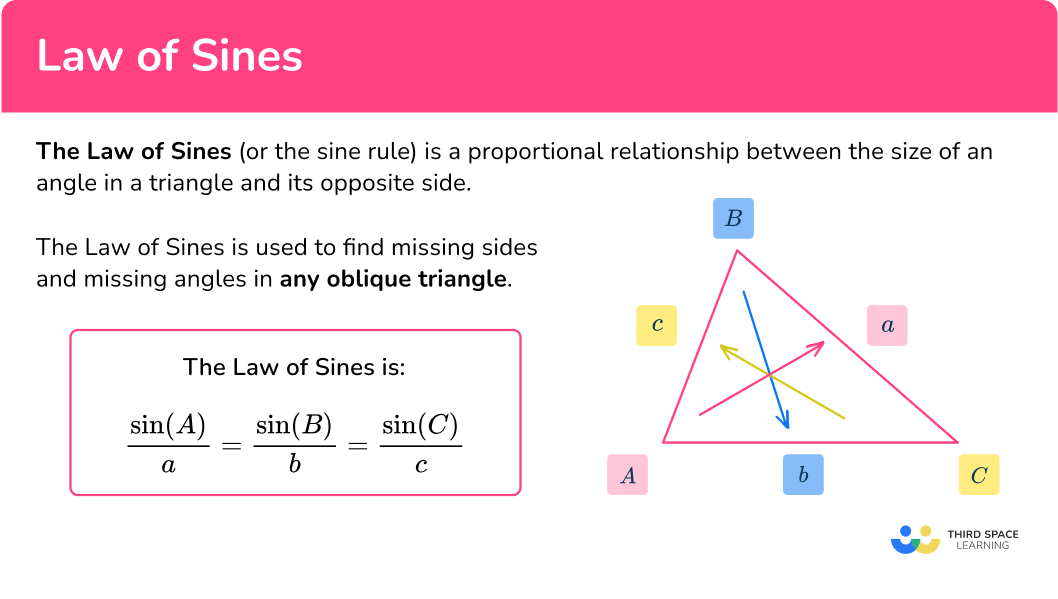
The Law of Sines (or the sine rule) is a proportional relationship between the size of an angle in a triangle and its opposite side.
The Law of Sines is used to find the missing sides and missing angles of a triangle.
Recall that SOHCAHTOA is used to find missing sides and angles in right triangles (right-angled triangles).
The Law of Sines can be used with any oblique triangle and also with right triangles.
The proportional relationship is:
\cfrac{\sin(A)}{a}=\cfrac{\sin(B)}{b}=\cfrac{\sin(C)}{c}
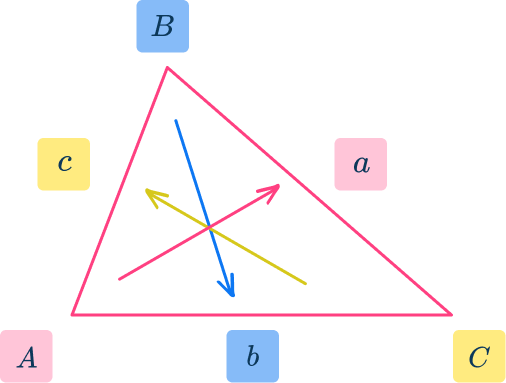
Notice that angle A (vertex A ) is across from side a, angle B (vertex B ) is across side b, and angle C (vertex C ) is across side c.

Even though there are three relationships in a triangle between the three angles and their opposite sides, you only need to use two of them for the Law of Sines.
This would look like:
\cfrac{\sin(A)}{a}=\cfrac{\sin(B)}{b}
The law of sines in this form is useful to find a missing angle. By rearranging the law of sines, the reciprocal is also useful to know if you want to find a missing side length:
\cfrac{a}{\sin(A)}=\cfrac{b}{\sin(B)}\left(=\cfrac{c}{\sin(C)}\right)
What is the Law of Sines?
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 9 to 12 students’ understanding of Trigonometry. 15+ questions with answers covering a range of 9th to 12th grade trigonometry topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)
![[FREE] Trigonometry Check for Understanding Quiz (Grade 9 to 12)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Trigonometry-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 9 to 12 students’ understanding of Trigonometry. 15+ questions with answers covering a range of 9th to 12th grade trigonometry topics to identify areas of strength and support!
DOWNLOAD FREECommon Core State Standards
How does this relate to high school math?
- High School Geometry – Similarity, Right Triangles, and Trigonometry – HSG-SRT.D.10 (+)
Prove the Laws of Sines and Cosines and use them to solve problems.
- High School Geometry – Similarity, Right Triangles, and Trigonometry – HSG-SRT.D.11 (+)
Understand and apply the Law of Sines and the Law of Cosines to find unknown measurements in right and non-right triangles (example, surveying problems, resultant forces).
How to use the law of sines
In order to use the law of sines to find missing sides and angles:
- Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
- Use the law of sines to write the proportional relationship.
- Solve for the missing side or angle.
Law of sines examples
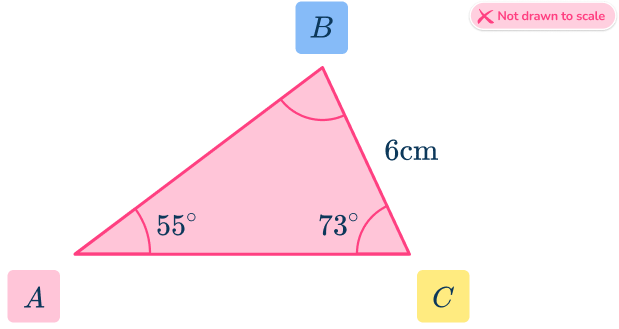
Example 1: finding a missing side of a triangle.
Find the length of side AB. Write your answer to two decimal places.
- Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
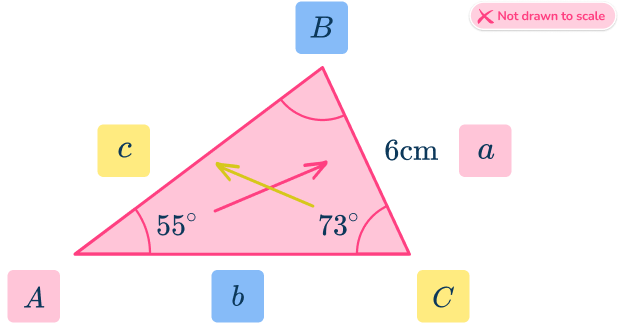
Side AB is labeled c, so you are solving for c.
2Use the law of sines to write the proportional relationship.
Using the values known and the law of sines, the proportional relationship is:
\cfrac{\sin(C)}{c}=\cfrac{\sin(A)}{a}Substitute in the values:
\cfrac{\sin(73)}{c}=\cfrac{\sin(55)}{6}3Solve for the missing side or angle.
Multiply both sides of the equation by c .
\cfrac{\sin(73)}{c}\times{c}=\cfrac{\sin(55)}{6}\times{c} \sin(73)=\cfrac{\sin(55)}{6}\times{c}Multiply both sides by 6 .
\sin(73)\times{6}=\cfrac{\sin(55)}{6}\times{c}\times{6} 6\sin(73)=c\sin(55)Divide both sides by \sin(55) .
\cfrac{6\sin(73)}{\sin(55)}=\cfrac{c\sin(55)}{\sin(55)} \cfrac{6\sin(73)}{\sin(55)}=cUse the calculator to calculate the value of c .
c=7.00\mathrm{~cm}\text{ (2dp)}Example 2: finding a missing side of a triangle.
Find side BC. Write your answer to two decimal places.

Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
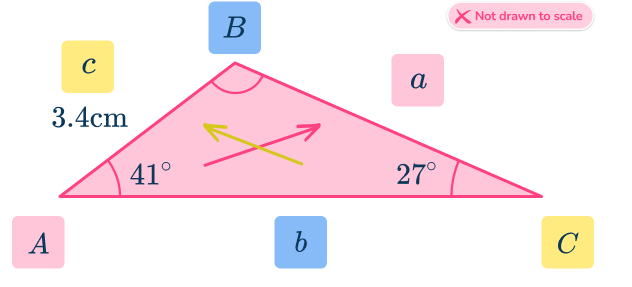
Side BC is labeled a.
Use the law of sines to write the proportional relationship.
Using the law of sines, the proportional relationship is:
\cfrac{\sin(A)}{a}=\cfrac{\sin(C)}{c}
Substitute in the values.
\cfrac{\sin(41)}{a}=\cfrac{\sin(27)}{3.4}
Solve for the missing side or angle.
Cross multiply to remove the denominators of each fraction.
\cfrac{\sin(41)}{a}\times{3.4}\times{a}=\cfrac{\sin(27)}{3.4}\times{3.4}\times{a}
3.4\sin(41)=a\sin(27)
Divide both sides by \sin(27) .
\cfrac{3.4\sin(41)}{\sin(27)}=a
Use a calculator to solve.
a=4.91\mathrm{~cm}\text{ (2dp)}
Example 3: finding a missing side of a triangle.
Find the length AC. Write your answer to two decimal places.
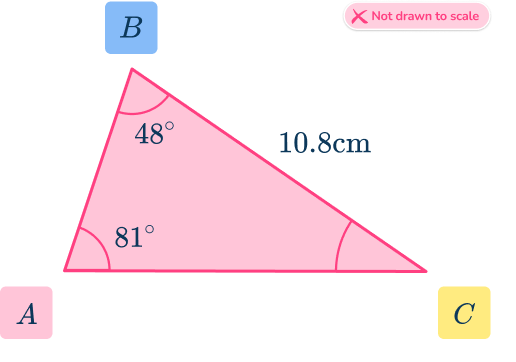
Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
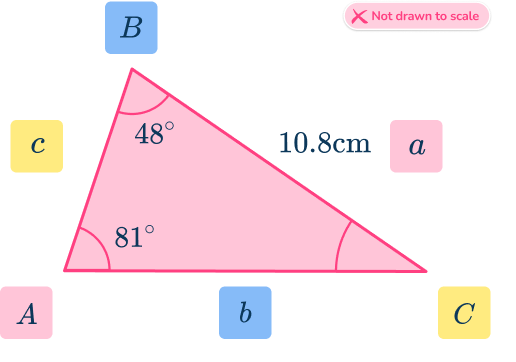
Side AC is labeled b.
Use the law of sines to write the proportional relationship.
Using the law of sines, the proportional relationship is:
\cfrac{\sin(A)}{a}=\cfrac{\sin(B)}{b}
Substitute in the known values.
\cfrac{\sin(81)}{10.8}=\cfrac{\sin(48)}{b}
Solve for the missing side or angle.
Cross multiply to solve for b .
\cfrac{\sin(81)}{10.8}=\cfrac{\sin(48)}{b}
b\times\sin(81)=10.8\times\sin(48)
Divide both sides by \sin(81) .
\cfrac{b\sin(81)}{\sin(81)}=\cfrac{10.8\sin(48)}{\sin(81)}
b=\cfrac{10.8\sin(48)}{\sin(81)}
Use a calculator to calculate the value of b (and therefore AC ).
b=A C=8.13 \mathrm{~cm}
Example 4: finding a missing angle of a triangle.
Find the measure of the missing angle \theta. Write your answer to one decimal place.
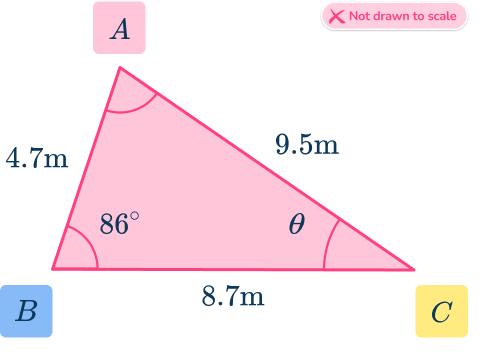
Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
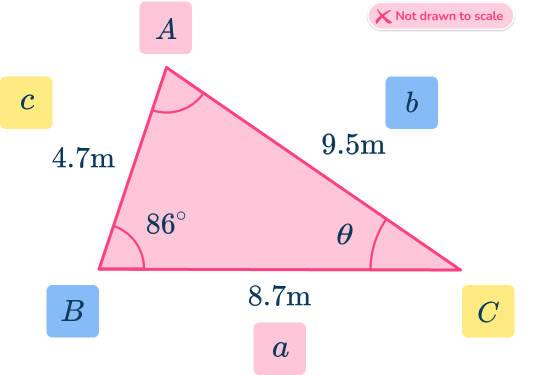
Angle \theta is the same as the angle at C.
Use the law of sines to write the proportional relationship.
Using the law of sines, the proportional relationship is:
\cfrac{\sin(C)}{c}=\cfrac{\sin(B)}{b}
Substitute the values in.
\cfrac{\sin(\theta)}{4.7}=\cfrac{\sin(86)}{9.5}
Solve for the missing side or angle.
Cross multiply to solve.
9.5\times\sin(\theta)=4.7\times\sin(86)
Divide both sides by 9.5 .
\cfrac{\sin(\theta)}{9.5}=\cfrac{4.7\sin(86)}{9.5}
\sin(\theta)=0.4935\text{ (4dp)}
Use the inverse sine function to make \theta the subject.
\sin^{-1}(\sin(\theta))=\sin^{-1}(0.4935)
\theta=\sin^{-1}(0.4935)=29.6^{\circ}\text{ (1dp)}
C=\theta=29.6^{\circ}
Be sure to check if your answer is reasonable. The larger the angle, the longer the opposite side length is. As 4.7\mathrm{~m}<9.5\mathrm{~m} , the angle at C must be smaller than the angle at B , so 29.6^{\circ} is reasonable.
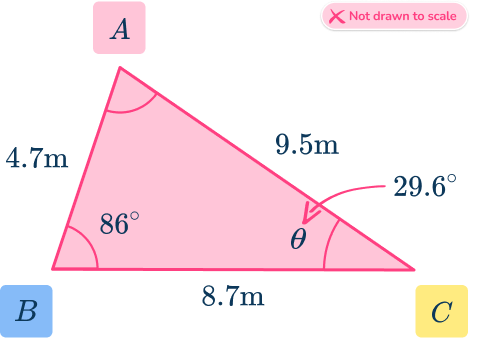
Example 5: finding a missing angle of a triangle.
Find the measure of the missing angle \theta. Write your answer to one decimal place.
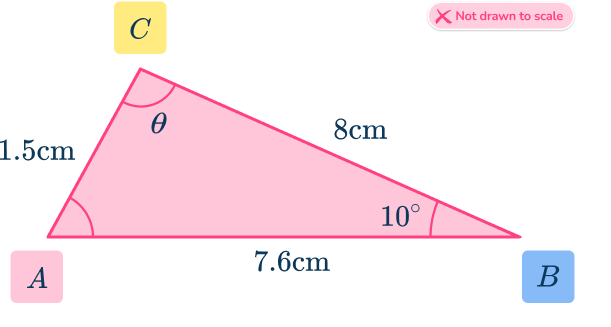
Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
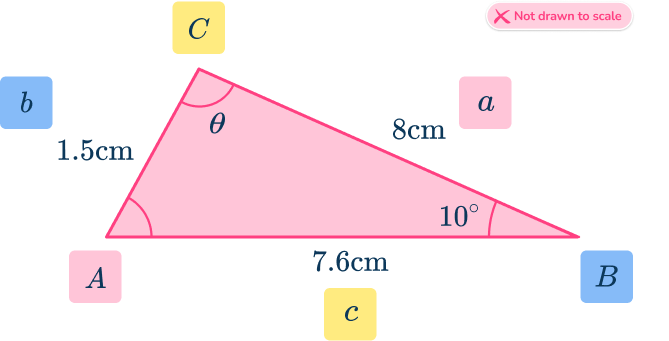
The angle \theta is labeled C.
Use the law of sines to write the proportional relationship.
Using the law of sines, the proportional relationship is:
\cfrac{\sin(C)}{c}=\cfrac{\sin(B)}{b}
Substitute in the values.
\cfrac{\sin(\theta)}{7.6}=\cfrac{\sin(10)}{1.5}
Solve for the missing side or angle.
Cross multiply to solve.
\cfrac{\sin(\theta)}{7.6}=\cfrac{\sin(10)}{1.5}
1.5\times\sin(\theta)=7.6\times\sin(10)
Divide both sides of the equation by 1.5
\cfrac{\sin(\theta)}{1.5}=\cfrac{7.6\sin(10)}{1.5}
\sin(\theta)=0.8798
Use the inverse sine function to make \theta the subject.
\sin^{-1}(\sin(\theta))=\sin^{-1}(0.8798)
\theta=\sin^{-1}(0.8798)
Use a calculator to work out the value of \theta .
\theta=61.62^{\circ}
Be sure to check if your answer makes sense. As a larger side is opposite a larger angle, it’s reasonable to think that angle \theta is about 61.6^{\circ} .
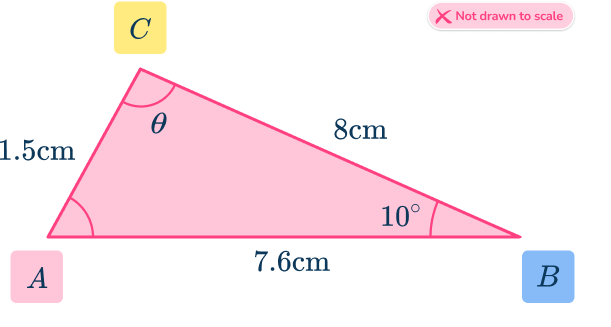
Example 6: finding a missing angle of a triangle.
Find the measure of the missing angle \theta.
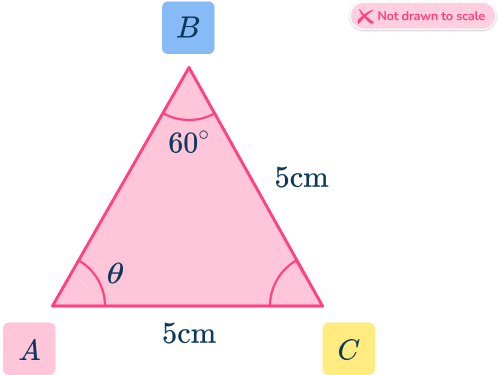
Label each angle \textbf{(A, B, C)} and each side \textbf{(a, b, c)} of the triangle.
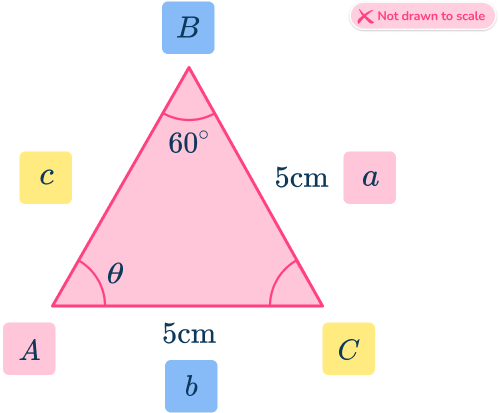
\theta is the angle at A.
Use the law of sines to write the proportional relationship.
Using law of sines, the proportional relationship is:
\cfrac{\sin(A)}{a}=\cfrac{\sin(B)}{b}
Substitute in the values:
\cfrac{\sin(\theta)}{5}=\cfrac{\sin(60)}{5}
Solve for the missing side or angle.
By comparing the two sides of the equation, \theta must be equal to 60^{\circ}.
The triangle is an equilateral triangle.
Teaching tips for Law of Sines
- Provide opportunities for students to investigate the law of sines.
- Give students different scenarios to determine when the law of sines works. For example, when the triangle has known parts set up as ASA, AAS, SAS, SSS\text{.}
- Accurately construct triangles for students to investigate the law of sines. What happens with similar triangles? What happens if one angle is double the original size?
Easy mistakes to make
- Labeling the triangle incorrectly
Labeling the triangle incorrectly can lead to incorrect calculations and incorrect use of the law of sines. For example, this triangle has been incorrectly labeled with the side next to the angle.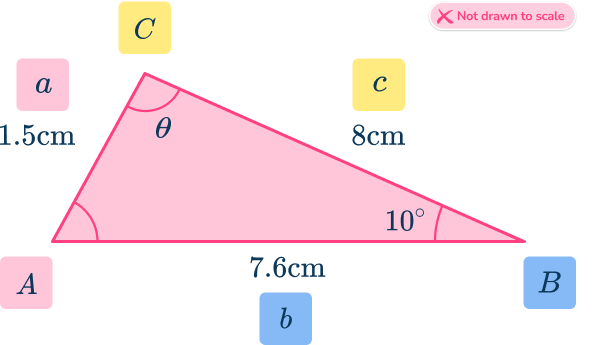
Remember, the correct way is to label the angles and across from the angle is the side, for example: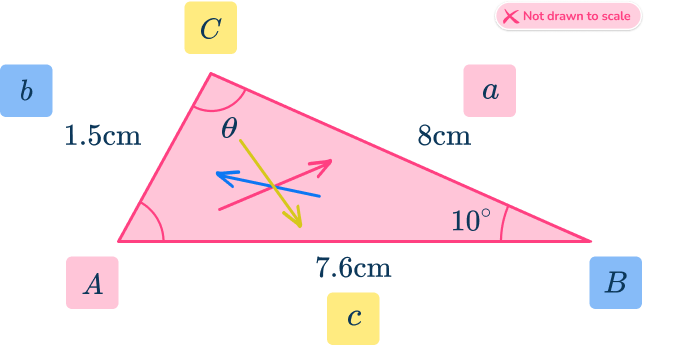
- Mixing up when to use the sine and inverse sine function
Remember that when finding a side, you have to use the sine function. When finding a missing angle, you need to use the inverse sine function (\sin^{-1}).
- Mixing up the radian measure and degree measure when using trigonometric functions
For example, when finding a missing angle of a triangle, be sure to express the angle in degree measure. So, in your calculator be sure the ‘mode’ is in degrees, not radians.
- Using the incorrect set up for the sine rule formula
It should not be too difficult to rearrange the formula once you have substituted the values, however small errors will make an impact on your solution.
- Canceling out sine functions
For example, when calculating the value for a in example 2, the following calculation error is made.
\begin{aligned}\cfrac{a}{\sin(41)}&=\cfrac{3.4}{\sin(27)} \\\\ a&=\cfrac{3.4\sin(41)}{\sin(27)} \\\\ &=\cfrac{3.4\times{41}}{27} \\\\ &=5.16\text{ (2dp)} \end{aligned}
Related trigonometry lessons
- Trig functions
- How to find the exact value of a trig function
- Trig formula for area of a triangle
- Trig formulas
- Trig identities
- Law of cosines
- Trig table
Law of sines practice questions
1. Find the length of BC. Write your answer to two decimal places.
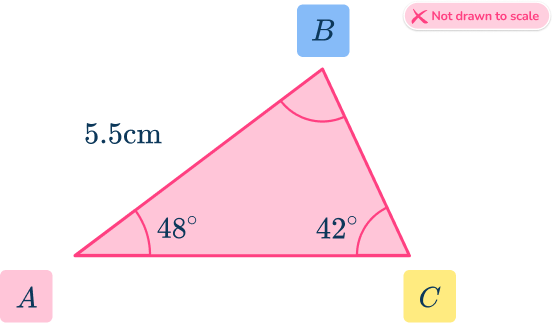




First label the angles and sides of the triangle.
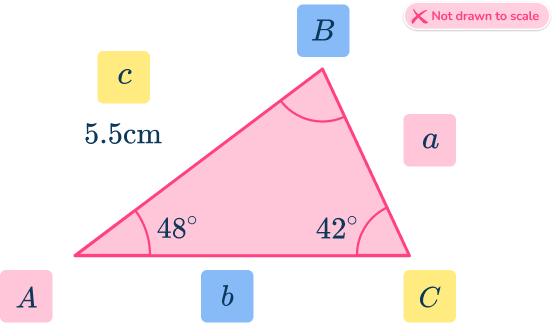
In this case, side BC is labeled a. Using the law of sines, the proportional relationship is:
\cfrac{a}{\sin(A)}=\cfrac{c}{\sin(C)}
Substitute the known values and solve for a.
\begin{aligned}\cfrac{a}{\sin(48)}&=\cfrac{5.5}{\sin(42)} \\\\ a&=\cfrac{5.5}{\sin(42)}\times\sin(48) \\\\ a&=6.11\mathrm{~cm} \end{aligned}
2. Find the length PQ. Write your answer to two decimal places.





First label the sides and the angles of the triangle using A, B, C.
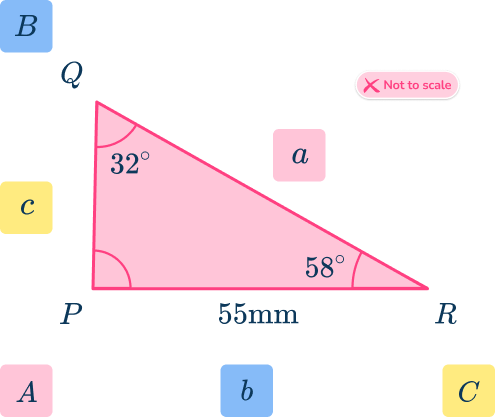
Using the law sines, the correct proportional relationship is:
\cfrac{b}{\sin(B)}=\cfrac{c}{\sin(C)}
Substitute in the values.
\begin{aligned}\cfrac{55}{\sin(32)}&=\cfrac{c}{\sin(58)} \\\\ c&=\cfrac{55}{\sin(32)}\times\sin(58) \\\\ &=88.02\mathrm{~mm} \end{aligned}
3. Find the unknown side length XY. Write your answer to two decimal places.
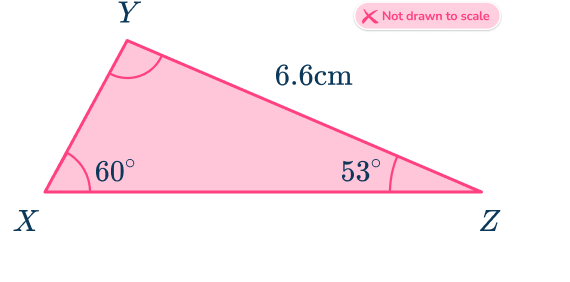




First, label the triangle using A, B, C and a, b, c.
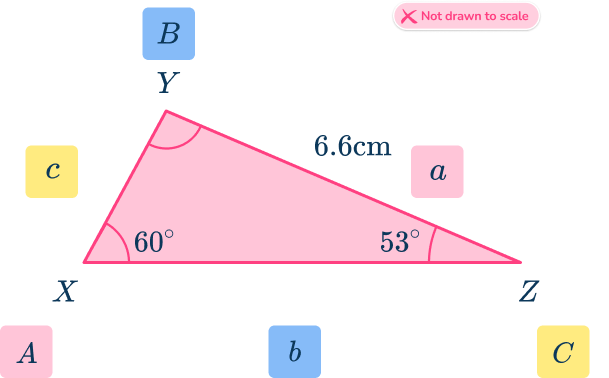
Using the law of sines, the proportional relationship is:
\cfrac{a}{\sin(A)}=\cfrac{c}{\sin(C)}
Substitute in the values.
\begin{aligned}\cfrac{6.6}{\sin(60)}&=\cfrac{c}{\sin(53)} \\\\ c&=\cfrac{6.6}{\sin(60)}\times\sin(53) \\\\ &=6.09\mathrm{~cm} \end{aligned}
4. Find the measure of the angle \theta. Write your answer to the nearest whole degree.
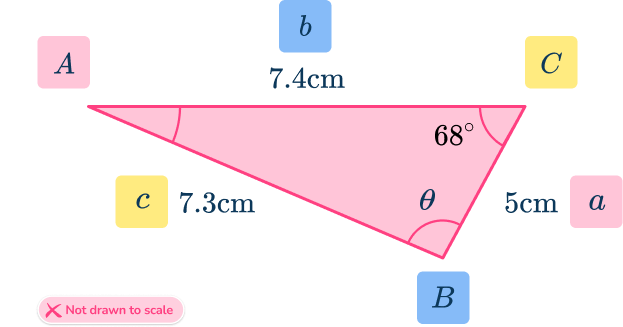




First, label the triangle using A, B, C and a, b, c.
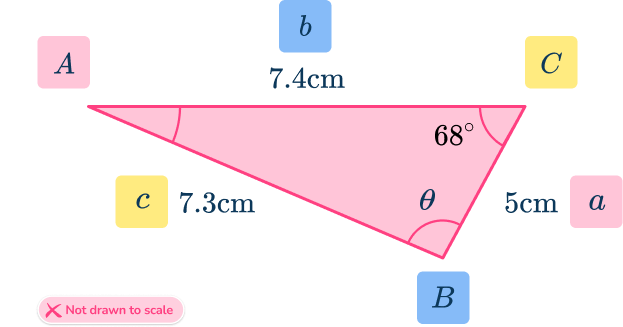
Using the law of sines, the proportional relationship is:
\cfrac{\sin(B)}{b}=\cfrac{\sin(C)}{c}
Substitute in the values:
\begin{aligned}\cfrac{\sin(\theta)}{7.4}&=\cfrac{\sin(68)}{7.3} \\\\ \sin(\theta)&=\cfrac{\sin(68)}{7.3}\times{7.4} \\\\ \sin(\theta)&=0.9398850033… \\\\ \theta&=\sin^{-1}(0.9398850033…) \\\\ \theta&=70^{\circ}\text{ (2sf)} \end{aligned}
5. Find the measure of angle \theta. Write your answer to two decimal places.
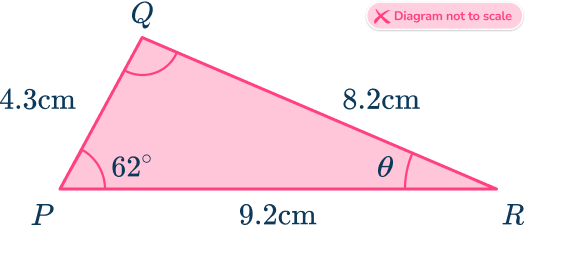




First, label the triangle using A, B, C and a, b, c.
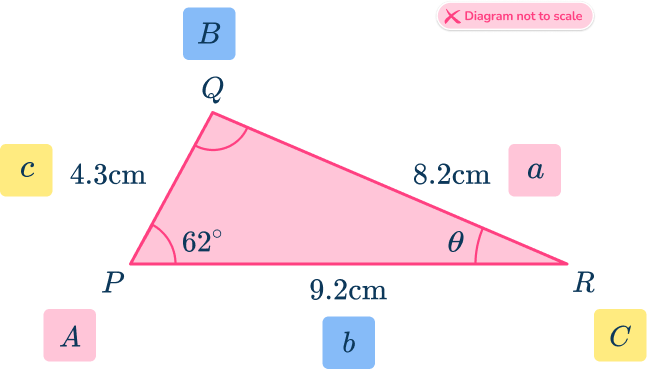
Using the law of sines, the proportional relationship is:
\cfrac{\sin(A)}{a}=\cfrac{\sin(C)}{c}
Substitute in the values:
\begin{aligned}\cfrac{\sin(62)}{8.2}&=\cfrac{\sin(\theta)}{4.3} \\\\ \sin(\theta)&=\cfrac{\sin(62)}{8.2}\times{4.3} \\\\ \sin(\theta)&=0.4630091036… \\\\ \theta&=\sin ^{-1}(0.4630091036) \\\\ \theta&=27.58^{\circ}\text{ (2dp)} \end{aligned}
6. Find the measure of the unknown angle which is labeled as \theta. Round your answer to the nearest tenth.
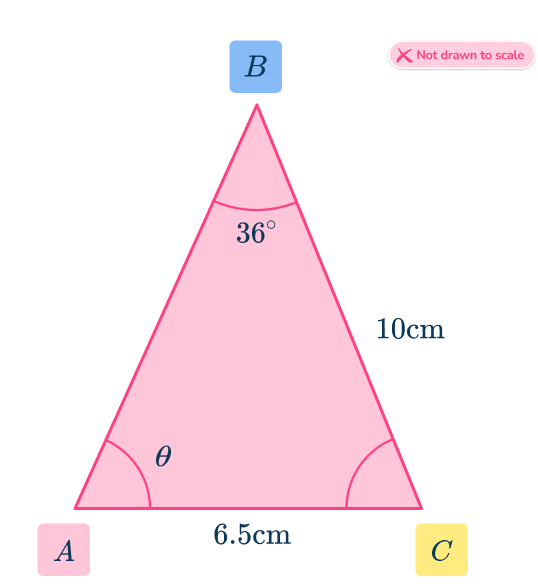




Label the triangle using A, B, C and a, b, c.
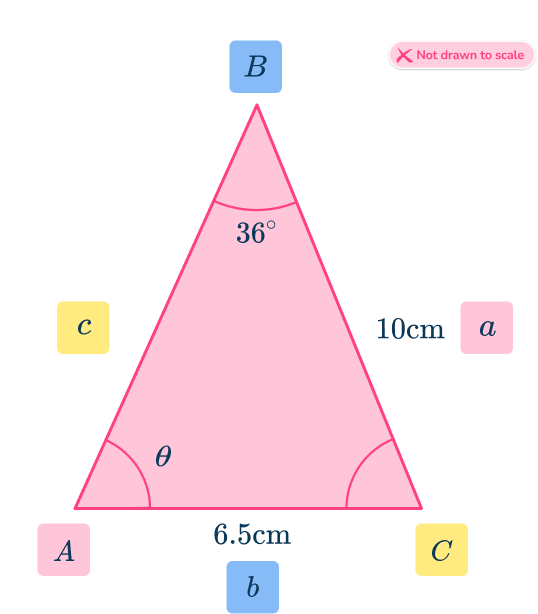
Using the law of sines, make the proportional relationship.
\cfrac{\sin(A)}{a}=\cfrac{\sin(B)}{b}
Substitute in the values:
\begin{aligned}\cfrac{\sin(\theta)}{10}&=\cfrac{\sin(36)}{6.5} \\\\ \sin(\theta)&=\cfrac{\sin(36)}{6.5}\times{10} \\\\ \sin(\theta)&=0.9042850035… \\\\ \theta&=\sin^{-1}(0.9042850035…) \\\\ \theta&=64.72^{\circ}\text{ (3sf)} \end{aligned}
Law of sines FAQs
Yes, you can use the law of sines to find the missing angles and sides of right triangles. The right angle and the hypotenuse would be one of the ratios you would use.
Yes, you can use the reciprocal of the law of sines meaning that you can write the proportion as \cfrac{a}{\sin(A)}=\cfrac{b}{\sin(B)}=\cfrac{c}{\sin(C)}.
No, not all the trigonometric functions have laws or rules.
The ambiguous case of the law of sines is when it is used to find a missing side length of a triangle and the result has two possible solutions for the measure of the same side. This will happen when the triangle has two sides and a non-included angle or (SSA) set up.
The law of sines can be helpful to find a missing side length, such as the base or the height of the triangle. Similarly, the law of cosines can also be used to help find the area of a triangle.
There is a law of tangents, but it is rarely or never taught in high school. However, if you pursue mathematics in college, there is a possibility you will learn about it.
The next lessons are
- Circle math
- Angles of a circle
- Circle theorems
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!