Hemisphere Shape - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Multiplying fractions Area of a circle Rounding Sphere shape Volume of a sphere Surface area of a sphere

Math resources Geometry 3D shapes

Hemisphere shape

Here you will learn about the hemisphere shape, including what a hemisphere is and how to calculate the volume of a hemisphere and the surface area of a hemisphere.

Students will first learn about the hemisphere shape as part of geometry in elementary school, but they will learn calculations involving the hemisphere shape in high school.

What is a hemisphere shape?

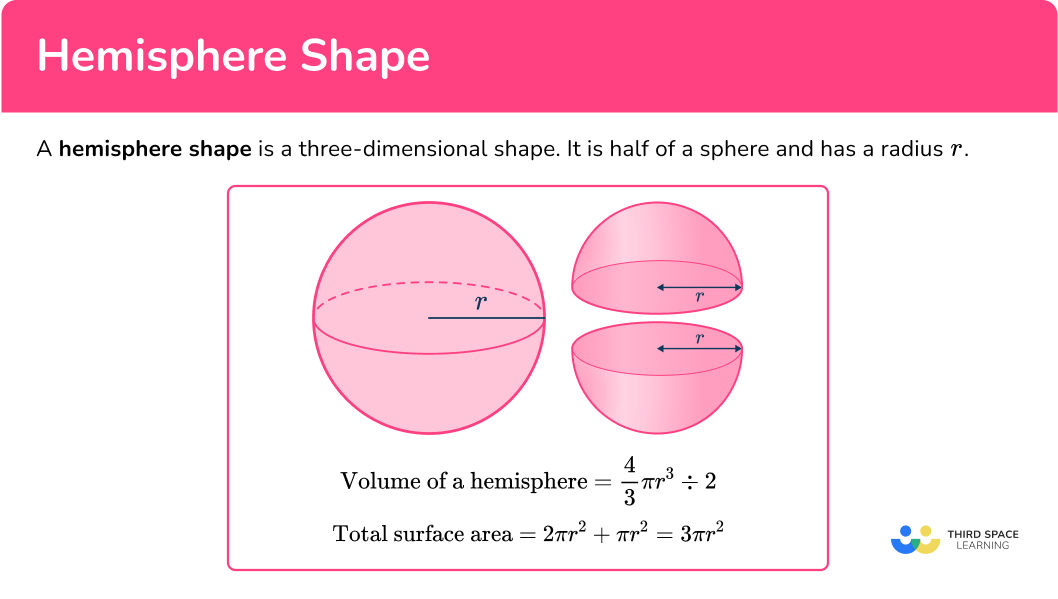
A hemisphere shape is a three-dimensional shape that is half of a sphere.

To visualize this, let’s look at a sphere. A sphere is a 3D shape where every point of its surface is equidistant (the same distance) from the center of the sphere. It has a radius $r.$

A hemisphere is half of a sphere. The prefix “hemi” means half.

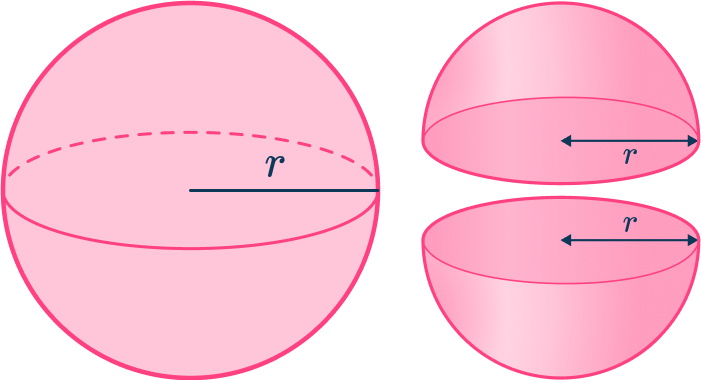
You may have come across the word hemisphere in the real life context of planet Earth. You can split the world into $2$ equal halves – the northern hemisphere and the southern hemisphere.

[FREE] 3D Shape Check for Understanding Quiz (Grade 1, 5 and 6)

Use this quiz to check your grade 1, 5 and 6 students’ understanding of 3D shape. 10+ questions with answers covering a range of 1st, 5th and 6th grade 3D shape topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] 3D Shape Check for Understanding Quiz (Grade 1, 5 and 6)

DOWNLOAD FREE

Properties of a hemisphere

A hemisphere is a geometric shape which has a curved surface area. The base of a hemisphere is a flat face, which is a circle.

A hemisphere has no vertices and one edge. It is not made up of polygons, so it is not a polyhedron.

Faces: $2$

Edges: $1$

Vertices: $0$

Volume of a hemisphere

The volume of a hemisphere is based on the volume of a sphere.

To calculate the volume of a sphere, where $r$ is the radius of the sphere, you use the formula:

$\text{Volume of a sphere}=\cfrac{4}{3} \pi r^3$

To find the volume of a hemisphere you halve the volume of a sphere.

Here is the volume of a hemisphere formula, with $r$ as the radius of the hemisphere:

$\text{Volume of a hemisphere}=\cfrac{4}{3} \pi r^3\div 2$

$\text{Volume of a hemisphere}=\cfrac{2}{3} \pi r^{3}$

Step-by-step guide: Volume of a hemisphere

Surface area of a hemisphere

The surface area of a hemisphere is based on the surface area of a sphere.

To calculate the surface area of a sphere with radius $r,$ you can use the formula

$\text{Surface area of a sphere}=4\pi{r}^{2}$

So to find the curved surface area of the hemisphere, you need to halve the surface area of the sphere.

$4\pi{r}^{2}\div 2=2\pi{r}^{2}$

However this would only give the curved surface area. If you want the total surface area, you need to add the area of the base of the hemisphere.

The area of the base, which is a circle, is given by $\pi r^{2}.$

Here is the surface area of a hemisphere formula, for a hemisphere with radius $r$

$\text{Total surface area of a hemisphere}=2\pi{r}^{2}+\pi{r}^{2}=3\pi{r}^{2}.$

Step-by-step guide: Surface area of a hemisphere

What is a hemisphere shape?

Common Core State Standards

How does this relate to high school math?

High School – Geometry – Geometric Measurement & Dimension (HS.G.GMD.A.2)
Give an informal argument using Cavalieri’s principle for the formulas for the volume of a sphere and other solid figures.

How to work out the volume or surface area of a hemisphere

In order to find the volume or surface area of a hemisphere:

Write down the formula for the sphere.
Adapt the formula for the question.
Substitute in the value.
Write the final answer.

Hemisphere shape examples

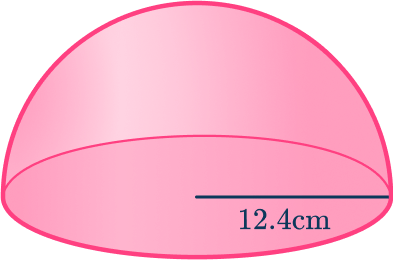
Example 1: volume

The radius of a hemisphere is $12.4\mathrm{~cm}.$ Find the volume of the hemisphere to the nearest whole number.

Write down the formula for the sphere.

You need to consider the formula for the volume of a sphere.

V=\cfrac{4}{3} \pi r^3

2Adapt the formula for the question.

The formula needs to be halved for the volume of a hemisphere.

V=\cfrac{4}{3} \pi r^3 \div 2

3Substitute in the value.

Then you substitute in the value $12.4$ in place of $r.$

V=\cfrac{4}{3} \pi (12.4)^3 \div 2

4Write the final answer.

The answer is $3993.223\dots$

This rounds to give $3993 \mathrm{~cm}^3$ (to the nearest whole number) as the volume of the hemisphere.

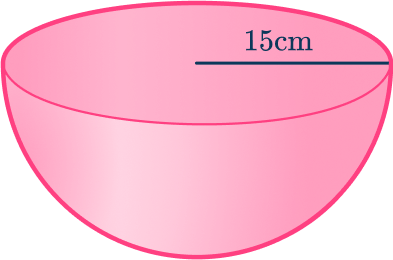
Example 2: volume in terms of π

Find the volume of a hemisphere with radius $15\mathrm{~cm}.$ Leave your answer in terms of $\pi.$

Write down the formula for the sphere.

You need to consider the formula for the volume of a sphere.

$V=\cfrac{4}{3} \pi r^3$

Adapt the formula for the question.

The formula needs to be halved for the volume of a hemisphere.

$V=\cfrac{4}{3} \pi r^3 \div 2$

Substitute in the value.

Then you substitute in the value $15$ in place of $r.$

$V=\cfrac{4}{3} \pi (15)^3 \div 2$

Write the final answer.

The volume of the hemisphere is $2250\pi \mathrm{~cm}^{3}.$

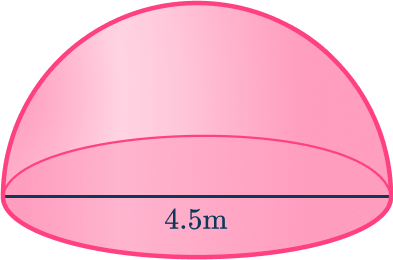
Example 3: curved surface area

Find the curved surface area of a hemisphere with diameter $4.5\mathrm{~m}.$ Give your answer to the nearest tenth.

Write down the formula for the sphere.

You need to consider the formula for the surface area of a sphere.

$\text{Surface Area}=4\pi{r}^{2}$

Adapt the formula for the question.

The formula needs to be halved for the curved surface area of a hemisphere.

$\text{Curved Surface Area}=4\pi{r}^{2}\div 2=2\pi{r}^{2}$

Substitute in the value.

You have been given a diameter $4.5.$ You need to divide the diameter by $2$ to get the radius. So you substitute in the value $2.25$ in place of $r.$

$\text{Curved Surface Area}=2\pi{(2.25)}^{2}$

Write the final answer.

The answer is $31.808\dots$

This rounds to give $31.8 \mathrm{~m}^2$ (to the nearest tenth) as the curved surface area of the hemisphere.

Example 4: total surface area

Find the total surface area of a hemisphere with radius $7.6\mathrm{~cm}.$ Give your answer to the nearest whole number.

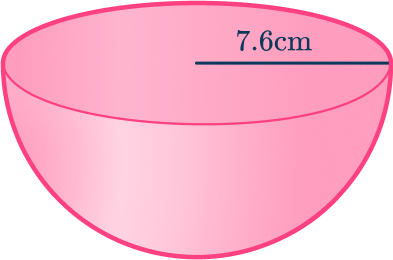
Write down the formula for the sphere.

You need to consider the formula for the surface area of a sphere.

$\text{Surface Area}=4\pi{r}^{2}$

Adapt the formula for the question.

The formula needs to be halved for the curved surface area of a hemisphere.

$\text{Curved Surface Area}=4\pi{r}^{2}\div 2=2\pi{r}^{2}$

But, you need to also add the area of the circular base to get the total surface area of the hemisphere.

$\text{Total Surface Area}=2\pi{r}^{2}+\pi{r}^{2}=3\pi{r}^{2}$

Substitute in the value.

Then you substitute in the value $7.6$ in place of $r.$

$\text{Total Surface Area}=3\pi{(7.6)}^{2}$

Write the final answer.

The answer is $544.375\dots$

This rounds to give $544 \mathrm{~cm}^2$ (to the nearest whole number) as the total surface area of the hemisphere.

Example 5: curved surface area in terms of π

Find the curved surface area of a hemisphere with radius $8\mathrm{~cm}.$ Leave your answer in terms of $\pi.$

Write down the formula for the sphere.

You need to consider the formula for the surface area of a sphere.

$\text{Surface Area}=4\pi{r}^{2}$

Adapt the formula for the question.

The formula needs to be halved for the curved surface area of a hemisphere.

$\text{Curved Surface Area}=4\pi{r}^{2}\div 2=2\pi{r}^{2}$

Substitute in the value.

Then you substitute in the value $8$ in place of $r.$

$\text{Curved Surface Area}=2\pi{(8)}^{2}$

Write the final answer.

The curved surface area of the hemisphere is $128\pi \mathrm{~cm}^{2}.$

Example 6: total surface area in terms of π

Find the total surface area of a hemisphere with diameter $10\mathrm{~cm}.$ Leave your answer in terms of $\pi.$

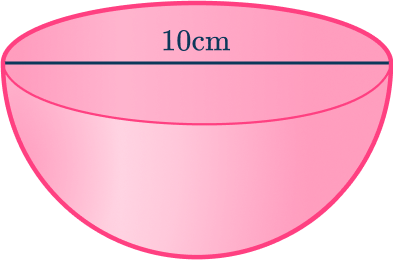
Write down the formula for the sphere.

You need to consider the formula for the surface area of a sphere.

$\text{Surface Area}=4\pi{r}^{2}$

Adapt the formula for the question.

The formula needs to be halved for the curved surface area of a hemisphere.

$\text{Curved Surface Area}=4\pi{r}^{2}\div 2=2\pi{r}^{2}$

But, you need to also add the area of the circular base to get the total surface area of the hemisphere.

$\text{Total Surface Area}=2\pi{r}^{2}+\pi{r}^{2}=3\pi{r}^{2}$

Substitute in the value.

You have been given a diameter $10.$ You need to divide the diameter by $2$ to get the radius. So you substitute in the value $5$ in place of $r.$

$\text{Total Surface Area}=3\pi{(5)}^{2}$

Write the final answer.

The total surface area of the hemisphere is $75 \pi \mathrm{~cm}^2.$

Teaching tips for hemisphere shape

Use physical models of spheres and hemispheres. Show everyday items like hemispherical bowls, domes, and igloos.

Incorporate problem-solving exercises and worksheets that require students to apply the formulas for volume of a hemisphere and surface area of a hemisphere in different contexts.

Hands-on activity: Have students create both solid and hollow hemisphere models using materials like clay for solid hemispheres and papier-mâché or plastic for hollow hemispheres. Let students weigh the solid and hollow models to understand the difference in mass. Have them calculate the volume and surface area for each type, reinforcing the mathematical concepts.

Easy mistakes to make

Using the incorrect formula
There are several formulas that can be used in geometry, so you need to match the correct formula to the correct context.

Mixing up total surface area and curved surface area
Double check whether the question requires you to find the curved surface area of a hemisphere or the total surface area of a hemisphere.

Rounding too soon
It is important to not round the answer until the end of the calculation. This will mean your final answer is accurate. It is useful to keep your answer in terms of $\pi$ until you round the answer at the very end of the question.

Using incorrect units
For area you use square units such as $\mathrm{cm}^2.$
For volume you use cube units such as $\mathrm{cm}^3.$

Mixing up the radius and the diameter
It is a common error to mix up radius and diameter. Remember, the radius is half of the diameter.

Practice hemisphere shape questions

3020 \mathrm{~cm}^3

3022 \mathrm{~cm}^3

6044 \mathrm{~cm}^3

6040 \mathrm{~cm}^3

You need to halve the formula for the volume of a sphere, and then substitute the value of $11.3$ in place of $r.$

\begin{aligned}V&=\cfrac{4}{3} \pi {r}^{3}\div 2 \\\\ V&=\cfrac{4}{3} \times \pi \times {11.3}^{3}\div 2 \\\\ V&=3021.996… \\\\\ V&=3022 \mathrm{~cm}^3 \\ & \text{(to the nearest whole number)} \end{aligned}

\cfrac{16}{3}\pi \mathrm{~cm}^3

\cfrac{32}{3}\pi \mathrm{~cm}^3

\cfrac{256}{3}\pi \mathrm{~cm}^3

\cfrac{128}{3}\pi \mathrm{~cm}^3

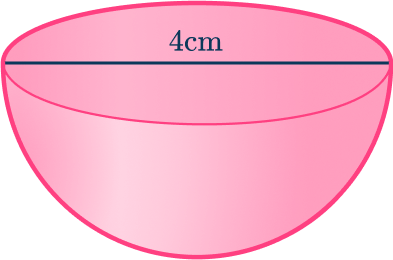
You have been given the diameter $4,$ which you need to divide by $2$ to get the radius. You need to halve the formula for the volume of a sphere, and then substitute the value of $2$ in place of $r.$

\begin{aligned}V&=\cfrac{4}{3} \pi {r}^{3}\div 2 \\\\ V&=\cfrac{4}{3} \times \pi \times {2}^{3}\div 2 \\\\ V&=\cfrac{16}{3}\pi \\\\ V&=\cfrac{16}{3}\pi \mathrm{~cm}^3 \end{aligned}

515 \mathrm{~cm}^2

1029 \mathrm{~cm}^2

1540 \mathrm{~cm}^2

2060 \mathrm{~cm}^2

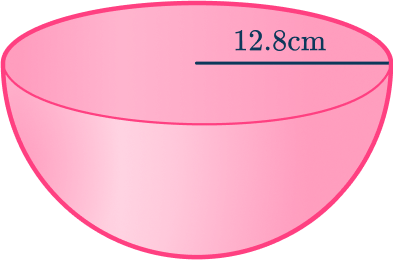
You need to halve the formula for the surface area of a sphere to find the curved surface area of a hemisphere (CSA). Then you substitute the value of $12.8$ in place of $r.$

\begin{aligned}CSA&=4 \pi {r}^{2}\div 2 \\\\ CSA&=2\pi {r}^{2} \\\\ CSA&=2\times \pi \times {12.8}^{2} \\\\ CSA&=1029.437… \\\\ CSA&=1029 \mathrm{~cm}^2 \\ & \text{(to the nearest whole number)} \end{aligned}

242\pi \mathrm{~cm}^2

121\pi \mathrm{~cm}^2

968\pi \mathrm{~cm}^2

44\pi \mathrm{~cm}^2

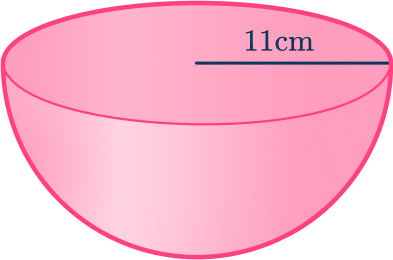
You need to halve the formula for the surface area of a sphere to find the curved surface area of a hemisphere (CSA). Then you substitute the value of $11$ in place of $r.$

\begin{aligned}CSA&=4 \pi {r}^{2}\div 2 \\\\ CSA&=2\pi {r}^{2} \\\\ CSA&=2\times \pi \times {11}^{2} \\\\ CSA&=242\pi \\\\ CSA&=242\pi \mathrm{~cm}^2 \end{aligned}

48400 \mathrm{~cm}^2

48300 \mathrm{~cm}^2

36300 \mathrm{~cm}^2

36200 \mathrm{~cm}^2

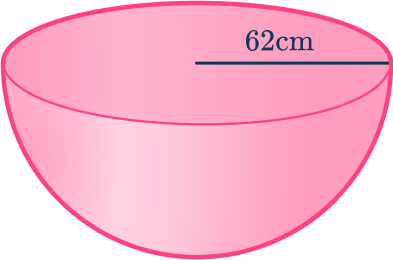
You need to halve the formula for the surface area of a sphere and add on the circular base to find the total surface area of a hemisphere (TSA). Then you substitute the value of $62$ in place of $r.$

\begin{aligned}TSA&=4 \pi {r}^{2}\div 2 + 2\pi {r}^{2} \\\\ TSA&=3\pi {r}^{2} \\\\ TSA&=3\times \pi \times {62}^{2} \\\\ TSA&=36228.8… \\\\ TSA&=36300 \mathrm{~cm}^2 \\ & \text{(to the nearest hundred)} \end{aligned}

225\pi \mathrm{~cm}^2

450\pi \mathrm{~cm}^2

675\pi \mathrm{~cm}^2

900\pi \mathrm{~cm}^2

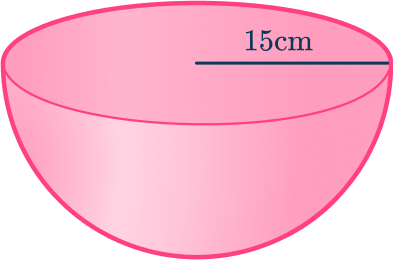
You need to halve the formula for the surface area of a sphere and add on the circular base to find the total surface area of a hemisphere (TSA). Then you substitute the value of $15$ in place of $r.$

\begin{aligned}TSA&=4 \pi {r}^{2}\div 2 + 2\pi {r}^{2} \\\\ TSA&=3\pi {r}^{2} \\\\ TSA&=3\times \pi \times {15}^{2} \\\\ TSA&=675\pi \\\\ TSA&=675\pi \mathrm{~cm}^2 \, \text{(to 3 sf)} \end{aligned}

Hemisphere shape FAQs

What is a hemisphere shape?

A hemisphere is a three-dimensional shape that represents half of a sphere. It is formed by cutting a sphere into two equal halves along a plane passing through its center.

What is the difference between the total surface area and the curved surface area of a hemisphere?

The total surface area of a hemisphere includes both the curved surface area and the area of the flat circular base, while the curved surface area (also called lateral surface area) includes only the curved part of the hemisphere.

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.