Volume of a Cone - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

3D shapes Cone Multiplying fractions

Math resources Geometry Volume

Volume of a cone

Here you will learn about the volume of a cone, including how to calculate the volume of a cone given its radius and perpendicular height and how to calculate a missing length within a cone, given its volume.

Students will first learn about the volume of a cone within geometry in $8$ th grade and will extend their learning throughout high school geometry.

What is the volume of a cone?

The volume of a cone is the amount of space inside a cone. A cone is a three-dimensional shape that has a flat surface and a curved surface that joins at a vertex.

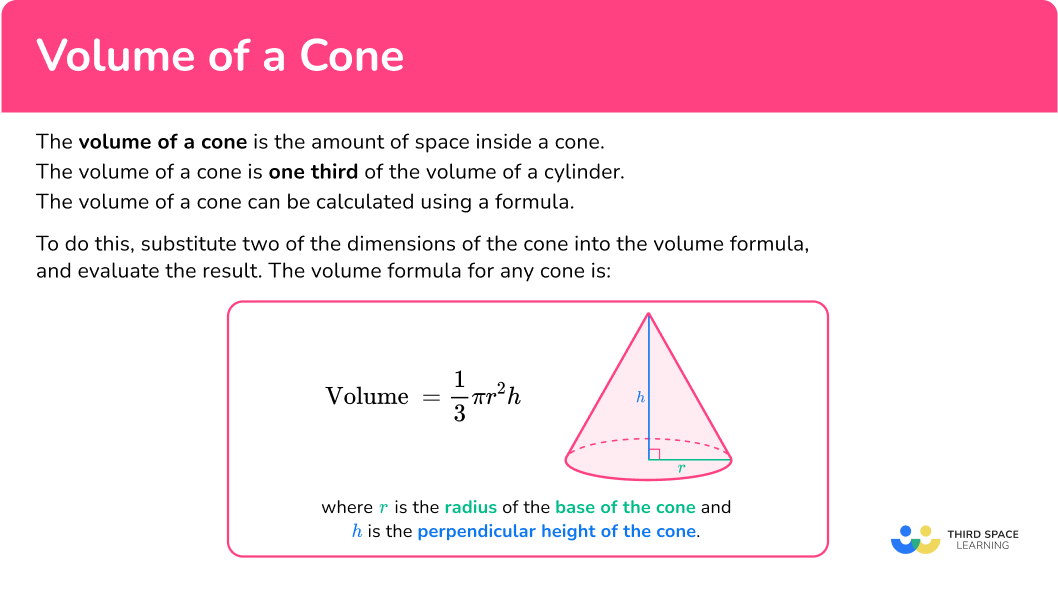
The volume of a cone is one third of the volume of a cylinder.

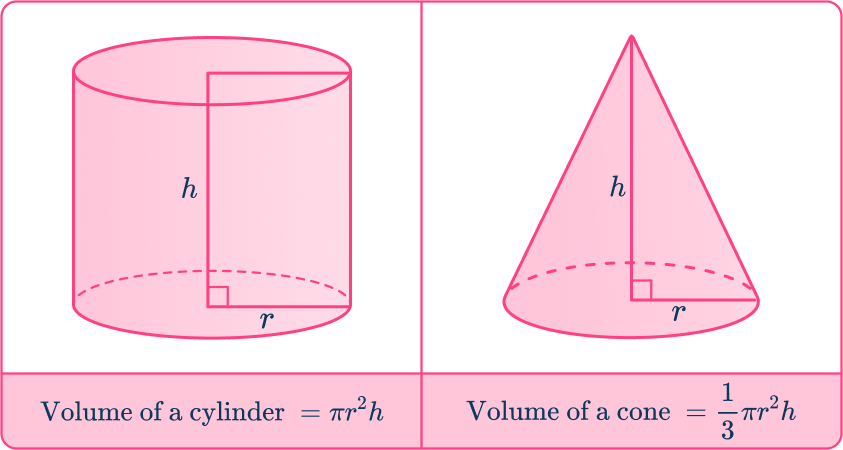
The volume of a cone can be calculated using a formula.

To do this, substitute two of the dimensions of the cone into the volume formula, and evaluate the result.

The volume formula for any cone is:

$\text{Volume}=\cfrac{1}{3} \pi r^2 h$

where $r$ is the radius of the base of the cone and $h$ is the perpendicular height of the cone.

For example,

Find the volume of this cone where the radius of the base of the cone is $5 \mathrm{~cm}$ and the perpendicular height of the cone is $8 \mathrm{~cm}.$

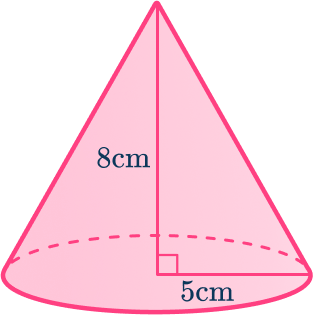
You will find the area of the circular base of a cone using $A=\pi r^2$ (the area of a circle).

Then multiply it by the vertical height, $h,$ and find one third of the answer.

\begin{aligned} \text{Volume}&=\cfrac{1}{3} \pi r^2 h \\\\ &= \cfrac{1}{3} \times \pi \times 5^2 \times 8 \\\\ &=\cfrac{200}{3}\pi \\\\ &=209.4 \, cm^3 \ \text{(1 dp)} \end{aligned}

[FREE] Volume Worksheet (Grade 6 to 8)

Use this quiz to check your grade 6 to 8 students’ understanding of volume. 10+ questions with answers covering a range of 6th, 7th and 8th grade volume topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Volume Worksheet (Grade 6 to 8)

DOWNLOAD FREE

Types of cones

A cone is a three dimensional object with a circular base that tapers to a point (a vertex). This vertex is also known as the apex.

If the apex is directly above the center of the base, the cone is referred to as a right circular cone. If the apex is not above the center of the base, this is referred to as an oblique cone.

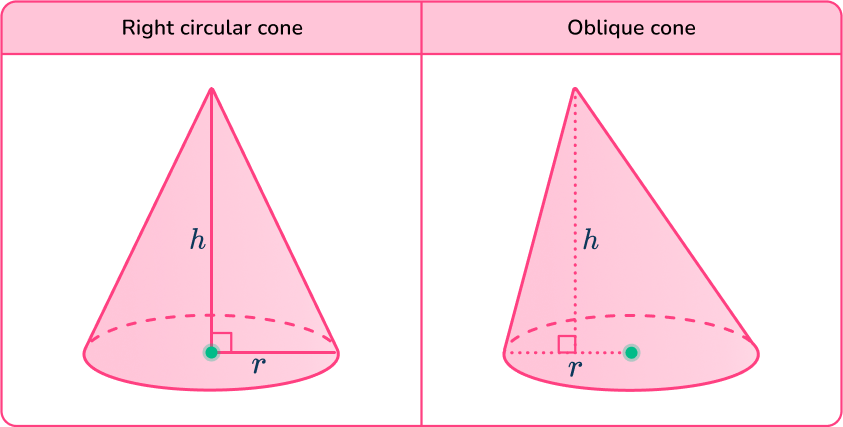
Note: The slant height of the cone $l$ is not needed to calculate the volume of a cone, but it is used to calculate the surface area of a cone.

See also: Surface area of a cone

The volume of cone stays the same if the apex moves parallel to the base but the surface area of the cone changes. This is because the slant heights are not equal for an oblique cone.

What is the volume of a cone?

Common Core State Standards

How does this relate to $8$ th grade and high school math?

Grade 8: Geometry (8.G.C.9)
Know the formulas for the volumes of cones, cylinders, and spheres and use them to solve real-world and mathematical problems.

High school: Geometry (HS.G.GMD.A.3)
Use volume formulas for cylinders, pyramids, cones, and spheres to solve problems.

How to calculate the volume of a cone

In order to calculate the volume of a cone:

Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$
Substitute the given values.
Work out the calculation.
Write the final answer, including the units.

Volume of a cone examples

Example 1: integer dimensions

Find the volume of the cone with radius $4 \mathrm{~cm}$ and perpendicular height $7 \mathrm{~cm}.$

Give your answer to $3$ significant figures.

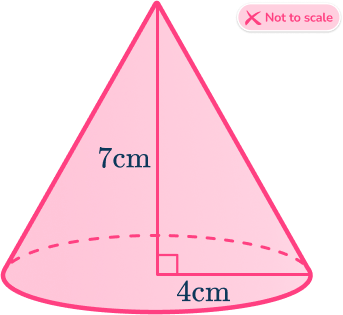
Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The formula for the volume of a cone is:

V=\cfrac{1}{3} \pi r^2 h

2Substitute the given values.

Substitute the values of the radius, $r$ , and the perpendicular height, $h$ , into the formula.

V= \cfrac{1}{3}\times\pi\times{4^2}\times{7}

3Work out the calculation.

Use a calculator to work out the volume.

V=\cfrac{112}{3}\pi = 117.286…

4Write the final answer, including the units.

Here you are asked to give the answer to $3$ significant figures.

V=117.286 \ldots=117 \mathrm{~cm}^3 \, (3sf)

The volume of the cone is $117 \mathrm{~cm}^3 \, (3sf).$

Example 2: decimal dimensions

Find the volume of the cone with radius $2.1 \mathrm{~cm}$ and perpendicular height $4.3 \mathrm{~cm}.$

Give your answer to $3$ significant figures.

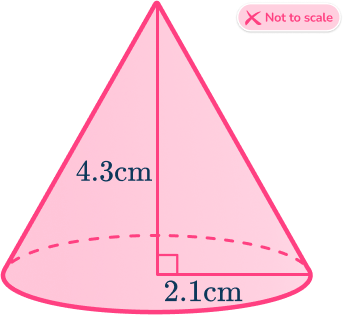
Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The volume formula of a cone is:

$V=\cfrac{1}{3} \pi r^2 h$

Substitute the given values.

Substitute the values of the radius, $r$ , and the perpendicular height, $h$ , into the formula.

$V= \cfrac{1}{3} \times \pi \times 2.1^2 \times 4.3$

Work out the calculation.

Use a calculator to work out the volume.

$V= 19.8580\dots$

Write the final answer, including the units.

Here you are asked to give the answer to $3$ significant figures.

$V=19.8580 \ldots=19.9 \mathrm{~cm}^3 \, (3sf)$

The volume of the cone is $19.9 \mathrm{~cm}^3 \, (3sf).$

Example 3: calculate the volume in terms of π

Calculate the volume of a cone with radius $3 \mathrm{~cm}$ and perpendicular height $5 \mathrm{~cm}.$

Write your answer in terms of $\pi.$

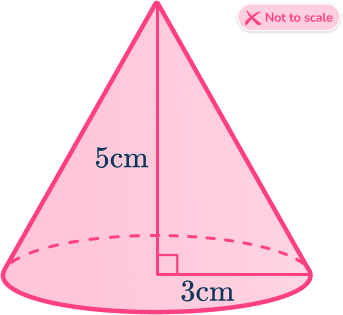
Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The formula for the volume of a cone is:

$V=\cfrac{1}{3} \pi r^2 h$

Substitute the given values.

Substitute the values of the radius, $r$ , and the perpendicular height, $h$ , into the formula.

$V= \cfrac{1}{3} \times \pi \times 3^2 \times 5$

Work out the calculation.

Because you can change the order of a multiplication and the solution stays the same, we have:

$V=\cfrac{1}{3}\times\pi\times{3^{2}}\times{5}=\cfrac{1}{3}\times{3^{2}}\times{5}\times{\pi}$

Simplifying this, you get:

$V=15\pi$

Write the final answer, including the units.

Here you need to leave the answer in terms of $\pi.$

$V=15\pi \mathrm{~cm}^3$

The volume of the cone is $15 \pi \mathrm{~cm}^3.$

Example 4: calculate the volume given the diameter

Find the volume of the cone with the diameter of $4 \mathrm{~cm}$ and perpendicular height $6 \mathrm{~cm}.$

Write your answer in terms of $\pi.$

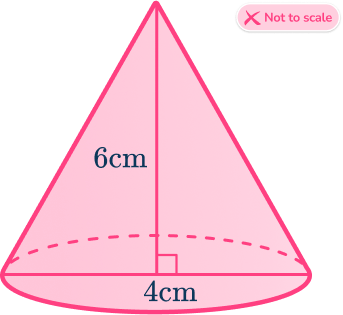
Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The formula for the volume of a cone is:

$V=\cfrac{1}{3} \pi r^2 h$

Substitute the given values.

As the diameter is twice the length of the radius, the radius is equal to $4 \div 2=2 \mathrm{~cm}.$

Substitute the values of $r$ and $h$ into the formula to get:

$V= \cfrac{1}{3} \times \pi \times 2^2 \times 6$

Work out the calculation.

Because you can change the order of a multiplication and the solution stays the same, we have:

$V=\cfrac{1}{3}\times\pi\times{2^{2}}\times{6}=\cfrac{1}{3}\times{2^{2}}\times{6}\times{\pi}$

Simplifying this, you get:

$V= 8\pi$

Write the final answer, including the units.

Here you are asked to leave the answer in terms of $\pi.$

$V=8\pi \mathrm{~cm}^3$

The volume of the cone is $8 \pi \mathrm{~cm}^3.$

Example 5: calculate a length given the volume

A cone has a volume of $500 \mathrm{~cm}^3$ and a radius of $8 \mathrm{~cm}.$ Calculate the perpendicular height of the cone, correct to $1$ decimal place.

Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The volume formula of a cone is:

$V=\frac{1}{3} \pi r^2 h$

Substitute the given values.

You are given the volume and the radius, so substitute these into the formula.

$500=\cfrac{1}{3} \times \pi \times 5^2 \times h$

As $5^2=25,$ we have:

$500=\cfrac{1}{3} \times \pi \times{25}\times h$

Work out the calculation.

Solve the equation for $h\text{:}$

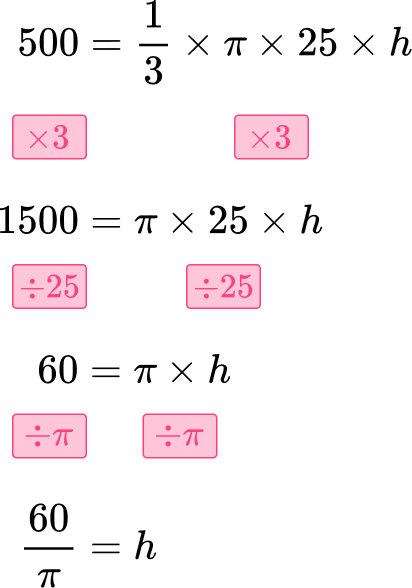
Write the final answer, including the units.

Here you are asked to give the answer to $1$ decimal place.

$h=\cfrac{60}{\pi}=19.1 \mathrm{~cm} \, (1 \, dp)$

The perpendicular height of the cone is: $19.1 \mathrm{~cm} \, (\text{to} \, 1 \, \text{dp})$ .

Example 6: calculate the radius given the volume

A cone has a volume of $800 \mathrm{~cm}^3$ and a perpendicular height of $15 \mathrm{~cm}.$ Calculate the radius of the cone. Give your answer to $2$ decimal places.

Write down the formula: $\bf{\textbf{Volume }=\cfrac{1}{3} \, \pi \textbf{r}^2 \textbf{h.}}$

The question involves the volume of a cone, so use the formula for the volume of a cone.

$V=\cfrac{1}{3} \pi r^2 h$

Substitute the given values.

You are given the volume and the perpendicular height, so substitute these into the formula.

$800=\cfrac{1}{3} \times \pi \times r^2 \times 15$

Work out the calculation.

As $\cfrac{1}{3} \times 15=5,$ simplify the right hand side of the equation, before finding the value of $r\text{:}$

$r^2=\cfrac{160}{\pi}$

To find the value of $r,$ square root both sides of the equation. By doing this, you get:

$r=\sqrt{\cfrac{160}{\pi}}=7.136…$

Write the final answer, including the units.

Here you are asked to give the answer to $2$ decimal places.

$r=7.136 \ldots=7.14 \mathrm{~cm} \; (2 \, dp)$

The radius of the cone is $7.14 \mathrm{~cm} \; (2 \, dp).$

Teaching tips for volume of a cone

Using visual representations is a great way for students to grasp what a cone really is. The use of diagrams and real world examples, like an ice cream cone is a way to make the concept relatable.

Allow students to work through examples on worksheets with a partner or in small groups and have them collaborate on how to answer each question. These conversations can be powerful in deepening student understanding.

Students can use a volume of a cone calculator or similar technology tool to check their work real time.

Easy mistakes to make

Using the wrong formula
There are different formulas when finding the volume of different 3D shapes. Using the wrong formula will result in an inaccurate answer. It’s important to be familiar with the different formulas to keep this from happening.

Not waiting to round the final answer
It is important to not round the answer until the end of the calculation. This will mean your final answer is accurate.

Unfamiliar with the difference between the radius and the diameter
It is important to be familiar with the difference between the radius and diameter, in order to get accurate answers. The diameter is the measure across a circle, and the radius is half of that.

Using the correct units
When finding the volume, you will need to use cubic units. For example, cubic inches, cubic feet and cubic inches.

Practice volume of a cone questions

541 \, cm^3

542 \, cm^3

719 \, cm^3

718 \, cm^3

Substitute the values of $r$ and $h$ into the formula.

\begin{aligned}V&=\cfrac{1}{3} \pi r^2 h \\\\ V&=\cfrac{1}{3}\times \pi \times 7.3^2 \times 9.7 \\\\ V&=541.310… \\\\ V&=541 \ cm^3 \ \text{(3sf)} \end{aligned}

562 \, cm^3

563 \, cm^3

611 \, cm^3

612 \, cm^3

Substitute the values of $r$ and $h$ into the formula.

\begin{aligned}V&=\cfrac{1}{3}\pi r^2 h \\\\ V&=\cfrac{1}{3}\times \pi \times 8.6^2 \times 7.9 \\\\ V&=611.860… \\\\ V&=612 \ cm^3 \ \text{(3sf)} \end{aligned}

42\pi \, cm^3

84\pi \, cm^3

126\pi \, cm^3

252\pi \, cm^3

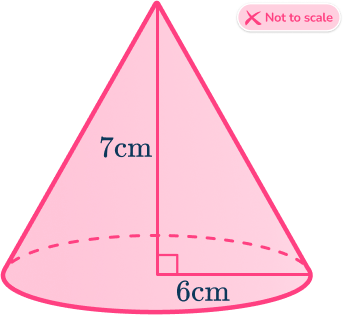
To find the volume of a cone, substitute the values of $r$ and $h$ into the formula.

\begin{aligned}V&=\cfrac{1}{3}\pi r^2 h \\\\ V&=\cfrac{1}{3}\times \pi \times 6^2 \times 7 \\\\ V&=84\pi \\\\ V&=84\pi \, cm^3 \end{aligned}

12\pi \, cm^3

32\pi \, cm^3

48\pi \, cm^3

96\pi \, cm^3

Calculate the value of the radius by dividing the diameter by $2,$ then substitute the values of $r$ and $h$ into the formula and solve.

r=8 \div 2=4 \mathrm{~cm}

\begin{aligned}V&=\cfrac{1}{3} \pi r^2 h \\\\ V&=\cfrac{1}{3}\times \pi \times 4^2 \times 6 \\\\ V&=32\pi \\\\ V&=32\pi \ cm^3 \end{aligned}

3.5 \mathrm{~cm}

8.2 \mathrm{~cm}

10.6 \mathrm{~cm}

11.1 \mathrm{~cm}

Substitute the volume and the radius into the volume formula and then rearrange to find the perpendicular height, $h.$

\begin{aligned}&V=\cfrac{1}{3} \pi r^2 h \\\\ &400=\cfrac{1}{3}\times \pi \times 6^2 \times h \\\\ &1200=\pi \times 36 \times h \\\\ &\cfrac{1200}{\pi \times 36}=h \\\\ &h=10.610… \\\\ &h=10.6 \ cm \ \text{(1dp)} \end{aligned}

5.40 \mathrm{~cm}

5.72 \mathrm{~cm}

9.14 \mathrm{~cm}

9.28 \mathrm{~cm}

Substitute the values of the volume and the perpendicular height into the formula, and rearrange to find the radius $r.$

\begin{aligned}&V=\cfrac{1}{3} \pi r^2 h \\\\ &700=\cfrac{1}{3}\times \pi \times r^2 \times 8 \\\\ &2100=\pi \times r^2 \times 8 \\\\ &\cfrac{1200}{\pi \times 8}=r^2 \\\\ &r=\sqrt{\cfrac{2100}{\pi \times 8}} \\\\ &r=9.1409… \\\\ &r=9.14 \ cm \ \text{(to 2 dp)} \end{aligned}

Volume of a cone FAQs

What does it mean to find the volume of a cone?

Finding the volume of a cone means finding the amount of space within the 3D shape, or how much material or substance can be inside of the shape. It can be thought of as how much water or sand could be inside the cone.

How do you find the surface area of a cone?

To find the surface area of a cone, you would use the formula, $A=\pi r^2+\pi r l,$ where $A$ is the surface area of the cone, $r$ is the radius of the base of the cone and $l$ is the slant height of the cone.

What is the frustum of a cone?

A frustum of a cone is a geometric solid that is the result of cutting a cone with a plane parallel to its base. It is similar to what you would think of when you cut off the top of a cone. You would be able to calculate the volume and surface area of both solids.

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.