Surface Area of a Cone - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Area of a circle Right triangle Right angle Hypotenuse

Math resources Geometry Surface area

Surface area of a cone

Here you will learn about the surface area of a cone, including how to find the surface area given the radius of a cone and the slant height.

Students will first learn about the surface area of a cone as a part of geometry in $8$ th grade.

What is the surface area of a cone?

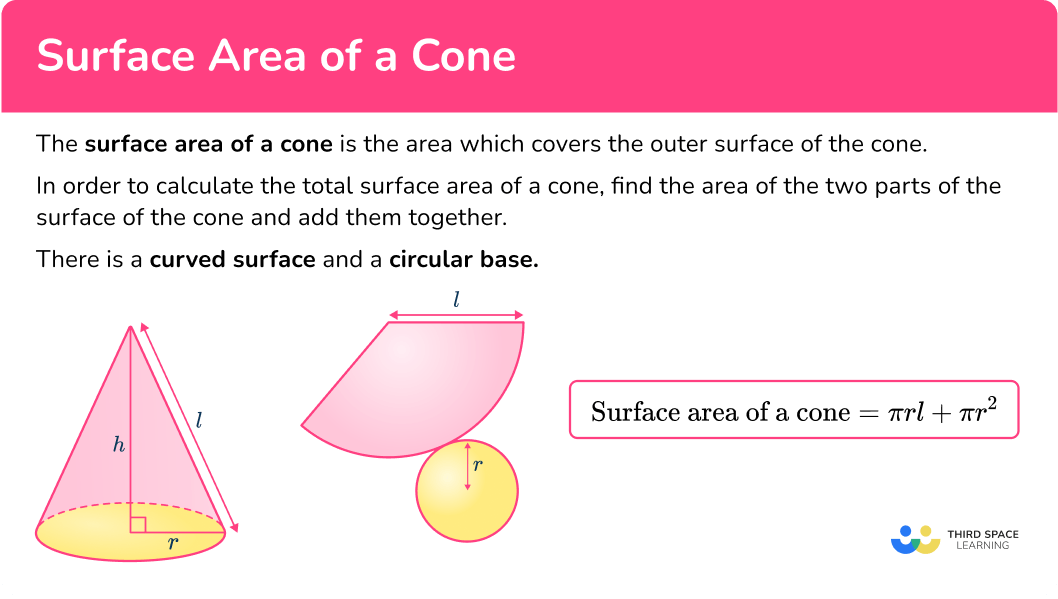
The surface area of a cone is the area which covers the outer surface of the cone.

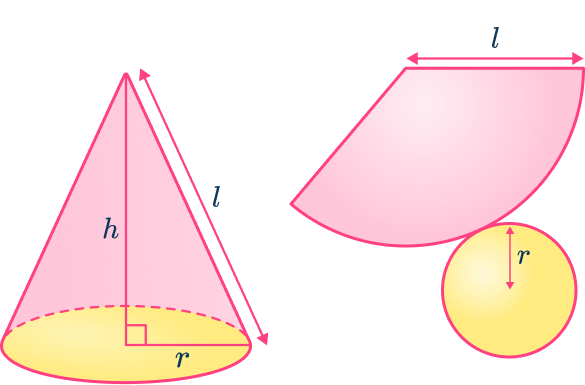
For any cone, $r$ is the radius of the base of the cone, $l$ is the slant height of the cone, and $h$ is the perpendicular height of the cone.

In order to calculate the total surface area of a cone (tsa), find the area of the two parts of the surface of the cone and add them together.

There is a curved surface area (CSA) and a circular base. The curved surface area of a cone is sometimes referred to as the lateral surface area of a cone (lsa).

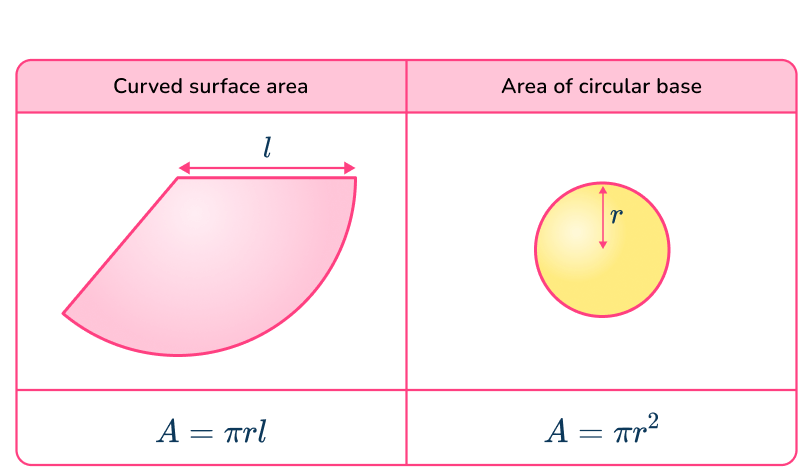
The surface area of a cone is the area of the two parts added together:

$\text{Surface area of a cone}=\pi{r}{l}+\pi{r^{2}}$

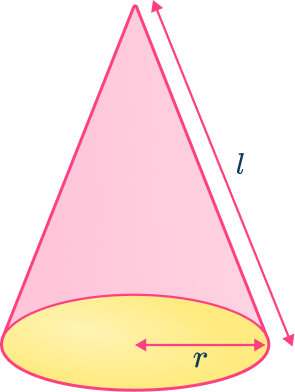
The perpendicular height of a cone $h$ is not required to calculate the surface area of a cone, although it is used to calculate the volume of the cone.

Step by step guide: Volume of a cone

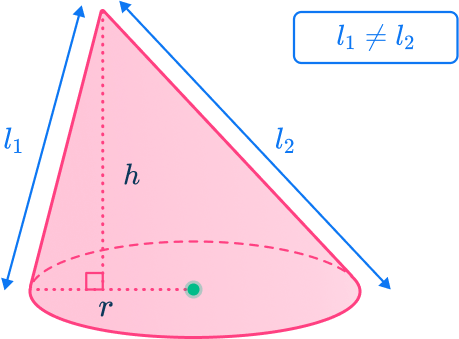
Note: You cannot use the formula for the surface area of a cone written above for an oblique cone. This is because the curved surface area does not have a constant slant height $l$ from the apex (a vertex) to the circular base.

Example

Calculate the surface area of the cone below to $1$ decimal place.

\text{Curved surface area}=\pi{rl}=\pi\times{6}\times{8}=48\pi

\text{Area of circular base }=\pi{r^{2}}=\pi\times{6^{2}}=36\pi

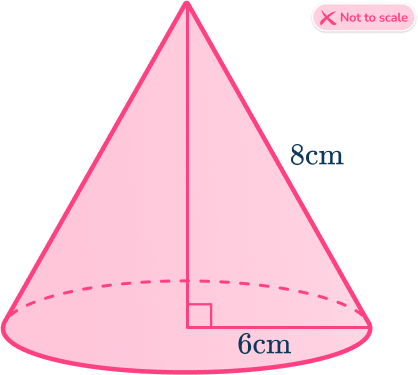
Add these together to find the total surface area of the cone:

\text { Surface area of the cone }=48 \pi+36 \pi=84 \pi=263.9 \mathrm{~cm}^2 \, (1 d p)

What is the surface area of a cone?

Common Core State Standards

How does this relate to $8$ th grade math?

Grade 8: Geometry (8.G.C.9)
Know the formulas for the volumes of cones, cylinders, and spheres and use them to solve real-world and mathematical problems.

[FREE] Surface Area Worksheet (Grade 6)

Use this quiz to check your grade 6 students’ understanding of surface area. 10+ questions with answers covering a range of 6th grade surface area topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Surface Area Worksheet (Grade 6)

Use this quiz to check your grade 6 students’ understanding of surface area. 10+ questions with answers covering a range of 6th grade surface area topics to identify areas of strength and support!

DOWNLOAD FREE

How to calculate the surface area of a cone

In order to calculate the surface area of a cone:

Find the area of each face.
Add the areas together.
Write the answer, including the units.

Surface area of a cone examples

Example 1: curved surface area

Find the curved surface area of the cone below. The radius of the cone is $3 \mathrm{~cm}$ and the slant height is $7 \mathrm{~cm}.$ Write your answer to $1$ decimal place.

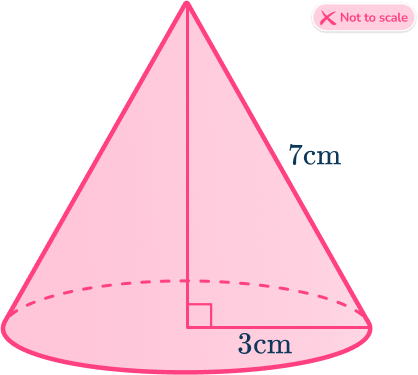
Find the area of each face.

You will only calculate the area of the curved surface for this question.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 3 \times 7 \\\\ &=21\pi \end{aligned}

2Add the areas together.

Because you are calculating the area of the curved surface, you can move on to step 3.

3Write the answer, including the units.

Round the answer to $1$ decimal place and include the units.

21 \pi=66.0 \, (1 d p)

The curved surface area of the cone is $66.0 \mathrm{~cm}^2 \, (1 d p).$

Note: If the question asks for $1$ decimal place, the answer must have $1$ decimal place, even if the digit is $0.$

Example 2: total surface area

Find the total surface area of the cone below to $1$ decimal place.

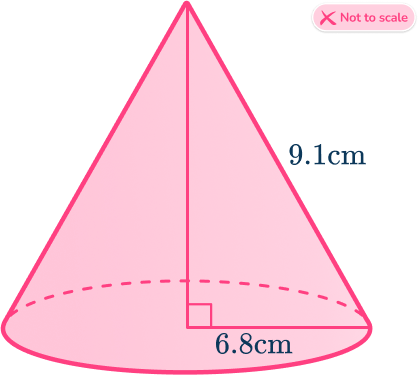
Find the area of each face.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 6.8 \times 9.1 \\\\ &=\cfrac{1547}{25}\pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 6.8^2 \\\\ &=\cfrac{1156}{25}\pi \end{aligned}

Add the areas together.

The sum of the areas is:

$\cfrac{1547}{25}\pi+\cfrac{1156}{25}\pi=\cfrac{2703}{25}\pi$

Write the answer, including the units.

Write the answer to $1$ decimal place and with square units.

$\cfrac{2703}{25}\pi=339.7 \, (1 d p)$

The total surface area of the cone is $339.7 \mathrm{~cm}^2 \, (1 d p).$

Example 3: calculate the surface area in terms of π

A chip shop sells mushy peas in a conical pot. The lid has a radius of $7 \mathrm{~cm},$ and the pot has a slant height of $10 \mathrm{~cm}.$ Calculate the outside surface area of the closed pot, including the lid. Write your answer in terms of $\pi.$

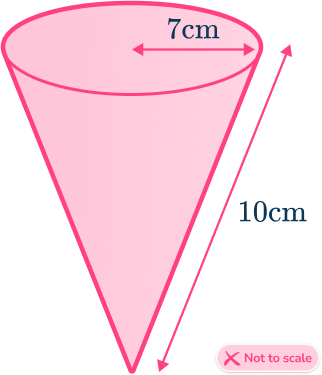
Find the area of each face.

\begin{aligned}\text{Curved surface area (the pot)}&=\pi rl \\\\ &=\pi \times 7 \times 10 \\\\ &=70 \pi \end{aligned}

\begin{aligned}\text{Area of a circle (the lid)}&=\pi r^2 \\\\ &=\pi \times 7^2 \\\\ &=49 \pi \end{aligned}

Add the areas together.

The sum of the areas is $70\pi+49\pi =119\pi$

Write the answer, including the units.

Write the answer in terms of $\pi,$ and state the units.

The total surface area of the cone is: $119 \pi \mathrm{~cm}^2$

Example 4: surface area in terms of π

The roof of a tower is a solid cone. The radius of the circular base is $4.5 \mathrm{~m},$ and the roof has a slope length of $8.3 \mathrm{~m}.$ Calculate the surface area of the roof. Write your answer in the form $\cfrac{a}{b}\pi$ where $a$ and $b$ are integers with no common factors.

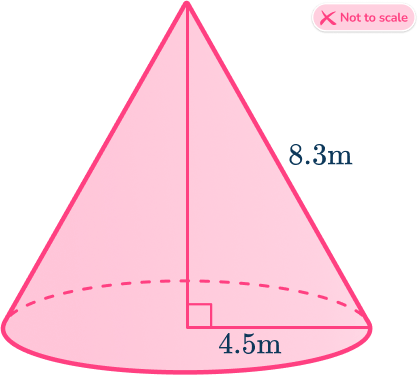
Find the area of each face.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 4.5 \times 8.3 \\\\ &=\cfrac{747}{20}\pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 4.5^2 \\\\ &=\cfrac{81}{4}\pi \end{aligned}

Add the areas together.

The sum of the areas is equal to:

$\cfrac{747}{20}\pi+\cfrac{81}{4}\pi=\cfrac{747}{20}\pi+\cfrac{405}{20}\pi=\cfrac{1152}{20}\pi$

Write the answer, including the units.

The answer needs to be written in the form $\cfrac{a}{b}\pi$ where $a$ and $b$ are integers with no common factors. By simplifying the fraction fully, you get $\cfrac{1152}{20}\pi=\cfrac{288}{5}\pi.$

The surface area of the solid cone is $\cfrac{288}{5}\pi \text{ m}^{2}.$

Example 5: calculate the surface area given the diameter

Calculate the surface area of a cone with a base diameter of $4 \mathrm{~cm}$ and a slant height of $6 \mathrm{~cm}.$ Give your answer to $3$ significant figures.

Find the area of each face.

Calculate the radius of the circular base first. As the diameter is twice the radius of a circle, divide the diameter of the circle by $2.$

$4 \div 2=2 \mathrm{~cm}$

You are left with $r=2 \mathrm{~cm}.$

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 2 \times 6 \\\\ &=12\pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 2^2 \\\\ &=4\pi \end{aligned}

Add the areas together.

Add the two areas together.

$12 \pi+4 \pi=16 \pi$

Write the answer, including the units.

Write the answer to $3$ significant figures, and state the units.

$16\pi=50.3 \, (3sf)$

The surface area of the cone is $50.3 \mathrm{~cm}^2 \, (3sf).$

Example 6: curved surface area given the perpendicular height

A cone has a perpendicular height of $4 \mathrm{~cm},$ and a circular base with a radius of $3 \mathrm{~cm}.$ The apex of the cone is directly above the center of the circular base. Calculate the area of the curved surface in terms of $\pi.$

Find the area of each face.

Find the slant height of the cone. Sketching a diagram of the cone with the dimensions given:

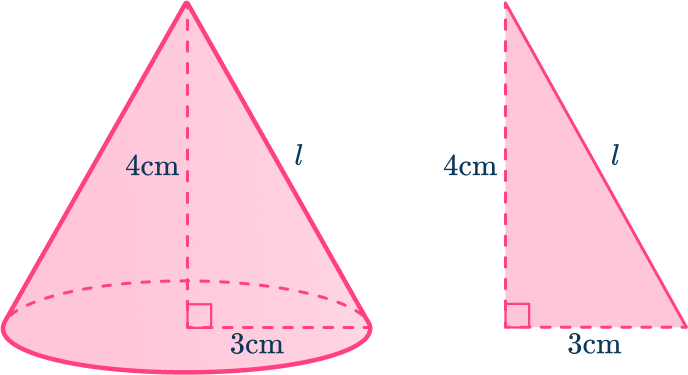
To find the slant height of a cone, $l,$ use Pythagoras’ theorem:

$a^2+b^2=c^2.$

Given that $a=4 \mathrm{~cm}, b=3 \mathrm{~cm},$ and $c=l,$ you have:

\begin{aligned}& 4^2+3^2=l^2 \\\\ & 16+9=l^2 \\\\ & l^2=25 \\\\ & l=5 \mathrm{~cm} \end{aligned}

With the value for the slant height, you can find the curved surface area.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 3 \times 5 \\\\ &=15\pi \end{aligned}

Add the areas together.

Because you are calculating the area of the curved surface, you can move on to step $3.$

Write the answer, including the units.

Leave the answer in terms of $\pi$ and include the units.

The curved surface area of the cone is $15 \pi \mathrm{~cm}^2.$

Teaching tips for surface area of a cone

Link finding the surface area of cones to finding the area and surface area of other geometric shapes students may be familiar with. The formula for finding the area of the circle of the lateral surface area of a cylinder have parallels with the formula for surface area.

Provide multiple opportunities for students to practice finding the surface area of a cone, including worksheets, games or the use of interactive online tools. Allow students to work with small groups and provide frequent feedback to help improve understanding.

Use of a problem solving strategy can help students tackle word problems. Model and prompt students to identify and label important information within the word problem before attempting to use the formula.

Easy mistakes to make

Forgetting to add the base
Make sure to include the area of the base when calculating the total surface area. The formula includes the curved surface and the area of the base.

Forgetting to simplify radicals
The formula for the curved surface (lateral surface area of the cone) often involves square roots. Keep an eye out for when you need to do this so you don’t leave the answers unsimplified.

Mixing up the slant height with the height
The slant height is the distance from the tip of the cone to any point on the circumference of the base and the height is the perpendicular distance from the base to the tip. Make sure to use the correct height value when calculating the total surface area of the cone.

Practice surface area of a cone questions

120 \mathrm{~cm}^2

376.991 \mathrm{~cm}^2

377 \mathrm{~cm}^2

3770 \mathrm{~cm}^2

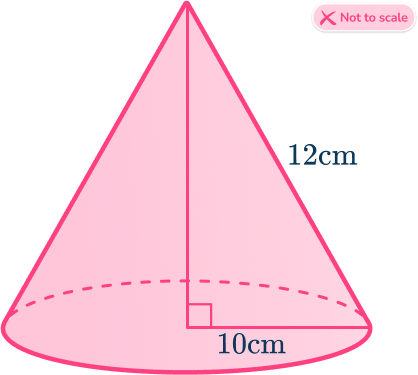
To find the curved surface area of a cone, substitute the value of $r$ and $l$ into the formula.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 12\times 10 \\\\ &=120\pi \\\\ &=376.9911184…\\\\ &=377 \ cm^2 \, \text{(3sf)} \end{aligned}

74.9 \mathrm{~mm}^2

117 \mathrm{~mm}^2

235 \mathrm{~mm}^2

235.242 \mathrm{~mm}^2

To find the curved surface area of a cone, substitute the value of $r$ and $l$ into the formula.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 7.8\times 9.6 \\\\ &=235.2424579… \\\\ &=235 \, \text{cm}^2 \, \text{(3sf)} \end{aligned}

138.2 \mathrm{~cm}^2

88.0 \mathrm{~cm}^2

117.3 \mathrm{~cm}^2

113.1 \mathrm{~cm}^2

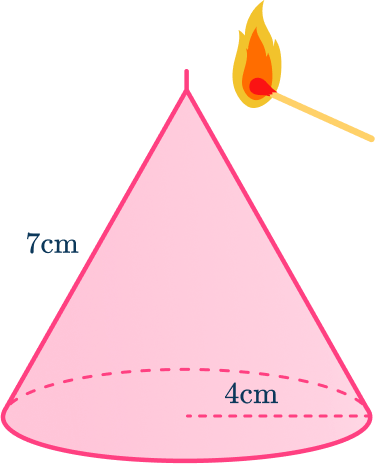
To find the total surface area of a cone, find the curved surface area and add on the area of the circular base.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 4 \times 7 \\\\ &= 28 \pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 4^2 \\\\ &=16 \pi \end{aligned}

Total surface area: $28\pi +16\pi =44\pi$

44\pi=138.2\text{ cm}^{2} \, (1 d p)

\cfrac{1027}{10}\pi\text{ cm}^{2}

\cfrac{5239}{40}\pi\text{ cm}^{2}

\cfrac{17407}{40}\pi\text{ cm}^{2}

\cfrac{1469}{20}\pi\text{ cm}^{2}

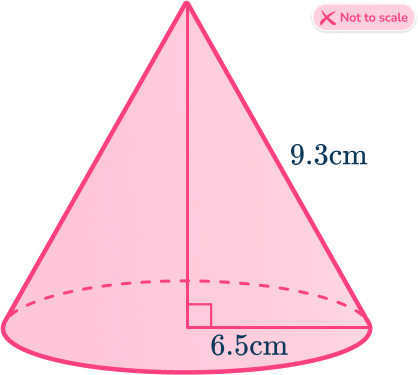
To find the total surface area of a cone, find the curved surface area and add on the area of the circular base.

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 6.5\times 9.3 \\\\ &=\cfrac{1209}{20}\pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 6.5^2 \\\\ &=\cfrac{169}{4}\pi \end{aligned}

Total surface area:

\cfrac{1209}{20}\pi+\cfrac{169}{4}\pi=\cfrac{1207}{10}\pi

Surface area is equal to $\cfrac{1207}{10} \pi \mathrm{~cm}^2.$

88 \pi \mathrm{~cm}^2

176 \pi \mathrm{~cm}^2

276 \pi \mathrm{~cm}^2

\cfrac{704}{3}\pi \mathrm{~cm}^2

The diameter is twice the length of the radius and so $r=16 \div 2=8 \mathrm{~cm}.$

Substituting the values for $r$ and $l$ into the formula for the curved surface area you get,

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 8\times 11 \\\\ &=88\pi \\\\ &=88\pi \mathrm{~cm}^2 \end{aligned}

94.2 \mathrm{~cm}^2

283 \mathrm{~cm}^2

188 \mathrm{~cm}^2

314 \mathrm{~cm}^2

Use Pythagoras’ theorem to calculate the slant height of the cone, then determine the surface area.

Pythagoras’ theorem: $a^{2}+b^{2}=c^{2}$

\begin{aligned}a^{2}+b^{2}&=c^{2} \\\\ 5^{2}+12^{2}&=l^{2} \\\\ 25+144&=l^{2} \\\\ l^{2}&=169 \\\\ l&=\sqrt{169} \\\\ l&=13\, cm \end{aligned}

The slant height $l=13 \, cm.$

\begin{aligned}\text{Curved surface area}&=\pi rl \\\\ &=\pi \times 5\times 13 \\\\ &=65\pi \end{aligned}

\begin{aligned}\text{Area of circular base }&=\pi r^2 \\\\ &=\pi \times 5^2 \\\\ &=25\pi \end{aligned}

Total surface area: $65\pi+25\pi=90\pi=283 \, \text{(3sf)}$

The total surface area of the cone is $283 \mathrm{~cm}^2 \, \text{(3sf)}$

Surface area of a cone FAQs

What is a cone?

A cone is a 3D geometric shape that tapers from a flat base to a point called the apex. The base of a cone is typically a circle, but not always.

How do you find the surface area of a right circular cone?

Finding the surface area of a right circular cone is the same as finding the surface area of any cone. You will first find the area of the curved surface using the formula $=\pi r l.$ You will add the answer of the area of the curved surface to the area of the base, using the formula $=\pi r^2.$

The next lessons are

Pythagorean theorem
Trigonometry
Circle math

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.