High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
Interior angles of a polygon
Here you will learn about interior angles of a polygon, including how to calculate the sum of interior angles for a polygon, single interior angles, and how to use this knowledge to solve problems.
Students will first learn about interior angles of a polygon as part of geometry in high school.
What are interior angles of a polygon?
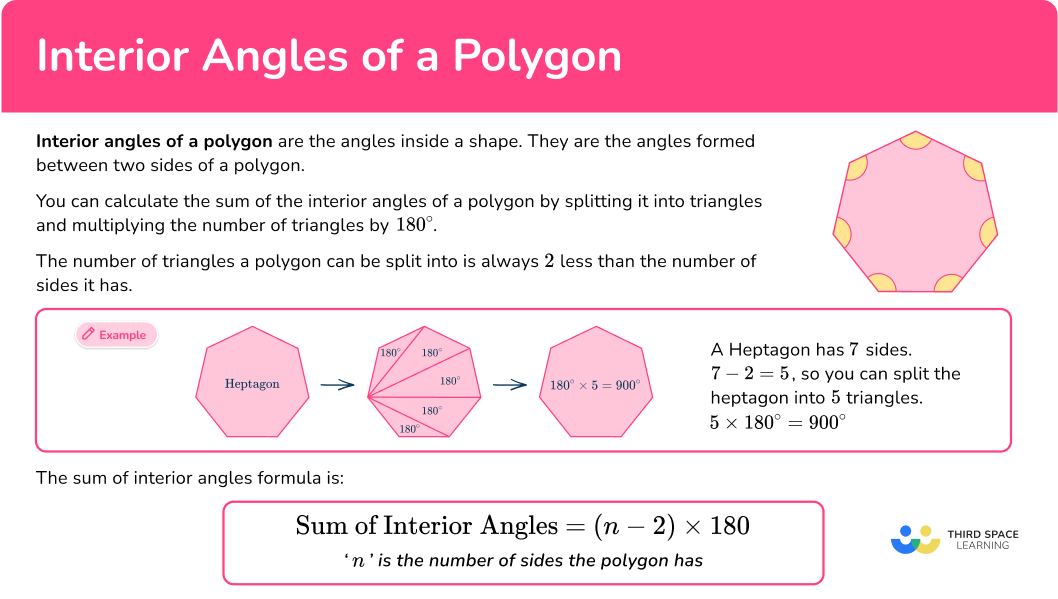
Interior angles of a polygon are the angles inside a shape. They are the angles formed between two sides of a polygon.

The interior and exterior angles of a polygon form a straight line and are therefore supplementary – together, they add up to 180^{\circ}.

You can calculate the sum of the interior angles of a polygon by splitting it into triangles and multiplying the number of triangles by 180^{\circ}.
The number of triangles a polygon can be split into is always 2 less than the number of sides it has.
For example,
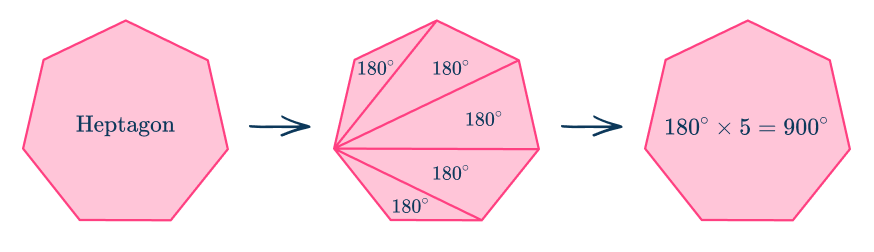
A Heptagon has 7 sides.
7- 2 = 5, so you can split the heptagon into 5 triangles.
5 \times 180^{\circ}=900^{\circ}.The sum of interior angles formula is:
Sum of Interior Angles = (n-2) \times 180
‘n’ is the number of sides the polygon has
![[FREE] Angles Check for Understanding Quiz (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Check for Understanding Quiz (Grade 4)
![[FREE] Angles Check for Understanding Quiz (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Angles Check for Understanding Quiz (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Check for Understanding Quiz (Grade 4)
![[FREE] Angles Check for Understanding Quiz (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREEKEYWORDS
Polygon: A polygon is a two dimensional shape with at least three sides, where the sides are all straight lines.
Regular & irregular polygons:
A regular polygon is where all angles are equal size and all sides are equal length, such as a square.
An irregular polygon is where all angles are not equal size and/or all sides are not equal length, such as a trapezoid.
What are interior angles of a polygon?
Common Core State Standards
How does this relate to high school math?
- High School – Geometry – Congruence (HS.G.CO.C.10)
Prove theorems about triangles. Theorems include: measures of interior angles of a triangle sum to 180^{\circ}\text{;} base angles of isosceles triangles are congruent; the segment joining midpoints of two sides of a triangle is parallel to the third side and half the length; the medians of a triangle meet at a point.
How to solve problems involving interior angles of a polygon
In order to solve problems involving interior angles of a polygon:
- Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular. - Find the sum of interior angles of the polygon(s) given.
- Identify what the question is asking.
- Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
Interior angles of a polygon examples
Example 1: finding a single interior angle of a regular polygon
Find the size of each interior angle for a regular decagon.
- Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
10 sides
Regular shape
2Find the sum of interior angles of the polygon(s) given.
Sum of interior angles =(n-2) \times 180
As a decagon has 10 sides, n=10, so you can substitute n=10 into the formula.
Sum of interior angles of a decagon =(10-2) \times 180
Sum of interior angles of a decagon =8 \times 180
Sum of interior angles of a decagon =1440^{\circ}
3Identify what the question is asking.
The question is asking for ‘each interior angle’.
This means the size of one interior angle.
4Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
You know the sum of the interior angles for this polygon is 1440^{\circ}.
Since it is a regular polygon, all the angles are of equal size.
Therefore, you can find the size of each interior angle by dividing the sum of interior angles by the number of angles in the polygon.
\text{Each interior angle} = \cfrac{1440}{10}The size of each interior angle is 144^{\circ}.
Example 2: finding a single interior angle of an irregular polygon
The diagram shows a polygon. Find the size of angle x.
Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
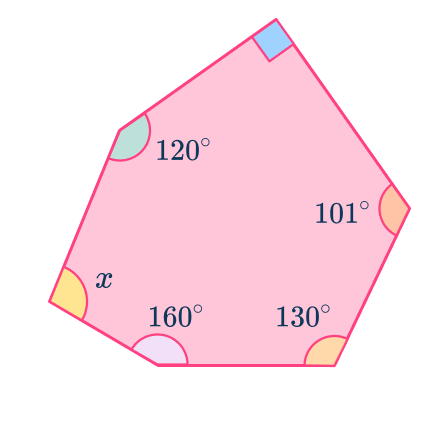
6 sides
Irregular hexagon
Find the sum of interior angles of the polygon(s) given.
Sum of Interior Angles =(n-2) \times 180
Sum of Interior Angles for a hexagon =(6-2) \times 180
Sum of Interior Angles for a hexagon =720^{\circ}
Identify what the question is asking.
Finding the missing angle labeled as x.
Note that you know the values of all the other angles.
Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
The size of angle x is 119^{\circ}.
Example 3: finding the number of sides given the interior angle of a regular polygon
Each of the interior angles of a regular polygon is 140^{\circ}. How many sides does the polygon have?
Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
Unknown number of sides
Regular shape
Find the sum of interior angles of the polygon(s) given.
You do not know the number of sides of the polygon so the sum of interior angles can be represented by (n-2) \times 180 .
Identify what the question is asking.
You need to find the number of sides.
Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
You know a single angle of this regular polygon is 140^{\circ}.
Therefore all the angles are 140^{\circ} .
You can write the sum of the interior angles as 140 multiplied by the number of sides or 140n.
Therefore,
\begin{aligned}140 n&=(n-2) \times 180 \\\\
140 n&=180 n-360 \\\\
360&=40n \\\\
9&=n \end{aligned}
The polygon has 9 sides.
NOTE: You can also solve this problem by calculating an exterior angle of a regular polygon.
Example 4: multiple shapes
Shown below are three congruent regular pentagons.
Find angle y.
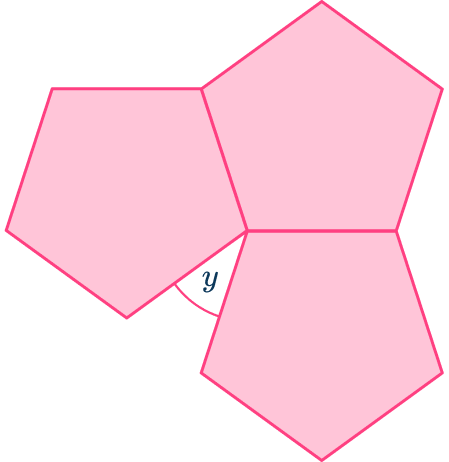
Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
Each polygon has 5 sides (pentagon) and is regular.
Find the sum of interior angles of the polygon(s) given.
As each polygon shown is a regular pentagon, they all have equal sums of their interior angles.
Sum of Interior Angles =(n-2) \times 180
Sum of Interior Angles for a pentagon =(5-2) \times 180
Sum of Interior Angles for each pentagon =540^{\circ}
Identify what the question is asking.
Find the missing angle y shown on the diagram.
Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
You know that angles around a point add to 360^{\circ}.
So if you add the three interior angles shown and y together, you will get 360^{\circ}.
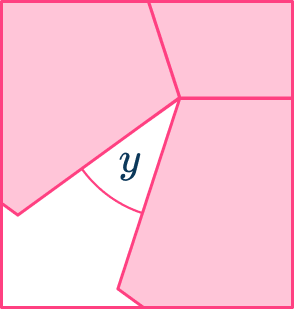
Each interior angle shown is 540 \div 5=108^{\circ}.
You can now calculate y by forming an equation:
\begin{aligned}108+108+108+y&=360 \\\\
324+y&=360 \\\\
y&=36 \end{aligned}
Angle y is equal to 36^{\circ}.
Example 5: problem solving to find the number of sides
Shown below are sections of three identical regular polygons where AB, \, BC and CA are all sides of the polygons.
ABC is an equilateral triangle formed by placing the three larger polygons together.
Calculate the number of sides each regular polygon has.
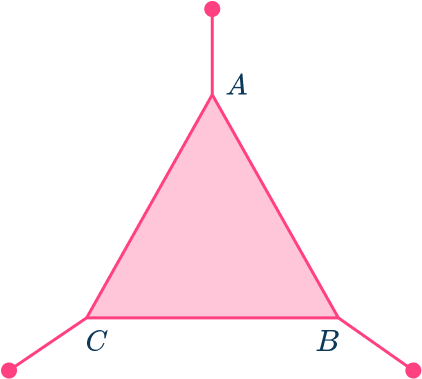
Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
Shown is an equilateral triangle (regular shape) made up of the adjacent sides AB, \, BC and CA.
You need to calculate the number of sides of the larger polygons.
Find the sum of interior angles of the polygon(s) given.
An equilateral triangles has the sum of interior angles of 180^{\circ}.
You do not know the number of sides of the polygons, so their sum of interior angles can be represented by (n-2) \times 180 .
Identify what the question is asking.
The number of sides of the regular polygons where you are only shown one side.
Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
Looking at point A , you can see there are three angles around a point. One of the angles is within the equilateral triangle, so it must be 60^{\circ}, and the other two angles are from the polygons you are attempting to find.
You will call these angles x.
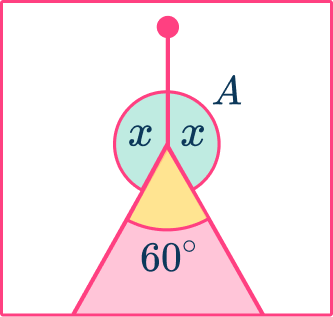
You know that angles around a point add to 360^{\circ} .
So,
\begin{aligned}60+2 x &=360 \\\\
2 x &=300 \\\\
x &=150 \end{aligned}
This means that each interior angle of the regular polygon is 150^{\circ}.
Therefore, the sum of interior angles is equal to 150 \times n or 150n.
So,
150 n=(n-2) \times 180
You can now solve for n .
\begin{aligned}150 n&=(n-2) \times 180 \\\\
150 n&=180 n-360 \\\\
360&=30 n \\\\
12&=n \end{aligned}
The polygon has 12 sides.
Therefore each polygon shown in the diagram has 12 sides.
Example 6. problem solving to find the number of angles
Shown is a regular pentagon. Find y.

Identify the number of sides in any polygon given in the question.
Note whether the shape is regular or irregular.
5 sides and regular
Find the sum of interior angles of the polygon(s) given.
Sum of Interior Angles =(n-2) \times 180
Sum of Interior Angles for a pentagon =(5-2) \times 180
Sum of Interior Angles for a pentagon =540^{\circ}
Identify what the question is asking.
Find angle y which is within one of the interior angles.
Solve the problem using the information you have already gathered with use of the formulae \textbf { interior angle } + \textbf { exterior angle }=\bf{180}^{\circ} and \textbf { Sum of exterior angles }=\bf{360}^{\circ} if needed.
As the polygon is regular, you can find the size of one interior angle by 540^{\circ} \div 5=108^{\circ}
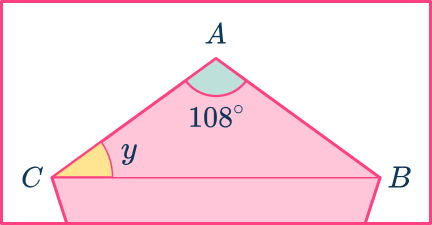
As the polygon is regular AC=AB .
Therefore ABC is an isosceles triangle where angles ACB and ABC are equal to one another and are therefore both y.
You know that the interior angles of a triangle add to 180^{\circ}.
Therefore,
\begin{aligned}108+y+y&=180 \\\\
108+2 y&=180 \\\\
2 y&=72 \\\\
y&=36 \end{aligned}
Angle y is equal to 36^{\circ}.
Teaching tips for interior angles of a polygon
- Provide a variety of problems where students calculate the sum of interior angles for different types of polygons. Include irregular polygons to challenge their understanding.
- Let students explore and come up with their own polygons, decide the measure of each interior angle, calculate the sums, and verify their findings.
- Promote group work where students can discuss and complete worksheets together.
- Expose students to as many different types of polygons as possible (convex polygons, trapezoids, parallelograms, etc.) and allow students to solve problems involving regular and irregular versions of the same type of polygon (regular hexagon vs irregular hexagon).
Easy mistakes to make
- Forgetting the formula
Mistaking the formula for the sum of interior angles. Remember, it’s (n−2) \times 180^{\circ}, not n \times 180^{\circ}.
- Misidentifying if a polygon is regular or irregular
Applying the formula for individual interior angles of regular polygons to irregular polygons. For irregular polygons, each interior angle can be different.
- Incorrect calculation of each interior angle
For regular polygons, forgetting to divide the sum of the interior angles by the number of sides to find each individual angle.
- Miscounting the number of sides
Miscounting the number of sides, especially in complex or irregular polygons, leading to incorrect angle sums.
- Ignoring triangles in calculation
Overlooking the fact that a polygon can be divided into (n−2) triangles, which is the basis for the formula.
Related angles in polygons lessons
- Interior and exterior angles of polygons
- Angles of a triangle
- Quadrilateral angles
- Sum of exterior angles of a polygon
- Angles of a hexagon
Practice interior angles of a polygon questions
1. Find the sum of interior angles for a polygon with 13 sides.




Sum of interior angles =(n-2) \times 180
In this case n=13, so the calculation becomes 11 \times 180=1980
2. Find the size of one interior angle for a regular quadrilateral.




The sum of interior angles in a quadrilateral is 360^{\circ}. For a regular shape all the angles are the same size, so you divide 360 by 4 to arrive at the answer.
360 \div 4=90
3. Find the size of one interior angle for a regular nonagon.




The sum of interior angles in a nonagon is 1260^{\circ}. For a regular shape all the angles are the same size, so you divide 1260 by 9 to arrive at the answer.
1260 \div 9=140
4. Each of the interior angles of a regular polygon is 165^{\circ}.
How many sides does the polygon have?
20 sides

22 sides

24 sides

26 sides

Sum of interior angles =(n-2) \times 180
With this in mind, you have 165n=(n-2) \times 180
Which simplifies to 15n=360
So n=24
5. Each of the interior angles of a regular polygon is 160^{\circ}.
How many sides does the polygon have?
16 sides

18 sides

20 sides

22 sides

Sum of interior angles =(n-2) \times 180
With this in mind, you have 160n=(n-2) \times 180
Which simplifies to 20n=360
So n=18
6. Four interior angles in a pentagon are each 55^{\circ}.
Find the size of the other angle.




By using the formula,
Sum of interior angles =(n-2) \times 180
You know that a pentagon has interior angles that add up to 540^{\circ}.
540-(4 \times 55) = 320
Interior angles of a polygon FAQs
The sum of the interior angles of a polygon with n sides is (n-2) \times 180^{\circ}. For example, for an octagon (n = 8), the sum of the interior angles is: (8-2) \times 180^{\circ}=6 \times 180^{\circ}=1080^{\circ}
The sum of the interior angles of a polygon with n sides is (n-2) \times 180^{\circ}, while the sum of the exterior angles of any polygon is always 360^{\circ} regardless of the number of sides.
The sum of the interior angles of a pentagon ( 5 -sided polygon) is 540^{\circ}.
The sum of the interior angles of a polygon with all right angles (a rectangle or square) is 360^{\circ}.
The next lessons are
- Congruence and similarity
- Transformations
- Mathematical proof
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!