High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
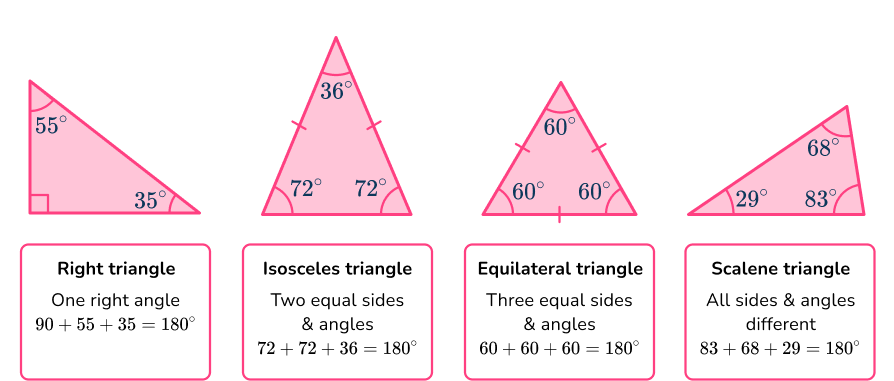
Types of triangles Obtuse angle Acute angle Right angle Straight angle Supplementary angles Complementary anglesAngles of a triangle
Here you will learn about angles of a triangle including what the sum of both interior and exterior angles of a triangle are, how to find missing angles, and how to use this alongside other angle facts to form and solve equations.
Students will first learn about angles of a triangle as a part of geometry in 8 th grade and will extend their knowledge throughout high school geometry.
What are angles of a triangle?
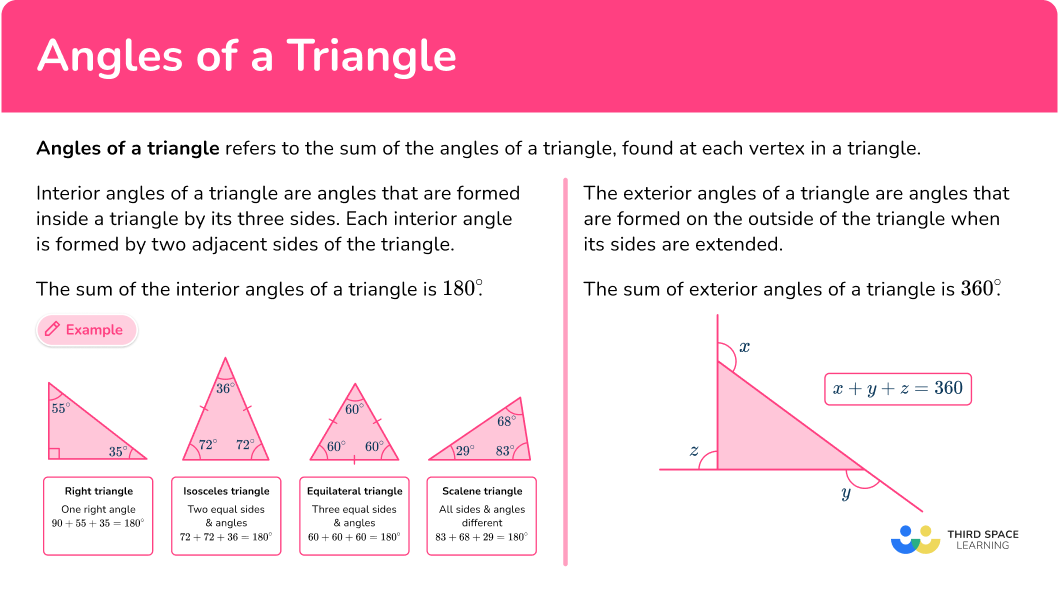
Angles of a triangle refer to the sum of the angles of a triangle, found at each vertex in a triangle.
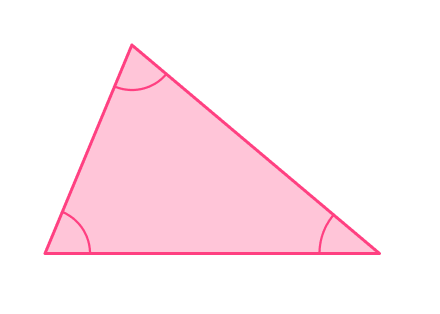
A triangle has both interior angles and exterior angles.
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Worksheet (Grade 4)
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Worksheet (Grade 4)
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREEInterior angles of a triangle
Interior angles of a triangle are angles that are formed inside a triangle by its three sides. Each interior angle is formed by two adjacent sides of the triangle.
The sum of the interior angles of a triangle is \bf{180}^{\circ}.
For example,
Exterior angles of a triangle
The exterior angles of a triangle are angles that are formed on the outside of the triangle when its sides are extended.
The sum of exterior angles of a triangle is \bf360^{\circ}.

The exterior angle is also equal to the sum of the two opposite interior angles. Each exterior angle is supplementary to its adjacent interior angle.

Other angle facts
Sometimes the problem will involve using other angle facts. Let’s recap some of the other important angle facts:
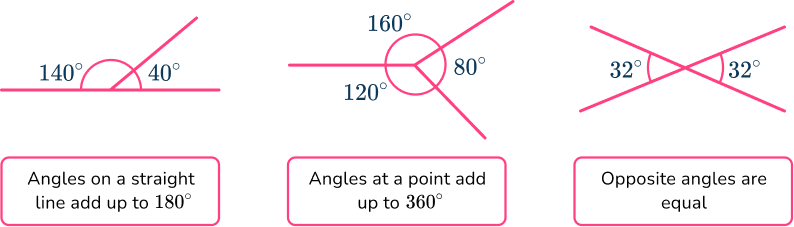
What are angles of a triangle?
Common Core State Standards
How does this relate to 8 th grade math and high school math?
- Grade 8: Geometry (8.G.A.5)
Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. For example, arrange three copies of the same triangle so that the sum of the three angles appears to form a line, and give an argument in terms of transversals why this is so.
- High school: Geometry (HS.G.CO.C.10)
Prove theorems about triangles. Theorems include: measures of interior angles of a triangle sum to 180^{\circ}; base angles of isosceles triangles are congruent; the segment joining midpoints of two sides of a triangle is parallel to the third side and half the length; the medians of a triangle meet at a point.
How to find a missing angle of a triangle
In order to find the measure of a missing angle of a triangle:
- Add up the angles that are given within the triangle.
- Subtract this total from \bf{180}^{\circ}.
Angles of a triangle examples
Example 1: scalene triangle
Find the measure of the unknown angle labeled a in the following triangle:
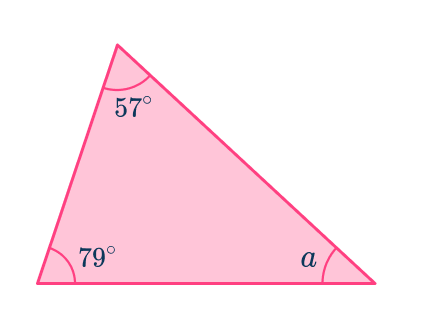
- Add up the angles that are given within the triangle.
The angles 57^{\circ} and 79^{\circ} are given. Add these together:
57+79=136^{\circ}2Subtract this total from \bf{180}^{\circ}.
Subtract 136^{\circ} from 180^{\circ} \text{:}
\begin{aligned}& 180-136=44^{\circ} \\\\ & a=44^{\circ}\end{aligned}Example 2: right triangle
Find the measure of the unknown angle labeled b in the following triangle:

Add up the angles that are given within the triangle.
The angles 90^{\circ} and 19^{\circ} are given. Add these together:
90+19=109^{\circ}
Subtract this total from \bf{180}^{\circ}.
Subtract 109^{\circ} from 180^{\circ} \text{:}
\begin{aligned}& 180-109=71^{\circ} \\\\ & b=71^{\circ}\end{aligned}
Example 3: isosceles triangle
Find the measure of the unknown angle labeled c in the following triangle:
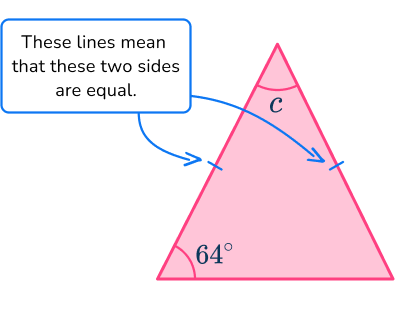
When two sides of a triangle are equal, the angles at the ends of those sides will also be equal.
Add up the angles that are given within the triangle.
The angle 64^{\circ} is given. This is an isosceles triangle (two equal length sides and two equal angles), so the other angle at the bottom will also be 64^{\circ}.
64+64=128^{\circ}
Subtract this total from \bf{180}^{\circ}.
Subtract 128^{\circ} from 180^{\circ} \text{:}
\begin{aligned}& 180-128=52^{\circ} \\\\
& c=52^{\circ}\end{aligned}
How to find a missing angle of a triangle using other angle facts
In order to find the missing angle of a triangle using other angle facts:
- Use angle facts to identify all possible angles.
- Calculate the missing angles in the triangle.
Example 4: using angles at a point
Find the measure of the angle labeled e \text{:}
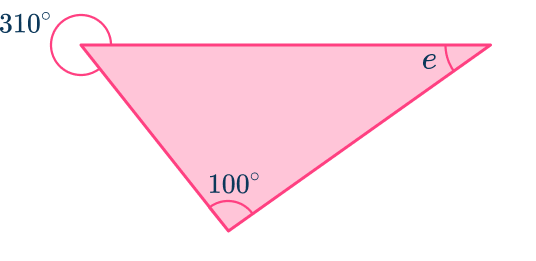
Use angle facts to identify all possible angles.
Angles at a point add up to 360^{\circ}, so you can subtract the known exterior angle from 360^{\circ}.
360-310=50^{\circ}

Calculate the missing angles in the triangle.
With the measure of two angles within the triangle, you can find the missing angle:
\begin{aligned}& 100+50=150 \\\\
& 180-150=30^{\circ} \\\\
& e=30^{\circ}\end{aligned}
Example 5: using opposite angles
Find the measure of the angle labeled f \text{:}
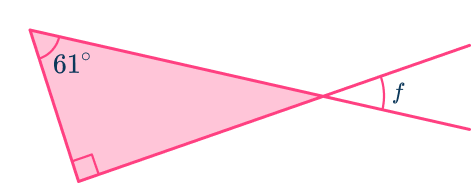
Use angle facts to identify all possible angles.
You are given two of the angles in the triangle, so start by finding the third angle:
\begin{aligned}& 90+61=151 \\\\ & 180-151=29^{\circ}\end{aligned}
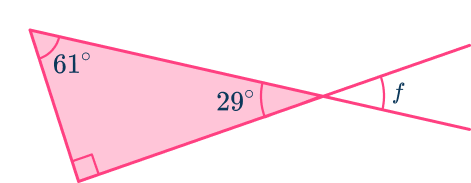
Calculate the missing angles in the triangle.
Use the fact that opposite angles are equal to find f.
f=29^{\circ}
Example 6: two different triangles
Find the measure of the angle labeled g \text{:}
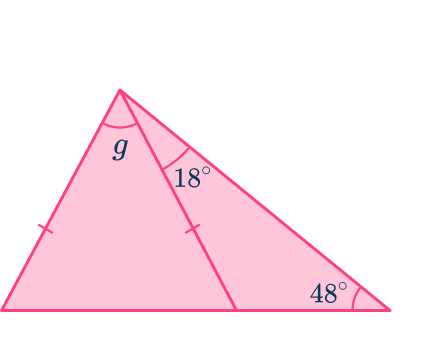
Use angle facts to identify all possible angles.
You know two of the angles in the triangle on the right, so now calculate the third.
\begin{aligned}& 48+18=66 \\\\ & 180-66=114^{\circ}\end{aligned}
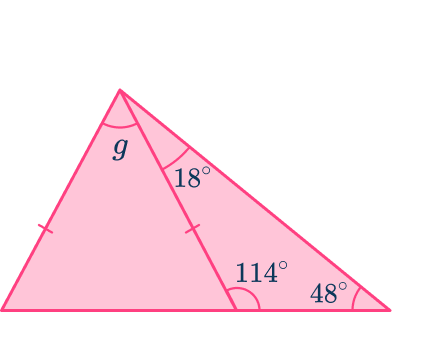
Calculate the missing angles in the triangle.
You can use the fact that angles on a straight line add up to 180^{\circ}.
180-114=66^{\circ}
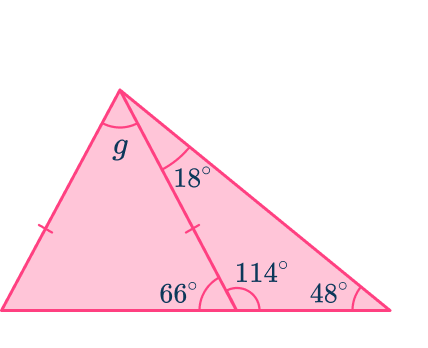
Since the sides of the triangle are equal, the triangle on the left is an isosceles triangle and the two angles at the bottom of the triangle are equal. Therefore you can find the third angle.
Teaching tips for angles of a triangle
- Visual aids, such as diagrams, and hands-on activities allow students to explore the properties of triangles in a different way.
- Allow students to work in pairs or small groups while working through practice problems on worksheets. This encourages discussions about math between peers, which allows for students to share their personal insights and strategies used.
- Provide real-time feedback to students as they are working through practice problems to clarify any misunderstandings as they arise. The use of a triangle calculator can support this, without having to take any additional time from you.
Easy mistakes to make
- Mixing up the sum of interior angles and exterior angles
Students may use 360^{\circ} instead of 180^{\circ} for the sum of the interior angles of the triangle and vice versa.
- Equal angles in an isosceles triangle
Selecting the wrong angles when identifying the equal angles in an isosceles triangle (particularly a problem when the equal angles are not at the bottom). The angle that is different in an isosceles triangle is the one between the two sides with equal length.
Related angles in polygons lessons
- Interior and exterior angles of polygons
- Quadrilateral angles
- Interior angles of a polygon
- Sum of exterior angles of a polygon
- Angles of a hexagon
Angles in a triangle practice questions
1. Find the measure of the angle b in the following triangle:
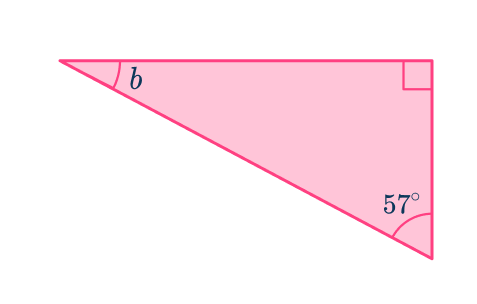




2. Find the measure of the angle c \text{:}
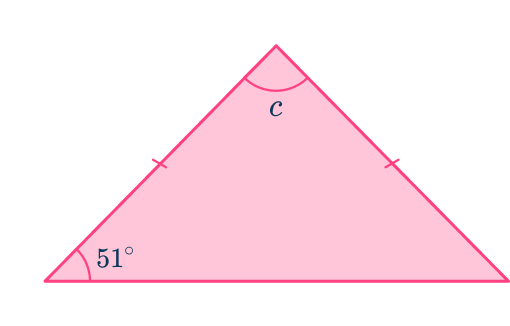




This is an isosceles triangle and the two angles at the bottom of the triangle are equal.
\begin{aligned}& 51+51=102 \\\\ & 180-102=78^{\circ}\end{aligned}
3. Find the measure of angle x in the following triangle:
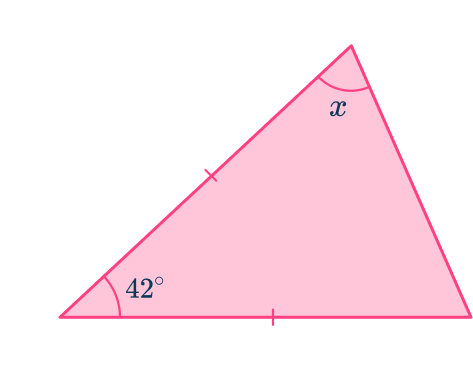




This is an isosceles triangle and the two angles on the right are equal.
180-42=138
138 \div 2 = 69^{\circ}
4. What is the size of each angle in an equilateral triangle?




An equilateral triangle has three equal sides so
180 \div 3 = 60^{\circ}
5. Find the measure of the angle labeled w in the following triangle:





The angle opposite 24^{\circ} is also 24^{\circ} since vertically opposite angles are equal. The triangle is an isosceles triangle and the two angles on the left are the same size.
180-24=156
156 \div 2 = 78^{\circ}
6. Find the measure of the angle labeled v \text{:}
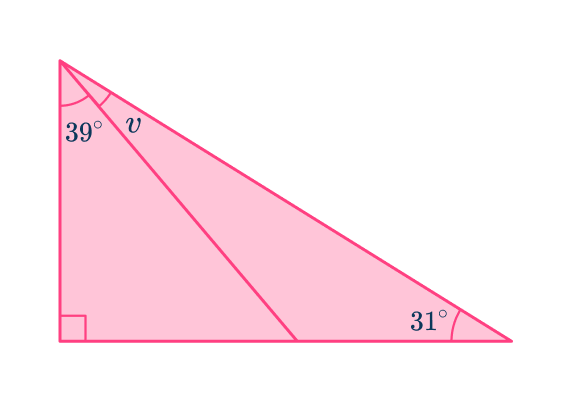




Let’s find the missing angle in the triangle on the left first,
\begin{aligned}& 90+39=129 \\\\ & 180-129=51^{\circ}\end{aligned}
Then use the fact that angles on a straight line add up to 180^{\circ} to find the unlabeled angle in the right hand triangle.
180-51=129^{\circ}
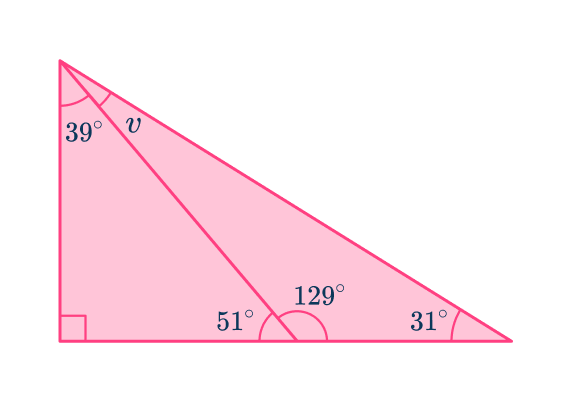
Now, find angle v \text{:}
\begin{aligned}& 129+31=160^{\circ} \\\\ & 180-160=20^{\circ}\end{aligned}
Angles of a triangle FAQs
No, the interior angles within a triangle can vary depending on the triangle. However, all of the measures of angles within a triangle will always equal 180 degrees.
Each exterior angle of a triangle is supplementary to its adjacent interior angle. The sum of each exterior angle and its adjacent interior angle will equal 180 degrees.
The Law of Sines is a principle in trigonometry that relates the side lengths of a triangle to the sines of its angles. It states that in any triangle, the ration of the length of a side to the sine of its opposite angle is constant for all three sides. It can be expressed as:
\cfrac{a}{\sin (A)}=\cfrac{b}{\sin (B)}=\cfrac{c}{\sin (C)}.
The next lessons are
- Congruence and similarity
- Transformations
- Mathematical proofs
- Trigonometry
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!