High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Types of angles Measuring angles Angles of a triangle Substitution Solving equationsVertical angles theorem
Here you will learn about the vertical angles theorem, including what it is and how to solve problems with it.
Students will first learn about the vertical angles theorem as part of geometry in 7 th grade.
What is the vertical angles theorem?
The vertical angles theorem states that angles that are opposite one another at a specific vertex and created by two straight intersecting lines are called vertical angles.
For example,
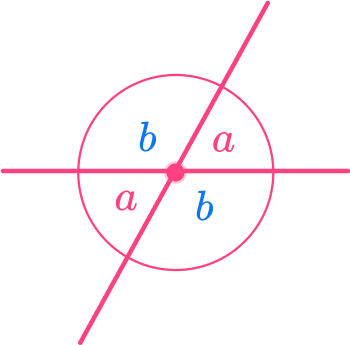
Here the two angles labeled a are congruent because they are vertical angles. This also applies to the angles labeled b. You can try out the above rule by drawing two crossing lines and measuring the angles opposite to one another.
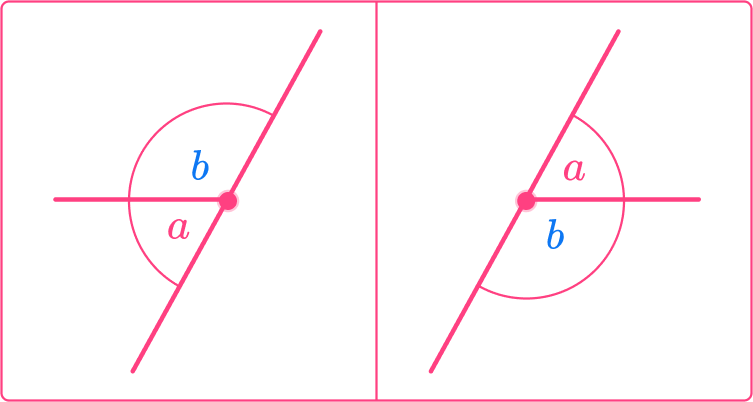
You will also notice that the adjacent angles a and b lie on a straight line. This means they are equal to 180^{\circ} when added together, so they are also supplementary angles.
What is the Vertical Angles Theorem?
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Worksheet (Grade 4)
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
[FREE] Angles Worksheet (Grade 4)
![[FREE] Angles Worksheet (Grade 4)](https://thirdspacelearning.com/wp-content/uploads/2023/08/Angles-check-for-understanding-quiz-listing-image.png)
Use this quiz to check your grade 4 students’ understanding of angles. 10+ questions with answers covering a range of 4th grade angles topics to identify areas of strength and support!
DOWNLOAD FREEKey words
Angle: defined as the amount of turn around a common vertex, measured most commonly in degrees or radians.
Vertex: the point created by two line segments meeting (plural is vertices).
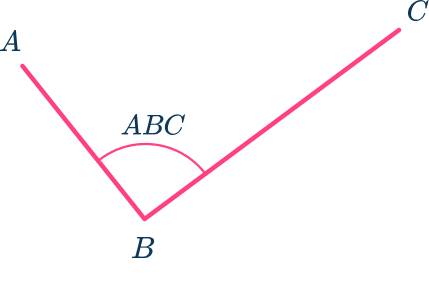
How to label an angle
You can label angles in two main ways:
- By giving the angle a ‘name’ which is normally a lower case letter such as a, x or y or the greek letter \theta (theta).
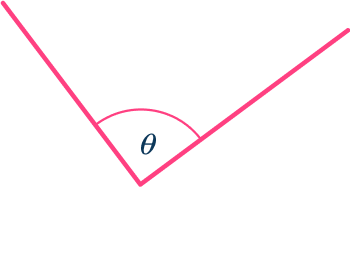
- By referring to the angle as the three letters that define the angle. The middle letter refers to the vertex at which the angle is.
Angles on a straight line equal 180°
Angles on one part of a straight line always add up to 180^{\circ}. See the diagram for an example where angles a and b are equal to 180^{\circ}.
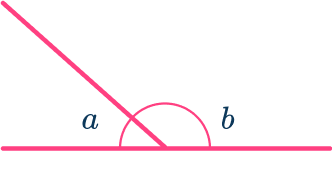
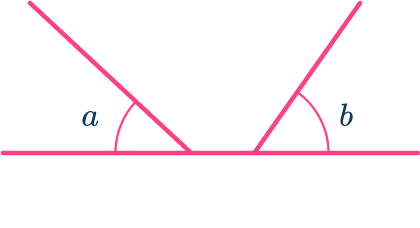
However, see the next diagram for an example of where a and b do not equal 180^{\circ} because they are not on one single part of a straight line, i.e. they do not share a vertex and are not adjacent to one another.
Note – you can try out the above rule by drawing out the above diagrams and measuring the angles using a protractor.
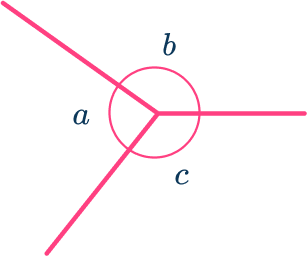
Angles around a point
Angles around a point will always equal 360^{\circ}. See the diagram for an example where angles a,b and c are sum to 360^{\circ}.
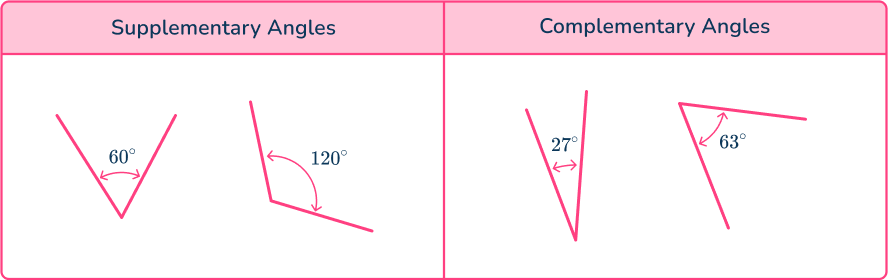
Supplementary and complementary angles
Two angles are supplementary when they add up to \bf{180^{\circ}}, they do not have to be next to each other. Two angles are complementary when they add up to \bf{90^{\circ}}, they do not have to be next to each other.
Common Core State Standards
How does this relate to 7 th grade math?
- Grade 7 – Geometry (7.G.B.5)
Use facts about supplementary, complementary, vertical, and adjacent angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure.
How to use the vertical angles theorem to solve problems
In order to solve problems involving the vertical angles theorem:
- Identify which angles are vertically opposite to one another.
- Identify which of the unknown angles the question is asking you to find the value of.
- Solve the problem and give reasons where applicable.
- Clearly state the answer using angle terminology.
Vertical angles theorem examples
Example 1: two angles that are vertically opposite
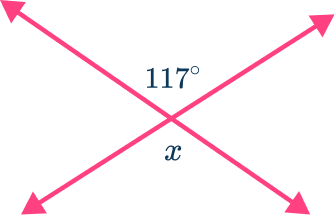
Find the value of angle x.
- Identify which angles are vertically opposite to one another.
The angle labeled x and the angle with value 117^{\circ} are vertical angles, since their vertex is created by two straight lines intersecting.
2Identify which of the unknown angles the question is asking you to find the value of.
The angle labeled x.
3Solve the problem and give reasons where applicable.
x=117 because vertical angles are congruent.
4Clearly state the answer using angle terminology.
x=117^{\circ}Example 2: two angles that are vertically opposite
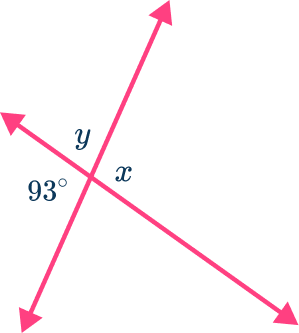
Find the values of angles x and y.
Identify which angles are vertically opposite to one another.
The angle labeled x and the angle with value 93^{\circ} are vertical angles, since their vertex is created by two straight lines intersecting.
Identify which of the unknown angles the question is asking you to find the value of.
The angles labeled x and y.
Solve the problem and give reasons where applicable.
x=93^{\circ} because vertical angles are congruent.
x and y are not vertical angles, but they are supplementary angles because they are on a straight line at the same vertex.
Clearly state the answer using angle terminology.
Example 3: finding all the angles around a point with vertically opposite angles

Find the values of the angles labeled a,b and c .
Identify which angles are vertically opposite to one another.
The angle labeled a and the angle with value 22^{\circ} are vertical angles.
The angle labeled b and the angle labeled c are vertical angles.
Identify which of the unknown angles the question is asking you to find the value of.
The angles labeled a,b and c.
Solve the problem and give reasons where applicable.
a=22^{\circ} because vertical angles are congruent.
a and b are not vertical angles, but they are supplementary angles, because they are on a straight line at the same vertex.
c=158^{\circ} because vertical angles are congruent.
Clearly state the answer using angle terminology.
Example 4: vertically opposite angles with algebra
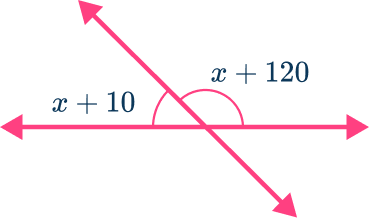
Use the vertical angles theorem to find the value of x.
Identify which angles are vertically opposite to one another.
The angle labeled ‘x+10’ and the angle on the other side of the vertex are vertical angles.
The angle labeled ‘x+120’ and the angle on the other side of the vertex are vertical angles.
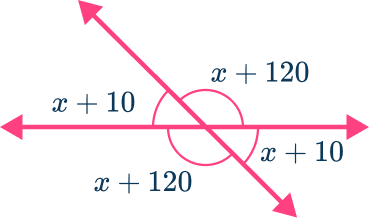
Identify which of the unknown angles the question is asking you to find the value of.
You are not being asked to find an angle, you are being asked to find the value of x.
Solve the problem and give reasons where applicable.
The four angles total 360^{\circ} because they are all around a vertex.
Therefore:
Clearly state the answer using angle terminology.
Example 5: vertical angles with more than two lines

What is the value of b?
Identify which angles are vertically opposite to one another.
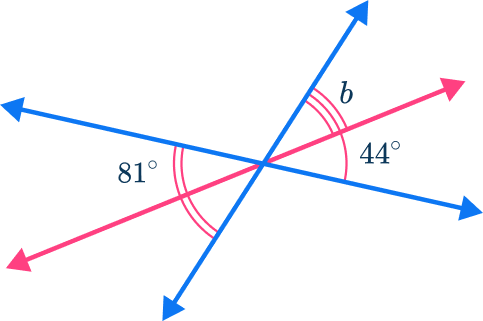
The two lines that form the 81^{\circ} angle create vertical angles. So, the 81^{\circ} angle is congruent to angle b and the 44^{\circ} angle.
Identify which of the unknown angles the question is asking you to find the value of.
The angle labeled b.
Solve the problem and give reasons where applicable.
Set the expressions equal to each other and solve for x.
Clearly state the answer using angle terminology.
Example 6: word problem
Two angles with values of x+30 and 4x -30 are vertically opposite one another. Prove the two angles are both 50^{\circ}.
Identify which angles are vertically opposite to one another.
The angles labeled x+30 and 4x-30 vertically opposite to one another meaning they are equal to one another.
Clearly identify which of the unknown angles the question is asking you to find the value of.
You are being asked to prove the size of each angle is 50^{\circ}. This means show all your working to reach this conclusion.
Solve the problem and give reasons where applicable.
Set the expressions equal to each other and solve for x.
Therefore, the size of the two angles can be found by substituting x=20 into each angle.
Angle 1
Angle 2
Clearly state the answer using angle terminology.
Therefore both angles are 50^{\circ}.
Teaching tips for vertical angles theorem
- Start with lesson plans that have pairs of vertical angles that do not involve variables and whose measure of angles is whole numbers. Also give students informal graphing opportunities to test vertical angle examples, before proving it with linear pairs.
- Before sending students off to do a worksheet on their own, be sure that they have a few solved examples they can refer back to if they get stuck.
Easy mistakes to make
- Assuming angles are vertical without proof
Angles that are formed by two straight lines crossing are congruent angles. However, you cannot assume congruence without this proof. Sometimes a set of angles may appear to be vertical angles, but this must be given information or proven.
For example,
If you are given just this image and no text or values, you should not assume they are vertical angles. The angles below are close to being vertical angles, but they are not.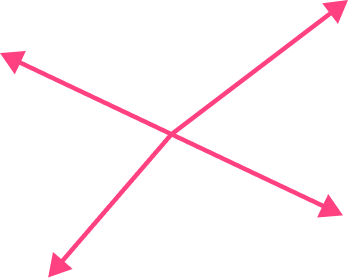
- Finding the incorrect angle due to misunderstanding the terminology
It’s easy to confuse the terms vertical angle, supplementary angle, complementary angle, straight angle, etc. Since these terms are often introduced and used together, it is important to always read problems carefully and consider the definition of each.
Related angles lessons
- Acute angle
- Obtuse angle
- Right angle
- Adjacent angles
- Supplementary angles
- Complementary angles
- Geometry theorems
- Straight angles
- Angles point
- Pentagon angles
Practice questions on angle rules
1. Find the value of the angle labeled x.
Angle AOC
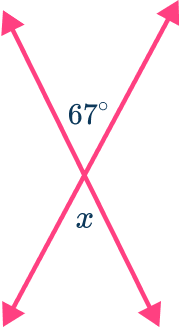




The angle labeled x and the angle with value 67^{\circ} are vertical angles since their vertex is created by two straight lines intersecting. This means the angles are equivalent.
2. Find the value of the angle labeled x.
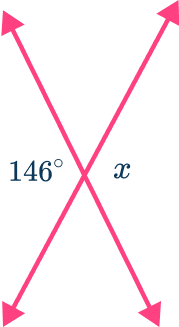




The angle labeled x and the angle with value 146^{\circ} are vertical angles since their vertex is created by two straight lines intersecting. This means the angles are equivalent.
3. Find the value of the angle labeled x.





The angle labeled x and the angle with value 98^{\circ} are vertical angles since their vertex is created by two straight lines intersecting. This means the angles are equivalent.
4. Find the value of the angle labeled x and y.
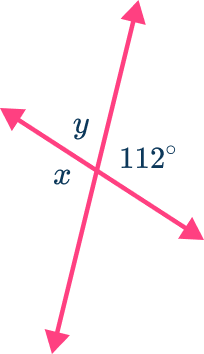




Angle x is vertically opposite the given angle 112^{\circ}, so it is the same.
x=112
Angle x and angle y lie on a straight line, so they must add up to 180^{\circ}.
\begin{aligned}& 112+y=180 \\\\ & y=68 \end{aligned}
5. Two angles with values of 2x and 50^{\circ} are vertically opposite one another. Find the value of x.




Angle 2x is vertically opposite the given angle of 50^{\circ} so it is equivalent.
\begin{aligned}& 2 x=50 \\\\ & x=25 \end{aligned}
6. Two angles with values of 6x+10 and 10x-70 are vertically opposite one another. Find the value of x.




Angle 6x+10 is vertically opposite angle 10x-70, so they are equivalent. Solving the equation, 6x+10=10x-70, leads to the correct value for x.
\begin{aligned}& 6 x+10=10 x-70 \\\\ & 10=4 x-70 \\\\ & 80=4 x \\\\ & 20=x \end{aligned}
Vertical angles theorem FAQs
A pair of opposite angles is a pair of non-adjacent angles that have an equal measure because they are formed by two lines crossing. This term is used interchangeably with vertical angles.
Yes, because they are formed by two lines crossing, making their vertical angles equivalent.
Angles on the outside of a figure formed by the side of a figure and an extended line; the opposite of interior angles.
A line that intersects two other lines at a distinct place.
A line that splits something geometrical in half.
A polygon with four sides.
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!