High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Parts of a circle Area of a circle Area of a sector Area of a triangle Arc length formula Pythagorean theorem Law of cosines Area of a triangle Rounding

Math resources Geometry

Segment of a circle

Sectors arcs and segments

Segment of a circle

Here you will learn about a segment of a circle including how to identify the segment of a circle and how to find the area of a segment given the different parts of a circle.

Students will first learn about a segment of a circle as part of geometry in high school.

What is a segment of a circle?

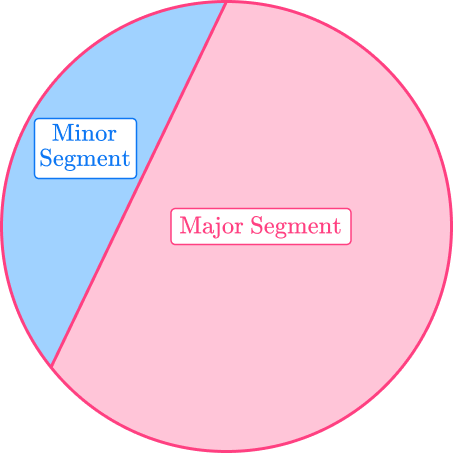
A segment of a circle, also called a circular segment, is the area enclosed by an arc of a circle and a chord.

There are two main types of segment:

The major segment is the segment where the arc length is greater than half the circumference of the circle.

The minor segment is the segment where the arc length is less than half the circumference of the circle.

An arc is a fraction of the circumference of a circle.

A chord is a line segment that connects two points of a circle.

A segment where the chord passes through the centre of the circle is called a semicircle.

What is a segment of a circle?

Common Core State Standards

How does this relate to high school math?

High School – Geometry – Circles (HS.G.C.B.5)
Derive using similarity the fact that the length of the arc intercepted by an angle is proportional to the radius, and define the radian measure of the angle as the constant of proportionality; derive the formula for the area of a sector.

[FREE] 2D Shape Check for Understanding Quiz (Grade 2 to 4)

Use this quiz to check your grade 2 to 4 students’ understanding of 2D shape. 15+ questions with answers covering a range of 2nd, 3rd and 4th grade 2D shape topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] 2D Shape Check for Understanding Quiz (Grade 2 to 4)

DOWNLOAD FREE

How to solve problems involving a segment of a circle

In order to solve problems involving a segment of a circle:

Questions involving area

Find the length of the radius.
Find the size of the angle creating the sector.
Find the area of the sector.
Find the area of the triangle created by the radii and the chord of a circle.
Subtract the area of the triangle from the area of the sector.
Clearly state your answer.

Questions involving perimeter

Find the length of the radius.
Find the size of the angle creating the sector.
Find the length of the arc of the segment of a circle.
Find the length of the chord of the segment.
Add the length of the arc and the chord.
Clearly state your answer.

Segment of a circle examples

Example 1: calculate the area of a segment of a circle

Calculate the area of the segment shown below. Give your answer to the nearest whole number.

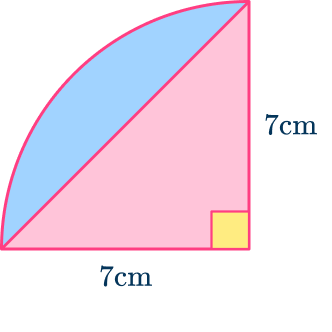
Find the length of the radius.

Length of the radius of the circle: $7{~cm}$

2Find the size of the angle creating the sector.

The angle subtended by the sector, formed by the two radii, is $90^{\circ}.$

3Find the area of the sector.

\begin{aligned} \text{Area of sector }&=\cfrac{\theta}{360} \times \pi r^{2}\\\\ &=\cfrac{90}{360} \times \pi \times 7^{2} \\\\ &=\cfrac{49}{4} \, \pi \end{aligned}

Area of sector: $\cfrac{49}{4} \, \pi \mathrm{cm}^{2}$

It is important to not round the answer at this stage of the question.

4Find the area of the triangle created by the radii and the chord.

\begin{aligned} \text{Area of triangle} &= \cfrac{1}{2} \, a b \sin C \\\\ &=\cfrac{1}{2} \times 7 \times 7 \times \sin(90)\\\\ &=24.5 \end{aligned}

Area of triangle: $24.5{~cm}^2$

5Subtract the area of the triangle from the area of the sector.

Area of sector $-$ Area of triangle $= \cfrac{49}{4} \, \pi-24.5$

=13.9845\dots

6Clearly state your answer.

The question asked you to round your answer to the nearest whole number.

Area of the segment of the circle $=13.9845…{~cm^2} =14.0 {~cm^2}$ (nearest whole number)

Example 2: calculate the perimeter of a segment

Calculate the perimeter of the segment shown below. Give your answer to the nearest thousandth.

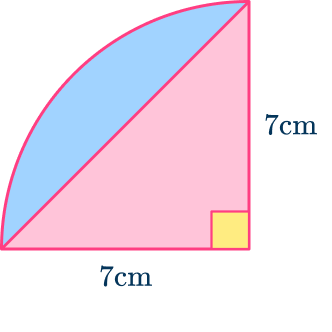
Find the length of the radius.

Length of radius: $7{~cm}$

Find the size of the angle creating the sector.

The angle subtended by the sector, formed by the two radii, is $90^{\circ}$ .

Find the length of the arc of the segment.

\begin{aligned} \text{Arc length}&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{90}{360} \times 2 \pi \times 7 \\\\ &=\cfrac{7}{2} \, \pi \end{aligned}

Length of the arc: $\cfrac{7}{2} \, \pi$

Find the length of the chord of the segment.

In this question, you can see the two radii and the chord form a right angled triangle. This means you can use Pythagoras’ theorem to find the length of the chord, which is the hypotenuse of the triangle.

$\begin{aligned} a^{2}+b^{2}&=c^{2} \\\\ 7^{2}+7^{2}&=c^{2} \\\\ 49+49&=c^{2} \\\\ 98&=c^{2} \\\\ 7 \sqrt{2}&=c \end{aligned}$

square root both sides of the equation

Therefore the length of the chord is $7 \sqrt{2} {~cm.}$

Add the length of the arc and the chord.

Length of the arc $+$ Length of the chord $= \cfrac{7}{2} \pi+7 \sqrt{2}$

$=20.895069\dots$

Clearly state your answer.

The question asked you to round your answer to the nearest thousandth.

Perimeter of segment: $20.895069\dots{~cm} = 20.895{~cm}$ (nearest thousandth)

Example 3: calculate the area of a segment given the length of the radii and the angle

Calculate the area of the segment shown below. Give your answer to the nearest thousandth.

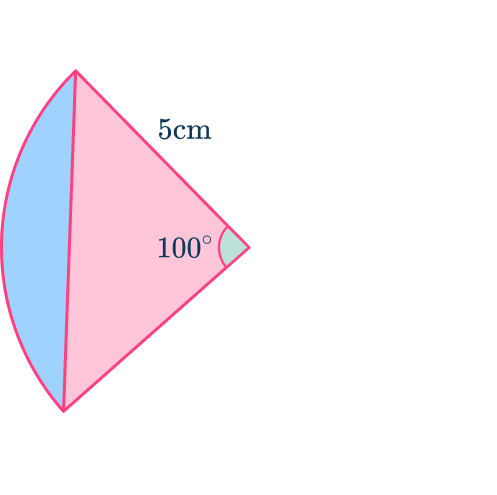
Find the length of the radius.

Length of radius: $5{~cm}$

Find the size of the angle creating the sector.

The angle subtended by the sector, formed by the two radii, is $100^{\circ}$ . This is given in the question.

Find the area of the sector.

\begin{aligned} \text{Area of sector}&=\cfrac{\theta}{360} \times \pi r^{2}\\\\ &=\cfrac{100}{360} \times \pi \times 5^{2} \\\\ &=\cfrac{125}{18} \pi \end{aligned}

Area of sector: $\cfrac{125}{18} \, \pi \mathrm{cm}^{2}$

Find the area of the triangle created by the radii and the chord.

\begin{aligned} \text{Area of triangle }&=\cfrac{1}{2} \, a b \sin C \\\\ &=\cfrac{1}{2} \times 5\times 5 \times \sin(100)\\\\ &=12.3100… \end{aligned}

Area of triangle: $12.3100...{~cm^2}$

Subtract the area of the triangle from the area of the sector.

Area of sector $-$ Area of triangle $=\cfrac{125}{18} \, \pi -12.3100...$

=9.50661565\dots

Clearly state your answer.

The question asked you to round your answer to the nearest thousandth.

Area of segment: $9.50661565…cm^2= 9.507 {~cm^2}$ (nearest thousandth)

Example 4: calculate the perimeter of a segment given the length of the radii and the angle

Calculate the perimeter of the segment shown below. Give your answer to the nearest thousandth.

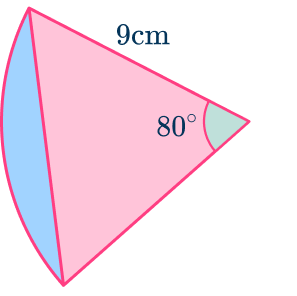
Find the length of the radius.

Length of radius: $9{~cm}$

Find the size of the angle creating the sector.

The angle subtended by the sector, formed by the two radii, is $80^{\circ}$ . This is given in the question.

Find the length of the arc of the segment of a circle.

\begin{aligned} \text{Arc length}&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{80}{360} \times 2 \pi \times 9 \\\\ &=4 \pi \end{aligned}

Length of the arc: $4 \, \pi$

Find the length of the chord of the segment.

In this question, the triangle is not a right angled triangle so you cannot use Pythagoras’ Theorem to find the missing length. Instead you can use the cosine rule.

\begin{aligned} a^{2}&=b^{2} + c^{2} -2bc \cos(A)\\\\ a^{2}&=9^{2} + 9^{2} - 2 \times 9 \times 9 \times \cos(80)\\\\ a^{2}&=162-162 \cos(80)\\\\ a^{2}&=133.8689952\\\\ a&=11.57017697 \end{aligned}

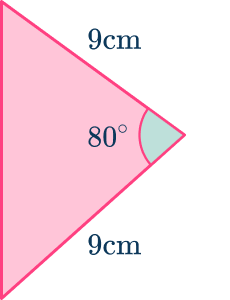
Add the length of the arc and the chord.

Length of the arc $+$ Length of the chord $= 4 \pi+11.57017697$

$=24.13654759$

Clearly state your answer.

The question asked you to round the answer to the nearest thousandth.

Perimeter = $24.137{~cm}$

Example 5: calculate the area of a segment without the angle of the segment being given

Calculate the area of the segment shown below. Give your answer to the nearest tenth.

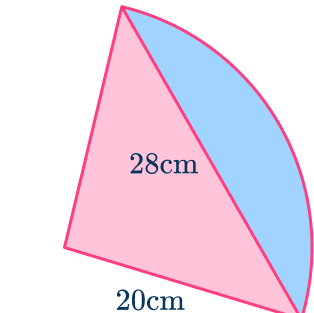
Find the length of the radius.

Length of radius: $20{~cm}$

Find the size of the angle creating the sector.

The angle of the sector, created by the two radii, is not given to you in this question.

Here you can use the triangle created by the two radii and the chord to find the angle.

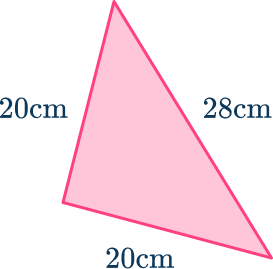
You need to apply the cosine rule to find the size of the angle.

A is the angle you are trying to find. You can therefore use the rearranged cosine rule to find the angle.

\begin{aligned} \cos{A}&=\cfrac{b^{2}+c^{2}-a^{2}}{2 b c} \\\\ \cos{A}&=\cfrac{20^{2}+20^{2}-28^{2}}{2 \times 20 \times 20} \\\\ \cos{A}&=\cfrac{1}{50} \\\\ A&=\cos^{-1}\left(\cfrac{1}{50}\right) \\\\ A&=88.8540008^{\circ} \end{aligned}

The size of the angle creating the sector (made by the two radii) is $88.854^{\circ}$ .

Find the area of the sector.

\begin{aligned} \text{Area of sector }&=\cfrac{\theta}{360}\times \pi r^{2}\\\\ &=\cfrac{88.854}{360} \times \pi \times 20^{2} \\\\ &=310.15897 \end{aligned}

Area of sector: $310.15897 {~cm^2}$

Find the area of the triangle created by the radii and the chord.

\begin{aligned} \text{Area of triangle}&=\cfrac{1}{2} \, a b \sin C \\\\ &=\cfrac{1}{2} \times 20\times 20 \times\sin(88.854)\\\\ &=199.95999… \end{aligned}

Area of triangle: $199.95999…{~cm^2}$

Subtract the area of the triangle from the area of the sector.

Area of sector $-$ Area of triangle $=310.15897-199.959..$

$=110.19897\dots$

Clearly state your answer.

The question asked you to round your answer to the nearest tenth.

Area of segment: $110.19897{~cm^2}= 110.2{~cm^2}$ (nearest tenth)

Example 6: calculate the perimeter of a segment without the angle of the segment being given

Calculate the perimeter of the segment shown below. Give your answer to the nearest whole number.

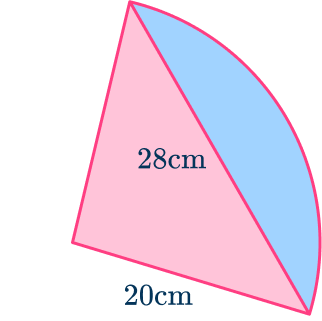
Find the length of the radius.

Length of radius: $20{~cm}$ (given to you in the question)

Find the size of the angle creating the sector.

The angle of the sector, created by the two radii, is not given to you in this question. Here you can use the triangle created by the two radii and the chord to find the angle.

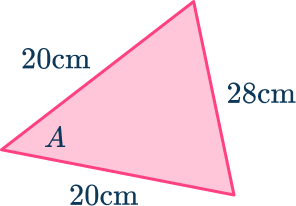
You need to apply the cosine rule to find the size of the angle.

$A$ is the angle you are trying to find. You can therefore use the rearranged cosine rule to find the angle.

$\begin{aligned} \cos{A}&=\cfrac{b^{2}+c^{2}-a^{2}}{2 b c} \\\\ \cos{A}&=\cfrac{20^{2}+20^{2}-28^{2}}{2 \times 20 \times 20} \\\\ \cos{A}&=\cfrac{1}{50} \\\\A&=\cos^{-1}\left(\cfrac{1}{50}\right) \\\\ A&=88.8540008 \end{aligned}$

The size of the angle creating the sector (made by the two radii) is therefore $88.854^{\circ}$ .

Find the length of the arc of the segment.

\begin{aligned} \text{Arc length }&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{88.854}{360} \times 2 \pi \times 20 \\\\ &=31.0159… \end{aligned}

Length of the arc: $31.0159..{~cm}$

Find the length of the chord of the segment.

Length of chord: $28{~cm}$

Add the length of the arc and the chord.

Length of the arc $+$ Length of Chord $=31.0159..+28=59.0159{~cm}$

Clearly state your answer.

The question asked you to round your answer to the nearest whole number.

Perimeter of segment: $59.0159\dots{~cm}= 59{~cm}$ (nearest whole number)

Teaching tips for segment of a circle

Provide worksheets with word problems based on practical scenarios where understanding segments of a circle is useful, such as designing a circular park with a walkway.

Work through problems involving finding the area of a segment, given different sets of information (like radius of a circle and central angle).

Link the concept of segments to trigonometry (especially when dealing with the associated triangle) and other geometric concepts.

Easy mistakes to make

Confusing the segment/sector
A segment is the area enclosed by the arc of a circle and a chord. A sector is the area enclosed by the arc of a circle and two radii.

Confusing the chord/diameter
A chord is a line segment with both endpoints on the circle and can cross a circle at any two points along the circle’s circumference. The diameter of a circle is a special type of chord that must pass through the center of the circle.

Incorrect use of the cosine rule
Many mistakes are made when applying other rules within a segment question, such as the cosine rule. Take your time with these parts and regularly check that your answer makes sense within the context of the question.

Not including the chord length when finding the perimeter of a segment
Remember, the perimeter of a shape is the sum of the lengths of each of the sides. Therefore, the perimeter of a segment is made up of the arc and the chord.

Angles of a circle
Area of a segment of a circle
Arc of a circle
Sector of a circle
Perimeter of a sector

Practice segment of a circle questions

36 \pi \mathrm{~cm}^2

72 \mathrm{~cm}^2

41 \mathrm{~cm}^2

41.097 \mathrm{~cm}^2

\begin{aligned} \text{Area of sector }&=\cfrac{\theta}{360} \, \pi r^{2}\\\\ &=\cfrac{90}{360}\times \pi \times 12^{2}\\\\ &=36 \pi \end{aligned}

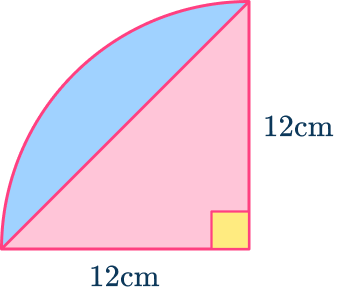
\begin{aligned} \text{Area of triangle }&=\cfrac{1}{2} \, ab \sin C\\\\ &=\cfrac{1}{2}\times 12 \times 12 \sin(90)\\\\ &=72 \end{aligned}

\begin{aligned} \text{Area of segment }&=36 \pi – 72\\\\ &=41.09733553\\\\ &=41 \mathrm{~cm}^{2} \end{aligned}

12 \pi

40.9{~cm}

20.7{~cm}

35.8{~cm}

\begin{aligned} \text{Arc length}&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{90}{360} \times 2 \pi \times 12 \\\\ &=6 \pi \end{aligned}

Length of chord:

\begin{aligned} a^{2}+b^{2}&=c^{2} \\\\ 12^{2}+12^{2}&=c^{2} \\\\ 144+144&=c^{2} \\\\ 288&=c^{2} \\\\ 12 \sqrt{2}&=c \end{aligned}

Length of the arc $+$ Length of the chord $= 6\pi + 12 \sqrt{2}$

\begin{aligned} \quad \quad &=35.82011867\\\\ \quad \quad &=35.8\mathrm{~cm} \end{aligned}

22.3{~m^2}

19.6{~m^2}

25.1{~m^2}

15.9{~m^2}

\begin{aligned} \text{Area of sector }&=\cfrac{\theta}{360} \, \pi r^{2}\\\\ &=\cfrac{160}{360}\times \pi \times 4^{2}\\\\ &=\cfrac{64}{9} \pi \end{aligned}

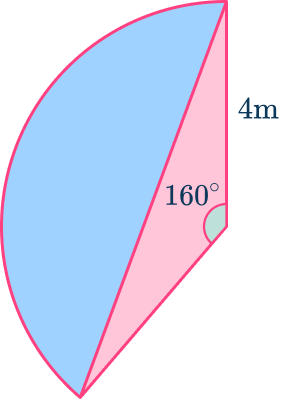
\begin{aligned} \text{Area of triangle }&=\cfrac{1}{2} \, ab \sin C\\\\ &=\cfrac{1}{2}\times 4 \times 4 \sin(160)\\\\ &=2.73616… \end{aligned}

\begin{aligned} \text{Area of segment }&=\cfrac{64}{9} \, \pi – 2.73616\\\\ &=19.60405\\\\ &=19.6\mathrm{~m}^{2} \end{aligned}

1.92{~m}

3.76{~m}

1.85{~m}

3.77{~m}

\begin{aligned} \text{Arc length}&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{55}{360} \times 2 \pi \times 2 \\\\ &=\cfrac{11}{18} \pi \end{aligned}

Length of chord:

\begin{aligned} a^{2}&=b^{2} + c^{2} -2bc \cos(A)\\\\ a^{2}&=2^{2} + 2^{2} – 2 \times 2 \times 2 \times \cos(55)\\\\ a^{2}&=8-8 \cos(55)\\\\ a^{2}&=3.411388509\\\\ a&=1.846994453 \end{aligned}

Length of the arc $+$ Length of the chord $=\cfrac{11}{18} \pi + 1.846994453$

\quad \quad =3.76685663

14.21{~cm^2}

25.40{~cm^2}

11.19{~cm^2}

18.66{~cm^2}

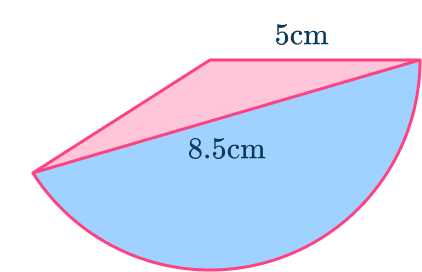
First you need to find the angle using the cosine rule.

\begin{aligned} \cos{A}&=\cfrac{b^{2}+c^{2}-a^{2}}{2 b c} \\\\ \cos{A}&=\cfrac{5^{2}+5^{2}-8.5^{2}}{2 \times 5 \times 5} \\\\ \cos{A}&=-\cfrac{89}{200} \\\\ A&=\cos^{-1}\left(-\frac{89}{200}\right) \\\\ A&=116.4233388^{\circ} \end{aligned}

\begin{aligned} \text{Area of sector }&=\cfrac{\theta}{360} \, \pi r^{2}\\\\ &=\cfrac{116.423}{360}\times \pi \times 5^{2}\\\\ &=25.39955844 \end{aligned}

\begin{aligned} \text{Area of triangle }&=\cfrac{1}{2} \, ab \sin C\\\\ &=\cfrac{1}{2}\times 5 \times 5 \sin(116.423)\\\\ &=11.194165 \end{aligned}

Area of segment $=25.39955844-11.194165$

\begin{aligned} \quad \quad &=14.20539344\\\\ &=14.21\mathrm{cm}^{2} \end{aligned}

22.0{~mm}

16.5{~mm}

8.5{~mm}

22.5{~mm}

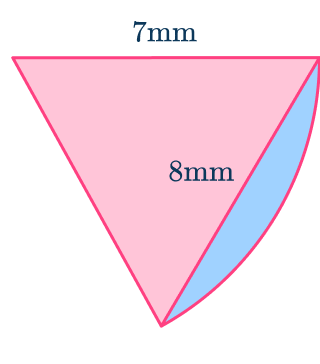
First you need to find the angle using the cosine rule.

\begin{aligned} \cos{A}&=\cfrac{b^{2}+c^{2}-a^{2}}{2 b c} \\\\ \cos{A}&=\cfrac{7^{2}+7^{2}-8^{2}}{2 \times 7 \times 7} \\\\ \cos{A}&=\cfrac{17}{49} \\\\ A&=\cos^{-1}\left(\frac{17}{49}\right) \\\\ A&=69.69980^{\circ} \end{aligned}

\begin{aligned} \text{Arc length}&=\cfrac{\theta}{360} \times 2 \pi r \\\\ &=\cfrac{69.6998}{360} \times 2 \pi \times 7 \\\\ &=8.515436986 \end{aligned}

Chord length: $8{~mm}$

\begin{aligned} \text{Perimeter }&=8.515436986+8\\\\ &=16.515436986\\\\ &=16.5\mathrm{~mm} \end{aligned}

Segment of a circle FAQs

What is the segment of a circle?

A segment of a circle, also called a circular segment, is the area enclosed by an arc of a circle and a chord.

How do you find the area of a segment of a circle?

The area of a segment of a circle can be found by subtracting the area of the triangle formed by the chord and the two radii from the area of the sector defined by the same arc and its corresponding central angle.

How does the area of a segment of a circle differ from the area of the circle?

The area of a segment is a specific portion of the circle’s total area, defined by a chord and the arc it subtends, whereas the area of the circle encompasses the entire space within the circumference.

What is the Alternate Segment Theorem?

The Alternate Segment Theorem states that in a circle, the angle between a chord and the tangent at one end of the chord is equal to the angle in the opposite segment of the circle, subtended by the chord.

The next lessons are

Circle theorems
Prisms
Types of data

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.