High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Simplifying fractions Percent to decimal Percent to fractionPercent
Here is everything you need to know about percentages. You’ll learn how to find the percent of a number, how to calculate with percent multipliers, and how to increase and decrease a number by a percent. You’ll also learn about percent changes.
Students will first learn about percents as part of ratios and proportional relationships in 6th grade.
What is a percent?
A percent is a number that is expressed as a fraction of 100. To do this, you need to know that “percent” means “number of parts per hundred.” The symbol we use for percent is the percent sign \%.
43\%=\cfrac{43}{100}=0.43 1\%=\cfrac{1}{100}=0.01There are different types of questions involving percents.
How to find a percent of a number
This is when you are asked to find a certain percentage of an amount.
For example,
find 25\% of \$32.
This is the same as finding a \cfrac{1}{4} of \$32.
32\div4=8The answer is \$8.
You can also write the percent as a fraction (\cfrac{25}{100}) and then multiply the fraction by the total amount (32).
\cfrac{25}{100}\times32=\cfrac{25}{100}\times\cfrac{32}{1}=\cfrac{800}{100}=8Step-by-step guide: Percent of a number
![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Worksheet (Grade 6 to 7)
![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Worksheet (Grade 6 to 7)
![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREEPercent conversions
Percents, fractions, and decimals can all be used to represent part of a whole.
For example,
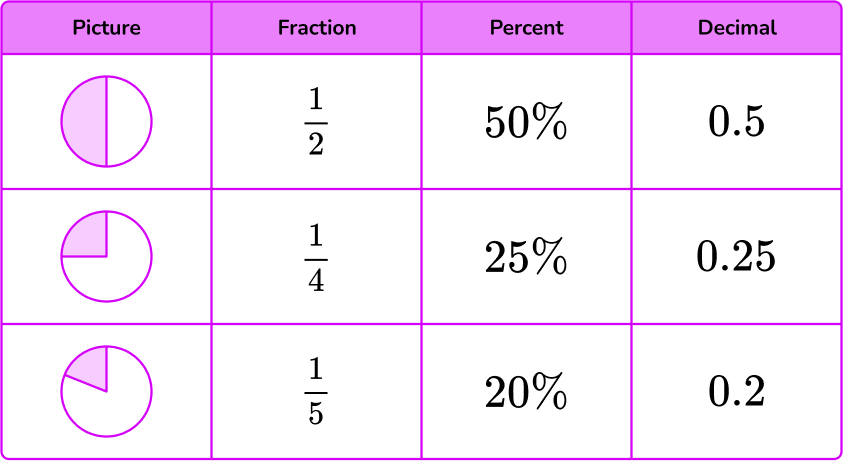
It is useful to be able to convert between percents, fractions, and decimals.
To change a percent into a fraction, write the percent as the numerator of a fraction and 100 as the denominator, and then simplify the resulting fraction if possible.
For example,
42\%= \cfrac{42 \, \div \, 2}{100 \, \div \,2} = \cfrac{21}{50}To change a fraction into a percent, there are two methods.
Method 1 : First, see if it is possible to write an equivalent fraction with a denominator of 100. If so, the numerator will be the percent.
For example,
\cfrac{7}{20} = \cfrac{7 \, \times \, 5}{20 \, \times \, 5}=\cfrac{35}{100}=35\%Method 2 : Carry out the division represented in the fraction and then multiply by 100.
For example,
\cfrac{1}{8} = 1 \div 8 =0.125 0.125 \times 100 = 12.5 \cfrac{1}{8} = 12.5 \%To change a percent into a decimal, divide the percent number by 100.
For example,
73\% = 73 \div 100 = 0.73To change a decimal into a percent, multiply the decimal by 100.
For example,
0.29 0.29 \times 100 = 29 29\%Percent as operator
In order to find a percent of an amount, a percent increase, or a percent decrease, you can use a percent multiplier.
To do this, you change the percent that you want into a decimal, then multiply the amount by that decimal to calculate the answer.
For example,
34\% of 58.
Here you want \bf{34\%}, which as a decimal is \bf{0.34}.
Therefore, the calculation is:
58 \times 0.34 = 19.72For example,
Increase 78 by 15\%.
Here you want an increase of 15\% , which means in total you want \bf{115\%}.
This is because you want another 78 \, (100\%) plus another 15\% of 78 \, ( + 15\%).
115\% as a decimal is \bf{1.15}.
Therefore, the calculation is:
78 \times 1.15 = 89.7For example,
Decrease 45 by 20\%.
Here you want a decrease of 20\%, which means in total you want \bf{80\%}.
Since you want to decrease 45 by 20\%, that means you need to determine 80\% of 45. \, 80\% as a decimal is \bf{0.8}.
Therefore, the calculation is:
45 \times 0.8 = 36Percent increase
This is when you are asked to increase (make bigger) a value by a certain amount.
For example,
increase 40 \, g by 10\%.
You can find 10\% and add it on.
10\% of 40 is 4.
40+4 = 44The answer is 44 \, g.
Step-by-step guide: Percent increase
Percent decrease
This is when you are asked to decrease (make smaller) a value by a certain amount.
For example,
decrease 60 \, kg by 10\%.
You can find 10\% and subtract it.
10\% of 60 is 6.
60-6=54The answer is 54 \, kg.
Step-by-step guide: Percent decrease
Step-by-step guide: Percent increase and decrease
Percent change
When values change, you can express this change as a percent of the original value.
For example,
work out the percent change of 26 \, kg from 25 \, kg.
26-25=1 \cfrac{1 \, \times \, 4}{25 \, \times \, 4}=\cfrac{4}{100}=4\%The answer is 4\%.
Step-by-step guide: Percent change
Percent error
This is when you find the difference between an estimated value and the exact or known value. The difference is then divided by the known value and multiplied by 100\%.
For example,
I estimated that 200 people would attend the concert, but 250 people attended.
250-200=50 50\div250=0.2 0.2\times100=20\%Step-by-step guide: Percent error
Simple interest
This is when you find the percent of an original amount and multiply it by the time period of the investment.
Simple interest = principal amount × rate of interest × time period
For example,
calculate the interest earned on \$3,000 with a simple interest rate of 5\% over 2 years.
\begin{aligned} \text{Simple interest } &= \$3000\times0.05\times2 \\\\ &= \$300 \end{aligned}Step-by-step guide: Simple interest
Reverse percents
This is when you are given a certain percent of a number and you have to find the original number.
For example,
20\% of a number is 6, what is the number?
20\%=\cfrac{1}{5}To find the original number you need to find 100\% or “one whole.”
5\times6=30The answer is 30.
Percent calculations
Let’s look at different methods that can be used to perform percent calculations.
For example,
what is 40\% of 70?
Method
Find 1\% first by dividing the amount by 100 and then multiply the amount by the percent you want.
\cfrac{70}{100} \times 40 = 28Method
Write the percent you want as a decimal and then multiply the amount by this decimal.
40\%=0.4 70 \times 0.4=28Method
Write the percent you want as a fraction in simplest form and then multiply the amount by this fraction.
40\% = \cfrac{40}{100} =\cfrac{4}{10} =\cfrac{2}{5} 70 \times \cfrac{2}{5} = \cfrac{70\times 2}{5} = \cfrac{140}{5} = 28Method
Using simple percents, you can build up the answer to the question.
For this question, if you know 10\% of 70, you can multiply this answer by 4 to find 40\%.
10\% of 70 = 7
40\% of 70 = 7 \times 4 = 28
Note, methods 1 and 2 lend themselves best to questions where you are allowed to use a calculator. Methods 3 and 4 are most helpful when calculators are not permitted.
What is a percent?
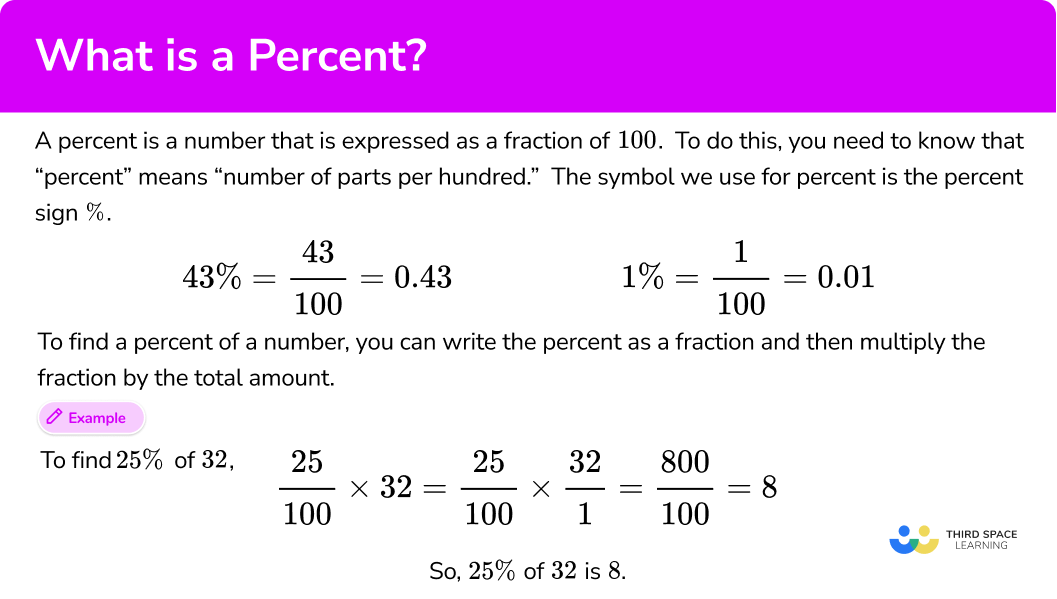
Common Core State Standards
How does this relate to 6th grade math?
- 6th grade – Ratios and Proportional Relationships (6.RP.3c)
Find a percent of a quantity as a rate per 100 (for example, 30\% of a quantity means \frac{30}{100} times the quantity); solve problems involving finding the whole, given a part and the percent.
How to find the percent
In order to work out percents, you need to be clear about what you have and what you are trying to find out. Percent questions can vary and there will be links in the other sections with more details.
- Write down what you have and what you are trying to find.
- Work out what you need.
- Write down the final answer.
Percent examples
Example 1: percent of a number
Find 23\% of \$160.
- Write down what you have and what you are trying to find.
100\% is \$160.
You need to find 23\% of \$160.
2Work out what you need.
Using the one percent method:
\cfrac{160}{100} \times 23 = 1.6 \times 23 = 36.83Write down the final answer.
23\% of \$160 is \bf{\$36.80}.
Example 2: percent multipliers
Find 39\% of \$4,700.
Write down what you have and what you are trying to find.
100\% is \$4,700.
We want to find 39\% of \$4,700.
Work out what you need.
We can write the percent as a decimal number.
39\%=0.39
This gives you the percent in decimal form. This is the percent multiplier.
4700\times0.39=1833
Write down the final answer.
39\% of \$4,700 is \bf{\$1,833}.
Example 3: percent increase
Increase \$200 by 30\%.
Write down what you have and what you are trying to find.
100\% is \$200.
We want to find 130\% of \$200.
100\%+30\%=130\%
Work out what you need.
10\% is 20.
200\div10=20
30\% is 60.
3\times20=60
So 130\% is:
200 + 60 = 260
OR
You could use the decimal multiplier.
100+30=130
130\%=\cfrac{130}{100}=1.30
200\times1.3=260
Write down the final answer.
\$200 increased by 30\% is \bf{\$260}.
Example 4: percent decrease
Decrease 700 \, g by 20\%.
Write down what you have and what you are trying to find.
100\% is 700 \, g.
We want to find 80\% of 700 \, g.
100\%-20\%=80\%
Work out what you need.
10\% is 70.
700\div10=70
20\% is 140.
2\times70=140
So 80\% is:
700-140=560
OR
You could use the decimal multiplier.
100-20=80
80\%=\cfrac{80}{100}=0.80
700\times0.80=560
Write down the final answer.
700 \, g decreased by 20\% is \bf{560 \, g}.
Example 5: percent change
A t-shirt went from \$10 to \$12. Work out the percent change.
Write down what you have and what you are trying to find.
The original amount is \$10.
We need to find the change as a percent of the original amount.
Work out what you need.
The change is 12-10=2.
The change written as a fraction of the original is \, \cfrac{2}{10}.
The change is the numerator (top number). The original number is the denominator (bottom number).
Convert the fraction to an equivalent fraction where the denominator is 100 (the bottom number is 100 ).
\cfrac{2}{10}=\cfrac{20}{100}=20\%
OR
Write the change as a fraction of the original and multiply by 100.
\cfrac{2}{10}\times100=20
Write down the final answer.
The percent change of \$12 from \$10 is \bf{20\%}.
Example 6: reverse percent
A coat costs \$40 after a 20\% price cut. Find the original price.
Write down what you have and what you are trying to find.
Some people call the price after the price cut the “new number.”
100\%-20\%=80\%
We have 80\% = \$40.
We need the original price which is 100\%.
Work out what you need.
80\% is 40.
We can work out 10\%.
40\div8=5
Then you can work out 100\%.
5\times 10 = 50
OR
You can make an equation using x as the original number. Use the decimal multiplier and the new number.
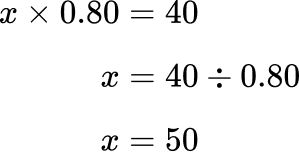
Write down the final answer.
The price after the cut is \$40. The original price was \bf{\$50}.
Teaching tips for percent
- If needed, provide students with hundreds grids to help them understand percent. This will provide them with a visual of 100 and they can fill in squares to represent the percents.
- Provide students with real-world examples and situations as often as possible. This will help them gain a deeper understanding of the content.
- Create an anchor chart to display in the classroom showing each type of percent problem for students to refer to when needed.
Easy mistakes to make
- Money needs two digits for the cents
Find 34\% of \$620.
620\times0.34=210.8
The answer is \$210.80.
- The decimal multiplier to work out a percent increase can be greater than \bf{1}
Increase 50 \, km by 3\%.
We can find 103\%.
50\times1.03 =51.5
The answer is 51.5 \, km.
- Percents can be greater than \bf{100\%}
Calculate the percent change of 450 from 200.
The percent change 450-200=250.
\cfrac{250}{200}\times100=125
So the percent change of 450 from 200 is 125\%.
Percent practice questions
1. Find 40\% of \$300.




10\% of \$300 is \$30. We can multiply this by 4 to get 40\%.
2. Calculate 12.4\% of \$3,000.




As a multiplier, 12.4\% is 0.124. To get the answer you can calculate
0.124\times3000
3. Increase 600 \, m by 30\%.




30\% of 600 is 180. We can add this to the original amount to find the quantity after the increase.
4. Decrease 80 \, kg by 5\%.




5\% of 80 is 4. We can subtract this from the original amount to find the quantity after the decrease.
5. Calculate the percent change from 400 \, kg to 600 \, kg.




The increase is given by:
600-400=200
The percent increase is then given by:
\cfrac{200}{400}\times100=50\%
6. A book costs \$4 after a price reduction of 20\%. What was the original price?




\$4 represents 80\% of the original amount, which means 10\% of the original amount is \$0.50. Multiplying by ten to get 100\% means the original price was \$5.
Practice percent questions – word problems
1. Charlotte invests \$3,000 for 4 years. She gets a simple interest rate of 2\% per year. Find the total interest Charlotte gets.
4\times{60}
=\$240
2. Last year, Ron paid \$450 for his car insurance. This year, he has to pay \$603 for his car insurance. Find the percent increase in his car insurance.
The change is:
603-450=
\cfrac{153}{450}\times 100
=34\%
Percent FAQs
A percent is a number that is expressed as a fraction of 100. The symbol we use for percent is the percent sign \%.
To find a percent of a number, you can write the percent as a fraction and then multiply the fraction by the total amount.
To convert a percent to a decimal, divide it by 100. For example, 25\%=0.25.
The next lessons are
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!