High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Simplifying fractions Percent to decimal Percent to fractionPercent of a number
Here is everything you need to know about finding the percent of a number, including how to solve with or without a calculator.
Students will first learn about finding the percent of a number as part of ratios and proportions in 6th grade.
What is a percent of a number?
The percent of a number can be calculated using a variety of different methods.
For example, what is 35\% of 60?
Method
Start with 60, which is the whole, or 100\%, and work down the table using ratios.
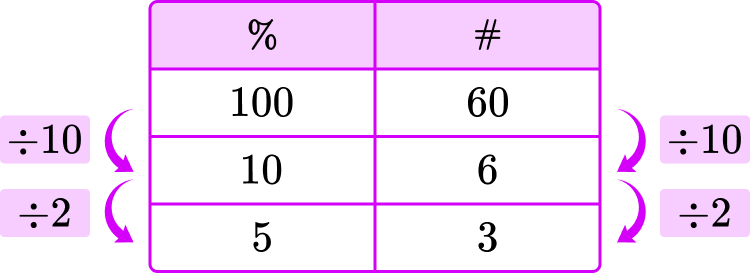
Now use the ratios in the table to find 35\%.
10 \% \times 3=30\% and 6 \times 3=18, so 30\% is 18 and the table shows that 5\% is 3.
\begin{aligned} 35\% \text{ of } 60 &= 18 \, + \, 3=21 \\ & \quad \; ↑ \quad \;\; ↑ \\ & \;\; 30\% \, + \, 5\% \end{aligned}Method
Write the percent as a fraction in its lowest terms and then multiply the number by this fraction.
35\% = \cfrac{35}{100} =\cfrac{7}{20} 60 \times \cfrac{7}{20} = \cfrac{60\times 7}{20} = \cfrac{420}{20} =\cfrac{42}{2}= 21Method
Find 1\% first by dividing the amount by 100 and then multiply the percent (as a whole number).
60 \div 100 \times 35=21Note, this works because 60 \div 100 \times 35=60 \times \cfrac{35}{100}.
Method
First, convert the percent into a decimal, and then multiply the amount by this decimal.
35\%=0.35 60 \times 0.35=21Methods 1 and 2 lend themselves to questions where a calculator is not allowed.
Methods 3 and 4 lend themselves to questions where calculators are allowed.
What is a percent of a number?

![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Worksheet (Grade 6 to 7)
![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Worksheet (Grade 6 to 7)
![[FREE] Percents Worksheet (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREECommon Core State Standards
How does this relate to 6th grade math?
- Grade 6 – Ratios and Proportional Relationships (6.RP.A.3c)
Find a percent of a quantity as a rate per 100 (for example, 30\% of a quantity means \frac{30}{100} times the quantity); solve problems involving finding the whole, given a part and the percent.
How to find the percent of a number
In order to find the percent of a number with or without a calculator:
- Identify the percent and consider if there is a simple equivalent fraction.
- Decide which method to use.
- Work out the answer.
Percent of a number examples
Example 1: simple percent without a calculator
Find 75\% of 120 \ km.
- Identify the percent and consider if there is a simple equivalent fraction.
2Decide which method to use.
You can use 75\% as a fraction to solve or, since 75\% is easily related to 25\% and 50\% , you can use ratios to solve.
3Work out the answer.
Start with 120, which is the whole, or 100\%, and work down the table using ratios.
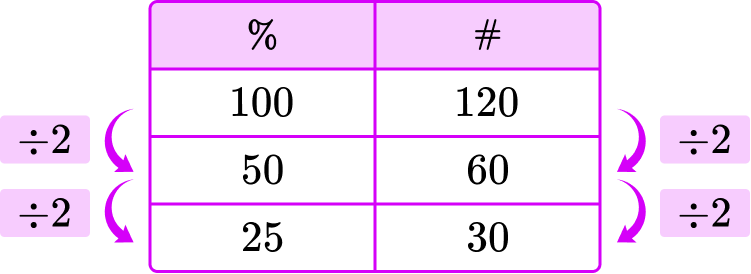
Now use the ratios in the table to find 75\%.
75\% = 50\% + 25\%, so 75\% = 60 + 30.
75\% of 120 is 90.
Example 2: simple percent without a calculator
Find 10\% of 450 \ kg.
Identify the percent and consider if there is a simple equivalent fraction.
Decide which method to use.
To find 10\% , you can solve 450 \times \cfrac{1}{10} \, (which is equal to 450 \div 10 ).
Work out the answer.
OR
450\div 10 = 45
10\% of 450 \ kg is 45 \ kg.
Example 3: percent decomposed to 10% and 1%
Find 16\% of \$300.
Identify the percent and consider if there is a simple equivalent fraction.
Decide which method to use.
You could use a fraction method with any of the equivalent fractions, but since the numbers in the numerator and denominator are not small numbers, there are simpler ways to solve.
In this case, you can break 16\% down into groups of 10\% and 1\%.
Work out the answer.
To find 10\% . you can divide by 10 :
300\div 10 = 30
To find 1\% , you can divide by 100 :
300\div 100 = 3
So, 16\% of 300 is:
\begin{aligned} & \; 30 + (6 \times 3) = 30 + 18 = 48\\ & \;\; ↑ \quad \quad ↑ \\ &10 \%+1 \% \times 6 \end{aligned}
16\% of \$300 is \$48.
Example 4: the 1% method
Find 23\% of 700.
Identify the percent and consider if there is a simple equivalent fraction.
Decide which method to use.
\cfrac{23}{100} \, is in lowest terms, so this relationship can’t be shown with smaller numerators or denominators.
It will be quicker to use a method that doesn’t involve fractions. In this case, you can find 1\% of 700 and use that to find 23\%.
Work out the answer.
To find 1\%, you can divide by 100 :
700\div 100 = 7
So, 23\% of 700 is:
\begin{aligned}
&7 \times 23=161 \\
&↑ \\
&1\%
\end{aligned}
23\% of 700 is 161.
Example 5: decimal multiplier
Work out 78\% of \$411.
Identify the percent and consider if there is a simple equivalent fraction.
Decide which method to use.
You could use a fraction method with any of the equivalent fractions, but since the numbers in the numerator and denominator are not small numbers, there are simpler ways to solve.
In this case, you can convert 78\% to a decimal and multiply by the number to solve.
Work out the answer.
411 \times 0.78=320.58
78\% of \$411 is \$320.58.
Example 6: decimal multiplier
Work out 61\% of 728.
Identify the percent and consider if there is a simple equivalent fraction.
Decide which method to use.
\cfrac{61}{100} is in lowest terms, so this relationship can’t be shown with smaller numerators or denominators. It will be quicker to use a method that doesn’t involve fractions.
In this case, you can convert 61\% to a decimal and multiply by the number to solve.
Work out the answer.
728 \times 0.61=444.08
61\% of \$728 is \$444.08.
Teaching tips for percent of a number
- Worksheets have their place for learning how to find the percent of a number, but they also ensure that students have plenty of opportunities to share their thinking with others. As this page outlines, there are many different ways to go about solving percentage problems, which means there are many opportunities for students to talk and think critically about their work and the work of others.
- There are many different real world situations that students can connect to when learning this topic, such as finding the sales tax on an original price or calculating the sale price based on a percentage decrease.
- Give students time to specifically look for patterns with a calculator as they find different percents of the same number. Ask questions like, “How do you notice the decimal places changing?” or “What do you notice about the percent of a number and the original number?” to encourage student thinking.
- Once students have a foundational understanding of this topic and can flexibly move between strategies, it is okay to let them use a percentage calculator or other online calculators that do the calculations for them. This allows students to shift their focus to more complicated word problems or real-world application for percent of a number.
Easy mistakes to make
- Incorrectly converting from a percent to a decimal
When a percent is represented with a percent symbol, to convert it to a decimal you must divide by 100 (which is the same as moving the decimal point two places to the left) before multiplying.
For example,
What is 53\% of 200?

- Adding or subtracting the same thing in a ratio table
A ratio table can be used to represent the multiplicative relationship between a number and a percent of that number. Adding or subtracting the same thing from both sides does not preserve that relationship.
For example,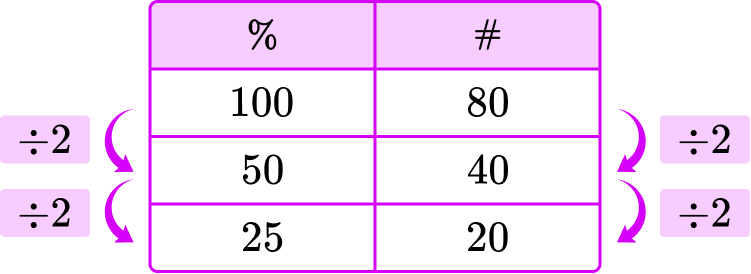
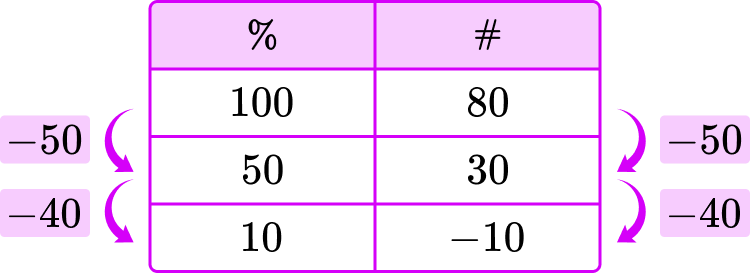


- Making calculation mistakes when multiplying by a fraction or dividing by a whole number
It is a good idea to always check your work after solving (with or without a calculator). A good way to do this is to consider what a reasonable answer should be.
For example,
What is 11\% of 220?
Before solving, notice that 11\% is close to 10\% and 10\% of a number is like dividing by 10 or moving the decimal to the left once, so the answer should be close to 22. If you get an answer that is much smaller or larger than 22, it most likely means you made a mistake calculating and you should check your work.
- Not filling both decimal positions for a money answer
For example, an answer of \$16.5 should be written as \$16.50. There are two digits needed to include the penny position.
Related lessons on percent
Percent of a number practice questions
1. Find 25\% of \$280 without a calculator.




25\% is easily related to 100\% and 50\%, so you can use ratios to solve.
Start with 280, which is the whole, or 100\%, and work down the table using ratios.
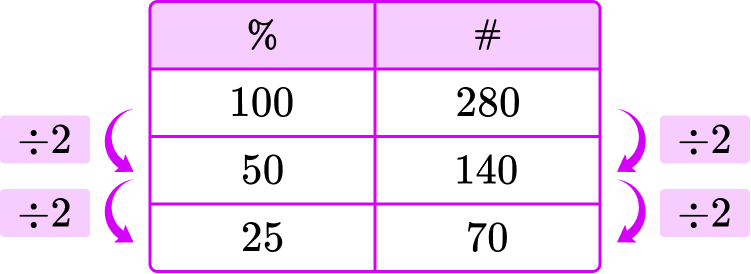
25\% of \$280 is \$70.
2. Find 50\% of 70 \, kg without a calculator.




\cfrac{1}{2} \, is the simplest fraction for 50\%, so it will be the easiest to solve with.
So you can calculate…
\$ 70 \times \cfrac{1}{2}=\cfrac{\$ 70}{2}=\$ 35
OR
\$ 70 \div 2=\$ 35
3. Find 12\% of 200 \, g without a calculator.




10\% of 200 :
200 \div 10 = 20
1\% of 200 :
200 \div 100 = 2
\begin{aligned} 12\%&=10\%+(2 \times 1\%)\\\\ &=20+(2 \times 2)\\\\ &=24 \end{aligned}
4. Find 27\% of 300 \, km without a calculator.




10\% of 300 \, km :
300 \div 10 = 30
1\% of 300 \, km :
300 \div 100 = 3
\begin{aligned} 27\%&=(2 \times 10\%) + (7 \times 1\%)\\\\ &=(2 \times 30) + (7 \times 3)\\\\ &=60+21\\\\ &=81 \, km \end{aligned}
5. Find 57\% of \$710 with a calculator.




Convert the percent to a decimal, then multiply.
57\%=\cfrac{57}{100} = 0.57
\$710 \times 0.57 = \$404.70
6. Find 83\% of \$179 with a calculator.




Convert the percent to a decimal, then multiply.
83\%=\cfrac{83}{100}=0.83
\$179 \times 0.83 = \$148.57
Percent of a number FAQs
A percent is a ratio out of 100, so the denominator of the fraction is always 100 , and the numerator is the percent written as a whole number. To convert this to a decimal, write the percent as a decimal ending in the hundredths column. Note this is true for whole number percents only.
In our number system, percents are a special type of ratio and we use 100 to represent the whole. The word percent broken up is per-cent, per representing a ratio and ‘cent’ meaning 100, like in the word century or 100 cents in a dollar.
The next lessons are
- Compound measures
- Converting fractions, decimals, and percentages
- Exponents
- Algebraic expressions
- Ratio
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!