High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Place value

Multiplying and dividing by 10, 100, 1000

Simplifying a fraction

Math resources Ratio and proportion Converting F D and P

Repeating D to F

Repeating decimal to fraction

Here you will learn about converting a repeating decimal to a fraction including how to define a repeating decimal.

Students will first learn about converting repeating decimals (often called recurring decimals) to fractions as part of the number system in $8$ th grade.

What is converting a repeating decimal to fraction?

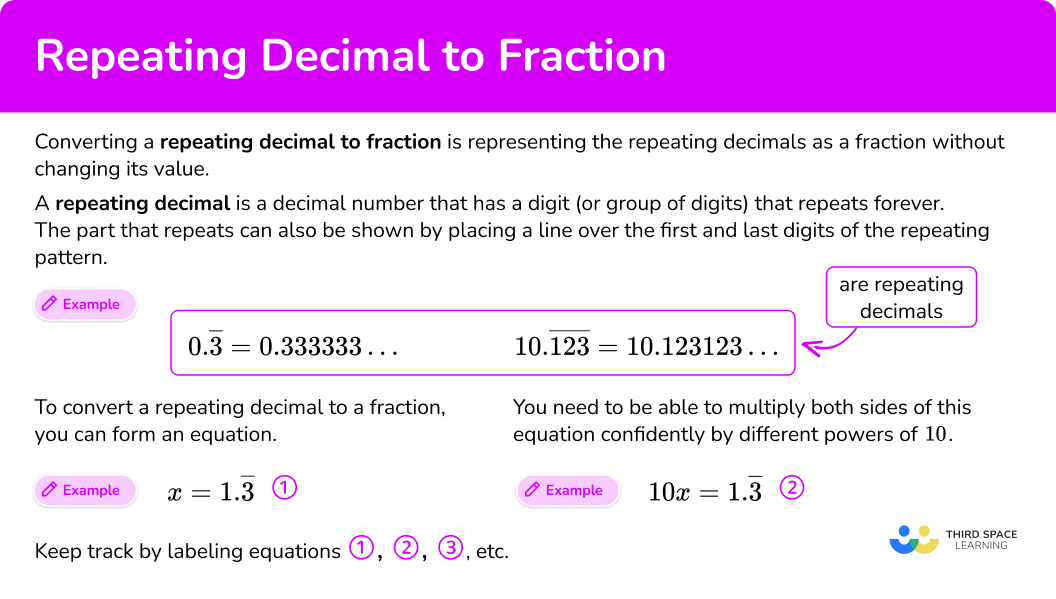
Converting a repeating decimal to fraction is representing the repeating decimals as a fraction without changing its value.

A repeating decimal (recurring decimal) is a decimal number that has a digit (or group of digits) right of the decimal point that repeats forever. The part that repeats can also be shown by placing a line over the first to the last digit of the repeating pattern. Repeating decimals are also known as non-terminating decimals.

For example,

0.\overline{3}=0.333333\ldots

4.\overline{24}=4.242424\ldots

10.\overline{123}=10.123123\ldots

6.5\overline{8}=6.588888\ldots

are all repeating decimals.

To convert a repeating decimal to a fraction, you can form an equation. E.g.

x=1.\overline{3} \hspace{1cm} ①

Keep track by labeling equations $①, ②, ③,$ etc.

You need to be able to multiply both sides of this equation confidently by different powers of $10.$ E.g.

10x=1.\overline{3} \hspace{1cm} ②

100x=13.\overline{3} \hspace{0.65cm} ③

What is converting a repeating decimal to fraction?

$What is converting a repeating decimal to fraction?$

Common Core State Standards

How does this relate to $8$ th grade math?

Grade 8 – The Number System (8.NS.A.1)
Know that numbers that are not rational are called irrational. Understand informally that every number has a decimal expansion; for rational numbers show that the decimal expansion repeats eventually, and convert a decimal expansion which repeats eventually into a rational number.

[FREE] Converting Fractions, Decimals and Percents Worksheet (Grade 4 to 6)

Use this quiz to check your grade 4 to 6 students’ understanding of converting fractions, decimals and percents. 10+ questions with answers covering a range of 4th, 5th and 6th grade converting fractions, decimals and percents topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Converting Fractions, Decimals and Percents Worksheet (Grade 4 to 6)

DOWNLOAD FREE

How to convert a repeating decimal to fraction

In order to convert from a repeating decimal to a fraction, you need to:

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$
Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$
Subtract $\bf{②}$ from $\bf{①}.$
Divide the value by the coefficient of $\textbf{x}.$
Simplify the fraction.
State the answer.

Repeating decimal to fraction examples

Example 1: converting with a simple repeating decimal (one repeated digit)

Convert $0.\overline{1}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

0.\overline{1}=x \hspace{0.8cm} ①

2Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $0.\overline{1}$ has one repeating digit, multiply by $10.$ Remember, because you are multiplying the whole of $①$ by $10$ , you also need to multiply the variable $x$ by $10.$

\begin{aligned}& 0.\overline{1}=x \hspace{0.8cm} ① \\\\ &0.\overline{1}\times 10=x\times10 \\\\ &1.\overline{1}=10x \hspace{0.5cm} ② \end{aligned}

3Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

\begin{aligned}&1.\overline{1}=10x \hspace{1cm} ② \\\\ -&0.\overline{1}=x \hspace{1.35cm} ① \\\\ &1.0=9x \end{aligned}

4Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}&1=9x \\\\ &\cfrac{1}{9}=x\end{aligned}

As $x$ is equal to $0.\overline{1}$ and $\cfrac{1}{9},$ it can be stated that $0.\overline{1}$ and $\cfrac{1}{9}$ are equal to one another.

5Simplify the fraction.

This fraction cannot be simplified as the only factor that $1$ and $9$ share is $1.$

6State your answer.

0.\overline{1}=\cfrac{1}{9}

Example 2: converting with a repeating decimal with simplifying (two repeated digits)

Convert $0.\overline{12}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

0.\overline{12}=x \hspace{0.8cm} ①

Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $0.\overline{12}$ has two repeating digits, multiply by $100.$ Remember, because you are multiplying the whole of $①$ by $100,$ you also need to multiply the variable $x$ by $100.$

$\begin{aligned}& 0.\overline{12}=x \hspace{1.2cm} ① \\\\ &0.\overline{12}\times 100=x\times100 \\\\ &12.\overline{12}=100x \hspace{0.5cm} ② \end{aligned}$

Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

$\begin{aligned}&12.\overline{12}=100x \hspace{1cm} ② \\\\ -&0.\overline{12}=x \hspace{1.7cm} ① \\\\ &12=99x \end{aligned}$

Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}12&=99x \\\\ \cfrac{12}{99}&=x\end{aligned}

Simplify the fraction.

$3$ is the greatest common factor of both $12$ and $99$ so, divide the numerator and the denominator by $3.$

$\cfrac{12\div3}{99\div3}=\cfrac{4}{33}$

State your answer.

0.\overline{12}=\cfrac{4}{33}

Example 3: converting with a repeating decimal (one repeated digit)

Convert $0.0\overline{1}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

0.0\overline{1}=x \hspace{0.8cm} ①

Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $0.0\overline{1}$ has one repeating digit, multiply by $10.$ Remember, because you are multiplying the whole of $①$ by $10$ , you also need to multiply the variable $x$ by $10.$

$\begin{aligned}& 0.0\overline{1}=x \hspace{0.8cm} ① \\\\ &0.0\overline{1}\times 10=x\times10 \\\\ &0.1\overline{1}=10x \hspace{0.5cm} ② \end{aligned}$

Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

$\begin{aligned}&0.1\overline{1}=10x \hspace{1cm} ② \\\\ -&0.0\overline{1}=x \hspace{1.35cm} ① \\\\ &0.1=9x \end{aligned}$

Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}0.1&=9x\\\\ \cfrac{0.1}{9}&=x\end{aligned}

Simplify the fraction.

\cfrac{0.1}{9}=x

Here, notice that there is a decimal as the numerator, so multiply the numerator and the denominator by $10$ to remove the decimal.

$\cfrac{0.1\times10}{9\times10}=\cfrac{1}{90}$

You cannot simplify the fraction $\cfrac{1}{90}.$

State the answer.

0.0\overline{1}=\cfrac{1}{90}

Example 4: converting with a repeating decimal (two repeated digits)

Convert $0.\overline{23}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

0.\overline{23}=x \hspace{0.8cm} ①

Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $0.\overline{23}$ has two repeating digits, multiply by $100.$ Remember, because you are multiplying the whole of $①$ by $100$ , you also need to multiply the variable $x$ by $100.$

$\begin{aligned}& 0.\overline{23}=x \hspace{1.5cm} ① \\\\ &0.\overline{23}\times 100=x\times100 \\\\ &23.\overline{23}=100x \hspace{0.8cm} ② \end{aligned}$

Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

$\begin{aligned}&23.\overline{23}=100x \hspace{1cm} ② \\\\ -&0.\overline{23}=x \hspace{1.7cm} ① \\\\ &23.00=99x \end{aligned}$

Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}23&=99x \\\\ \cfrac{23}{99}&=x\end{aligned}

Simplify the fraction.

This fraction cannot be simplified as the only factor that $23$ and $99$ share is $1.$

State the answer.

0.\overline{23}=\cfrac{23}{99}

Example 5: converting with a repeating decimal greater than 1 (one repeated digit)

Convert $8.\overline{7}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

8.\overline{7}=x \hspace{0.8cm} ①

Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $8.\overline{7}$ has one repeating digit, you will multiply by $10.$ Remember, because you are multiplying the whole of $①$ by $10$ , you also need to multiply the variable $x$ by $10.$

$\begin{aligned}& 8.\overline{7}=x \hspace{1.5cm} ① \\\\ &8.\overline{7}\times 10=x\times10 \\\\ &87.\overline{7}=10x \hspace{1cm} ② \end{aligned}$

Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

$\begin{aligned}&87.\overline{7}=10x \hspace{1cm} ② \\\\ -&8.\overline{7}=x \hspace{1.55cm} ① \\\\ &79=9x \end{aligned}$

Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}79&=9x \\\\ \cfrac{79}{9}&=x\end{aligned}

Simplify the fraction.

This fraction cannot be simplified as the only factor that $79$ and $9$ share is $1.$

State the answer.

8.\overline{7}=\cfrac{79}{9}

Example 6: converting with a more complex repeating decimal (two repeated digits)

Convert $4.0\overline{46}$ to a fraction.

Equate the repeating decimal to a variable, $\textbf{x},$ to create $\bf{①}.$

4.0\overline{46}=x \hspace{0.8cm} ①

Multiply both sides of $\bf{①}$ by a power of $\bf{10}$ to create $\bf{②}.$

As $4.0 \overline{46}$ has two repeating digits, you will multiply by $100.$ Remember, because you are multiplying the whole of $①$ by $100$ , you also need to multiply the variable $x$ by $100.$

$\begin{aligned}& 4.0\overline{46}=x \hspace{1.85cm} ① \\\\ &4.0\overline{46}\times 100=x\times100 \\\\ &404.6\overline{46}=100x \hspace{1cm} ② \end{aligned}$

Subtract $\bf{②}$ from $\bf{①}.$

Subtract the first equation from the second equation.

$\begin{aligned}&404.6\overline{46}=100x \hspace{0.65cm} ② \\\\ -&4.0\overline{46}=x \hspace{1.55cm} ① \\\\ &400.6=99x \end{aligned}$

Divide the value by the coefficient of $\textbf{x}.$

\begin{aligned}400.6&=99x \\\\ \cfrac{400.6}{99}&=x \end{aligned}

Simplify the fraction.

\cfrac{400.6}{99}=x

There is a decimal as the numerator, so you will multiply the numerator (and denominator) by $10.$

$\cfrac{400.6\times10}{99\times10}=\cfrac{4006}{990}$

You can simplify the fraction by dividing the numerator and the denominator by their greatest common factor, $2.$

$\cfrac{4006\div2}{990\div2}=\cfrac{2003}{495}$

State the answer.

4.0\overline{46}=\cfrac{2003}{495}

Teaching tips for converting repeating decimals to fractions

Students should have a firm grasp on what recurring or repeating decimals are before beginning to convert them. Make sure students have a clear definition to refer back to.

Encourage students to identify the repeating pattern in the decimals as the repeating digits. Make sure to emphasize that the recurring part of the decimal repeats indefinitely.

Use of a converting repeating decimals to fractions worksheet will provide students with plenty of opportunities to practice.

Post step-by-step instructions within the classroom or have students write steps down in a math journal to refer back to when needed.

Easy mistakes to make

Multiplying by an incorrect power of $\bf{10}$
You must make sure you are multiplying by the correct power of 10 \; (10, 100, 1000) so the repeating part of the decimal can be eliminated. For example,
Multiplying 0.\overline{13} by 10 does not help us eliminate the repeating decimal by subtraction. In this example you need to multiply by 100 because you can now eliminate the repeating aspect of the decimal by subtraction.
- Incorrect:
  $0 . \overline{13} \times 10=1 . \overline{31}$
  $1.\overline{31}-0.\overline{13}=1.\overline{18}$ does not eliminate the repeating decimal..
- Correct:
  $0.\overline{13}\times100=13.\overline{13}$
  $13.\overline{13}-0.\overline{13}=13$ does eliminate the repeating decimal.

Leaving the fraction with a decimal in the numerator
Fractions should not include a decimal. For example, $\cfrac{0.1}{2}.$
In this example, multiply the numerator and denominator by $10$ to be left with $\cfrac{1}{20}.$

Not simplifying the fraction
Always check to see if you have simplified the fraction into its simplest form.
The easiest way to do this is by using a divisibility check to see if the numerator and denominator are divisible by another number besides $1.$

Practice converting a repeating decimal to fraction questions

1

10

2

5

If the decimal has one repeating digit, then multiply by $10.$

0.\overline{2}\times10=2.\overline{2}

1

10

100

14

If the decimal has two repeating digits, then multiply by $100.$

0.\overline{14}\times100=14.\overline{14}

1

10

100

1000

If the decimal has two repeating digits, then multiply by $100.$

0.0\overline{15}\times100=1.5\overline{15}

\cfrac{2}{9}

\cfrac{2}{10}

\cfrac{2}{99}

\cfrac{2}{999}

Set the repeating decimal equal to a variable and multiply the sides by the correct multiple of $10.$

\begin{aligned}&0.\overline{2}=x \hspace{1cm} ① \\\\ &0.\overline{2}\times10=x\times10 \\\\ &2.\overline{2}=10x \hspace{0.65cm} ② \end{aligned}

Subtract $①$ from $②.$

\begin{aligned}&2.\overline{2}=10x \hspace{1cm} ② \\\\ -&0.\overline{2}=x \hspace{1.35cm} ① \\\\ &2.0=9x \end{aligned}

Divide both sides of the equation by $9$

\cfrac{2}{9}=x

\cfrac{34}{100}

\cfrac{34}{99}

\cfrac{3}{33}

\cfrac{34}{999}

Set the repeating decimal equal to a variable and multiply the sides by the correct multiple of $10.$

\begin{aligned}&0.\overline{34}=x \hspace{2.0cm} ① \\\\ &0.\overline{34}\times100=x\times100 \\\\ &34.\overline{34}=100x \hspace{1.35cm} ② \end{aligned}

Subtract $①$ from $②.$

\begin{aligned}&34.\overline{34}=100x \hspace{1cm} ② \\\\ -&0.\overline{34}=x \hspace{1.7cm} ① \\\\ &34.00=99x \end{aligned}

Divide both sides of the equation by $99$ .

\cfrac{34}{99}=x

This does not simplify further.

6

\cfrac{60}{99}

\cfrac{60}{100}

\cfrac{61}{9}

Set the repeating decimal equal to a variable and multiply the sides by the correct multiple of $10.$

\begin{aligned}&6.\overline{7}=x \hspace{1cm} ① \\\\ &6.\overline{7}\times10=x\times10 \\\\ &67.\overline{7}=10x \hspace{0.65cm} ② \end{aligned}

Subtract $①$ from $②.$

\begin{aligned}&67.\overline{7}=10x \hspace{1cm} ② \\\\ -&6.\overline{7}=x \hspace{1.55cm} ① \\\\ &61.0=9x \end{aligned}

Divide both sides of the equation by $9$ .

\cfrac{61}{9}=x

This does not simplify further.

Repeating decimal to fraction FAQs

Is there a difference between repeating decimal numbers and recurring decimal numbers?

No, both terms can be used synonymously.

What is a surd?

A surd is an irrational number expressed in radical form. They cannot be simplified to whole numbers or fractions.

What is a terminating decimal?

Terminating decimals are decimals that contain a finite number of digits after the decimal point.

Are there decimal numbers that are neither terminating or repeating?

Yes. These are called irrational numbers and include pi $(\pi=3.14159265\ldots)$ and Euler’s number $(e=2.7182818284590\ldots).$

The next lessons are

Ratio
Percent
Compound measures
Proportion

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.