High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Percent Percent of a number Multiplication and division Simplifying fractions Mixed numbers and improper fractions Equivalent fractionsPercent to fraction
Here you will learn about converting percents to fractions.
Students will first learn about converting fractions to percentages in 6th grade math as part of their work with ratios and proportional relationships and will expand that knowledge to solving problems such as finding the whole given a part and the percent or finding the part given the whole and the percent.
This will later be used to find percent increase/decrease in 7th grade.
What is percent to fraction?
Converting a percent to a fraction is representing the percentage as a fraction without changing its value.
The word “percent” means one part out of one hundred, and you can use this information to express a percent as a fraction.
For example,
\begin{aligned} 25\% &=\cfrac{1}{4} \\\\ 45\% &=\cfrac{9}{20} \\\\ 33.3\% &=\cfrac{1}{3} \\\\ 80\% &=\cfrac{4}{5} \end{aligned}
What is percent to fraction?
Common Core State Standards
How does this apply to 6th grade math?
- Ratios and Proportional Relationships (6.RP.A.3a)
Use ratio and rate reasoning to solve real-world and mathematical problems, for example, by reasoning about tables of equivalent ratios, tape diagrams, double number line diagrams, or equations.
a. Make tables of equivalent ratios relating quantities with whole-number measurements, find missing values in the tables, and plot the pairs of values on the coordinate plane. Use tables to compare ratios.
How to convert percentages to fractions
In order to convert from a percentage to a fraction you need to:
- Divide the percentage by \bf{100} .
- Write in fraction form.
- Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
- Simplify the fraction to lowest terms.
- Clearly state the answer showing ‘percentage’ = ‘fraction’.
![[FREE] Converting Fractions, Decimals and Percents Check for Understanding Quiz (Grade 4 to 6)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Converting-fractions-decimals-and-percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Converting Fractions, Decimals and Percents Check for Understanding Quiz (Grade 4 to 6)
Use this quiz to check your grade 4 to 6 students’ understanding of converting fractions, decimals and percents. 10+ questions with answers covering a range of 4th, 5th and 6th grade converting fractions, decimals and percents topics to identify areas of strength and support!
DOWNLOAD FREE [FREE] Converting Fractions, Decimals and Percents Check for Understanding Quiz (Grade 4 to 6)
Use this quiz to check your grade 4 to 6 students’ understanding of converting fractions, decimals and percents. 10+ questions with answers covering a range of 4th, 5th and 6th grade converting fractions, decimals and percents topics to identify areas of strength and support!
DOWNLOAD FREEConverting percentages to fractions examples
Example 1: converting a simple percentage to a fraction
Convert 7\% to a fraction.
- Divide the percentage by \bf{100} .
7 \div 100
2Write in fraction form.
7 \div 100=\cfrac{7}{100}
3Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
The numerator is not a decimal number and therefore already an integer, so you do not need to multiply the numerator and denominator by a multiple of 10.
4Simplify the fraction to lowest terms.
This fraction cannot be simplified because the only factor 7 and 100 share is 1.
5Clearly state the answer showing ‘percentage’ = ‘fraction’.
7\%=\cfrac{7}{100}
You can read this as ‘ 7 hundredths’ or ‘ 7 out of 100 ’.
This is a decimal as the denominator is a power of 10.
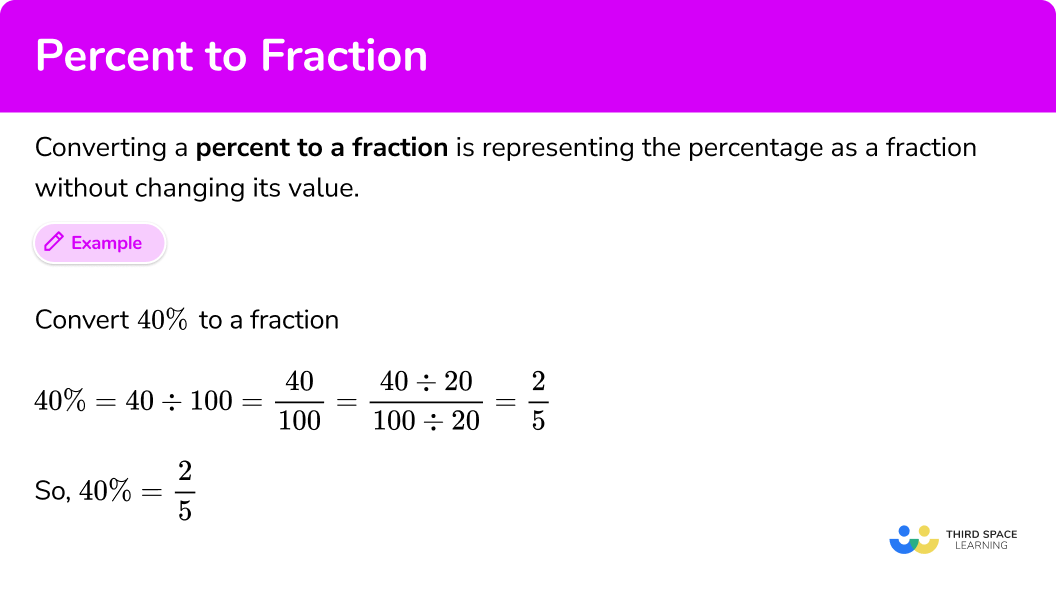
Example 2: converting a percentage to a fraction (with simplifying)
Convert 40\% to a fraction, give your answer in its simplest form.
Divide the percentage by \bf{100} .
40 \div 100
Write in fraction form.
40 \div 100=\cfrac{40}{100}
Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
The numerator is already an integer so you do not need to multiply the numerator and denominator by a multiple of 10.
Simplify the fraction to lowest terms.
\cfrac{40}{100} \,
can be simplified by dividing the numerator and denominator by 20 (the greatest common factor (GCF) or greatest common divisor of 40 and 100 ).
\cfrac{40 \, \div \, 20}{100 \, \div \, 20}
\cfrac{2}{5}
Clearly state the answer showing ‘percentage’ = ‘fraction’.
40\%=\cfrac{2}{5}
Example 3: converting a percentage to a fraction where the percentage contains a decimal
Convert 60.2\% to a fraction.
Divide the percentage by \bf{100} .
60.2 \div 100
Write in fraction form.
60.2 \div 100=\cfrac{60.2}{100}
Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
This is where your knowledge of place value will help.
The lowest value in the number 60.2 is the 2 ‘tenths’ in the first decimal place right of the decimal. This means if you multiply 60.2 by 10, you will end up with the integer value 602.
If you only multiplied the numerator by 10 you would change the value of the whole fraction, so you also need to multiply the denominator by 10.
\cfrac{60.2}{100}
\cfrac{60.2 \, \times \, 10}{100 \, \times \, 10}
\cfrac{602}{1000}
Simplify the fraction to lowest terms.
\cfrac{602}{1000} \,
can be simplified by dividing the numerator and denominator by 2 (the GCF of 602 and 1000 ).
\cfrac{602 \, \div \, 2}{1000 \, \div \, 2}
\cfrac{301}{500}
Clearly state the answer showing ‘percentage’ = ‘fraction’.
60.2\%=\cfrac{301}{500}
Example 4: converting a percentage to a fraction (where the answer will be an improper fraction)
Convert 120\% to a fraction. Give your answer as an improper fraction.
Divide the percentage by \bf{100} .
120 \div 100
Write in fraction form.
120 \div 100=\cfrac{120}{100}
Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
The numerator is already an integer so you do not need to multiply the numerator and denominator by a multiple of 10.
Simplify the fraction to lowest terms.
\cfrac{120}{100} \,
can be simplified by dividing the numerator and denominator by 20 (the GCF of 120 and 100 ).
\cfrac{120 \, \div \, 20}{100 \, \div \, 20}
\cfrac{6}{5}
Clearly state the answer showing ‘percentage’ = ‘fraction’.
120\%=\cfrac{6}{5}
\cfrac{6}{5} \, is an improper fraction as the numerator is greater than the denominator.
Example 5: converting a percentage to a fraction (where the answer will be a mixed number)
Convert 150\% to a fraction. Give your answer as a mixed number.
Divide the percentage by \bf{100} .
150 \div 100
Write in fraction form.
150 \div 100=\cfrac{150}{100}
Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
The numerator is already an integer so you do not need to multiply the numerator and denominator by a multiple of 10.
Simplify the fraction to lowest terms.
\cfrac{150}{100} \,
can be simplified by dividing the numerator and denominator by 50 (the GCF of 150 and 100 ).
\cfrac{150 \, \div \, 50}{100 \, \div \, 50}
\cfrac{3}{2}
Clearly state the answer showing ‘percentage’ = ‘fraction’.
150\%=\cfrac{3}{2}
However,
\cfrac{3}{2} \, is not a mixed number, it is an improper fraction. You therefore need to convert it.
\cfrac{3}{2}=1 \, \cfrac{1}{2}
Therefore,
150\%=1 \, \cfrac{1}{2}
Example 6: converting a percentage to a fraction
Convert 0.008\% to a fraction, give your answer in its simplest form.
Divide the percentage by \bf{100} .
0.008 \div 100
Write in fraction form.
0.008 \div 100=\cfrac{0.008}{100}
Convert the numerator to an integer by multiplying by a power of \bf{10} , for example, \bf{10, 100, 1000} . You need to do the same to the denominator to create an equivalent fraction.
The lowest value in the number 0.008 is the third digit after the decimal point, 8 ‘thousandths’. This means if you multiply 0.008 by 1000 you will end up with the integer value 8.
If you only multiplied the numerator by 1000 , you would change the value of the whole fraction, so you also need to multiply the denominator by 1000. For example:
\cfrac{0.008}{100}
\cfrac{0.008 \, \times \, 1000}{100 \, \times \, 1000}
\cfrac{8}{100000}
Simplify the fraction to lowest terms.
\cfrac{8}{100000} \,
can be simplified by dividing the numerator and denominator by 8 (the GCF of 8 and 100000 ).
\cfrac{8 \, \div \, 8}{100000 \, \div \, 8}
\cfrac{1}{12500}
Clearly state the answer showing ‘percentage’ = ‘fraction’.
0.008\%=\cfrac{1}{12500}
Teaching tips for percent to fraction
- Use visual models such as hundreds grids or pie charts to illustrate the equivalence of percents and fractions and to demonstrate how both forms are ways to represent a part of a whole or a rate.
- Use real world contexts to demonstrate how percentages can be thought of as fractions.
- Worksheets for converting percentages to fractions have their place, but make sure that students have a conceptual understanding of the relationship between percentages and fractions.
Easy mistakes to make
- Multiplying by an incorrect multiple of \bf{10}
You must multiply by a power 10 (for example, 10, 100 or 1000 ) that results in the numerator being an integer (a whole number). Use your knowledge of place value to help decide which multiple of 10 to multiply by.
For example,
0.003 \times 10 = 0.03. This is not an integer.
0.003 \times 1000 = 3. This is an integer.
- Simplifying the fraction to lowest terms
Often questions will say “give your answer in simplest form.” Always take a moment to see if the fraction can be simplified. To simplify a fraction, you need to divide the numerator and the denominator by a common factor.
To simplify a fraction to the lowest terms, you must divide the numerator and the denominator by the greatest common factor.
- Not multiplying the denominator by the same number as the numerator
When you multiply the numerator by a multiple of 10 you must do the same for the denominator otherwise you are changing the value of the fraction.
- Converting between a mixed number and an improper fraction
Not correctly converting between numbers in different forms, for example, mixed numbers and improper fractions.
Related converting fractions, decimals, and percentages lessons
Practice percent to fractions questions
1. Convert 10\% to a fraction in its simplest form.
Start by writing the percent number as a fraction over 100.
\cfrac{10}{100}
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 10, the GCF of 10 and 100.
This gives you \, \cfrac{1}{10} \, .
2. Convert 20\% to a fraction in its simplest form.
Start by writing the percent number as a fraction over 100.
\cfrac{20}{100}
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 20, the GCF of 20 and 100.
This gives you \, \cfrac{1}{5} \, .
3. Convert 130\% to a fraction in its simplest form.
Start by writing the percent number as a fraction over 100.
\cfrac{130}{100}
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 10, the GCF of 130 and 100.
This gives you \, \cfrac{13}{10} \, .
4. Convert 0.6\% to a fraction in its simplest form.
Start by writing the percent number as a fraction over 100.
\cfrac{0.6}{100}
Make sure that the numerator is an integer by multiplying it by a power of 10.
In this case, you can make 0.6 an integer by multiplying it by 10.
Multiply the denominator by 10 as well to make an equivalent fraction!
Now you have \, \cfrac{6}{1000}.
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 2, the GCF of 6 and 1000.
This gives you \, \cfrac{3}{500} \, .
5. Convert 3005\% to a fraction in its simplest form.
Start by writing the percent number as a fraction over 100.
\cfrac{3005}{100}
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 5, the GCF of 3005 and 100.
This gives you \, \cfrac{601}{20} \, .
6. Which of the below is the fractional equivalent of 12\%?
Start by writing the percent number as a fraction over 100.
\cfrac{12}{100}
Then simplify the fraction to lowest terms by dividing the numerator and denominator by 4, the GCF of 12 and 100.
This gives you \, \cfrac{3}{25} \, .
Percent to fraction FAQs
In some cases, yes. For instance, if you wanted to express a rate per 100 in fraction form, sometimes this may be easiest to represent with the denominator as 100 given the context, even if it is not in simplest form.
Multiples of 10 are the products of 10 with any other integer. Powers of 10 are also multiples of 10, but more specifically the multiples that can be expressed as 10 to the power of another number, indicating the number of times to multiply 10 to itself.
No. Either the improper fraction or the mixed number answer will be correct. Most often, answers are preferred as improper fractions to represent the rate more clearly.
The next lessons are
- Percent
- Compound measures
- Arithmetic
- Properties of equality
- Decimals
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.
Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!