GCF And LCM

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Understanding multiplication

Add and subtract within 100

Distributive property

Math resources Number and quantity Factors and multiples

GCF and LCM

Here you will learn about GCF and LCM (greatest common factor and least common multiple), including how to find the GCF and LCM of two or more numbers using the prime factorization method and recognize when to find the GCF or the LCM in word problems.

Students will first learn about GCF and LCM as part of the number system in 6th grade.

What is GCF and LCM?

GCF and LCM are two abbreviations for the greatest common factor (GCF) and the least common multiple (LCM).

The greatest common factor (GCF) is the largest whole number that two or more numbers can be divided by. The lowest common multiple (LCM) is the smallest whole number which is a multiple of two or more whole numbers.

Let’s take a look at some examples below:

Example of GCF, also known as the greatest common divisor (GCD) and the highest common factor (HCF).

Find the GCF of $8$ and $12.$

Let’s start by writing the factors of $8$ and $12.$

Factors of ${\bf{8}} {\textbf{: }} 1, 2, 4, 8$
Factors of ${\bf{12}} {\textbf{: }} 1, 2, 3, 4, 6, 12$

There are several numbers that occur in both lists ( $1, 2,$ and $4$ ).

The largest factor that occurs in each list is $4,$ and so the greatest common factor of $8$ and $12$ is $\bf{4}.$

Example of LCM

Find the LCM of $8$ and $12.$

Let’s start by writing the first $12$ multiples of $8$ and $12.$

Multiples of ${\bf{8}} {\textbf{: }} 8, 16, 24, 32, 40, 48, 56, 64, 72, 80, 88, 96$
Multiples of ${\bf{12}} {\textbf{: }} 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, 132, 144$

There are several values that occur in both lists ( $24, 48, 72,$ and $96$ ). The lowest of these is $24,$ hence the least common multiple of $8$ and $12$ is $\bf{24}.$

Prime factor decomposition

To calculate the GCF or LCM of two or more numbers, you can write out a list of factors or multiples as we have above, however, this approach can be very time consuming and can be complicated when dealing with factors and multiples of large numbers ( $3$ digit numbers in particular).

You can therefore use prime factorization to find these values.

The fundamental theorem of arithmetic states that every positive whole number greater than one is either a prime number, or can be written as a product of its prime factors. Every number has a unique set of numbers called prime factors.

By presenting prime factors within a Venn diagram, you can quickly determine both the GCF and LCM of the two or more numbers in the question.

For example,

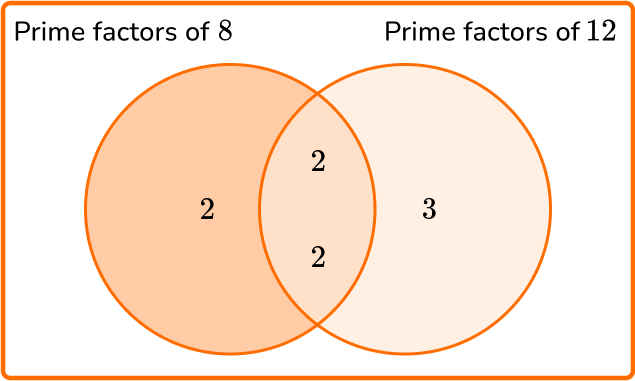
$8=2\times{2}\times{2}$

$12=2\times{2}\times{3}$

The intersection of the two circles contains the greatest common factor, where you multiply the values within the intersection together.

Here, the GCF of $8$ and $12$ is equal to $2\times{2}=4.$

The union of the two circles contains the least common multiple where you multiply the values within both circles together.

Here, the LCM of $8$ and $12$ is equal to $2\times(2\times{2})\times{3}=24.$

As the least common multiple is found by multiplying all of the factors together within the Venn diagram, the least common multiple can be found by multiplying the greatest common factor by the remaining prime factors.

\text{LCM } = \text{ GCF } \times \text{ Remaining prime factors}

This allows you to solve problems where you are given the GCF and LCM of two numbers and you need to determine the original two numbers.

What is GCF and LCM?

Common Core State Standards

How does this relate to 6th grade math?

Grade 6 – The Number System (6.NS.4)
Find the greatest common factor of two whole numbers less than or equal to $100$ and the least common multiple of two whole numbers less than or equal to $12.$ Use the distributive property to express a sum of two whole numbers $(1-100)$ with a common factor as a multiple of a sum of two whole numbers with no common factor. For example, express $36 + 8 \,$ as $\, 4 (9 + 2).$

[FREE] GCF And LCM Worksheet (Grade 6)

Use this worksheet to check your 6th grade students’ understanding of GCF and LCM. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

[FREE] GCF And LCM Worksheet (Grade 6)

Use this worksheet to check your 6th grade students’ understanding of GCF and LCM. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

How to find the greatest common factor

In order to find the greatest common factor of two or more numbers:

State the product of prime factors for each number.
Write all the prime factors for each number into a Venn diagram.
Multiply the prime factors in the intersection to find the GCF.

How to find the least common multiple

In order to find the least common multiple of two or more numbers:

State the product of prime factors for each number.
Write all the prime factors for each number into a Venn diagram.
Multiply each prime factor in the Venn diagram to find the LCM.

GCF and LCM examples

Example 1: GCF of two simple composite numbers

Find the greatest common factor of $30$ and $42.$

State the product of prime factors for each number.

30=2\times{3}\times{5}

42=2\times{3}\times{7}

2Write all the prime factors for each number into a Venn diagram.

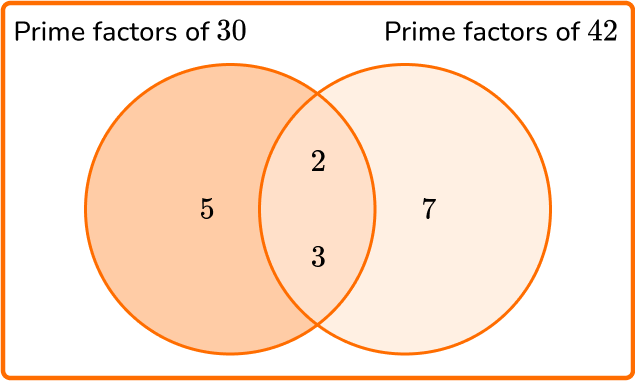
3Multiply the prime factors in the intersection to find the GCF.

GCF $=2\times{3}=6.$

Example 2: LCM of two simple composite numbers

Calculate the least common multiple of $16$ and $18.$

State the product of prime factors for each number.

$16=2\times{2}\times{2}\times{2}$

$18=2\times{3}\times{3}$

Write all the prime factors for each number into a Venn diagram.

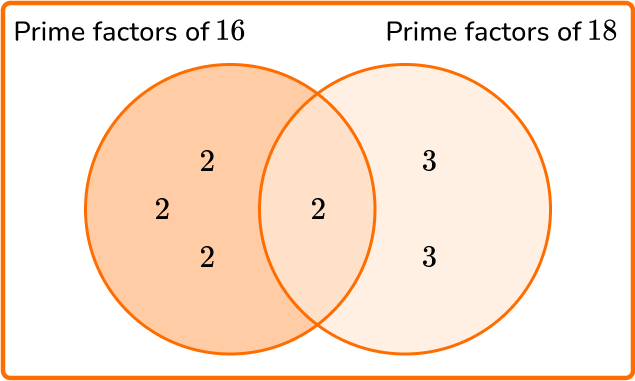
Multiply each prime factor in the Venn diagram to find the LCM.

LCM $=(2\times{2}\times{2})\times{2}\times(3\times{3})=8\times{2}\times{9}=144.$

Example 3: GCF word problem

$120 \ ml$ of red paint and $156 \ ml$ of blue paint are mixed together to create a tin of purple paint. The paint is then distributed equally into sample tubes. Each tube must contain the same amount of paint that must be over $20 \ ml.$

What is the maximum number of tubes that can be filled with the minimum amount of paint?

State the product of prime factors for each number.

$120=2\times{2}\times{2}\times{3}\times{5}$

$156=2\times{2}\times{3}\times{13}$

Write all the prime factors for each number into a Venn diagram.

Multiply the prime factors in the intersection to find the GCF.

GCF $=2\times{2}\times{3}=12.$

The total amount of paint is $120 + 156 = 276 \ ml.$

Dividing $276 \ ml$ into $12$ equal shares (the GCF), we have

$276\div{12}=23.$

As each tube must contain over $20 \ ml$ of paint, we must have $12$ tubes, each containing $23 \ ml$ of paint.

Example 4: LCM word problem

A plumber is fixing multiple leaking pipes. Pipe $A$ drips water every $12$ seconds. Pipe $B$ drips water every $22$ seconds. Both pipes drip at the same time. How much time passes before they next drip at the same time? Write your answer using minutes and seconds.

State the product of prime factors for each number.

$12=2\times{2}\times{3}$

$22=2\times{11}$

Write all the prime factors for each number into a Venn diagram.

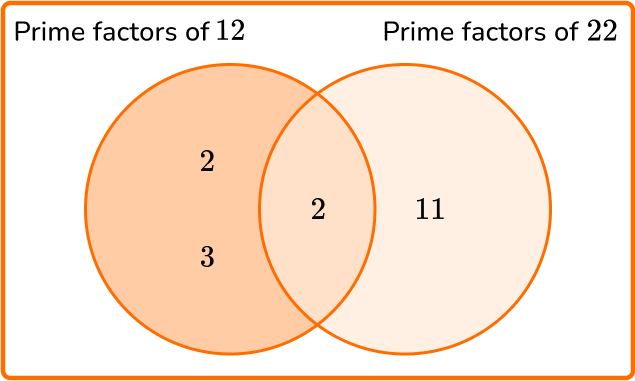
Multiply each prime factor in the Venn diagram to find the LCM.

LCM $=(2\times{3})\times{2}\times{11}=6\times{2}\times{11}=132$

$132$ seconds pass. Converting this to minutes and seconds is $2$ minutes and $12$ seconds ( $60 + 60 + 12 = 132$ , with $60$ seconds $= 1$ minute).

How to find the original values given the GCF and the LCM

In order to find the original values given the GCF and the LCM:

Divide the LCM by the GCF.
Calculate the product of primes of the remainder.
Determine which prime factors match each original number.

Example 5: find the numbers, given the GCF

The greatest common factor of $3$ numbers is $7.$ The product of their remaining prime factors is $30$ and each number is greater than $10.$ Determine the value of the three numbers.

Divide the LCM by the GCF to determine the remainder.

As we already know the remainder ( $30$ ), we can move on to step 2.

Calculate the product of primes of the remainder.

Using a prime factor tree, the product of primes for $30$ is:

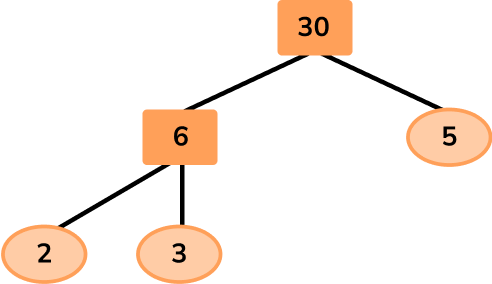
$30=2\times{3}\times{5}$

Determine which prime factors match each original number.

As each value is greater than $10,$ the GCF $7$ must be a factor of all $3$ numbers and it must be multiplied by another factor. $30$ has $3$ prime factors, $2, 3,$ and $5$ and so the original three numbers are:

$A=7\times{2}=14$

$B=7\times{3}=21$

$C=7\times{5}=35$

Example 6: find the original numbers given the GCF and LCM

Two numbers, $A$ and $B,$ have the following number properties:

GCF $(A,B) = 7$
LCM $(A,B) = 2,310$
$A$ is divisible by $3$
$B$ is an even number
$100<A<B$

Determine the values of $A$ and $B.$

Divide the LCM by the GCF to determine the remainder.

$2310\div{7}=330$

Calculate the product of primes of the remainder.

Using a prime factor tree, the product of primes for $330$ is:

$330=2\times{3}\times{5}\times{11}$

Determine which prime factors match each original number.

As $A$ is divisible by $3,$ two factors of $A$ must be $3$ and $7$ (the GCF).

As $B$ is even, two factors of $B$ must be $2$ and $7$ (the GCF).

Writing this up so far, we have

$A=3\times{7}\times{x}$

$B=2\times{7}\times{y}$

As $330=2\times{3}\times{5}\times{11},$ we have the remaining factors of $5$ and $11$ to place.

As $100<A<B,$ both $A$ and $B$ are greater than $100$ with $A$ being smaller than $B.$ The only way this is possible is by making $x=5$ and $y=11.$

This means that,

$A=3\times{7}\times{5}=105$

$B=2\times{7}\times{11}=154$

The solution is $A = 105$ and $B = 154.$

[FREE] GCF And LCM Worksheet (Grade 6)

Use this worksheet to check your 6th grade students’ understanding of GCF and LCM. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

[FREE] GCF And LCM Worksheet (Grade 6)

Use this worksheet to check your 6th grade students’ understanding of GCF and LCM. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

Tips for teaching GCF and LCM

Before introducing GCF and LCM, students should have a strong understanding of factors and multiples, which they would have first learned in 4th grade. Review these terms and practice listing factors and multiples if needed.

Easy mistakes to make

Finding the GCF instead of the LCM (and vice versa)
A very common misconception is mixing up the greatest common factor with the least common multiple. Factors are composite numbers that are split into smaller factors. Multiples are composite numbers that are multiplied to make larger numbers.

Incorrect evaluation of powers
It is possible to write prime factors into a Venn diagram with their associated exponent or power. This only becomes an issue when the powers are not correctly interpreted. We suggest having students write the prime factors without the use of exponents.
Take, for example, the numbers $12$ and $18.$
$12=2^{2}\times{3}$
$18=2\times{3}^{2}$
Here, $2^{2}=2\times{2}=4$ which is correct, however, the same misconception could then be continued to $3^{2}=3\times{2}=6,$ which is incorrect. Instead, $3^{2}=3\times{3}=9.$ This will have an impact on the value of the GCF and the LCM.

Practice GCF and LCM questions

540

36

6

2

54=2\times{3}\times{3}\times{3}

60=2\times{2}\times{3}\times{5}

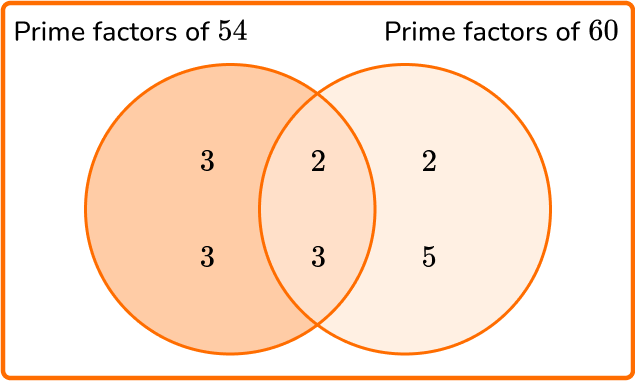
GCF And LCM practice question 1

GCF $(54,60) = 2\times{3}=6$

8

16

96

768

24=2\times{2}\times{2}\times{3}

32=2\times{2}\times{2}\times{2}\times{2}

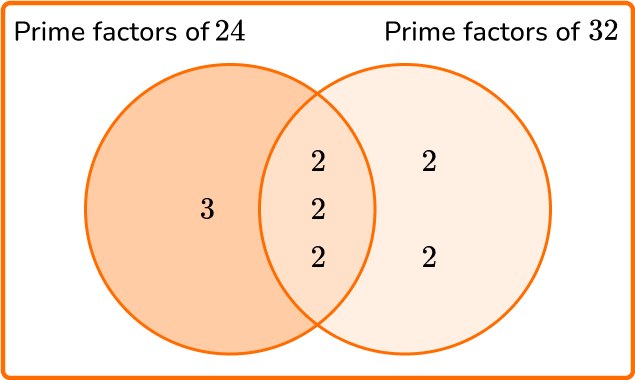
GCF And LCM practice question 2

LCM $(24,32) = 3\times(2\times{2}\times{2})\times(2\times{2})=3\times{8}\times{4}=96$

12 \ cm

40 \ cm

0.1 \ m

240 \ cm

1.2 \ m = 120 \ cm

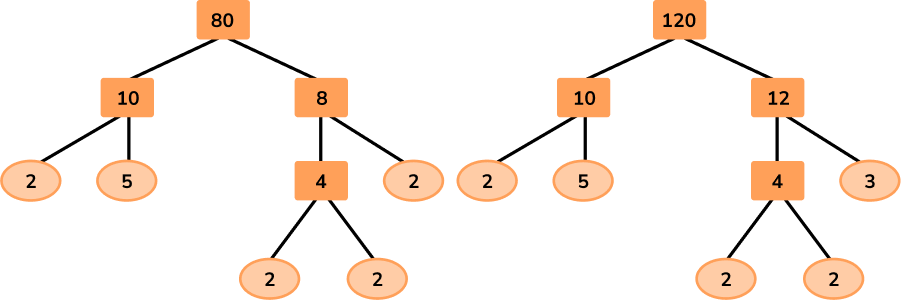
GCF And LCM practice question 3

80=2\times{2}\times{2}\times{2}\times{5}

120=2\times{2}\times{2}\times{3}\times{5}

GCF $(80,120) = 2\times{2}\times{2}\times{5}=40$

1.2 \ km

1 \ km

800 \ m

200 \ m

Converting both lap times to seconds, runner $A$ takes $60$ seconds, and runner $B$ takes $72$ seconds.

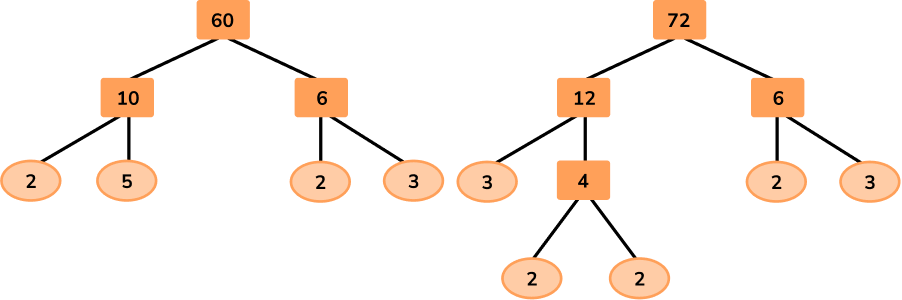
GCF And LCM practice question 4 image 1

60=2\times{2}\times{3}\times{5}

72=2\times{2}\times{2}\times{3}\times{3}

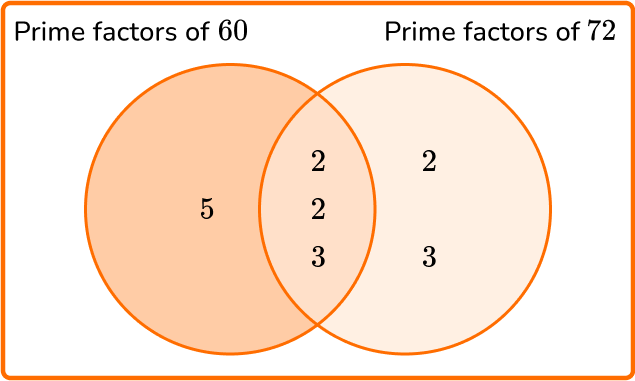
GCF And LCM practice question 4 image 2

GCF $(60,72)=2\times{2}\times{3}=12$

LCM $(60,72) = 5 \times 12 \times (2 \times 3)=5 \times 12 \times 6=360$

$360$ seconds $= 6$ minutes

$6\div{1.2}=5$ laps

5\times{200}=1000 \ m=1 \ km

2

1120

280

385

33=3\times{11}

Smaller number: $35\times{3}=105$

Larger number: $35\times{11}=385$

385-105 = 280

56

64

104

23

96\div{8}=12

12=2\times{2}\times{3}

8\times{2}\times{2}=32

8\times{3}=24

32+24=56

GCF and LCM questions

1. A farm needs to divide their two fields into equal-sized enclosures for some horses. Field $1$ is $240 \ m^2.$ Field $2$ is $160 \ m^2.$ Each horse must have at least $42 \ m^2.$

(a) What is the minimum possible area for each enclosure?

(b) What is the maximum number of horses that can use these two fields?

Show answer

(a)

$240=2^4 \times 3 \times 5 \,$ or $\, 240=2 \times 2 \times 2 \times 2 \times 3 \times 5$

$160=2^5 \times 5 \,$ or $\, 160=2 \times 2 \times 2 \times 2 \times 2 \times 5$

GCF $(240,160)=80 \ m^2$

(b)

$2+3=5 \,$ or $\, (240+160) \div 80=5$

2. Given that $6480=2^4 \times 3^4 \times 5,$ simplify the ratio $10800:6480.$

Show answer

10800=2^4 \times 3^3 \times 5^2

GCF $(10800,6480)=2^4 \times 3^3 \times 5$

Remaining factors are $5$ (for $10800$ ) and $3$ (for $6480$ ).

5:3

3. The least common multiple of $x$ and $y$ is $2^3 \times 3^2 \times 5^2$ where $x$ is a square number such that $36<x<225.$

(a) Find the exact value of $x.$

(b) The greatest common factor of $x$ and $y$ is $4.$ Determine the value of $y.$ Use the Venn diagram below to help you.

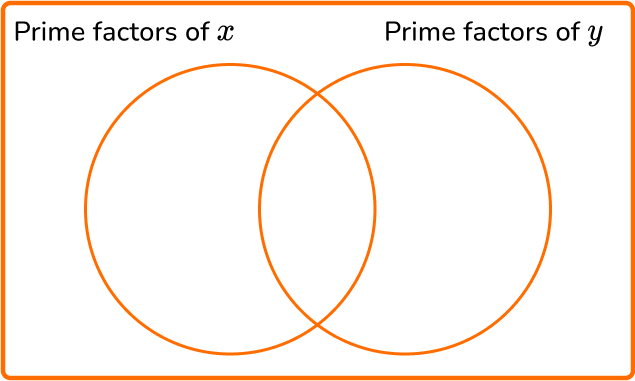
GCF And LCM image 2

Show answer

(a)

$x=2^2 \times 5^2$ or

x=100

(b)

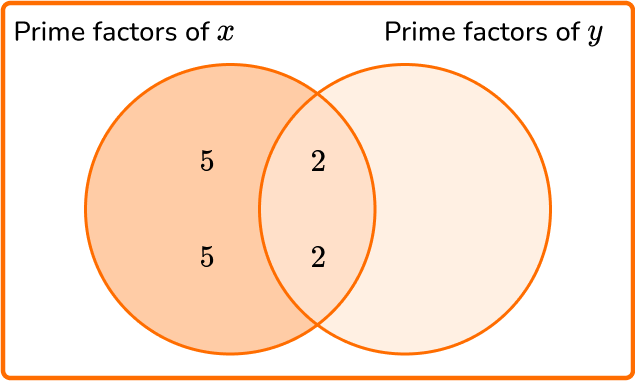
GCF And LCM image 3

GCF And LCM image 4

y=(2 \times 2) \times (2 \times 3 \times 3)=4 \times 18=72

GCF and LCM FAQs

How do you find the GCF?

Step 1: State the product of prime factors for each number.
Step 2: Write all the prime factors for each number into a Venn diagram.
Step 3: Multiply the prime factors in the intersection to find the GCF.

How do you find the LCM?

Step 1: State the product of prime factors for each number.
Step 2: Write all the prime factors for each number into a Venn diagram.
Step 3: Multiply each prime factor in the Venn diagram to find the LCM.

What is the prime factorization method of finding the GCF & LCM?

To find the GCF, list all prime factors that are common between the two numbers and multiply them together. To find the LCM, multiply the GCF by all the prime factors of both numbers that have not yet been used.

What is the difference between least common multiple (LCM) and least common denominator (LCD)?

The least common denominator (LCD) is the least common multiple (LCM) of the denominators of two or more fractions.

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.