High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Exponents Simplifying expressions Order of operationsExponential notation
Here you will learn about exponential notation, including how to write an expression using exponential notation, simplify an expression written in exponent form, simplify expressions involving variables and exponential notations, and calculate the value of a term to the power of zero.
Students will first learn about exponential notation as a part of expressions and equations in 8 th grade math, and will continue to expand on the knowledge throughout high school.
What is exponential notation?
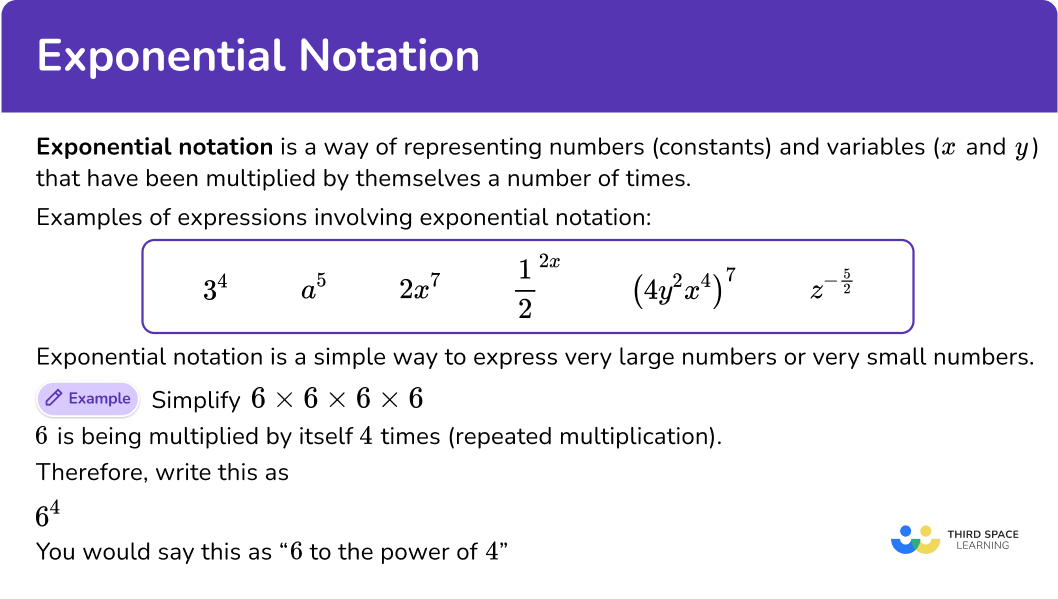
Exponential notation, also known as scientific notation or exponential form, is a way of representing numbers (constants) and variables (x and y) that have been multiplied by themselves a number of times.
Examples of expressions involving exponential notation:
3^{4} \hspace{1cm} a^{5} \hspace{1cm} 2 x^{7} \hspace{1cm} \cfrac{1}{2}^{2 x} \hspace{1cm} \left(4 y^{2} x^{4}\right)^{7} \hspace{1cm} z^{-\frac{5}{2}}Exponential notation is a simple way to express very large numbers or very small numbers. We use exponential notation, or exponents, to simplify expressions or solve equations involving powers, and is a simplified way to represent repeated multiplication and division.
For example,
Simplify \bf{6 \times 6 \times 6 \times 6} .
6 is being multiplied by itself 4 times (repeated multiplication).
Therefore write this as,
6^4You would say this as “6 to the power of 4” .
For example,
Simplify \bf{\textbf{x} \times \textbf{x} \times \textbf{x} \times \textbf{x} \times \textbf{x}} .
x is being multiplied by itself 5 times (repeated multiplication).
Therefore write this as,
x^5You would say this as “x to the power of 5” .
For example,
Simplify \bf{2 \textbf{y} \times 2 \textbf{y} \times 2 \textbf{y} \times 2 \textbf{y}} .
In this question the term 2y is being multiplied by itself 4 times (repeated multiplication).
Therefore write this as,
(2 y)^4Decimal numbers can also be expressed using exponential notation. The standard form for a decimal number is \text { a. } b \times 10^n.
For example,
The number 0.0032 can be written in exponential notation as 3.2 \times 10^{-3}.
![[FREE] Exponential Notation Worksheet (Grade 8)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Exponential-Notation-Worksheet-listing-image.png)
[FREE] Exponential Notation Worksheet (Grade 8)
![[FREE] Exponential Notation Worksheet (Grade 8)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Exponential-Notation-Worksheet-listing-image.png)
Use this worksheet to check your 8th grade students’ understanding of exponential notation. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Exponential Notation Worksheet (Grade 8)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Exponential-Notation-Worksheet-listing-image.png)
[FREE] Exponential Notation Worksheet (Grade 8)
![[FREE] Exponential Notation Worksheet (Grade 8)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Exponential-Notation-Worksheet-listing-image.png)
Use this worksheet to check your 8th grade students’ understanding of exponential notation. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREEWhat are significant figures?
Significant figures are each of the digits of a number that are used to express it to the required degree of accuracy, starting from the first nonzero digit.
Mathematicians use significant figures to ensure that measurements and calculations are presented with precision and accuracy.
Some of the key rules for determining significant figures include:
- All non-zero numbers are considered significant. For example, in the number 123, all three digits are significant (1, 2, 3).
- Leading zeros are not considered significant. For example, in the number 0.0091, only the digits 9 and 1 are considered significant.
- Trailing zeros in a whole number without a decimal point.
What is exponential notation?
Common Core State Standards
How does this relate to 8 th grade math?
- Grade 8: Expressions and Equations (8.EE.A.1)
Know and apply the properties of integer exponents to generate equivalent numerical expressions. For example, 32 \times 3–5 = 3–3 = \cfrac{1}{33} = \cfrac{1}{27}.
How to simplify exponential notation
In order to simplify expressions involving exponential notation, you will:
- Identify whether the base numbers for each term are the same.
- Identify the operation(s) being used.
- Simplify the expression, using the rules of exponential notation.
Simplifying exponential notations examples
Example 1: finding the value of an expression involving exponential notation and multiplication
Simplify 3^2 \times 3^3 .
- Identify whether the base numbers for each term are the same.
The base number is 3 and is the same in each term.
2Identify the operation(s) being used.
The terms are being multiplied.
3Simplify the expression, using the rules of exponential notation.
3^2 is equivalent to 3 \times 3.
3^3 is equivalent to 3 \times 3 \times 3.
Therefore:
\begin{aligned}3^{2} \times 3^{3} &=3 \times 3 \times 3 \times 3 \times 3 \\\\ &=3^{5} \end{aligned}Example 2: simplifying an expression involving unknowns and multiplication
Simplify 3 x^{2} \times 4 x^{3} .
Identify whether the base numbers for each term are the same.
The base number is x and is the same in each term.
3 and 4 are the coefficients of the x terms.
Identify the operation(s) being used.
The terms are being multiplied.
Simplify the expression, using the rules of exponential notation.
3 x^2 is equivalent to 3 \times x \times x .
4 x^3 is equivalent to 4 \times x \times x \times x .
Therefore:
\begin{aligned}3x^{2} \times 4x^{3} &= 3 \times x \times x \times 4 \times x \times x \times x \\\\
&= 3 \times 4 \times x \times x \times x \times x \times x \\\\
&= 12 \times x^{5} \\\\
&= 12x^{5}\end{aligned}
Example 3: finding the value of an expression involving exponential notation and division
Simplify 6^5 \div 6^2 .
Identify whether the base numbers for each term are the same.
The base number is 6 and is the same in each term.
Identify the operation(s) being used.
The terms are being divided.
Simplify the expression, using the rules of exponential notation.
6{ }^5 is equivalent to 6 \times 6 \times 6 \times 6 \times 6 .
6{ }^2 is equivalent 6 \times 6 .
You can write 6{ }^5 \div 6{ }^2 in the form,
\cfrac{6^{5}}{6^{2}}
Therefore:
\begin{aligned}6^{5} \div 6^{2} &= \cfrac{6^{5}}{6^{2}} \\\\ &=\cfrac{6 \times 6 \times 6 \times 6 \times 6}{6 \times 6}\end{aligned}
6 \div 6=1, so keep canceling a 6 on the top with a 6 on the bottom until you are left with,
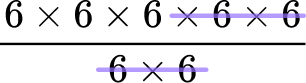
which is just 6 \times 6 \times 6=6^3.
Example 4: simplifying an expression involving unknowns and division
Simplify 10 y^6 \div 5 y^4 .
Identify whether the base numbers for each term are the same.
The base number is y and is the same in each term.
Identify the operation(s) being used.
The terms are being divided.
Simplify the expression, using the rules of exponential notation.
10 y^6 is equivalent to 10 \times y \times y \times y \times y \times y \times y .
5 y^4 is equivalent to 5 \times y \times y \times y \times y .
Therefore:
10y^{6} \div 5y^{4} = \cfrac{10 \times y \times y \times y \times y \times y \times y}{5 \times y \times y \times y \times y}
Since 10 \div 5=2, and canceling the y terms, we are left with
\begin{aligned}\frac{10 \times y \times y \times y \times y \times y \times y}{5 \times y \times y\times y \times y} &= 2 \times y \times y \\\\\ &=2y^{2}\end{aligned}
Example 5: simplifying an expression involving index notation and brackets
Simplify \left(3^2\right)^3 .
Identify whether the base numbers for each term are the same.
The base number is 3 for the term inside the bracket.
Identify the operation(s) being used.
The term 3^2 is “to the power of 3 ”. \left(3^2\right)^3 is equivalent to 3^2 \times 3^2 \times 3^2.
Simplify the expression, using the rules of exponential notation.
Example 6: simplifying an expression involving unknowns and brackets
Simplify \left(2 z^3\right)^5 .
Identify whether the base numbers for each term are the same.
The base number inside the brackets is z.
Identify the operation(s) being used.
The term 2 z^3 is all “to the power of 5 ”.
\left(2 z^3\right)^5 is equivalent to 2 z^3 \times 2 z^3 \times 2 z^3 \times 2 z^3 \times 2 z^3.
Simplify the expression, using the rules of exponential notation.
Teaching tips for exponential notation
- Use language that students can comprehend easily when discussing exponential notation. For example, emphasize that it’s a way to write very large or very small numbers easily, relate it to money or population growth so students can connect easily.
- Compare exponential notation with standard notation with students and allow them to discuss the advantages and disadvantages of both.
- Allow students to use technology, such as calculators and online resources, that allow them to practice and engage with exponential notation.
Easy mistakes to make
- Shifting a decimal place in the wrong direction
The exponent indicates which way the number of places a decimal point should be shifted in the original number. Positive exponents shift the decimal place to the left, and negative exponents shift the decimal place to the right.
For example,- 6 \times 10^4 is equivalent to 6,000 because you will shift the decimal place 4 places to the left of the decimal.
- 1.4 \times 10^{-4} is equivalent to 0.00014 because the decimal point will shift 4 places.
- Identifying the incorrect exponent power
If no exponent power is shown, remember it is being multiplied by itself ‘one time.
For example,
2=2^{1}
- Not including coefficients and brackets when expanding brackets
For example,
(2 x)^{3}≠ 2 x^{3}
This should be (2x)^{3}=2x\times 2x\times 2x=8x^{3}
- Only using exponential notation with whole numbers
You can write fractions and decimals using exponential notation.
For example,
0.5^{3}=0.5\times 0.5\times 0.5=0.125=\cfrac{1}{8}
\cfrac{1}{2}^{3}=\cfrac{1}{2} \times \cfrac{1}{2} \times \cfrac{1}{2}=\cfrac{1}{8}
Related laws of exponents lessons
Exponential notation practice questions
1. Simplify 8 \times 8 \times 8 \times 8 .




We are multiplying 8 by itself 4 times which can be written as 8^{4}.
2. Simplify 3 a \times 3 a \times 3 a \times 3 a .




We are multiplying 3a by itself 4 times which can be written as (3a)^{4}.
3. Simplify 5 x^{5} \times 3 x^{6} .




3 and 5 are the coefficients being multiplied in this expression.
\begin{aligned} 5 x^5&=5 \times x \times x \times x \times x \times x \\\\ 3 x^6&=3 \times x \times x \times x \times x \times x \times x \\\\ 5 x^5 \times 3 x^6&=5 \times x \times x \times x \times x \times x \times 3 \times x \times x \times x \times x \times x \times x \\\\ \quad&=15 x^{11} \end{aligned}
4. Simplify z^{5} \div z^{3} .




The base z is being divided in this expression.
z^5 is equivalent to z \times z \times z \times z \times z.
z^3 is equivalent to z \times z \times z.
Therefore,
\begin{aligned}z^{5} \div z^{3} &= \cfrac{z \times z \times z \times z \times z}{z \times z \times z} \\\\ &=z^{2}\end{aligned}
5. Simplify (3c^{4})^{2} .




The term 3c^{4} is “to the power of 2 ”.
(3c^{4})^{2} is equivalent to (3c^{4})^{2} = 3c^{4} \times 3c^{4}.
\begin{aligned}(3c^{4})^{2} &= 3c^{4} \times 3c^{4} \\\\ &=3 \times c \times c \times c \times c \times 3 \times c \times c \times c \times c \\\\ &=9c^{8}\end{aligned}
6. Simplify \left(3 a^3\right)^2 .




The term 3 a^3 is all “to the power of 2 ”.
\left(3 a^3\right)^2 is equivalent to \left(3 a^3\right)^2=3 a^3 \times 3 a^3.
\begin{aligned} 3 a^3 \times 3 a^3&=3 \times a \times a \times a \times 3 \times a \times a \times a \\\\ & =9 a^6\end{aligned}
Exponential notation FAQS
Significant figures are each of the digits of a number that are used to express it to the required degree of accuracy, starting from the first nonzero digit.
Exponential notation is a general concept that is used across various fields, while engineering notation is a more specific form of exponential notation that is commonly used within the engineering field.
The next lessons are
- Scientific notation
- Math formulas
- Quadratic graphs
- Straight line graphs
- Factoring
- Radicals
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!