Multiplying Exponents - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Exponents Simplifying expressions Order of operations

Math resources Algebra Laws of exponents

Multiplying exponents

Here you will learn about multiplying exponents, including how to apply the properties of exponents when multiplying exponents with the same base and with different bases.

Students will first learn about multiplying exponents as a part of expressions and equations in $8$ th grade math and will expand on that knowledge into high school.

What is multiplying exponents?

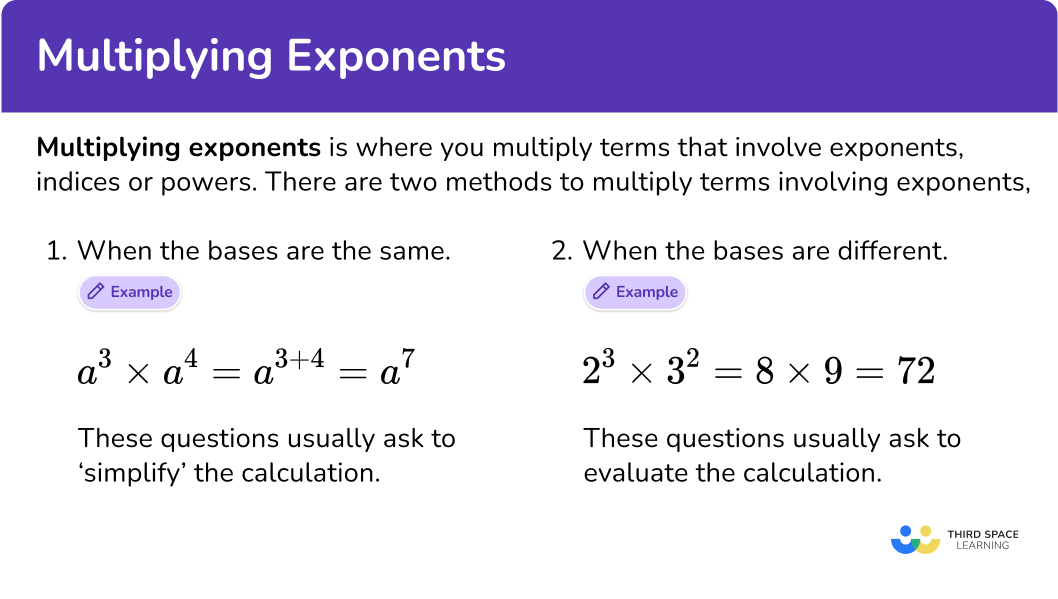
Multiplying exponents is where you multiply terms that involve exponents, indices or powers. You can multiply exponents in various forms, including whole numbers, negative numbers, fractions, and decimals. There are two methods to multiply terms involving exponents,

When the bases are the same, add the exponents.

For example,

$a^{3} \times a^{4}=a^{3 \, + \, 4}=a^{7}$

These questions usually ask to ‘simplify’ the calculation.
When the bases are different, multiply the coefficients and keep the exponent the same.

For example,

$2^{3} \times 3^{2}=8 \times 9=72$

These questions usually ask to ‘evaluate’ the calculation.

What is multiplying exponents?

[FREE] Multiplying Exponents Worksheet (Grade 8)

Use this worksheet to check your 8th grade students’ understanding of multiplying exponents. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Multiplying Exponents Worksheet (Grade 8)

Use this worksheet to check your 8th grade students’ understanding of multiplying exponents. 15 questions with answers to identify areas of strength and support!

DOWNLOAD FREE

Multiplying exponents when the bases are the same

In order to multiply exponents when the bases are the same, you can use one of the laws of exponents.

When multiplying exponents with the same base, add the powers.

$a^{m} \times a^{n}=a^{m \, + \, n}$

For example,

To simplify the following expression,

$8^{3} \times 8^{4}$

Write out each power in its expanded form.

$\begin{aligned} {\color{red} 8^{3}} \times {\color{blue} 8^{4}} &= {\color{red}8 \times 8 \times 8} \times {\color{blue} 8 \times 8 \times 8 \times 8} \\\\ &=8^{7} \end{aligned}$

Is there a quicker way to work this out?

Simplifying

$8^{3} \times 8^{4}$ to $8^{3 \, + \, 4}$

work out the simplified answer as,

$8^{7}$

$8^{3} \times 8^{4}=8^{3 \, + \, 4}=8^{7}$

The base has stayed the same and you have added the exponents together.

This is the multiplication law of exponents.

Multiplying exponents when the bases are different

When multiplying exponents with different bases, multiply the coefficients and keep the exponent the same.

$a^n \times b^n=(a \times b)^n$

For example,

To evaluate the following expression:

$2^{3} \times 3^{2}$

Write each term of the calculation without using exponential notation. The exponent will tell you the number of times to represent the term.

$2^{3} \times 3^{2}=2 \times 2 \times 2 \times 3 \times 3$

$\begin{aligned} 2^{3}&=2 \times 2 \times 2=8 \\\\ 3^{2}&=3 \times 3=9\end{aligned}$

Work out the final answer by multiplying these numbers together.

$\begin{aligned}2^{3} \times 3^{2} &=8 \times 9 \\\\ &=72\end{aligned}$

Common Core State Standards

How does this relate to $8$ th grade math?

Grade 8: Expressions and Equations (8.EE.A.1)
Know and apply the properties of integer exponents to generate equivalent numerical expressions.
For example, $32 \times 3-5=3-3=\cfrac{1}{33}=\cfrac{1}{27} \, .$

How to multiply exponents with the same base

In order to multiply exponents with different bases:

Add the exponents.
Multiply any coefficients.

Multiplying exponents examples

Example 1: exponents with the same base, different exponents

Simplify and leave your answer in exponential form.

$a^{5} \times a^{2}$

Add the exponents.

$5+2=7$

2Multiply any coefficients.

The coefficients are both $1.$

The answer is $a^{7}.$

Example 2: bases have a coefficient greater than 1.

Simplify and leave your answer in index form.

$4 a^{3} \times 7 a^{3}$

Add the exponents.

Add together the exponents $3$ and $3.$

$3+3=6$

Multiply any coefficients.

Multiply $4$ and $7$ together.

$4 \times 7 =28$

The answer is $28a^{6}.$

Example 3: with negative exponents

Simplify and leave your answer in index form.

$7 a^{-6} \times 9 a^{-2}$

Add the exponents.

Add together the exponents $-6$ and $-2.$

$-6+-2=-6-2=-8$

Multiply any coefficients.

Multiply the $7$ and $9$ together.

$7 \times 9=63$

$=63a^{-8}$

How to multiply exponents when the bases are different

In order to multiply exponents with different bases:

Write out each term without the exponents.
Work out the calculation.

Example 4: with positive exponents

Evaluate:

$4^{2} \times 2^{4}$

Write out each term without the exponents.

$4^{2} \times 2^{4}=4 \times 4 \times 2 \times 2 \times 2 \times 2$

Work out the calculation.

$\begin{aligned} 4^{2}&=4 \times 4=16 \\\\ 2^{4}&=2 \times 2 \times 2 \times 2=16 \end{aligned}$

So,

$\begin{aligned}4^{2} \times 2^{4} &=16 \times 16 \\\\ &=256\end{aligned}$

Example 5: with negative exponents

Evaluate:

$3^4 \times 4^{-2}$

Write out each term without the exponents.

When multiplying with a negative exponent, like $4^{-2},$ you will find the reciprocal of the term.

$3^4 \times 4^{-2}=3 \times 3 \times 3 \times 3 \times \cfrac{1}{4} \times \cfrac{1}{4}$

Work out the calculation.

$\begin{aligned}& 3^4=3 \times 3 \times 3 \times 3=81 \\\\ & 4^{-2}=\cfrac{1}{4} \times \cfrac{1}{4}=\cfrac{1}{16}\end{aligned}$

So,

$\begin{aligned}& 3^{4} \times 4^{-2}=81 \times \cfrac{1}{16} \\\\ & 3^{4} \times 4^{-2}=5.0625\end{aligned}$

Example 6: with three terms

Evaluate:

$3^{2} \times 2^{3} \times 10^{2}$

Write out each term without the exponents.

$3^{2} \times 2^{3} \times 10^{2}=3 \times 3 \times 2 \times 2 \times 2 \times 10 \times 10$

Work out the calculation.

$\begin{aligned} 3^{2}&=3 \times 3=9 \\\\ 2^{3}&=2 \times 2 \times 2=8 \\\\ 10^{2}&=10 \times 10=100 \end{aligned}$

So,

$\begin{aligned} 3^{2} \times 2^{3} \times 10^{2} &=9 \times 8 \times 100 \\\\ &=7200\end{aligned}$

Teaching tips for multiplying exponents

Before introducing multiplying exponents, students should be able to simplify expressions with the same base, understand the product rule, the power of a power rule, and the other exponent rules.

Introduce students to real-life scenarios that use multiplying exponents, including scientific notation and compound interest.

Provide worksheets with a range of different types of problems on various levels, including negative exponents, fractions as exponents, and mixed expressions.

Easy mistakes to make

Using the multiplying exponents law with terms with different bases
For example,
You cannot simplify $a^{4} \times b^{3}$ as the bases are different.

Confusing exponents and fractional powers
Raising a term to the power of $2$ means square it.
For example,
$a^{2}=a \times a$

Raising a term to the power of $\cfrac{1}{2}$ means find the square root of it.
For example,
$a^{\frac{1}{2}}=\pm \sqrt{a}$

Raising a term to the power of $3$ means cube it.
For example,
$a^{3}=a \times a \times a$

Raising a term to the power of $\cfrac{1}{3}$ means find the cube root of it.
For example,
$a^{\frac{1}{3}}=\sqrt[3]{a}$

Practice multiplying exponents questions

x^{10}

x^{7}

2x^{7}

10x

The product of the coefficients is $1, 1 \times 1=1.$

The base numbers are the same, so add the exponents.

$5+2=7$

The answer is $x^{7}.$

4b^{-4}

4b^{-21}

4b^{4}

4b^{-10}

The product of the coefficients is $4, 4 \times 1=4.$

Because the same base number is the same, add the exponents.

$3+(-7)=-4$

The answer is $4b^{-4}.$

10a^{2}

24a^{-15}

10a^{-15}

24a^{2}

The product of the coefficients is $24, 6 \times 4=24.$

Because the base is the same number, add the index numbers.

$5+(-3)=2$

The correct answer is $24a^{2}.$

6^{6}

6^{5}

108

36

As the base numbers are different, evaluate each term and then multiply,

$3^{3} \times 2^{2}=27 \times 4=108$

4

32^{0}

32^{-4}

1,024

As the base numbers are different, evaluate each term and then multiply,

$8^{2} \times 4^{-2}=64 \times \cfrac{1}{16}=4$

30^{9}

30^{24}

10,800

720

As the base numbers are different, evaluate each term and then multiply,

$2^{4} \times 5^{2} \times 3^{3}=16 \times 25 \times 27 = 10,800$

Multiplying exponents FAQs

What is an exponent?

An exponent is a small number that is written above and to the right of a number, known as the base number. This indicates how many times a number is multiplied by itself (repeated multiplication). For example, $2^{3},$ read as $2$ to the third power, $2$ is the base number and $3$ is the exponent.

Can you multiply exponents that are fractions or decimals?

Yes, you can still apply the rules of exponents when dealing with exponential expressions that involve decimal or fractional exponents. For example, $a^{\frac{1}{3}} \times a^{\frac{1}{3}}=a(^{\frac{1}{3}}+^{\frac{1}{3}})=a^{\frac{2}{3}}.$ You would find a common denominator, if needed, and then add the numerators similarly to adding fractions.

What is the power of a power property?

To find a power of a power, you will multiply powers, or multiply the exponents. For example, $\left(x^n\right)^m=x^{n \cdot m}.$

What is the rule for dividing with exponents?

When dividing exponents with the same base, subtract the powers to calculate the quotient. For example, $\cfrac{x^n}{x^m}=x^{n-m}.$

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.