High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Simplifying expressions Expanding expressions Combining like termsLinear equations
Here you will learn about linear equations, including how to graph linear equations and how to solve linear equations.
Students will first learn about linear equations as part of expression and equations in 8th grade math and will continue to use them throughout high school.
What is a linear equation?
A linear equation is an algebraic equation that contains variables that have an exponent that is not higher than one. A linear equation is called linear because when you plot the graph of the linear function, it will make a straight line graph.
Examples of equations
- 3 x-5=7
- 2 x+5 y=15
Nonlinear equations are a type of equations where terms can have variables raised to powers other than one, or terms that are multiplied or divided.
Examples of nonlinear equations:
- x^2+y^2=1
- \cfrac{1}{x}+\cfrac{1}{y}=2
All linear equations have an equal sign, this means that everything on the left-hand side of the sign is exactly the same as everything on the right-hand side.
The linear equation formula is the way of expressing a linear equation and can be done in various ways, depending on the number of variables present.
- Standard form
- Slope-intercept form of a linear equation
- Point slope form of a linear equation
The standard form is ax+by=c, where a, b and c are constants.
For example,
\begin{aligned}& 2x-3y+4=0 \\\\ &3y-7x=8 \\\\ &4x=12 \end{aligned}
Slope-intercept form
The slope-intercept form of a linear equation can be written as y=mx+b, where m is the slope of a line and b is the y -intercept.
Point slope form
The point slope form of a linear equation can be written as y-y_1=m\left(x-x_1\right), where m is the slope of the line and \left(x_1, y_1\right) is a point on the line.
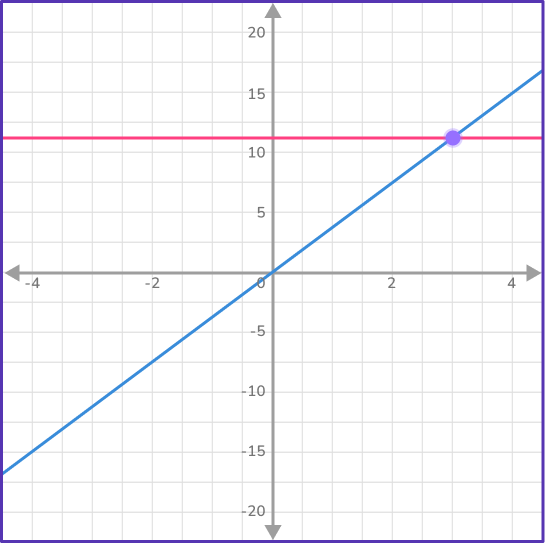
Graphing linear equations
Linear equations are related to coordinate grids, as they provide a way to graphically represent and solve linear equations.
For example,
Given the equation
\begin{aligned}&2x-3y+4=0 \\\\ &3y-7x=8 \\\\ &4x=12\end{aligned}
we can graphically represent this equation on a coordinate grid
A one variable linear equation with the variable x forms a vertical line that is parallel to the y -axis.
For example,
Given the equation x-4=0
You need to isolate the variable by adding 4 to both sides of the equation.
\begin{aligned}& x-4=0 \\\\ & +4+4 \\\\ & x=4\end{aligned}
To graph the line x=4, you will draw a straight, vertical line through point (4,0).
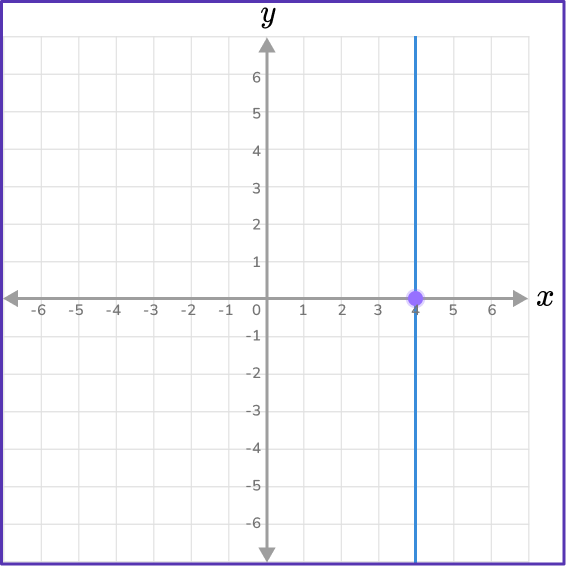
The slope in a vertical line is undefined.
A one variable linear equation with the variable y forms a horizontal line that is parallel to the y -axis.
For example,
Given the equation y+6=0
You need to isolate the variable by subtracting 6 from both sides of the equation.
\begin{gathered}y+6=0 \\\\ -6-6 \\\\ y=-6\end{gathered}
To graph the line y=-6, you will draw a straight, vertical line through point (0,-6).
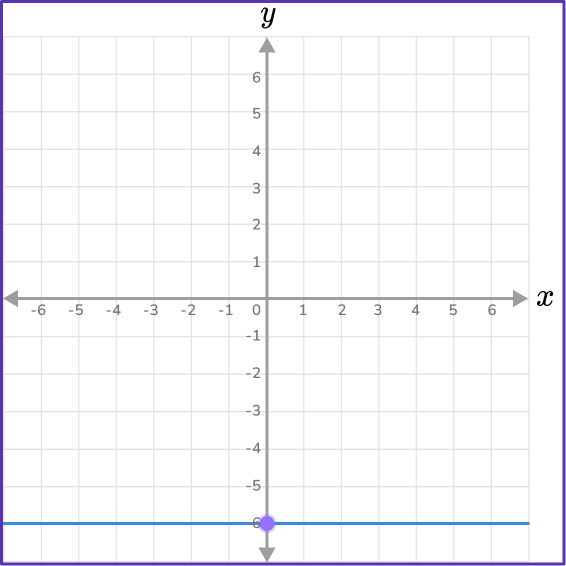
The slope in a horizontal line is 0.
What is a linear equation?
Common Core State Standards
How does this relate to 8 th grade math?
- Grade 8: Expressions and Equations (8.EE.C.7)
Solve linear equations in one variable.
a. Give examples of linear equations in one variable with one solution, infinitely many solutions, or no solutions. Show which of these possibilities is the case by successively transforming the given equation into simpler forms, until an equivalent equation of the form x = a, a = a, or a = b results (where a and b are different numbers).
b. Solve linear equations with rational number coefficients, including equations whose solutions require expanding expressions using the distributive property and collecting like terms.
- High School: Algebra – Reasoning with Equations and Inequalities (HSA.REI.B.3)
Solve linear equations and inequalities in one variable, including equations with coefficients represented by letters.
![[FREE] Linear Equations Worksheet (Grade 7 to High School)](https://thirdspacelearning.com/wp-content/uploads/2024/02/Linear-equations-worksheet-listing-image-1.png)
[FREE] Linear Equations Worksheet (Grade 7 to High School)
Use this worksheet to check your 7th grade to high school students’ understanding of linear equations. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREE [FREE] Linear Equations Worksheet (Grade 7 to High School)
Use this worksheet to check your 7th grade to high school students’ understanding of linear equations. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREEHow to solve linear equations
In order to solve a linear equation, you will need to:
- Simplify both sides of the equation.
- Isolate the variable on one side of the equation.
- Solve for the variable.
Linear equation examples
Example 1: linear equation with one unknown
Solve 3 x+6=18.
- Simplify both sides of the equation.
Both sides of the equation are simplified.
2Isolate the variable on one side of the equation.
Here, the opposite of +6 is -6.
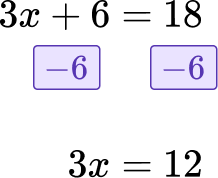
3Solve for the variable.
Here,
3 x=3\times x, so divide by 3.
x=4
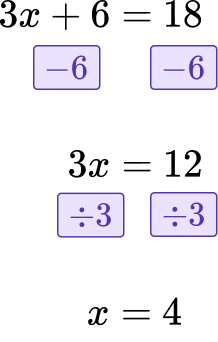
Example 2: linear equation with an unknown on both sides
Solve 5 x+6=2 x+9.
Simplify both sides of the equation.
Here, 2x is smaller than 5x, so eliminate it by -2x.
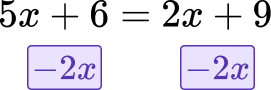
Isolate the variable on one side of the equation.
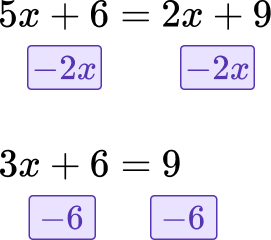
Solve for the variable.
Here,
3x=3\times x, so we divide by 3.
Example 3: linear equation with an unknown on both sides
Solve -3 x-11=-12 x+16.
Simplify both sides of the equation.
Here -3x is smaller than -12x, so eliminate it by adding 3x to both sides.
\begin{aligned} -3 x-11&=-12 x+16 \\\\ +3 x \hspace{0.2cm} & \hspace{0.5cm}+3 x \\\\ -11&=-9 x+16\end{aligned}
Isolate the variable on one side of the equation.
To isolate the variable, subtract 16 from both sides of the equal sign.
\begin{aligned} -11&=-9 x+16 \\\\ -16 & \hspace{0.5cm}-16 \\\\ -27&=-9 x\end{aligned}
Solve for the variable.
To solve for the variable, divide each side by -9.
\begin{aligned} -27&=-9 x \\\\ \div(-9) & \hspace{0.2cm} \div(-9) \\\\ x&=3\end{aligned}
Example 4: solve a linear equation with parentheses
Solve 4(x-2)=12.
Simplify both sides of the equation.
Start by distributing the 4 to the factors within the parentheses.
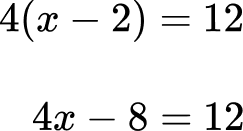
Isolate the variable on one side of the equation.
Here the opposite of -8 is +8, so you will add 8 to both sides.
\begin{aligned}4 x-8&=12 \\\\ +8 & \hspace{0.3cm} +8 \\\\ 4 x&=20\end{aligned}
Solve for the variable.
Because 4x=4\times x, divide by 4 to solve for the variable.
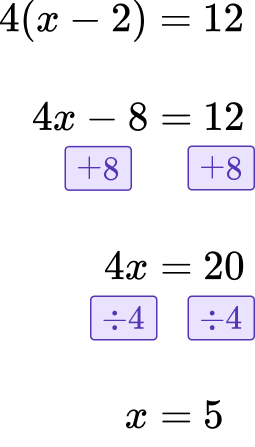
Example 5: linear equation with parentheses
Solve -2(4 x+10)=12.
Simplify both sides of the equation.
Start by distributing the -2 to the factors within the parentheses.
\begin{aligned}& -2(4 x+10)=12 \\\\ & -8 x-20=12\end{aligned}
Isolate the variable on one side of the equation.
The opposite of -20 is +20, so you will add 20 to both sides.
\begin{aligned}-8 x-20&=12 \\\\ +20 & \hspace{0.2cm} +20 \\\\ -8 x&=32\end{aligned}
Solve for the variable.
To solve for the variable, we will divide both sides of the equation by -8.
\begin{aligned}-8 x& =32 \\\\ \div(-8) & \hspace{0.2cm} \div(-8) \\\\ x&=4\end{aligned}
Example 6: linear equation with fractions
Solve \cfrac{5 x-2}{4}=\cfrac{2 x+2}{2} \, .
Simplify both sides of the equation.
Cross multiply, by multiplying each fraction by the denominator on the other side of the equal sign to get rid of the fractions.
\begin{aligned}& (5 x-2) \times(2)=(2 x+2) \times(4) \\\\ & 10 x-4=8 x+8\end{aligned}
Isolate the variable on one side of the equation.
Start by subtracting 8x from both sides of the equation.
\begin{aligned} 10 x-4&=8 x+8 \\\\ - \, 8 x \hspace{0.1cm} & \hspace{0.2cm}-8 x \\\\ 2 x-4&=8\end{aligned}
Then add 4 to both sides of the equation.
\begin{aligned}2 x-4&=8 \\\\ +4 \hspace{0.1cm} & \hspace{0.2cm} +4 \\\\ 2 x&=12\end{aligned}
Solve for the variable.
Solve for x by dividing both sides of the equation by 2.
\begin{aligned} 2 x & =12 \\\\ \div 2 & \hspace{0.2cm} \div 2 \\\\ x&=6\end{aligned}
Teaching tips for linear equations
- Ensure students have a solid understanding of contents, variables, coefficients and terms before beginning linear equations.
- Use visual aids like graphs to help students understand the relationship between linear equations and lines. Show them how to plot linear equations on a coordinate plane and interpret the slope and y -intercept.
- Mistakes are opportunities to reinforce learning. Encourage students to make mistakes, as errors in problem solving can lead to class discussions about the mistakes and how to solve them.
Easy mistakes to make
- Not simplifying both sides of the equations
Both sides of the equal sign (=) must be simplified in order to solve the equation correctly. For example, in order to simplify the equation below, you would subtract two from both sides of the equal sign.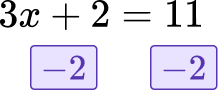
- Making errors when using the distributive property
When distributing a number to multiple terms, make sure to multiply by all of the numbers within parenthesis. For example, 5(x+6), you would multiply the 5 by both x and 6.
Related math equations lessons
- Math equations
- Rearranging equations
- How to find the equation of a line
- Solving equations
- Writing linear equations
- Substitution
- One step equations
- Identity math
- Solve equations with fractions
Practice linear equations problems
1. Solve:
2 x+8=20
2 x+8=20
First, subtract 8 from both sides.
2 x=12
Solve for the variable by dividing both sides by 2.
x=6
2. Solve:
5 x-3=12
5 x-3=12
First, add 3 to both sides.
5 x=15
Then divide both sides by 5.
x=3
3. Solve:
2 x-7=x+5
2 x-7=x+5
First, subtract x from both sides.
x-7=5
Then add 7 to both sides to solve for the variable.
x=12
4. Solve:
7 x-3=4 x+15
7 x-3=4 x+15
Start by subtracting 4x from both sides.
3 x-3=15
Then add 3 to both sides.
3 x=18
To solve for the variable, divide both sides by 3.
x=6
5. Solve:
5(x-3)=10
5(x-3)=10
Start by dividing both sides by 5.
x-3=2
Add 3 to both sides of the equal sign to solve for x.
x=5
6. Solve:
3(x-3)=6(x+5)
3(x-3)=6(x+5)
Start by distributing within the parenthesis.
3 x-9=6 x+30
Then, subtract 3x from both sides.
-9=3 x+30
Subtract 30 from both sides.
-39=3 x
To solve for the variable, divide both sides by 3.
x=-13
7. Solve:
\cfrac{3x+1}{2}+\cfrac{2x-2}{4}
\cfrac{3x+1}{2}+\cfrac{2x-2}{4}
Cross multiply, by multiplying each fraction by the denominator on the other side of the equal sign to get rid of the fractions.
(3 x+1) \times 2=(2 x-2) \times 4
12x+4=4x-4
From both sides of the equation, subtract 4x .
8x+4=-4
and subtract -4
8x=-8
Finally divide by 8.
x=-1
Linear equation FAQs
Yes, a linear equation in two variables can have an infinite amount of solutions. The solutions are all of the points that lie on the line represented by the given equation.
Simultaneous linear equations are a system of linear equations, two or more, with two or more variables. Solving such systems involves finding values for the variables that satisfy all the equations simultaneously.
See also: Systems of linear equations
Quadratic equations are an extension of linear equations, representing a more complex relationship between variables. Linear equations create straight lines, while quadratic equations create a parabola, or a U -shaped curve.
The general form of linear equations is another way to say “standard form”. The standard form, or general form, is ax+by=c, where a, b and c are constants.
The next lessons are
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.
Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!