High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Laws of exponents Algebraic Expression Expanding expressions Factors and multiple GCF and LCM Adding and subtracting integersFactoring out the GCF
Here you will learn about factoring out the GCF, including what the GCF is and how to factor out the GCF with different polynomials.
Students will first learn about factoring out the GCF as a part of expressions and equations in grades 6 and 7. Students will expand on their knowledge pre-algebra and algebra 1.
What is factoring out the GCF?
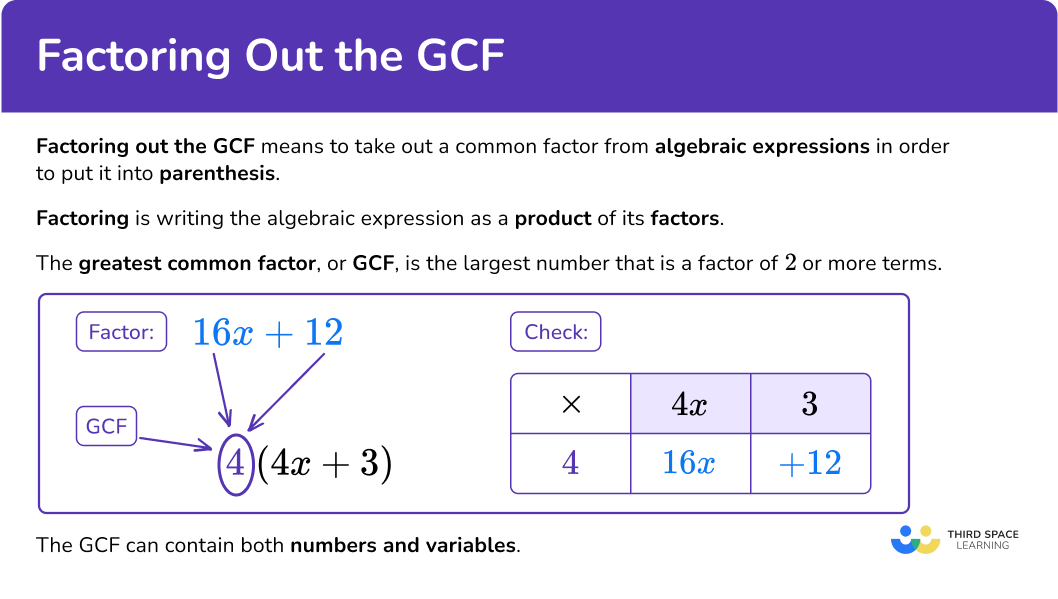
Factoring out the GCF means to take out a common factor from algebraic expressions in order to put it into parenthesis.
Factoring is writing the algebraic expression as a product of its factors.
The greatest common factor, or GCF, is the largest number that is a factor of 2 or more terms.
For example, the GCF of 16x+12 is 4 so the factored form is 4(4x+3). You can check this by multiplying out the parentheses using the distributive property: 4\times{4x}+4\times{3}=16x+12.
Another common factor of 16x+12 is 2 but this is not the greatest common factor.
The GCF can contain both numbers and variables. Example, the GCF of 12x^{2} and 8xy is 4x.
![[FREE] Factoring Out The GCF Worksheet (Grade 7)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Factoring-Out-the-GCF-Worksheet-listing-image.png)
[FREE] Factoring Out The GCF Worksheet (Grade 7)
![[FREE] Factoring Out The GCF Worksheet (Grade 7)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Factoring-Out-the-GCF-Worksheet-listing-image.png)
Use this worksheet to check your 7th grade students’ understanding of factoring out the GCF. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Factoring Out The GCF Worksheet (Grade 7)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Factoring-Out-the-GCF-Worksheet-listing-image.png)
[FREE] Factoring Out The GCF Worksheet (Grade 7)
![[FREE] Factoring Out The GCF Worksheet (Grade 7)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Factoring-Out-the-GCF-Worksheet-listing-image.png)
Use this worksheet to check your 7th grade students’ understanding of factoring out the GCF. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREEFactoring polynomials
A polynomial is an algebraic expression with 2 or more terms, or a sum of one or more monomials. The terms do not include exponents that are negative or fractions.
A monomial is a polynomial containing one term which may be a number or variable.
For example,
3x^{2} is a monomial,
x+5 is a binomial (a polynomial containing two unlike terms)
2x^{2}+x+3y is a trinomial (a polynomial containing 3 or more unlike terms)
What is factoring out the GCF?
Common Core State Standards
How does this relate to 6 th grade math and 7 th grade math?
- Grade 6: Expressions and equations (6.EE.A.3)
Apply the properties of operations to generate equivalent expressions. For example, apply the distributive property to the expression 3(2+x) to produce the equivalent expression 6+3x; apply the distributive property to the expression 24x+18y to produce the equivalent expression 6(4x+3y); apply properties of operations to y+y+y to produce the equivalent expression 3y.
- Grade 7: Expressions and equations (7.EE.A.1)
Apply properties of operations as strategies to add, subtract, factor, and expand linear expressions with rational coefficients.
How to factor out the GCF
In order to factor out the GCF, you will need to:
- Find the GCF of all terms in the expression and place it in front of parentheses.
- Divide each term in the expression by the GCF.
- Put the divided terms in the parentheses.
- Express the GCF and the other factor(s) as products.
Factoring out the GCF examples
Example 1: factoring out GCF
Factor the algebraic expression.
3x+6- Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 3 and 6 separately:
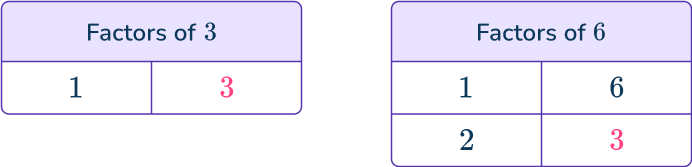
The greatest common factor in the expression is 3.
3(\quad\quad\quad)2Divide each term in the expression by the GCF.
Since 3 is the GCF,
3x\div{3}=x 6\div{3}=23Put the divided terms in the parentheses.
3(x+2)4Express the GCF and the other factor(s) as products.
3x+6=3(x+2)Example 2: variable in just one term
Factor the algebraic expression 14-7y.
Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 14 and 7 separately:

The greatest common factor in the expression is 7.
7(\quad\quad\quad)
Divide each term in the expression by the GCF.
Since 7 is the GCF,
14\div{7}=2
- \, 7y\div{7}=- \, y
Put the divided terms in the parentheses.
Express the GCF and the other factor(s) as products.
Example 3: variable in two terms
Factor the binomial 8x^{2}+12x .
Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 8 and 12 separately:

Then find the greatest common factor of the variables x^2 and x.
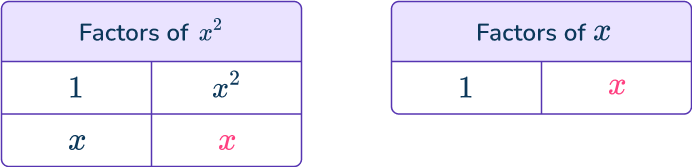
The greatest common factor of 8x^{2} and 12x is 4 \times x=4x.
4x(\quad\quad\quad)
Divide each term in the expression by the GCF.
Since 4x is the GCF,
8x^{2}\div{4x}=2x
12x\div{4x}=3
Put the divided terms in the parentheses.
Express the GCF and the other factor(s) as products.
Example 4: variables in two terms
Factor the binomial 15y^{2}-10xy.
Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 15 and 10 separately:
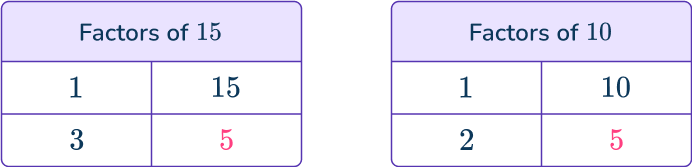
List all the factor pairs of y^2 and xy separately:
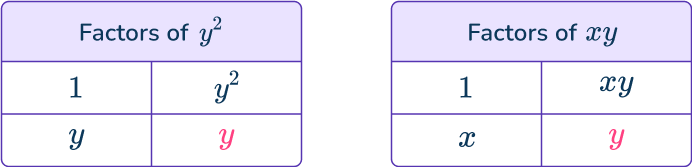
The greatest common factor of 15y^{2} and 10xy is 5y.
5y(\quad\quad\quad)
Divide each term in the expression by the GCF.
Since 5y is the GCF,
15y^{2}\div{5y}=3y
-10xy\div{5y}=-2x
Put the divided terms in the parentheses.
Express the GCF and the other factor(s) as products.
Example 5: factoring trinomials
Factor the trinomial 6x+2y-12.
Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 6, \, 2 and 12 separately:
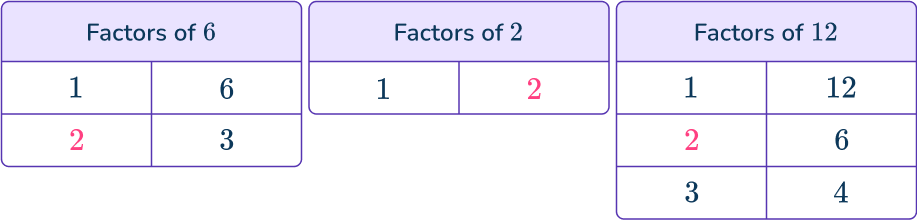
There are no common variables (letters) between the three terms.
The greatest common factor of 6x and 2y and 12 is 2.
2(\quad\quad\quad)
Divide each term in the expression by the GCF.
Since 2 is the GCF,
6x\div{2}=3x
2y\div{2}=y
- \, 12\div{2}=- \, 6
Put the divided terms in the parentheses.
Express the GCF and the other factor(s) as products.
Example 6: variables in three out of three terms
Factor the algebraic expression: 12xy-4x^{3}y+8xy^{2}
Find the GCF of all terms in the expression and place it in front of parentheses.
List all the factor pairs of 12, \, 4 and 8 separately:
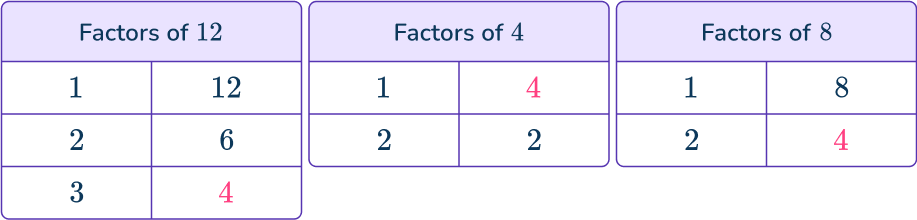
List all the factor pairs of xy,~x^{3}y and xy^{2} separately:
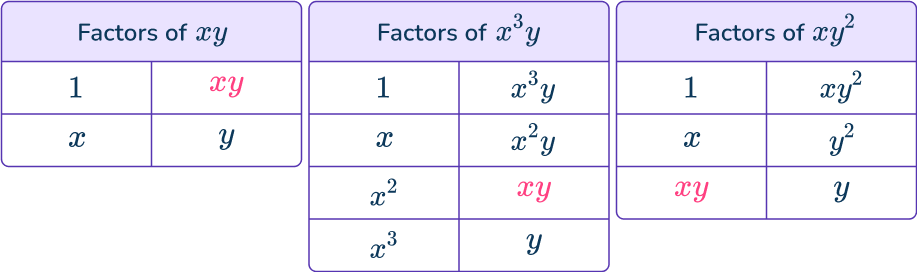
The greatest common factor of 12xy and 4x^{3}y and 8xy^{2} is 4xy.
4xy(\quad\quad\quad)
Divide each term in the expression by the GCF.
Since 4xy is the GCF,
12xy\div{4xy}=3
- \, 4x^{3}y\div{4xy}=- \, x^{2}
8xy^{2}\div{4xy}=2y
Put the divided terms in the parentheses.
Express the GCF and the other factor(s) as products.
Teaching tips for factoring out the GCF
- When worksheets with practice problems need to be used, consider having students work in pairs or small groups to solve the problems. This allows students to explain their reasoning when finding the right answer and work together to solve each problem.
- Provide students with step by step guides and example problems to refer back to.
- Incorporate games when teaching this topic.
Easy mistakes to make
- Making sign errors when factoring out the GCF
It is easy to forget to include the sign when factoring out the GCF. In order to double check your factoring, you can use the distributive property to ensure you have used the correct signs and factored correctly.
- Not factoring the GCF out completely
When factoring, make sure to find the true GCF of all terms.
For example, 12x^{2}-6x=2\left(6x^2-3x\right). Here the expression has been factored, however it is not fully factored because 2 is not the greatest common factor. 6x is the GCF, so the correct final answer is: 12x^{2}-6x=6x(2x-1).
Related factoring lessons
- How to factor quadratic equations
- Factoring the difference of two squares
- Factor by grouping
Practice factoring out the GCF questions
1. Factor the algebraic expression, 5x+10 .




The greatest common factor of 5 and 10 is 5.
5(\quad\quad\quad)
5x\div{5}=x
10\div{5}=2
5 x+10=5(x+2)
2. Factor the algebraic expression,
8-2y .




The greatest common factor of 8 and 2 is 2.
2(\quad\quad\quad)
8\div{2}=4
– \, 2y\div{2}=-y
8-2y=2(4-y)
3. Factor the algebraic expression, 18x^{2}-12x.




The greatest common factor of 18x^{2} and 12x is 6x.
6x(\quad\quad\quad)
Divide each term in the original expression by 6x.
18x^{2}\div{6x}=3x
– \, 12x\div{6x}=-2
18x^{2}-12x=6x(3x-2)
4. Factor the algebraic expression, 20y^{2}+16xy .




The greatest common factor of 20y^{2} and 16xy is 4y.
4y(\quad\quad\quad)
Divide each term in the original expression by 4y.
20y^{2}\div{4y}=5y
16xy\div{4y}=4x
20y^{2}+16xy=4y(5y+4x)
5. Factor the algebraic expression,
18-6y+15x .




The greatest common factor of 18, \, 6y and 15x is 3.
3(\quad\quad\quad)
Divide each term in the original expression by 3.
18\div{3}=6
– \, 6y\div{3}=- \, 2y
15x\div{3}=5x
18-6y+15x=3(6-2y+5x)
6. Factor the algebraic expression, 12y-9x^{2}y+6y^{2} .




The greatest common factor of 12y, \, 9x^{2}y and 6y^{2} is 3y.
3y(\quad\quad\quad)
Divide each term in the original expression by 3y.
12y\div{3y}=4
– \, 9x^2y\div{3y}=- \, 3x^2
6y^{2}\div{3y}=2y
12y-9x^{2}y+6y^{2}=3y\left(4-3x^{2}+2y\right)
Factoring out the GCF FAQs
The GCF is the largest factor that can be divided from all terms within an expression. It can include both numerical coefficients and any variable.
The greatest common factor (GCF) and least common multiple (LCM) are often confused within the math world. The GCF is the largest positive factor that divides all the numbers without leaving a remainder. The LCM is the smallest positive multiple that is divisible by all the numbers without leaving a remainder.
The GCF is the product of the smallest power of all the common prime factors. For example, find the GCF of 18 and 24 using prime factorization.
18=2\times{3^2}
24=2^{3}\times{3}
The common prime factors are 2 and 3.
GCF=2 \times 3=6
The next topics are:
- Radicals
- Rational functions
- Vectors
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!