Get Ahead In Foundation GCSE Maths With This Comprehensive Question & Topic Analysis Of Edexcel GCSE Maths Papers 2017-2023

For all maths teachers leading or teaching Edexcel GCSE Maths, here you have a detailed topic and question level analysis from all years of the foundation level GCSE maths exams since 2017

It’ll take some digesting so please do bookmark and share with colleagues for when you need to refer back.

Please note, this blog focuses on the Edexcel maths past papers, not AQA or OCR exam boards or IGCSE maths. It’s also just GCSE maths not Edexcel A level maths.

GCSE MATHS 2024: STAY UP TO DATE

Join our email list to stay up to date with the latest news, revision lists and resources for GCSE maths 2024. We’re analysing each paper during the course of the 2024 GCSEs in order to identify the key topic areas to focus on for your revision.

Thursday 16th May 2024: GCSE Maths Paper 1 2024 Analysis & Revision Topic List
Monday 3rd June 2024: GCSE Maths Paper 2 2024 Analysis & Revision Topic List
Monday 10th June 2024: GCSE Maths Paper 3 2024 Analysis

GCSE 2024 dates
GCSE 2024 results
GCSE results 2023

Edexcel maths past paper findings

If you are short on time, here are the key takeaways from this past paper analysis. You can read in more detail about each strand later in the article, along with tips for which topics to focus on in the first few questions, common questions, and so on. But if you’re just looking for the headlines, here they are:

Number and proportion

• Plan for plenty of work on context-rich problem solving with basic arithmetic, fractions, and percentages.
• Common real-life contexts include bank accounts, utility bills, wage increases, recipes, and best-buys.
• Place value, standard form, indices, rounding and estimation, factors, multiples, HCF, LCM and product of primes tend to be assessed in more of a procedural manner and could be ‘easy wins’.

Algebra

• Algebra at Foundation tends to be examined in a fairly standard procedural way. However, students find the topics themselves more challenging, so these are not necessarily ‘easier’ marks.
• Spend time ensuring students can apply standard algebraic methods accurately.
• Consider spending less time on simultaneous equations, solving quadratic formula, and equations, in favour of more time on graphs, sequences, and functions.

Ratio and proportion

• As with number and proportion, this strand includes lots of context-rich problem solving involving ratio and proportional reasoning.
• Ratio is frequently embedded in questions relating to other topics, such as shape or angle problems.
• Include more work on time, particularly misconceptions around fractions of an hour, and use of a calculator for time problems.

Geometry

• Include plenty of context-based problem-solving on perimeter, area, volume, and surface area, making sure students can flexibly apply these skills to 2D and 3D shapes and other problems.
• Allow sufficient time for scale drawing work and bearings, and linking this to other topics.

Probability 

• There are plenty of procedural marks up for grabs in probability, including using probability scales, writing simple probabilities, and completing or reading information from tree diagrams.
• There is an increased focus on ‘newer’ topics, such as Venn diagrams, sets, listing outcomes and frequency trees.
• Ensure students can apply fraction and ratio skills to unfamiliar contexts for mutually exclusive events or relative frequency.

Statistics

• Include plenty of practice on presenting data, particularly looking at pros and cons of different types of graphs, and spotting errors in given graphs and charts.
• Estimating the mean and average calculations from frequency tables both come up fairly frequently.
• Data collection and sampling is assessed less frequently.

Background to this research

I first started looking in detail at these papers in 2021, when I used the then available full set of six Edexcel GCSE maths Foundation papers from June 2017 through to November 2019.

Even then I noticed a few striking details: 

The set of papers available raised a few interesting and surprising statistics. For example, approximately two-fifths of the ‘Geometry’ marks were for perimeter, area, and volume, and many of these involved problem-solving in non-standard contexts.

Obviously, as a mathematician, I then wanted to investigate this further – an anomaly or a pattern? (As it turns out, the amount was slightly anomalous, but the assessment of perimeter, area and volume mainly as non-procedural questions was a pattern across all papers).

Since writing that article, we’ve had a few more sets of papers and I’ve had more time to refine my analysis process. The refreshed article has been fully expanded and now covers:

• Frequency analysis by strand and topic – which strands or topics are the heaviest hitters at Foundation level?
• Which topics appear most in the first five questions in Edexcel Foundation papers?
• Which topics appear the most in common or crossover questions at GCSE?
• Which topics are more likely on Maths Paper 1?

Edexcel maths past papers methodology

Categorising by topic

When I began my analysis work in 2021, I applied a broad approach to categorise each question, using only topic and sub-topic (for example, Statistics / Charts and Graphs).

Since then, I have added several more papers and further detail to my data-set, allowing for a more in-depth look at how different GCSE maths topics are examined.

The categorisations are based on the GCSE specification (Edexcel), and align closely with Third Space Learning’s topic categories:

• Algebra
• Number
• Geometry and Measure
• Probability
• Ratio and Proportion
• Statistics

Classifying some of the multi-mark, deeper problem-solving questions presented a bit of a challenge, so I decided to pick a main topic for each question and classify it under that heading, rather than try and assign marks for topics within questions.

So, for example, June 2019 1F Q18 required candidates to use skills working with four operations and decimal calculation as well as area. However, as the question is inaccessible without some knowledge of the area of a rectangle, I classified this as ‘perimeter and area’.

I also had some minor issues with particular topic classifications due to some topics appearing in more than one strand. For example, in Edexcel’s GCSE specification, fractions and percentages appear in both Number and in Ratio, Proportion and Rates of Change. In my categorisations, fraction and percentage calculations (i.e. fraction arithmetic, fractions and percentages as operators) are assigned to Number, whereas percentage change is assigned to Ratio and Proportion.

It should be noted that the proportional reasoning category only covers standard proportion and ratio-type problems – so recipes, conversions, splitting into ratios, and so on. However, many ratio problems do include fractions, percentages or both.

Third Space Learning’s online GCSE mathematics courses cover the entire GCSE mathematics curriculum. They use diagnostic testing to assess where the gaps in knowledge lie and then use this information to tailor the course specifically to the needs of each student. These individualised lessons help students to practice the areas of maths they need more support on. This helps them to develop mathematical fluency and increase their confidence going into the GCSE maths exam.

Categorising by complexity

In order to simplify the analysis process, I decided to classify the high-mark problem-solving questions by their main topic; this does lose a little bit of detail but makes it easier to look for trends across a larger data set.

I also wanted an idea of the difficulty or complexity of each question. I decided to base this broadly on the GCSE assessment objectives:

• AO1 – using and applying standard techniques (50%);
• AO2 – reasoning, interpreting and communicating mathematically (25%);
• AO3 – solving problems in mathematics and other contexts (25%).

It should be noted that this is a very rough basis, as longer or multi-step questions often award marks for more than one assessment objective. My complexity classifications can be stated as follows:

• C1 – standard procedural problems, often worth one or two marks.
• C2 – problems asking candidates to explain their reasoning, interpret information from tables, diagrams or other contexts, or ‘show that’ type questions.
• C3 – non-standard multi-step problems, often requiring the application of skills from a variety of topics, worth three, four, or more marks.

This is also not a ‘difficulty’ measure – it should be noted that ‘higher grade’ topics, such as simultaneous equations or estimating the mean, are often examined as standard procedural.

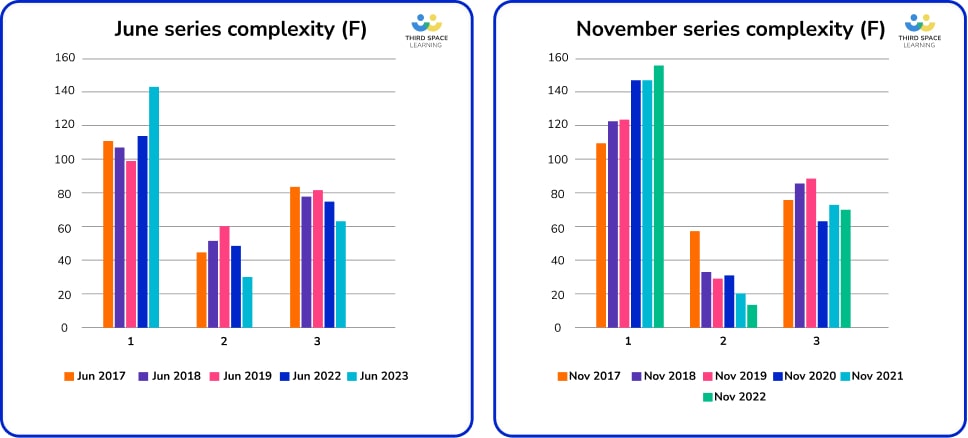
The earlier series (prior to June 2020) have roughly a third of the marks on the mark scheme allocated to C3 problems, with a higher ratio of C1 to C2 questions on the November series.

Post-2020 papers trend towards a slightly higher ratio of C1 to C3 questions; this difference is particularly noticeable on the June 2023 exam papers. It will be interesting to see whether this continues as we ease back into “exams as normal”.

Analysis by strand and topic

Despite some minor variations, pre and post-pandemic exam series have some similar and interesting patterns about the types of questions set and the complexity distribution for each topic, with some potential implications for exam preparation. 

I have split the data set out into six strands, ‘Number’, ‘Algebra’, ‘Ratio and proportion’, ‘Geometry’, ‘Probability’ and ‘Statistics’, and have added commentary on each strand below.

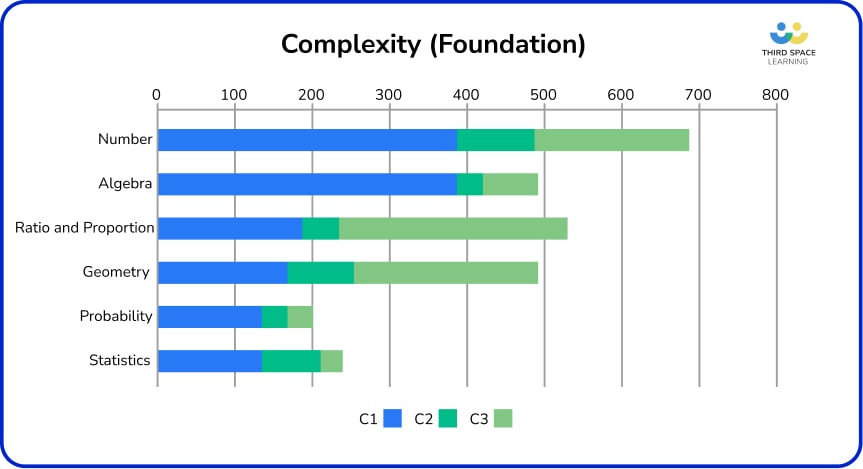
Before we get into the strand-by-strand analysis, it is interesting to look at patterns across the strands and papers as a whole. 

Particularly of note:

• There is a higher proportion of C3 non-standard, context-based problems within Geometry and Ratio and Proportion;
• There is a higher proportion of C1 procedural problems in the Algebra, Probability, and Statistics strands, with nearly all Algebra content (~80%) assessed in non-complex questions;
• The highest proportion of C2 questions involving reasoning or interpretation from charts or diagrams occurs within Statistics.

This has implications when preparing Foundation groups for sitting exams, particularly when working on the more complex or unfamiliar problems that students struggle with the most. When time is short, we can identify strands where these problems are more likely to occur (Geometry, Ratio and proportion) and invest more time in these, and perhaps less time on more complex algebra.

Foundation – number

First to note when looking at number skills at Foundation level is the large proportion of marks available for four operations calculations, with 55% of these marks for C3 questions. This topic includes integer and fraction calculations, which are more generally procedural, but also includes calculations from contexts. The takeaway from this? At Foundation, being able to work confidently with arithmetic and use this in unfamiliar problems is key.

Elsewhere, number, fractions, percentages and FDP calculations also attract a significant number of C3 marks. Percentages in particular contain 64% of the marks overall for problem-solving questions.

On the other hand, there are plenty of C1 marks up for grabs in the following topics: 

• Place value and standard form (91%)
• Ordering (88%)
• Operations and relationships (90%)
• Powers and roots (92%)
• FDP conversions (89%)
• Rounding and estimation (78%)

Rounding and estimation is 22% C2. This is mostly asking candidates to explain the effects of rounding or estimation on answers, whether an approximation will result in an over or under-estimate, and so on. Negative numbers also tends to be assessed as C2.

22% of number properties is C2; there are a few questions asking candidates to give examples or counterexamples for a given statement, such as June 2018 P1 Q11, a two-part question asking for examples to show that two statements about (a) factors of even numbers and (b) digits in odd numbers are incorrect.

What would I teach based on this data analysis?

• Context-rich problem solving with basic arithmetic, fractions, and decimals.
• Real-life contexts, including bank accounts, utility bills, wage increases, recipes and best-buys.
• Content to assure automaticity in basic number facts (identifying factors, multiples, primes, FDP equivalence and working interchangeably with these).
• Procedural work on place value, standard form, ordering, operations and relationships, powers and roots, FDP conversions, and rounding and estimation.

Foundation – algebra

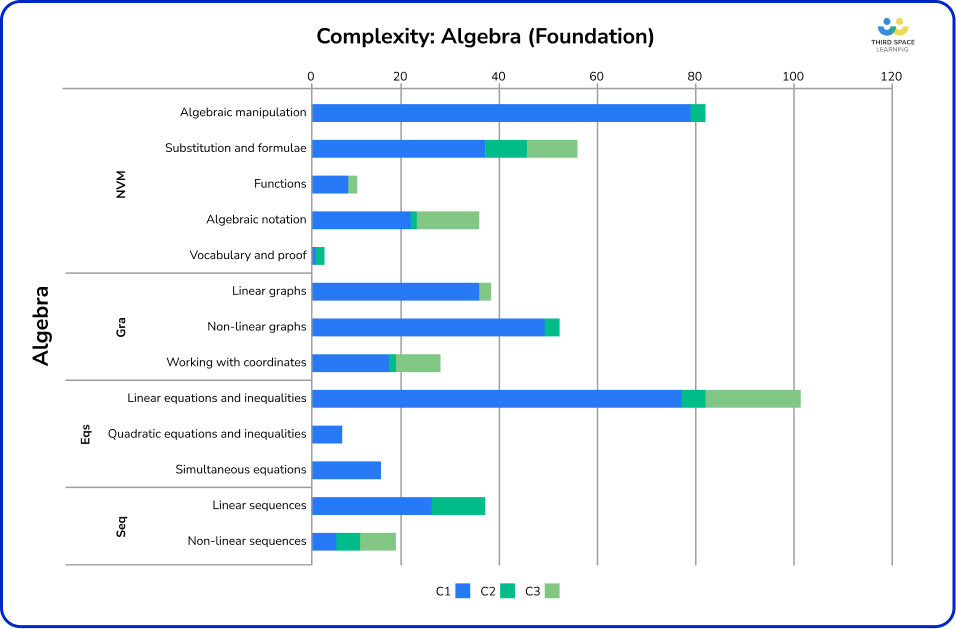
Algebra is a stark contrast to Number in terms of complexity. There are a lot of topics here that rarely, if ever, go beyond standard procedural C1 marks, and some topics do not seem to attract C2 marks either. For example, changing the subject of a formula is generally assessed procedurally at Foundation.

Algebraic manipulation, perhaps because it is the algebra toolkit of simplifying, expanding, factorising and so on, is 95% C1 marks, with no C3. A lot of the harder topics have a higher proportion of C1 marks as well: 

• Simultaneous equations (100%)
• Quadratic equations (100%) – rarely appear on Foundation
• Functions (82%)
• Linear graphs (92%), including gradients
• Non-linear graphs (94%)

In substitution and formulae, there is slightly more of a balance (62% C1, 20% C2, 13% C3). This is one of the main areas within the Foundation series, where candidates are asked to solve problems using algebra, such as looking at context-based formulae such as temperature or a formula for working out costs. The C2 marks within this topic include things like translating a worded context into an algebraic formula.

The 20% of C3 marks on linear equations were mostly for forming and solving equations from context, such as in combination with shape and angle problems.

Along with substitution and formulae, sequences attract one of the highest proportions of C2 marks (linear sequences 29%, non-linear sequences 21%). This is due to questions that ask candidates to show that n is or is not a term in the sequence, or to critique a false statement made about a sequence.

What would I teach based on this data analysis?

• Content to ensure procedural fluency with basic algebra (simplifying, expanding, factorising, laws of indices).
• Solving linear equations and inequalities, including applying these to geometrical contexts.
• Applying mathematical formulae to a variety of real-life and unfamiliar contexts.
• Sequences, functions and graphs, with focus on procedural ‘easy wins’ in straight line graph work and sequences.
• Less time on solving quadratic equations, cubic and reciprocal graphs, parallel lines and quadratic and geometric sequences. 

Foundation – ratio and proportion

Similarly to Number, a large percentage of Ratio and proportion questions were classified C3 (problem-solving). This was particularly evident within work specifically on proportion (e.g. recipes, best buys, direct and inverse proportion) and ratio (e.g. splitting into a ratio, combining ratios, solving problems using FDP and ratio).

Of the 34% of the C3 marks within units of measurement, quite a few of these were allocated to four or five-mark questions. This was partly accounted for by compound measures, which does a lot of heavy lifting at Foundation and has appeared on almost all series so far. Nearly half of the marks for compound measures came from C3 questions, meaning that the ability to problem-solve within this topic is crucial.

Topics that are more likely to be assessed procedurally include:

• Distance and speed-time graphs
• Standard measures (i.e. unit conversions)
• Proportion graphs
• Reverse percentages
• Simple and compound interest

What would I teach based on this data analysis?

• Context-rich problems requiring students to work flexibly with fractions, percentages and ratios
• Splitting into a ratio and combining ratios, particularly in context
• ‘Number-puzzle’ problems where students are asked to work out a missing amount when the other proportions are given as a mixture of fractions, percentages, and ratios (e.g. June 2018 P1 Q14)
• Place a greater focus on time, particularly misconceptions around fractions of an hour, and the use of a calculator for time problems

Foundation – geometry

Geometry was the strand that surprised me the most when completing this analysis, particularly the huge amount of C3 marks within: 

• Perimeter and area (84%);
• Volume and surface area (81%). 

Also surprising was the relatively high proportion (56%) of C3 marks for Pythagoras’ theorem and trigonometry – as one of the more challenging topics on Foundation. It is interestingly often assessed in unfamiliar contexts or in combinations with other topics. It is worth mentioning that all but one of these more complex questions have appeared so far on November series, and these topics have historically been assessed more procedurally on June papers.

As already mentioned, perimeter, area and volume was very context-heavy, with some series having all of this topic assigned as C3. These included real-life contexts such as calculating a circular lawn area and fencing for a farmer’s field, and there was also some crossover with fractions work, such as showing that a certain fraction of a shape was shaded. Many of the 2D shapes involved in area work were standard: squares, rectangles, triangles and circles, with less on compound shapes.

We have content relating to the farmer’s field question, and crossover with fractions work, in our GCSE lesson dedicated to the application of perimeter and area.

Free GCSE maths revision resources

You are free to download any resource from the extensive library of free secondary maths resources, worksheets, formula sheets and GCSE maths topic lists for AQA, Edexcel and OCR – all have been made by teacher for school like yours. Specifically designed for GCSE maths revision, the GCSE maths past papers, GCSE maths worksheets and GCSE maths questions, will help to familiarise students with the type of materials covered in the exam as well as how questions are marked.

Transformations, congruent shapes, and similar shapes feel the same as on previous specification papers; a mixture of ‘do this transformation’ and ‘describe this transformation’, with perhaps a little more work on congruence and similarity beyond just knowing the meanings of the words (see Nov 19 P1 Q29 for example). So far, all enlargements have been C1. Similarly, most vectors content is C1, with very limited problem-solving in this area.

2D shape and angle properties attracted the highest proportions of C2 marks (47%), purely because many of the questions in these topics contain the statement: ‘give reasons for your answer’. Interestingly, quite a few of the C3 marks involved solving problems with the interior and exterior angles in polygons (see Nov 19 P3 Q29 and Nov 18 P1 Q28 for examples).

It’s worth noting that the very low proportion of work at Foundation level on constructions and loci – so far this topic has only appeared three times across ten series.

What would I teach based on this data analysis?

• Include far more problem-solving and context-based problems in units on perimeter, area and volume
• Make sure that students could flexibly apply these skills to other problems, such as in the tennis court scale drawing problem mentioned above

Spend more time on scale drawing work and bearings, linking this to other topics.

Foundation – probability

Over eleven series of papers, the ‘new spec content’ (i.e. that was added in 2015), as opposed to ‘old spec content’, is strongly represented in the probability strand. Earlier on in the life of the specification, frequency trees appeared much more frequently than tree diagrams, although this balance appears to have been redressed in more recent series. It is still not unusual to see frequency trees and tree diagrams on the same series, and, in one instance, on the same paper.

Sets and Venns carried nearly 20% of the total probability marks, with completing a Venn diagram and using this to find a probability featuring very highly.

Mutually exclusive events were most likely to be examined at C3 (52%), usually with an application of ratio or more complex fractions work (see Nov 19 P3 Q16 for a good example of this). The rest of the probability content had a high proportion of C1 marks.

What would I teach based on this data analysis?

• Ensure procedural fluency in using probability scales, writing simple probabilities, and completing or reading information from tree diagrams
• Increase focus on the ‘newer’ topics, as these seem to be examined frequently at the moment
• Particularly adapt the teaching of tree diagrams to include plenty of work on frequency trees
• Give students ample experience in applying their fraction and ratio skills to unfamiliar contexts for mutually exclusive events

Foundation – statistics

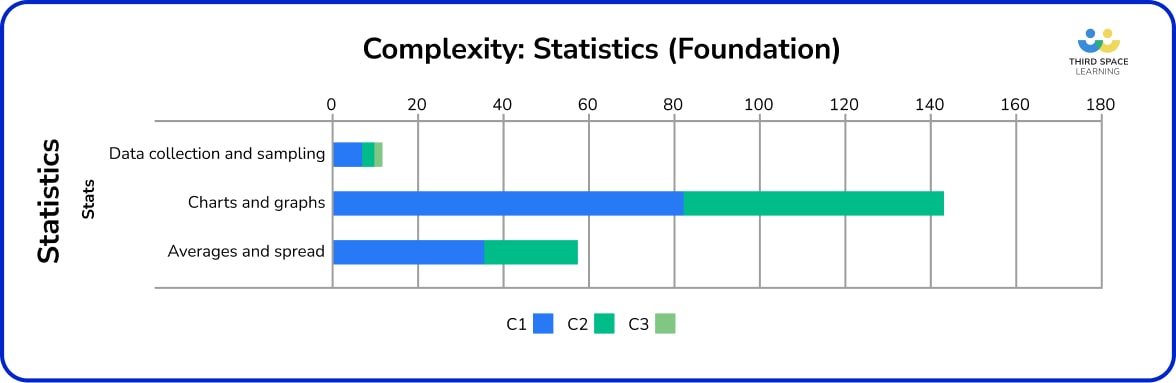
The vast majority of statistics content is C1 and C2; allocation of C3 marks in the two major topics were: 

• Charts and graphs 10%
• Averages and spread 26%

Furthermore, 64% of the statistics marks were for charts and graphs. A large proportion (39%) of work on charts and graphs is C2, meaning that candidates are almost as likely to be asked to analyse, read information from, or critique a graph or chart as they are to draw one. Pictograms seemed to feature quite highly, alongside pie charts, with slightly fewer bar charts.

In processing data, in addition to some easier C1 or C2 questions asking candidates to find an average or range from a small ungrouped set of data, estimation of the mean for grouped data comes up fairly frequently. Data collection and sampling is not examined at all on quite a few of the series.

What would I teach based on this data analysis?

Give greater emphasis to presenting data, particularly looking at the pros and cons of different types of graphs, and spotting errors in given graphs and charts.

Frequently occurring topics – first five / next five

In this section of the article I look at the most frequently occurring topics in the earlier questions on the Foundation papers. For your students, these are the simplest and easiest place to pick up a decent proportion of marks, so these are topics in which Foundation candidates should be fluent.

When students sat the first series for the new specification in 2017, Edexcel were criticised for the difficulty of some questions on Foundation, particularly the first few questions on the paper and content in the crossover or common questions to Foundation and Higher.

From June 2018 onwards, the first five questions of Edexcel Foundation papers have consisted mainly of one-mark standard procedural questions. Due to these changes, I have omitted June and November 2017 papers from this section of the analysis.

Foundation first five

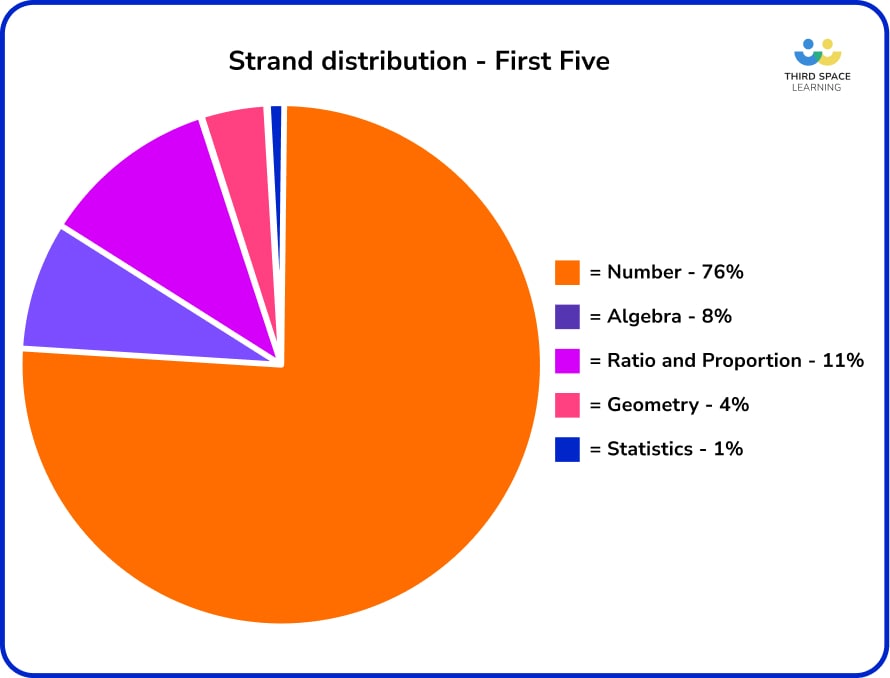
Key takeaways

• Heavy skew towards Number topics
• Some inclusion of simple Algebra and Ratio and proportion topics
• Very little Geometry or Statistics, and no Probability

Frequency of topics in the first five questions

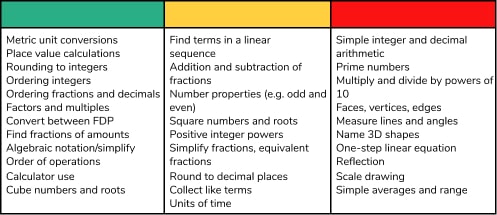
This table shows the topics that have appeared so far in the first five questions on Edexcel Foundation, traffic-light coded to indicate frequency of appearance.

Most of the topics in this list are absolutely fundamental for further work on the Foundation maths papers, so even those in red should be covered in detail.

Foundation next five

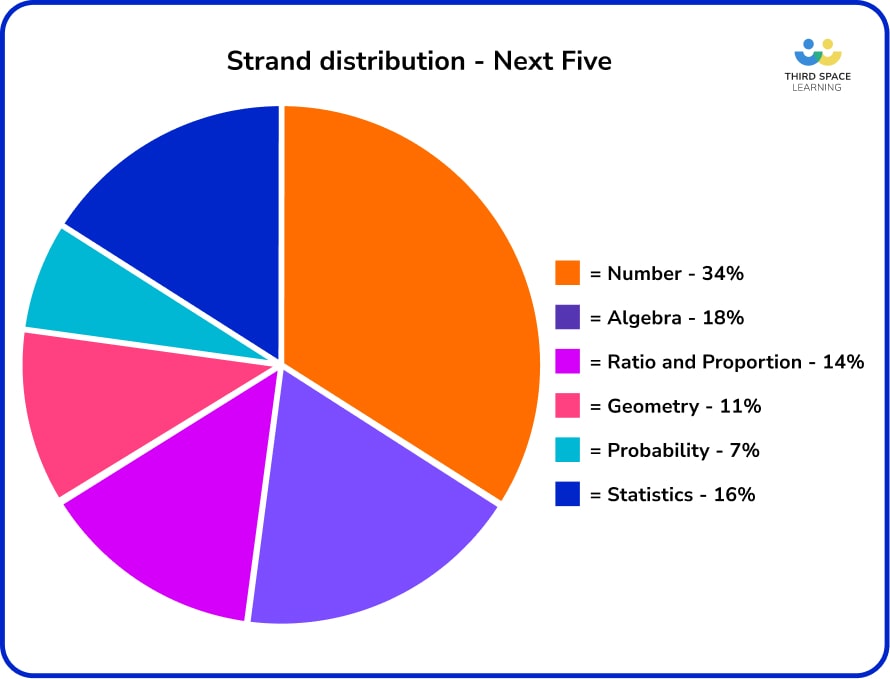
Key takeaways

• Q6-10 have a wider mix of topics, more reflective of the general proportions of the paper
• There is a greater proportion of Statistics in Q6-10 compared to the whole paper
• Most lower-grade Foundation topics appear somewhere in here on one series or another
• Many lower-grade skills are still fundamental for students to master for later applications, even if they don’t appear very frequently in this section of the paper
• There are a few very common topics or types of questions that crop up between question 6 and 10 on most papers

This table is not a fully exhaustive list, but looks at topics with three or more appearances in Q6-10.

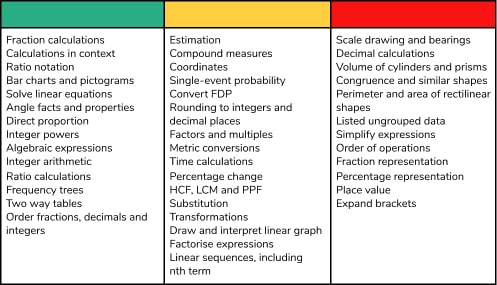
Within the most frequently occurring topics there are a few further points to draw out. First, let’s look in more detail at the most frequently occurring topic, money calculations:

• Money calculations appears on average, almost twice per series
• This topic accounts for nearly 15% of the total number of marks across all topics on all papers (for comparison, the next closest is bar charts at 6%)
• A large majority of these questions are C3 and worth 3 or 4 marks

Statistics content in these questions is relatively high, with a bar chart or pictogram (or occasionally both) on most series, along with simple average and range calculations, such as median or mode from a short list of data, and the occasional appearance of time series graphs.

Also of note is the relatively high frequency of time calculations appearances, particularly as this topic can sometimes get overlooked or assumed that students “should” be able to do. On average, there are approximately 13 marks per paper distributed over Q6-10, meaning that by the time pupils have got to Q10, they have the opportunity to gain nearly a quarter of the marks on some of the less challenging content.

Frequently occurring topics – common questions

This section of the article focuses on the common or crossover questions – those that appear on both tiers. These are the final few questions of the Foundation papers, and the first few questions of the Higher papers (although there is often an “extra” question or two at the end of the crossover content on Foundation that doesn’t appear on Higher).

On average, we’re looking at around 20-25 questions per series, split over three papers. So a typical Foundation paper will contain approximately 8 common questions (often split into parts), carrying nearly a third of the overall marks per paper.

So towards the end of the paper we’re seeing questions typically worth more marks, and it’s crucial that students aiming for grades 4-5 can access these. This section contains some suggestions for doing this.

As the changes made for the 2018 summer series onwards also affected the crossover content, I have omitted the June and November 2017 series from the data set.

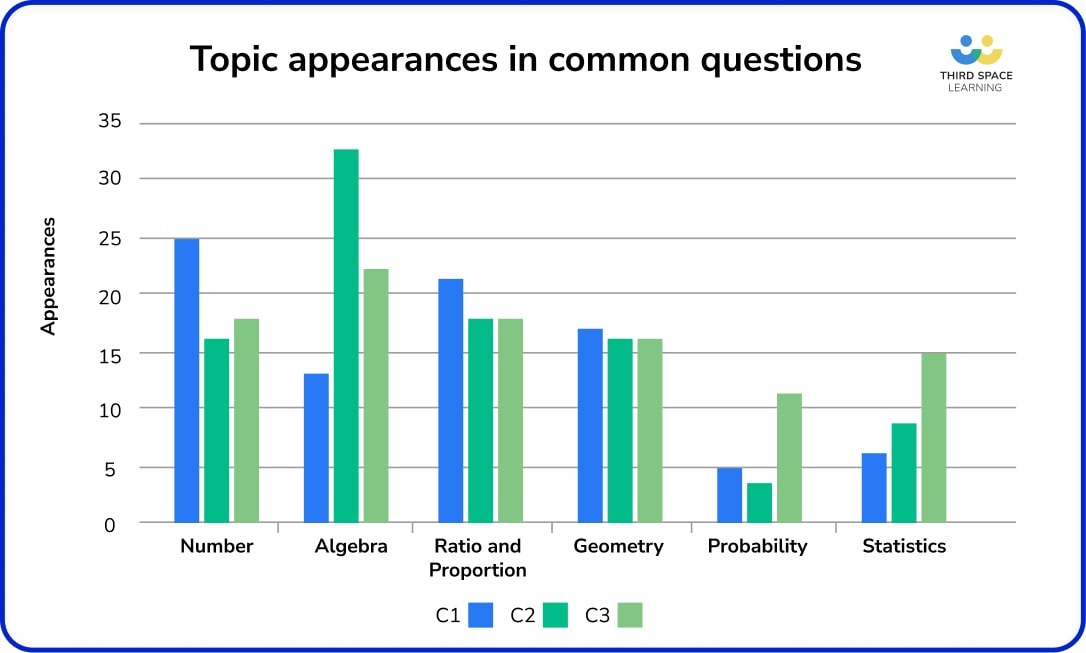
On this graph, part questions are counted as separate appearances to account for different topics assessed in different parts of questions.

Key points

• There is a higher proportion of Number content on Paper 1 (non-calculator) – this follows the pattern for the rest of the papers at both tiers, i.e. Number is assessed more frequently on the non-calculator paper.
• Compared to the rest of the average Foundation paper, there is proportionally more algebra and slightly more of other non-Number strands in the common questions – this reflects higher-level topics being assessed towards the end of the paper.
• There is lots more Probability and Statistics on Paper 3 than on the other two papers – this is probably a curiosity rather than by deliberate design, as the content on Papers 2 and 3 (calculator papers) should be functionally identical.

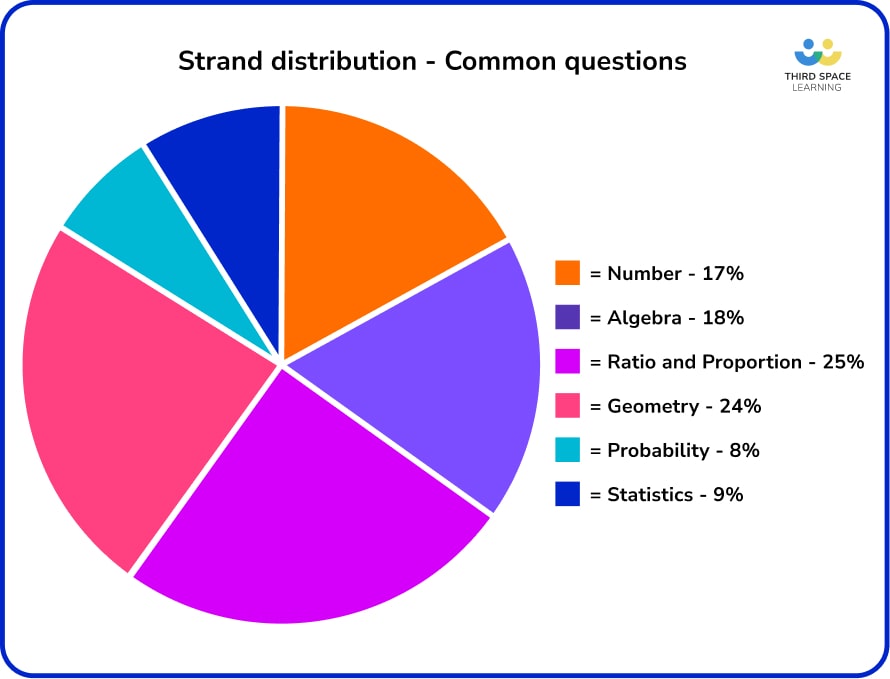
Frequently occurring topics

As we’d probably expect, some topics appear much more frequently than others in common questions. Although this shouldn’t be used to narrow down taught content, it does perhaps give an indication of where to focus final revision when time is tight.

This table contains topics with three or more appearances in common questions from June 2018 onwards. 

Topics that attract the most marks

There is considerable crossover between the topics in this list and the one above; we should expect topics which appear more often to come out with a higher average mark. Here is the list of twenty common question topics worth the most marks:

1. Speed, distance, time
2. Ratio and FDP problem solving
3. Compound interest and depreciation
4. Angles in polygons
5. Plot graph of quadratic function
6. Venn diagrams
7. Convert numbers in standard form
8. Simple laws of indices
9. Find missing sides and angles using trigonometry
10. Problem solving using perimeter and area
11. Multiply and divide decimals
12. LCM
13. Combine ratios
14. Find roots and estimate solutions from quadratic graph
15. Percentage increase and decrease
16. Adding and subtracting fractions
17. Multiply and divide fractions
18. Find percentages of amounts
19. Error intervals
20. Draw and use scatter graphs

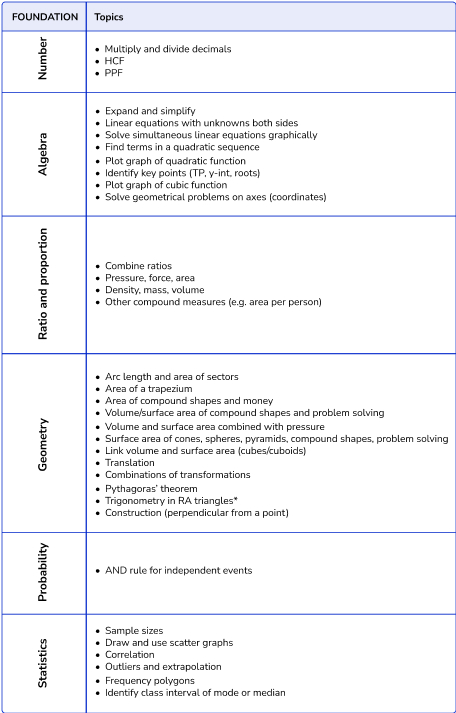
Topics that have only appeared as common questions (so far)

There are some topics that have only appeared in common questions on the Foundation papers so far – i.e. they haven’t been assessed at a lower level in the Foundation only questions on the paper. Please note again that this only looks at categorisation by the main topic, or the initial knowledge needed to gain any marks on the question.

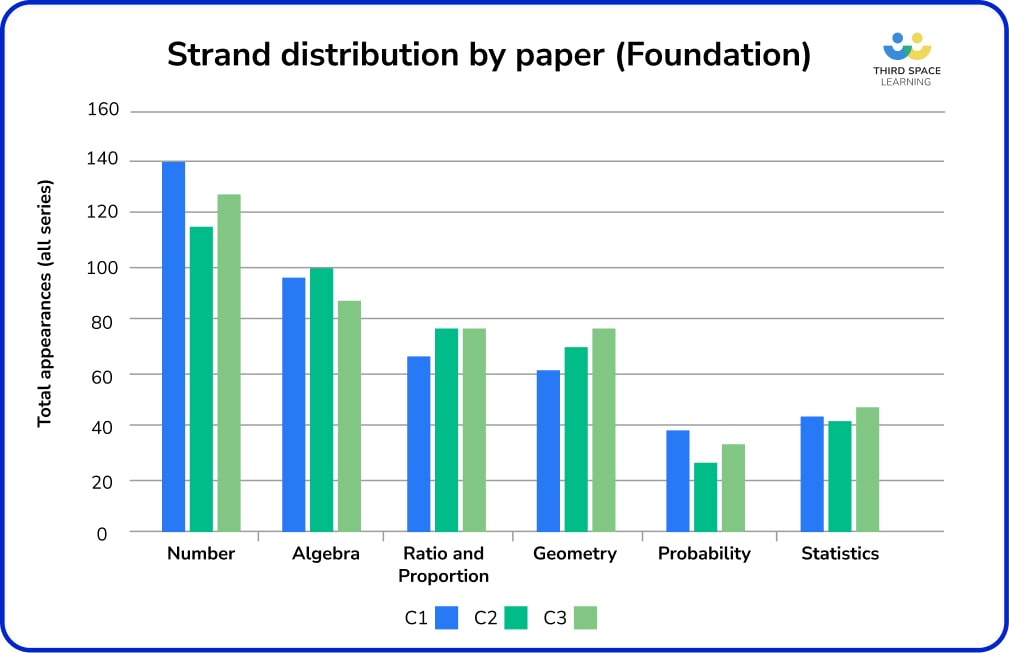
Paper 1 foundation topics

At GCSE, Paper 1 is a non-calculator paper, whereas Papers 2 and 3 allow use of a calculator. This impacts which topics are more likely to appear on Paper 1, or on Papers 2 and 3.

Broadly speaking:

• Paper 1 is significantly more arithmetic intensive, featuring topics like written calculation methods, fraction arithmetic and so on.
• There are slightly fewer questions on ratio and proportion and geometry on Paper 1.
• Topics which don’t require a calculator (e.g. transformations, drawing and interpreting charts and graphs, constructions and loci, plans and elevations) are more likely to appear on Papers 2 or 3, simply because there’s less room for them on Paper 1.

Topics with a high proportion of appearances on Paper 1

This table lists topics with four or more appearances on Paper 1. Note that any topic could theoretically appear on any paper, and many of these topics also feature highly on Papers 2 and 3.

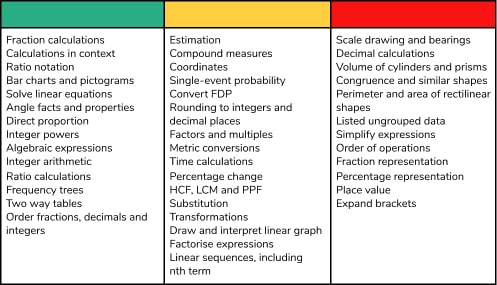
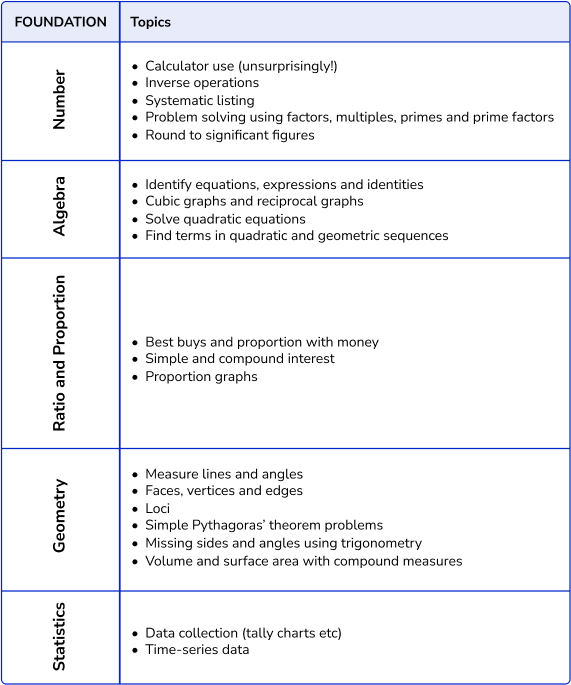
Topics with no significant appearance on Paper 1 (so far!)

This list contains topics that have not appeared on any Edexcel Foundation Paper 1 (2017-2023). Please note that many of the topics on this list have had very few appearances overall (for example, loci has only appeared once since 2017).

Final thoughts from this analysis of Edexcel maths foundation papers

Writing this blog has been very interesting from a personal perspective. Even as an experienced maths teacher, it has caused me to re-evaluate some of the ideas I had about exam preparation for the new GCSE, and to think carefully about the time weightings given to certain topics once maths revision begins.

It was clear from the Sample Assessment Materials and the messaging from exam boards that one of the goals for GCSE maths 2015 was to increase the demand in problem-solving, and also to ask more questions requiring connections to be made between topics.

The publication of Ofsted’s mathematics subject report, Coordinating Mathematical Success, in July 2023, puts significant further emphasis on the importance of teaching problem-solving skills.

Part of this needs to be allowed for in a scheme of work. For example, when teaching mutually exclusive events, give students plenty of problems requiring an understanding of fractions, decimals, percentages and ratios. This cannot be done as a quick fix at the end.

However, with regard to final exam preparation, there are a few things to consider. A significant amount of algebra, statistics, and probability is procedural C1 content, so this suggests there is less value in spending a lot of vital exam preparation time on rich problems for these topics.

Furthermore, we need to consider the frequency at which topics like quadratic equations or factorising quadratics appear on Foundation papers. For borderline grade 4/5 candidates, we may in fact be better off spending more time on problem-solving using proportional reasoning or shape, returning to the low-frequency procedural topics in the very final weeks.

What students clearly do need is plenty of practice on number skills, so these are not limiting their ability to access the context-based problems, and plenty of time and opportunity to work with number and proportion skills in a wide variety of contexts.

They also need lots of time on context-based shape work, particularly perimeter, area and volume, and 2D shape and angle properties. This could in part be achieved by using all of the papers analysed above as practice papers, mock exams or elements of a worksheet, both in the classroom and at home.

Finally, it’s important to assess all of this with regard to your specific setting and students. For some, a grade 3 represents an outstanding achievement, and a careful selection of topics to focus on may help a student to achieve this.

Other students may have ‘dropped down’ to Foundation at the last minute, so might need some rapid intervention to boost number and proportional reasoning problem-solving skills, particularly C3 questions, as GCSE maths revision in a Higher tier group may not have targeted these areas.

READ MORE

• How To Revise For GCSE
• Exam Techniques
• GCSE grade boundaries