Exchange rates

GCSE Tutoring Programme

"Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring."

Teacher-trusted tutoring

In order to access this I need to be confident with:

Operations involving decimal numbers Ratio Proportion

This topic is relevant for:

GCSE Maths Ratio and Proportion Conversion Graphs

Exchange Rates

Here we will learn about how to work out exchange rates, including how to convert between British pounds and foreign currencies and read conversion graphs.

There are also how to work out exchange rate worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is an exchange rate?

An exchange rate is the rate at which the money of one country can be exchanged for the money of another country. It can also be referred to as a foreign exchange rate and be seen as the price of one currency expressed in terms of another currency.

In the United Kingdom our currency is the British pound or pound sterling. The exchange rate tells us the value of $£1$ in terms of a foreign country’s currency. Different countries have different currencies.

For example,

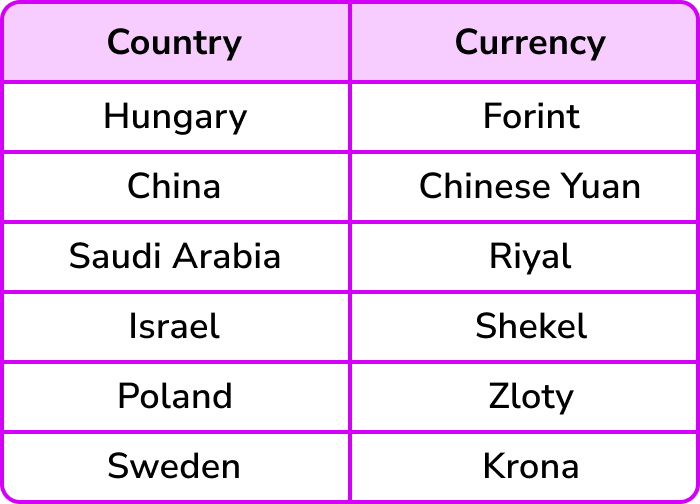
The exchange rates are constantly changing depending on the country’s economic status. It is the role of the central bank to monitor these fluctuations and adjust the country’s monetary policy accordingly.

Using the exchange rate we can convert between pounds and foreign currencies. To do this we need to either multiply or divide the quantity we are trying to convert with the exchange rate.

To convert from British pounds to a foreign currency you must multiply by the exchange rate.

For example,

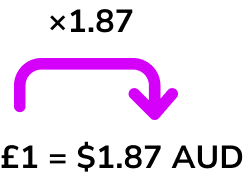
So $£150$ would be $\$276$ because $150 \times 1.87=276.$

For example,

To convert from a foreign currency into British pounds you must divide by the exchange rate.

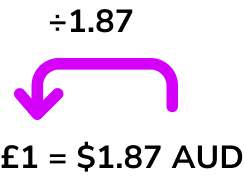
So, $\$430$ would be $£229.95$ because $430 \div 1.87=229.95$ (to $2$ dp).

A GCSE Mathematics question may ask you to convert between other currencies. It will normally give the exchange rate in a form similar to a $1:n$ ratio.

E.g.

Dollars to Euros

\$1 = €0.92

To convert from the currency given as $“1”$ we would multiply by the exchange rate.

To convert to the currency given as $“1”$ we would divide by the exchange rate.

What is an exchange rate?

How to work out exchange rates

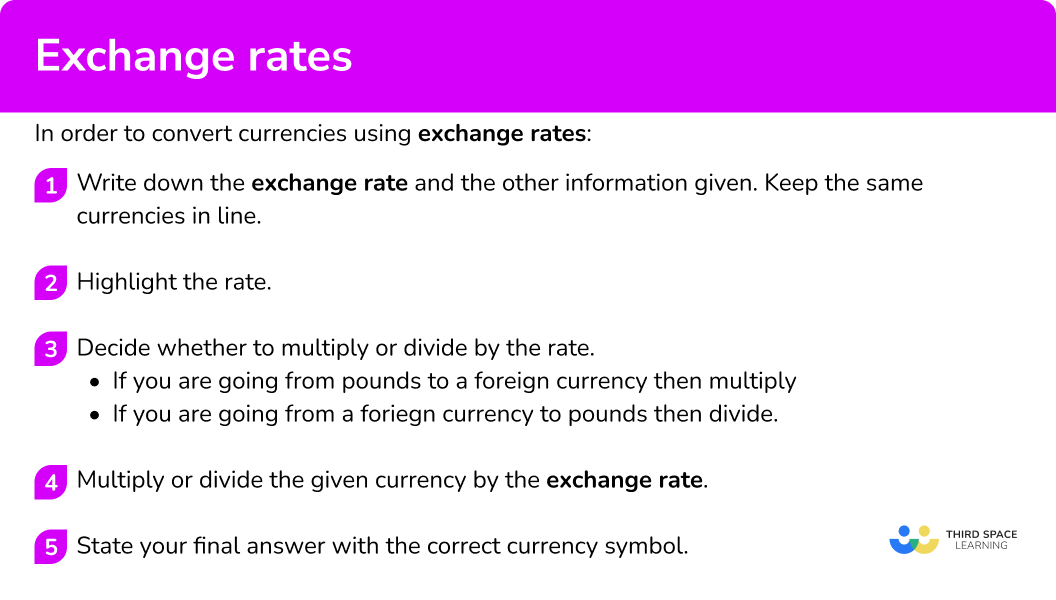
In order to convert currencies using exchange rates:

Write down the exchange rate and the other information given. Keep the same currencies in line.
Highlight the rate.
Decide whether to multiply or divide by the rate.
If you are going from the $“1”$ to the other currency then multiply.
If you are going to the $“1”$ from the other currency then divide.
Multiply or divide the given currency by the exchange rate.
State your final answer with the correct currency symbol.

Explain how to work out exchange rates

How to work out exchange rates worksheet

Get your free how to work out exchange rates worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

How to work out exchange rates worksheet

Get your free how to work out exchange rates worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

How to work out exchange rates examples

Example 1: converting from pounds to a foreign currency

Given the exchange rate between pound and Australian dollars is $£1 = \$1.87$ , convert $£70$ to Australian dollars.

Write down the exchange rate and the other information given. Keep the same currencies in line.

In the question we are given,

\begin{aligned} &£1 = \$1.87 \\\\ &£70^* \end{aligned}

*Make sure to line up the $£1$ and $£70$ as they are the same currency.

2Highlight the rate.

\begin{aligned} & £1 = \colorbox{yellow}{$\$1.87^{*}$} \\\\ &£70 \end{aligned}

*This tells us that every $£1$ is equal to $\$1.87.$

3Decide whether to multiply or divide by the rate.

If you are going from the $“1”$ to the other currency then multiply.

If you are going to the $“1”$ from the other currency then divide.

In this case we are going from pounds to Australian dollars so we need to multiply.

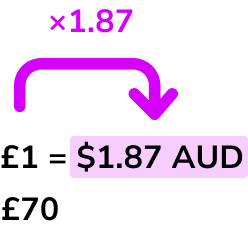
4Multiply or divide the given currency by the exchange rate.

Based on Step $3$ , we need to multiply $£70$ by the rate which is $\$1.87.$

70 \times 1.87=130.90

5State your final answer with the correct currency symbol.

\colorbox{yellow}{$\bf{\$130.90} \textbf{ (Australian dollars)}$}

Example 2: converting from a foreign currency into pounds

Sally just came back from a visit to the Philippines. In her purse she still had $₱135$ (peso) which she wanted to convert back into pounds. A quick check on her foreign exchange app told her the current exchange rate was $£1 = ₱69.$ How many pounds will she get?

Write down the exchange rate and the other information given. Keep the same currencies in line.

In the question we are given,

$\begin{aligned} £1 = \ &₱69 \\\\ &₱135^* \end{aligned}$

*Make sure to line up the $£1$ and $₱135$ as they are the same currency.

Highlight the rate.

\begin{aligned} £1 = & \colorbox{yellow}{$ ₱69^{*}$} \\\\ & \ ₱135 \end{aligned}

*This tells us that every $£1$ is equal to $₱69.$

Decide whether to multiply or divide by the rate.

If you are going from the $“1”$ to the other currency then multiply.

If you are going to the $“1”$ from the other currency then divide.

In this case we are going from pesos to pound sterling so we need to divide.

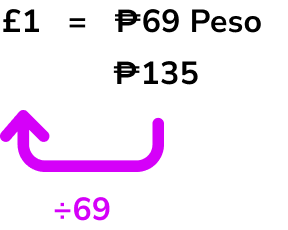
Multiply or divide the given currency by the exchange rate.

Based on Step $3$ , we need to divide $₱135$ by the rate which is $₱69.$

$135 \div 69=1.9565...$

State your final answer with the correct currency symbol.

\colorbox{yellow}{$\bf{£1.96} \textbf{ (to the nearest pence)}$}

Example 3: comparing different currencies

In the USA a computer costs $\$790.$ The same mobile phone costs $£575$ in the UK. Given that the current exchange rate is $£1 = 1.35 \ USD,$ where is the mobile phone cheaper?

Write down the exchange rate and the other information given. Keep the same currencies in line.

In the question we are given,

$£1 = \$1.35 \ USD$

We are also asked to compare $£575$ and $\$790.$ To compare them, we must convert them to the same currency. In this case, we can choose to either convert $£575$ into US dollars or $\$790$ into great British pounds $(GBP).$

For the purposes of this question, we will convert $£575$ to American dollars.

$\begin{aligned} & £1 = \$1.35 \\\\ &£575^* \end{aligned}$

*Make sure to line up the $£1$ and $£575$ as they are the same currency.

Highlight the rate.

\begin{aligned} & £1 = \colorbox{yellow}{$ \$1.35 $} \\\\ & £575 \end{aligned}

*This tells us that every $£1$ is equal to $\$1.35.$

Decide whether to multiply or divide by the rate.

If you are going from the $“1”$ to the other currency then multiply.

If you are going to the $“1”$ from the other currency then divide.

In this case we are going from American dollars to British pounds so we need to multiply.

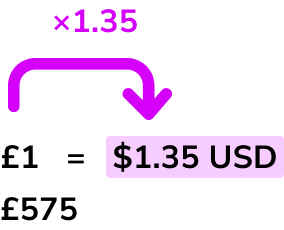
Multiply or divide the given currency by the exchange rate.

Based on Step $3$ , we need to multiply $\$575$ by the rate which is $\$1.35.$

$575 \times 1.35=776.25$

State your final answer with the correct currency symbol.

The computer costs $\$776.25$ in England and $\$790$ in the United States.

Therefore, it is cheaper to buy the computer in the UK.

Example 4: worded question

Bonnie is flying home from Spain. She buys some food and drink on the plane.

Price List

Crisps £2.50

Chocolate Bar £1.75

Pretzels £1.50

Bottled water £3.70

Tea £2

Juice £1.80

Bonnie buys $2$ chocolate bars, $2$ pretzels and a bottle of water. The exchange rate is $£1 = €1.17$ (euros). She decided to use her leftover euros from her holiday to pay for her bill. Work out the cost of her bill in euros.

Write down the exchange rate and the other information given. Keep the same currencies in line.

In the question we are given,

$£1 = €1.17 \ euros$

We are also told that Bonnie buys $2$ chocolate bars, $2$ pretzels and a bottle of water.

We need to add together the cost of these items.

Total cost $= 2$ chocolate bars $+ \ 2$ pretzels $+ \ 1$ bottle of water

$\begin{aligned} &=(2 \times 1.75)+(2 \times 1.50)+3.70 \\\\ &=£10.20 \end{aligned}$

Since this total is in pounds we can put it under the pounds side of the exchange rate.

$\begin{aligned} &£1 = €1.17 \\\\ &£10.20 \end{aligned}$

Highlight the rate.

\begin{aligned} & £1 = \colorbox{yellow}{$ €1.17^* $} \\\\ & £10.20 \end{aligned}

*This tells us that every $£1$ is equal to $€1.17.$

Decide whether to multiply or divide by the rate.

If you are going from the $“1”$ to the other currency then multiply.

If you are going to the $“1”$ from the other currency then divide.

In this case we are going from pound sterling to euros so we need to multiply.

Multiply or divide the given currency by the exchange rate.

Based on Step $3$ , we need to multiply $£10.20$ by the rate which is $€1.17.$

$10.20 \times 1.17=11.93$

State your final answer with the correct currency symbol.

The total cost of her bill in euros is $\colorbox{yellow}{$ €11.93.$}$

Example 5: worded question

Samantha is planning a holiday to Turkey.

She needs to exchange some of her British pounds into Turkish lira.

The bank only stock $₺100$ notes.

Samantha only wants to exchange up to $£550$ into Turkish lira.

She wants to get as many $₺100$ notes as possible.

The current exchange rate is $£1=₺12.81.$

How many $₺100$ notes should she get?

Write down the exchange rate and the other information given. Keep the same currencies in line.

In the question we are given,

$\begin{aligned} &£1=₺12.81 \\\\ &£550^* \end{aligned}$

*Make sure to line up the $£1$ and $£550$ as they are the same currency.

Highlight the rate.

\begin{aligned} & £1 = \colorbox{yellow}{$ ₺12.81^{*} $} \\\\ & £550 \end{aligned}

*This tells us that every $£1$ is equal to $₺12.81.$

Decide whether to multiply or divide by the rate.

If you are going from the $“1”$ to the other currency then multiply.

If you are going to the $“1”$ from the other currency then divide.

In this case we are going from pounds to Turkish lira so we need to multiply.

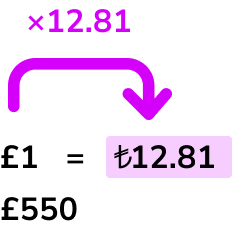
Multiply or divide the given currency by the exchange rate.

Based on Step $3$ , we need to multiply $£550$ by the rate which is $₺12.81.$

$550 \times 12.81=6655$

State your final answer with the correct currency symbol.

Samantha would get $₺6655$ if she converted $£550.$ The bank only stock $₺100$ notes so we need to divide $₺6655$ by $100$ to see how many notes she would get.

This would give us $₺66.55.$ Since you cannot get $0.55$ of a note, this value can be rounded to $66.$

She would get $\colorbox{yellow}{$\bf{66 \ ₺100} $}$ notes.

Example 6: conversion graphs

Use the graph below to answer the following questions.

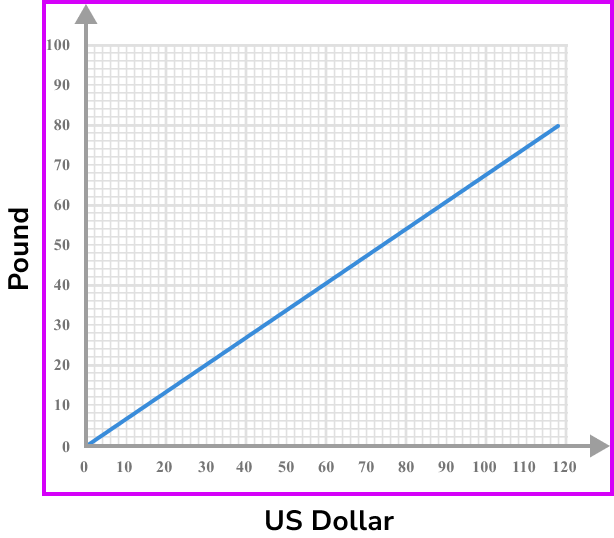
a) Change $\$30$ USD to pounds.

b) Change $£65$ to US dollars.

c) Change $£100$ to US dollars.

a Change $\bf{\$30}$ USD to pounds.

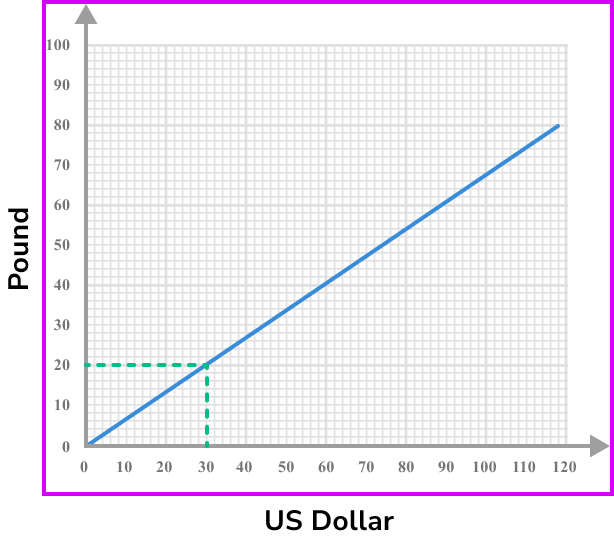
Draw a line going up from $\$30$ until you hit the line, then look across to the vertical axis and read the value.

In this case $\$30 = £20.$

b Change $\bf{£65}$ to US dollars.

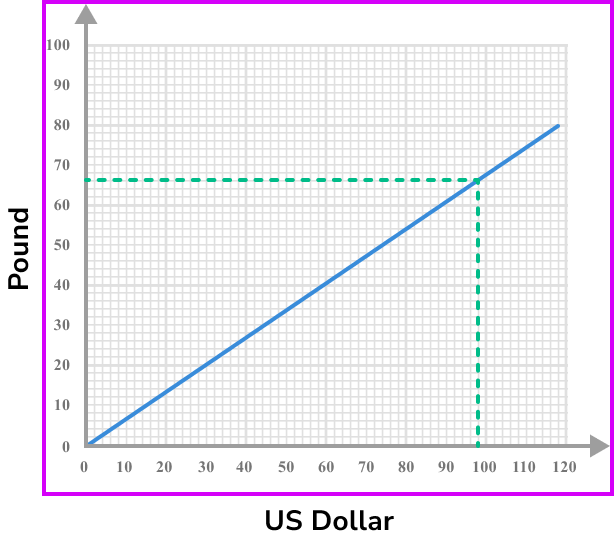
Draw a line going across $£65$ until you hit the line, then look down to the horizontal axis and read the value.

In this case $£65 = \$96$ USD.

c Change $\bf{£100}$ to US dollars.

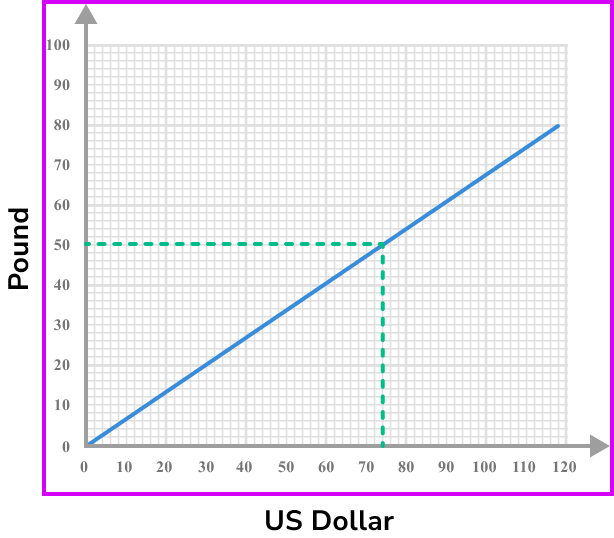
Since the graph does not extend to $£100,$ we need to use the values that we know and use them to calculate how many USD $£100$ is equal to.

Draw a line going across from $\$50$ USD to the line and down to $\$74.$

Since we know that

$£50 = \$74$ .

If we multiply both sides by $2$ we get

$£100 = \$148$ USD.

Step-by-step guide: Conversion graphs

Common misconceptions

Multiply or divide by the exchange rate

Pupils may get confused whether they need to multiply or divide by the exchange rate. Remember, If you are going from the $“1”$ to the other currency then multiply. If you are going to the $“1”$ from the other currency then divide.

Lining up the same currencies together

When given information in the question be careful to check what currency the values are in and line up accordingly. If you mix up the currencies it will be difficult to know whether to multiply or divide by the exchange rate.

Rounding

When rounding, it is usual that we need to round to $2$ decimal places. For example, if calculating British pounds we get $231.7851,$ the final answer would be $£217.79.$ The $2$ decimal places would be the pence.

Practice how to work out exchange rates questions

\$175.50

\$46.15

\$91.95

£46.15

Multiply $£90$ by the exchange rate $1.95.$

90\times 1.95=175.5

This would be $£175.70$ .

£48.50

£515,500

£5103.10

£4896.90

Divide $₹5000$ by the exchange rate $103.10.$

5000\div 103.10=48.4966…

This would be $£48.50$ to the nearest pence.

The UK by $¥155$

China by $¥2200$

China by $¥155$

The price is the same in both countries

Convert $£300$ into Chinese Yuan by multiplying by $8.85.$

300\times 8.85=2655

This would be more expensive than the price in China by $¥155.$

€447

€351.97

€546.10

€567.69

Add up the prices.

\pounds 150 + \pounds 280+ \pounds 17=\pounds 447

Multiply the total by the exchange rate $1.27.$

447\times 1.27=567.69

This gives $€567.69$ .

5

4

45

4595

Multiply $=£100$ by $45.95,$ then divide by $1000$ to determine how many notes she should get.

100\times 45.95=4595

This gives $฿4595.$

We then need to work out the number of $฿1000$ notes.

4595\div 1000=4.595

So Sangits would be able to get $4 \ ฿1000$ notes.

How to work out exchange rates GCSE questions

1. Samantha goes on holiday to Japan.

She exchanged $£850$ into Japanese yen.

The exchange rate was $£1 = JP¥156.88$ .

(a) Change $£850$ into Japanese yen.

(b) When she returned she had $JP¥180$ left which she wanted to exchange back into pounds.

She checked the currency exchange rates app on her phone and found that the new rate was $£1 = JP¥157.22.$

Change $JP¥180$ into British pounds.

(4 marks)

Show answer

(a)
$850 \times 156.88$

(1)

JP¥133348

(1)

(b)
$180 \div 157.22$

(1)

£1.14

(1)

2. (a) On a flight back to London from Hong Kong, Jackie purchased the following items from the in-flight dining options.

A blueberry muffin costing $£2.50$
A diet coke costing $£3.50$
A tuna sandwich costing $£5.50$

She paid part of the bill with the $HK\$60$ left from her holiday and paid the rest in British pounds.

The exchange rate between the British pound and the Hong Kong dollar is $£1= HK\$10.68.$

How much does Jackie pay in British pounds?

(b) When paying the bill Jackie realised that there was a $10\%$ service charge on her bill.

What was her total bill in Hong Kong dollars (HKD)? Give your answer to the nearest Hong Kong dollar.

(6 marks)

Show answer

(a)

$2.50+3.50+5.50=£11.50$

(1)

60 \div 10.68=£5.62

(1)

11.50-5.62 = £5.88

(1)

(b)

$11.50 \times 1.10=£12.65$

(1)

£12.65 \times 10.68=135.102

(1)

HK\$135

(1)

3. Paula goes on holiday to Canada.

She exchanged $£550$ into Canadian dollars.

The exchange rate was $£1 = \$1.70$ .

(a) Change $£550$ into Canadian dollars.

(b) While in Canada Paula noticed a blazer she really liked. The blazer cost $\$132 \ CDN.$ She had seen the exact same blazer in England for $£79.$

Using the same exchange rate as (a), determine where the blazer is cheaper and by how much.

(5 marks)

Show answer

(a)

550 \times 1.70

(1)

\$935

(1)

(b)

79 \times 1.70=\$134.30

(1)

134.30-132=\$2.30

(1)

The blazer is cheaper in Canada by $\$2.30$ .

(1)

Alternatively

132\div 1.70= \pounds 77.65

(1)

79-77.65=£1.35

(1)

The blazer is cheaper in Canada by $£1.35$ .

(1)

Learning checklist

You have now learned how to:

Convert between related units (prices, currencies) in numerical contexts

Interpret graphs that illustrate direct proportion, such as currencies

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Introduction

What is an exchange rate?

How to work out exchange rates

How to work out exchange rates worksheet

How to work out exchange rates examples

↓

Example 1: converting from pounds to a foreign currency Example 2: converting from a foreign currency into pounds Example 3: comparing different currencies Example 4: worded question Example 5: worded question Example 6: conversion graphs

Common misconceptions

Practice how to work out exchange rates questions

How to work out exchange rates GCSE questions

Learning checklist

Next lessons

Still stuck